Haddad W.M. Nonlinear Dynamical Systems and Control: A Lyapunov-Based Approach

Подождите немного. Документ загружается.

NonlinearBook10pt November 20, 2007

ROBUST NONLINEAR CONTROL 715

satisﬁes x(t) → 0 as t → ∞ with σ(u) = M

u}.

Problem 11.33. Consider the controlled dynamical system

˙x(t) = Ax(t) + Bσ(u(t)), x(0) = x

, t ≥ 0, (11.325)

where A ∈ R

n×n

, B ∈ R

n×m

, and σ(·) ∈ M, where M is given by (11.313),

and u(t) ∈ R

for all t ≥ 0, w ith performance functional

J(x

, u(·)) =

∞

(t)R

x(t) + 2x

(t)R

u(t) + u

(t)R

u(t)]dt, (11.326)

where R

∈ R

n×n

, R

∈ R

n×m

, and R

∈ R

m×m

such that R

> 0, R

> 0,

and R

− R

−1

≥ 0. Assume there exists an n × n positive-deﬁnite

matrix P satisfying

0 = A

P + P A + R

+ P BB

P − P

−1

, (11.327)

and let K be given by

K = −R

−1

, (11.328)

where P

△

P +R

and R

△

= R

M. Show that, with

u = Kx, the zero solution x(t) ≡ 0 to (11.325) is globally asymptotically

stable for all σ(·) ∈ M. If, in addition, σ(·) ∈ M

, where

△

= {σ : R

→ R

: M

≤ σ

(u)u

≤ M

≤ u

, i = 1, ..., m}, (11.329)

where u

< 0 and u

> 0, i = 1, . . . , m, are given, then show that the zero

solution x(t) ≡ 0 to (11.325) with u = Kx is locally asymptotically s table,

and

△

= {x ∈ B : x

P x ≤ V

}, (11.330)

where B

△

i=1

, B

△

= {x ∈ R

: u

≤ φ

(x) ≤ u

} and

= min



min

i=1,...,m

row

(K)P

−1

row

(K)

, min

i=1,...,m

row

(K)P

−1

row

(K)



(11.331)

is a subset of the domain of attraction for (11.325). Furth ermore, show that

the performance functional (11.326) satisﬁes

sup

σ(·)∈M

J(x

, φ(x(·))) ≤ J(x

, φ(x(·))) = V (x

), (11.332)

where

J(x

, u(·))

△

∞

x + 2x

u + u

u + (

Mu + B

P x)

·(

Mu + B

P x)]dt, (11.333)

where u(·) is admissible, and x(t), t ≥ 0, solves (11.325) with σ(u) = M

In addition, show that the performance functional (11.333) is minimized in

NonlinearBook10pt November 20, 2007

716 CHAPTER 11

the sense that

J(x

, φ(x(·))) = min

u(·)∈S(x

)

J(x

, u(·)), (11.334)

where

S(x

)

△

= {u(·) : u(·) is admissible and x(·) given by (11.325)

satisﬁes x(t) → 0 as t → ∞ with σ(u) = M

u}.

11.11 Notes and References

Quadratic Lyapunov functions form the basis for linear-quadratic control

theory and have provided one of the principal tools for robust analysis and

synthesis [25–27,29,41,59,83,99,115,131,194,195,200,233,237,243,266,267,

300,312,313,337,347,352,356,401,416,430,442,447,471,472,480]. Among the

earliest Lyapunov function frameworks for linear robus t control were those

developed by Michael and Merriam [313], Chang and Peng [83], Horisberger

and Belanger [200], Vinkler and Wood [447], and Leitmann [266, 267]. In

contrast to the above cited literature, the work of Chang and Peng [83]

additionally addressed bounds on worst-case quadratic performance within

full-state feedback control design. Extensions of the guaranteed cost control

approach of Chang and Peng to full- and reduced-order dynamic control were

addressed by Bernstein [42], Bernstein and Haddad [48,51], and Haddad and

Bernstein [146]. A systematic treatment of quadratic Lyapunov bounds is

given by Bernstein and Haddad [50]. More recent results involving shifted

quadratic guaranteed cost bounds for robust stability and performan ce are

developed by Haddad, Chellaboina, and Bernstein [161], and Bernstein and

Osburn [53].

Connections between absolute stability theory and robust control were

ﬁrst noted by Popov [363] where the notion of hyperstability was used

to describe nonlinear robustness implicit in the inequalities of Lyapunov

stability theory. The input-output functional analysis approach to absolute

stability theory was identiﬁed by Zames [476] as a way of capturing

system uncertainty. A more modern treatment of the connections of

absolute stability and stability robustness was given by Safonov [377] using

topological separation of graphs of feedback operators. More recently,

explicit connections between absolute stability theory and robust stability

and performance were given by Haddad and Bernstein [147, 148, 151]

and Haddad, How, Hall, and Bernstein [172] using ﬁxed and parameter-

dependent Lyapunov functions.

Even though the theory of nonlinear robust control for nonlinear uncer-

tain systems with parametric uncertainty remains relatively undeveloped in

comparison to linear r ob ust control, notable exceptions include the work of

NonlinearBook10pt November 20, 2007

ROBUST NONLINEAR CONTROL 717

Spong [412], Basar and Bernhard [31], van der Schaft [438,439,441], Freeman

and Kokotovi´c [128], Haddad, Chellaboina, and Fausz [164], and Haddad,

Chellaboina, Fausz, and Leonessa [166]. The nonlinear-nonquadratic robust

control framework presented in this chapter is adopted from Haddad,

Chellaboina, an d Fausz [164] and Haddad, Chellaboina, Fau sz, and Leonessa

[166].

NonlinearBook10pt November 20, 2007

Chapter Twelve

Structured Parametric Uncertainty

and Parameter-Dependent

Lyapunov Functions

12.1 Introduction

The analysis and synthesis of robust feedback controllers entails a fu n-

damental distinction between parametric and nonparametric uncertainty.

Parametric uncertainty refers to plant uncertainty that is modeled as

constant real parameters, whereas nonparametric uncertainty refers to

uncertain transfer function gains that may be modeled as complex frequency-

dependent quantities or nonlinear dynamic operators. In the time domain,

nonparametric uncertainty is manifested as u ncertain real parameters that

may be time varying.

The distinction between parametric and nonparametric uncertainty

is critical to the achievab le performance of feedback control s y s tems. For

example, in the problem of vibration suppr ession for ﬂexible structures,

if stiﬀness matrix uncertainty is modeled as nonparametric uncertainty,

then perturbations to the damping matrix will inadvertently be allowed.

Predictions of stability and performance for given feedback gains will con-

sequently be extremely conservative, thus limiting achievable performance

[52]. Alternatively, this problem can be viewed by considering the classical

analysis of Hill’s equation (e.g., the Mathieu equation) which shows that

time-varying p arameter variations can destabilize a system even when the

parameter variations are conﬁned to a region in which constant variations

are nondestabilizing. Consequently, a f eedback controller designed for time-

varying parameter variations will unnecessarily sacriﬁce performance when

the uncertain real parameters are actually constant.

To further illuminate the above discuss ion consider the nonlinear

uncertain system

˙x(t) = f

(x(t)) + ∆f(x(t)), x(0) = x

, t ≥ 0, (12.1)

NonlinearBook10pt November 20, 2007

720 CHAPTER 12

where x(t) ∈ R

is th e system state vector, f

: R

→ R

satisﬁes f

(0) = 0,

and ∆f (·) ∈ ∆ ⊂ {∆f : R

→ R

: ∆f(0) = 0}, where ∆ is a set of sys tem

perturbations. To determine whether the zero solution x(t) ≡ 0 to (12.1)

remains stable, one can construct functions V : R

→ R and Γ : R

→ R,

where V (·) is continuously diﬀerentiable, such that V (0) = 0, V (x) > 0,

x ∈ R

, x 6= 0, V (x) → ∞ as kxk → ∞,

′

(x)∆f(x) ≤ Γ(x), x ∈ R

, ∆f(·) ∈ ∆, (12.2)

′

(x)f

(x) + Γ(x) < 0, x ∈ R

, x 6= 0, (12.3)

L(x) + V

′

(x)f

(x) + Γ(x) = 0, x ∈ R

, (12.4)

where L : R

→ R and satisﬁes L(x) > 0, x ∈ R

, x 6= 0. Now, it follows

from Corollary 11.1 that the zero solution x(t) ≡ 0 to (12.1) is globally

asymptotically stable for all ∆f(·) ∈ ∆, and the performance functional

∆f

) =

∞

L(x(t))dt, (12.5)

satisﬁes the boun d sup

∆f(·)∈∆

∆f

) ≤ V (x

), x

∈ R

As shown in Section 11.3, although the Lyapun ov-function-based

framework discussed above applies to pr oblems in which f(x) is perturbed

by an uncertain function ∆f(x), a reinterpretation of these bounds yields

standard nonlinear system theoretic criteria. For example, the bounding

function (11.26) form s the basis f or nonlinear nonexpansivity theory while

the bounding function (11.28) forms the basis for nonlinear passivity theory.

Although not immediately evident, a defect of the above framework is

the fact that stability is guaranteed even if ∆f is an explicit function of

t. This observation follows from the fact that the Lyapunov derivative

V (x(t))

△

= V

′

(x(t))[f(x(t)) + ∆f (t, x(t))] need only be negative for each

ﬁxed value of time t. Although this feature is d esirable if ∆f is time-

varying, as discussed above, it leads to conservatism when ∆f is actually

time invariant. This defect, however, can be remedied as in the linear robust

control literatur e [145, 147, 151] by utilizing an alternative approach based

upon parameter-dependent Lyapunov functions. The idea behind parameter-

dependent Lyapunov fun ctions is to allow the Lyapunov function to be a

function of the uncertainty ∆f . In the usu al case, V (x) is a ﬁxed function,

whereas a parameter-dependent Lyapunov function represents a family of

Lyapunov functions.

To demonstrate parameter-dependent Lyapunov functions for robust

analysis of nonlinear systems in a manner consistent with the above

discussion, consider the Lyapunov function

V (x) = V

(x) + V

∆f

(x), (12.6)

where V : R

→ R satisﬁes the above conditions with (12.2)–(12.4) replaced

NonlinearBook10pt November 20, 2007

STRUCTURED PARAMETRIC UNCERTAINTY 721

′

(x)∆f(x) ≤ Γ(x) −V

′

∆f

(x)(f

(x) + ∆f(x)), x ∈ R

, ∆f(·) ∈ ∆,

(12.7)

′

(x)f

(x) + Γ(x) < 0, x ∈ R

, x 6= 0, (12.8)

0 = L(x) + V

′

(x)f

(x) + Γ(x), x ∈ R

, (12.9)

respectively. I n contrast to th e framework developed in Chapter 11, the

bounding function Γ(·) is not assu med to satisfy (12.2) and (12.3) but rather

(12.7) and (12.8). Note that if V

∆f

(x) is identically zero, then (12.7) and

(12.8) specialize to (12.2) and (12.3). The idea behind this framework is that

only the ﬁxed part of the Lyapunov function V

(x) is a solution to the steady-

state Hamilton-Jacobi-Bellman equation for the nominal system, while the

overall Lyapunov fu nction V (x) is needed to establish robust stability.

For practical purposes the form of the parameter-dependent Lyapunov

function V (x) given by (12.6) is useful since the presence of ∆f within

(12.6) restricts the allowable time-varying uncertain parameters. That is,

if ∆f(t, x(t)) were permitted, then terms involving

∂∆f (t,x)

∂t

would arise and

potentially subvert the negative deﬁniteness of

V (x).

In this chapter, we extend the framework developed in Chapter 11

to address the p roblem of optimal nonlinear-nonquadratic robust feedback

control via parameter-dependent Lyapunov functions. Speciﬁcally, we

transform a robust nonlinear control problem into an optimal control

problem. This is accomplished by properly modifying the cost functional

to account for system uncertainty so that the solution of the modiﬁed

optimal nonlinear control problem serves as the solution to the robust

control problem. The present framework generalizes the linear guaranteed

cost control approach via parameter-dependent Lyapunov functions for

addressing robust stability and perf ormance [145,147,151,172] to nonlinear

uncertain systems with nonlinear-nonquadratic performance functionals.

The main contribution of this chapter is a methodology for designing

nonlinear controllers that provide both robust stability and robust per-

formance over a prescribed range of nonlinear time-invariant, structured

real parameter system uncertainty. The present framework is an extension

of the parameter-dependent Lyapunov fu nction approach developed in

[145, 147, 151, 162] to nonlinear systems by utilizing a performance bound

to provide robust performance in addition to robust stability. In particular,

the perf ormance bound can be evaluated in closed form as long as the

nonlinear-nonquadratic cost functional considered is related in a speciﬁc way

to the parameter-indepen dent part of an underlying parameter-dependent

Lyapunov function that is composed of a ﬁxed (parameter-independent) and

NonlinearBook10pt November 20, 2007

722 CHAPTER 12

a variable (parameter-dependent) part that guarantees robust stability over

a prescribed nonlinear time-invariant, real parameter uncertainty set. T he

ﬁxed part of the Lyapunov fu nction is shown to be the solution to the steady-

state form of the Hamilton-Jacobi-Bellman equation for the nominal sys tem

and plays a key role in constructing the optimal nonlinear robust control

law.

12.2 Linear Uncertain Systems and the Structured S ingular Value

For completeness, in this section we redirect our attention to the problem

of robust stability of linear systems with structured real and complex

parameter uncertainty. A general framework for this problem is provided

by mixed-µ theory [117] as a reﬁnement of the complex stru ctured singular

value. The ability of the structured singular value to account for complex,

real, and mixed uncertainty provides a powerful framework for robust

stability and performance problems in both analysis and synthesis (see

[110,117,344,381,475] and the references th erein). Since exact computation

of the structured singular value is, in general, an intractable problem, the

development of pr actically imp lementable bounds remains a high priority

in robust control research. Recent work in this area includes upper and

lower bounds for mixed uncertainty [117, 150, 153, 160, 263, 475] as well as

LMI-based computational techniques [66, 130].

An alternative approach to developing bounds for the structured

singular value is to specialize absolute stability criteria for sector-bounded

nonlinearities to the case of linear uncertainty. This approach, wh ich

has been explored by Chiang and Safonov [93], Haddad and Bernstein

[145, 147, 148, 150, 151], How and Hall [206], and Haddad et al. [172],

demonstrates the direct applicability of the classical theory of absolute

stability to the modern s tr uctured singular value f ramework. In particular,

the rich th eory of multiplier-based absolute stability criteria due to Lur´e and

Postnikov [5, 265, 331, 364], Popov [362], Yakubovich [469, 470], Zames and

Falb [479], and numerous others can be seen to have a close, fundamental

relationship with recently developed structured singular value bounds.

We start by stating and proving an absolute s tability criterion f or

multivariable systems with generalized positive real frequency-dependent

stability multipliers. This criterion involves a square nominal transfer

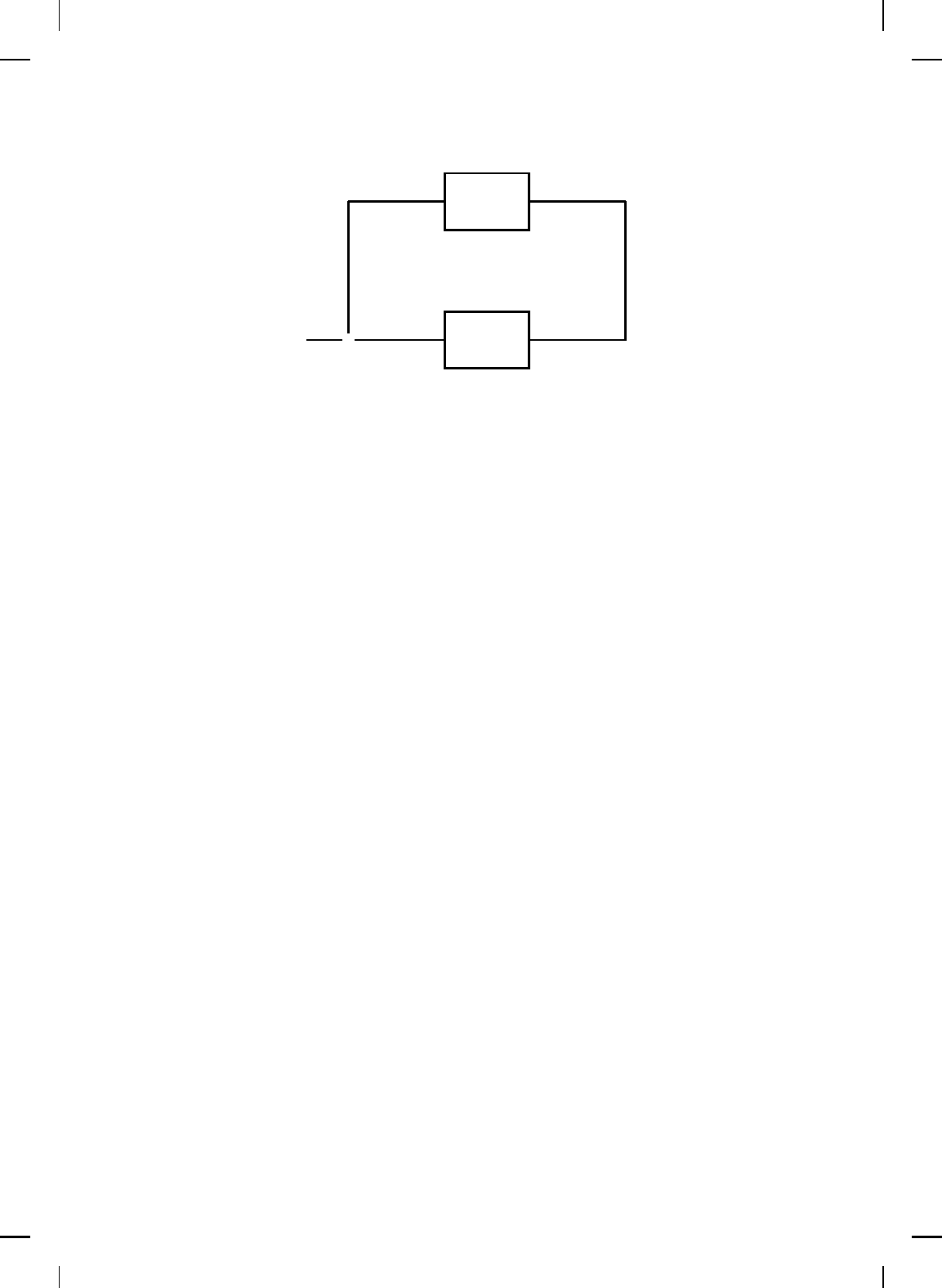
function G(s) in a negative feedback interconnection with a complex, square,

uncertain matrix ∆ as shown in Figure 12.1. Speciﬁcally, we consider the

set of block-diagonal matrices with possibly repeated blocks deﬁned by

∆

△

= {∆ ∈ C

m×m

: ∆ = block−diag[I

⊗ ∆

, I

⊗ ∆

, . . . , I

r+c

⊗ ∆

r+c

∆

∈ R

×m

, i = 1, . . . , r; ∆

∈ C

×m

, i = r + 1, . . . , r + c},

NonlinearBook10pt November 20, 2007

STRUCTURED PARAMETRIC UNCERTAINTY 723

∆

G(s)



-h-

(+)

(–)

Figure 12.1 Interconnection of transfer function G(s) with uncertain matrix ∆.

(12.10)

where the dimension m

and the number of repetitions l

of each block are

given and r + c ≥ 1. Furthermore, deﬁne the subset ∆ ⊆ ∆

consisting of

sector-b ounded matrices

∆

△

= {∆ = ∆

∗

∈ ∆

: M

≤ ∆ ≤ M

}, (12.11)

where M

, M

∈ ∆

are Hermitian matrices such that M

△

= M

− M

positive deﬁnite. Note that M

and M

are elements of ∆.

To prove the multivariable abs olute stability criterion for sector-

bounded uncertain matrices, deﬁne the sets D and N of Hermitian

frequency-dependent scaling matrix f unctions by

△

= {D : R → C

m×m

: D(ω) ≥ 0, D(ω)∆ = ∆D(ω), ω ∈ R, ∆ ∈ ∆

(12.12)

and

△

= {N : R → C

m×m

: N (ω) = N

∗

(ω), N(ω)∆ = ∆N(ω),

ω ∈ R, ∆ ∈ ∆

}. (12.13)

Furth ermore, deﬁne the set Z of complex multiplier matrix functions by

△

= {Z : R → C

m×m

: Z(ω) = D(ω) −N(ω), D(·) ∈ D, N (·) ∈ N}.

(12.14)

Note that if Z(·) ∈ Z, D(·) ∈ D, and N(·) ∈ N, then Z(ω) = D(ω) −

N(ω) if and only if D(ω) = He Z(ω) and N (ω) = Sh Z(ω). Hence,

since D(ω) ≥ 0, ω ∈ R ∪ ∞, Z(·) ∈ Z consists of arbitrary generalized

positive real functions [9]. For ∆ ∈ ∆

, D and N are given by

D = {D : R → C

m×m

: D = block−diag[D

⊗ I

, D

⊗ I

, . . . ,

NonlinearBook10pt November 20, 2007

724 CHAPTER 12

r+c

⊗I

r+c

], 0 ≤ D

∈ C

×l

, i = 1, . . . , r + c}, (12.15)

N = {N : R → C

m×m

: N = block−diag[N

⊗ I

, N

⊗ I

, . . . ,

r+c

⊗ I

r+c

], N

= N

∗

∈ C

×l

, N

⊗∆

= N

⊗ ∆

, i = 1, . . . , r + c}.

(12.16)

Although the condition D(ω)∆ = ∆D(ω) in D arises in complex

and mixed-µ analysis [117], the condition N (ω)∆ = ∆N(ω) in N has no

counterpart in [117]. As shown in [153] this condition generalizes mixed-µ

analysis to address nondiagonal real matrices which are not considered in

standard m ixed-µ theory. The cond ition N(ω)∆ = ∆N(ω) is an extension

of the condition used in [145] for Popov controller synthesis with constant

real matrix uncertainty.

Next, we introduce the following key lemma.

Lemma 12.1. Let Z(·) ∈ Z, let ω ∈ R ∪ ∞, and suppose det(I +

G(ω)M

) 6= 0. If

He [Z(ω)(M

−1

+ (I + G(ω)M

)

−1

G(ω))] > 0, (12.17)

then det (I + G(ω)∆) 6= 0 for all ∆ ∈ ∆.

Proof. For notational convenience we write D for D(ω) and N f or

N(ω). Suppose that there exists ∆ ∈ ∆ such that det (I + G(ω)∆) = 0.

Then there exists x ∈ C

, x 6= 0, such that (I + ∆G(ω))x = 0. Hence,

−x = ∆G(ω)x and −x

∗

= x

∗

(ω)∆.

Since M

≤ ∆ ≤ M

, it follows that

(∆ − M

−1

(∆ −M

) −(∆ −M

) ≤ 0,

or, equivalently,

He[∆M

−1

∆ − 2∆M

−1

+ M

−1

−∆ + M

] ≤ 0. (12.18)

Now, since D(·) ∈ D and M

, M

∈ ∆ it follows that DM

= M

D and

−1

= M

−1

D. Next, forming D(12.18) yields

He[∆DM

−1

∆ − 2∆DM

−1

+ M

−1

− ∆D + DM

] ≤ 0. (12.19)

Furth ermore, forming x

∗

(ω)(12.19)G(ω)x yields

∗

He[DM

−1

+ 2DM

−1

G(ω) + G

∗

(ω)M

−1

G(ω)

+DG(ω) + G

∗

(ω)DM

G(ω)]x ≤ 0. (12.20)

Next, note that He [Z(ω)(M

−1

+ (I + G(ω)M

)

−1

G(ω))] > 0 is