Haddad W.M. Nonlinear Dynamical Systems and Control: A Lyapunov-Based Approach

Подождите немного. Документ загружается.

NonlinearBook10pt November 20, 2007

STABILITY THEORY FOR NONLINEAR SYSTEMS 165

∞

γ(ks(τ, x)k)dτ, (3.112)

which implies that

V (s(t, x)) = −γ(ks(t, x)k) < 0, s(t, x) 6= 0. The result

is now immediate by noting that V

′

(x)f(x) =

V (s(0, x)) = −γ(kxk) < 0,

x ∈ B

(0), x 6= 0.

The next result gives a converse Lyapunov theorem for exponential

stability.

Theorem 3.10. Assume that the zero solution x(t) ≡ 0 to (3.1) is

exponentially stable, f : D → R

is continuously diﬀerentiable, and let

δ > 0 be such that B

(0) ⊂ D is contained in the domain of attraction

of (3.1). Then , for every p > 1, there exists a continuously diﬀerentiable

function V : D → R and scalars α, β, and ε > 0 such that

αkxk

≤ V (x) ≤ βkxk

, x ∈ B

(0), (3.113)

′

(x)f(x) ≤ −εV (x), x ∈ B

(0). (3.114)

Proof. Sin ce the zero solution x(t) ≡ 0 to (3.1) is, by assumption,

exponentially stable it follows that there exist positive scalars α

and β

such that if kx

k < δ, then kx(t)k ≤ α

−β

, t ≥ 0. Next, let x ∈

(0) and let s(t, x), t ≥ 0, denote the solution to (3.1) with the initial

condition x(0) = x so that for all kxk < δ, ks(t, x)k ≤ α

kxke

−β

, t ≥ 0.

Now, using identical arguments as in the proof of Theorem 3.9 it follows

that for α(δ) = α

, β(t) = e

−β

, γ(σ) = σ

, and (p − 1)β

> λ, where

λ = max

x∈B

(0)

′

(x)k,

∞

−β

dt < ∞ (3.115)

and

∞

pα

p−1

−β

(p−1)t

λt

dt < ∞. (3.116)

Hence,

V (x) =

∞

ks(t, x)k

dt (3.117)

is a continuously diﬀerentiable Lyapunov function candidate for (3.1).

To show that (3.113) holds, note that

V (x) =

∞

ks(t, x)k

≤

∞

kxk

−β

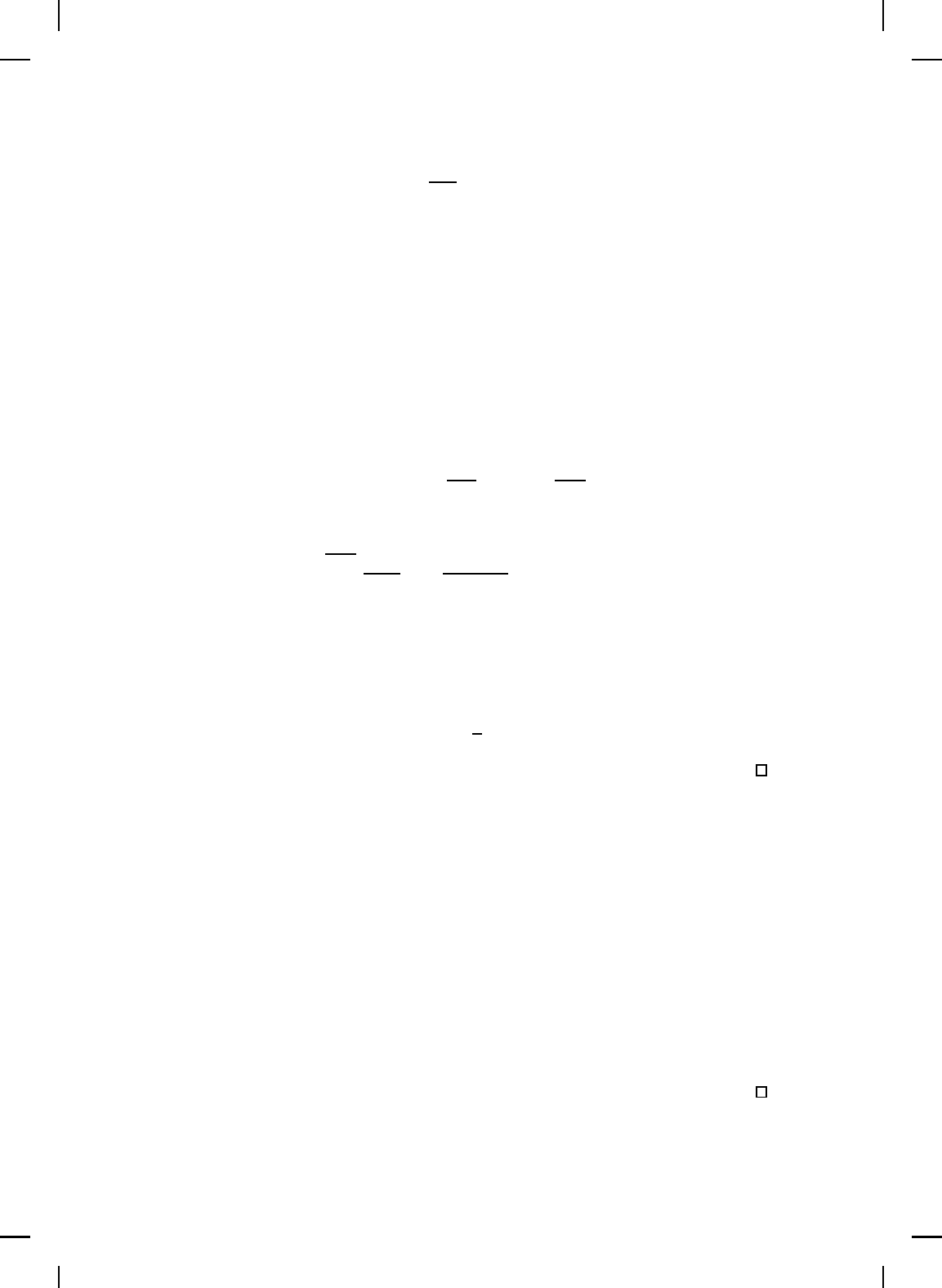
NonlinearBook10pt November 20, 2007

166 CHAPTER 3

pβ

kxk

, x ∈ D, (3.118)

which proves the upper bound in (3.113). Next, since kf

′

(x)k ≤ λ, x ∈

(0), an d f(·) is uniformly Lipschitz continuous on B

(0) with Lipschitz

constant L = λ it follows that

kf(s(t, x))k ≤ λks(t, x)k ≤ λα

kxke

−β

≤ λα

kxk, t ≥ 0. (3.119)

Hence,

s(t, x) = x +

f(s(τ, x))dτ (3.120)

implies

ks(t, x)k ≥ kxk − kxkλα

≥

kxk

, t ∈ [0,

2λα

]. (3.121)

Thus , it follows from (3.117) that

V (x) ≥

2λα

kxk

dt =

p+1

λα

kxk

, x ∈ B

(0), (3.122)

which proves the lower bound in (3.113).

Finally, to s how (3.114) note that with γ(σ) = σ

it follows as in the

proof of Theorem 3.9 that

V (x) = −γ(kxk) = −kxk

. Now, the result is

immediate from (3.113) by noting that

V (x) = −kxk

≤ −

V (x), x ∈ B

(0), (3.123)

which proves (3.114).

Next, we present a corollary to Theorem 3.10 that shows that p in

Theorem 3.10 can be taken to be equal to 2 without loss of generality.

Corollary 3.2. Assume that the zero solution x(t) ≡ 0 to (3.1) is

exponentially stable, f : D → R

is continuously diﬀerentiable, and let

δ > 0 be s uch that B

(0) ⊂ D is contained in the domain of attraction of

(3.1). Then there exists a continuously d iﬀerentiable function V : D → R

and scalars α, β, and ε > 0 such th at

αkxk

≤ V (x) ≤ βkxk

, x ∈ B

(0), (3.124)

′

(x)f(x) ≤ −εV (x), x ∈ B

(0). (3.125)

Proof. The proof is a direct consequence of Theorem 3.10 with p = 2.

NonlinearBook10pt November 20, 2007

STABILITY THEORY FOR NONLINEAR SYSTEMS 167

Finally, we present a converse theorem for global exponential stability.

Theorem 3.11. If the zero solution x(t) ≡ 0 to (3.1) is globally

exponentially stable and f : R

→ R

is continuously diﬀerentiable and

globally Lipschitz, then there exists a continuously diﬀerentiable function

V : D → R and scalars α, β, and ε > 0, su ch that

αkxk

≤ V (x) ≤ βkxk

, x ∈ R

, (3.126)

′

(x)f(x) ≤ −εV (x), x ∈ R

. (3.127)

Proof. By Proposition 2.26 f (·) is globally Lip schitz if and only if

′

(x)k ≤ λ, x ∈ R

. Now, the proof is identical to the proof of Theorem

3.10 by replacing B

(0) by R

and p = 2.

Finally, it is important to note that even though we assumed that the

vector ﬁ eld f is continuously diﬀerentiable in order to establish converse

Lyapunov theorems for asymptotic stability, these results also hold for

vector ﬁelds that are locally Lipschitz continuous as well as continuous. In

particular, Massera [307] proved a converse theorem involving the existence

of a smooth (i.e., inﬁnitely diﬀerentiable) Lyapunov function for locally

Lipschitz continuous vector ﬁelds. In addition, Kurzweil [250] proved the

existence of a smooth Lyapunov function for asymptotic stability under

the assumption of f only being continuous. The proofs of these results,

however, are considerably more involved than the converse proofs given in

this section and involve concepts not introduced in this book. For further

details, see [250,460].

3.6 Lyapunov Instability The orems

In the preceding sections we established suﬃcient conditions for Lyapunov

and asymp totic stability of non linear dynamical systems. In this section, we

provide thr ee key instability theorems based on Lyapunov’s direct method

for proving that the zero solution x(t) ≡ 0 to (3.1) is unstable. The main

utility of these instability theorems are when Lyapunov’s indirect method

(see Theorem 3.19) fails to provide any information about the stability of a

nonlinear dynamical system.

Theorem 3.12 (Lyapunov’s First Instability Theorem). Consider the

nonlinear dynamical system (3.1). Assume that there exist a continuously

diﬀerentiable function V : D → R and a scalar ε > 0 such that

V (0) = 0, (3.128)

′

(x)f(x) > 0, x ∈ B

(0), x 6= 0. (3.129)

Furth ermore, assume that for every suﬃciently small δ > 0 there exists

NonlinearBook10pt November 20, 2007

168 CHAPTER 3

∈ D such that kx

k < δ and V (x

) > 0. Then, the zero solution x(t) ≡ 0

to (3.1) is unstable.

Proof. Suppose, ad absurdum, that there exists δ > 0 such that if

∈ B

(0), then x(t) ∈ B

(0), t ≥ 0. By assumption, there exists x

∈ D

such that V (x

) = c > 0 and kx

k < δ. In this case, it follows from (3.129)

that V

′

(x(t))f(x(t)) ≥ 0, t ≥ 0, and hence, V (x(t)) ≥ c > 0, t ≥ 0. Thus,

on the tr aj ectory, V

′

(x(t))f(x(t)) > 0, t ≥ 0, where x(t), t ≥ 0, denotes the

solution of (3.1) with initial condition x

. Now, consider the s et S

△

= {y ∈

: y = x(t), t ≥ 0} and note that S is compact. Since V

′

(x(t))f(x(t)) > 0,

t ≥ 0, it follows th at there exists d = min

y ∈S

′

(y)f(y) > 0. Next, since

V (·) is continuous on B

(0) it follows that there exists α > 0 such that

V (x) ≤ α, x ∈ B

(0) ∩S. Hence, it follows that

α ≥ V (x(t)) = V (x(t

)) +

′

(x(s))f(x(s))ds ≥ c + (t − t

)d, t ≥ t

(3.130)

Since the right-hand side of (3.130) is unbounded it follows that there exists

t ≥ t

such that α < c + (t − t

)d, which contradicts (3.130). Hence, there

does not exist δ > 0 such that if x

∈ B

(0), then x(t) ∈ B

(0), t ≥ 0. Thus,

the zero solution x(t) ≡ 0 to (3.1) is unstable.

It is interesting to note that the function V (·) in Theorem 3.12 can be

positive as well as negative in D. However, V (·) is required to be positive

for some points x

6= 0 arbitrarily close to the origin of the nonlinear

dynamical system. A more r estrictive version of Theorem 3.12 is the case

where V : D → R is positive deﬁnite for all x ∈ B

(0). In this case, the

zero solution x(t) ≡ 0 to (3.1) is completely unstable in the sense that there

exists ε > 0 such that every trajectory starting in B

(0), other than the

trivial trajectory, eventually leaves B

(0). Similar remarks hold for the next

instability theorem.

Example 3.12. Consider the nonlinear d y namical system

˙x

(t) = −x

(t) + x

(t), x

(0) = x

, t ≥ 0, (3.131)

˙x

(t) = x

(t) + x

(t), x

(0) = x

. (3.132)

To examine the stability of this system consider the function V (x

, x

) =

−

. Note that on the line x

= 0, V (x

, x

) > 0 at points arbitrarily

close to the origin. Evaluating

V (x

, x

) yields

V (x

, x

) = −x

˙x

+ x

˙x

= x

+ x

− x

+ x

. (3.133)

Now, there exist a neighborhood N of the origin and δ ∈ (0, 1) such that

|−x

+ x

| ≤ δ(x

+ x

), (x

, x

) ∈ N, which implies that

V (x

, x

) ≥

(1−δ)(x

) > 0, (x

, x

) ∈ N. Hence, it follows f rom Theorem 3.12 that

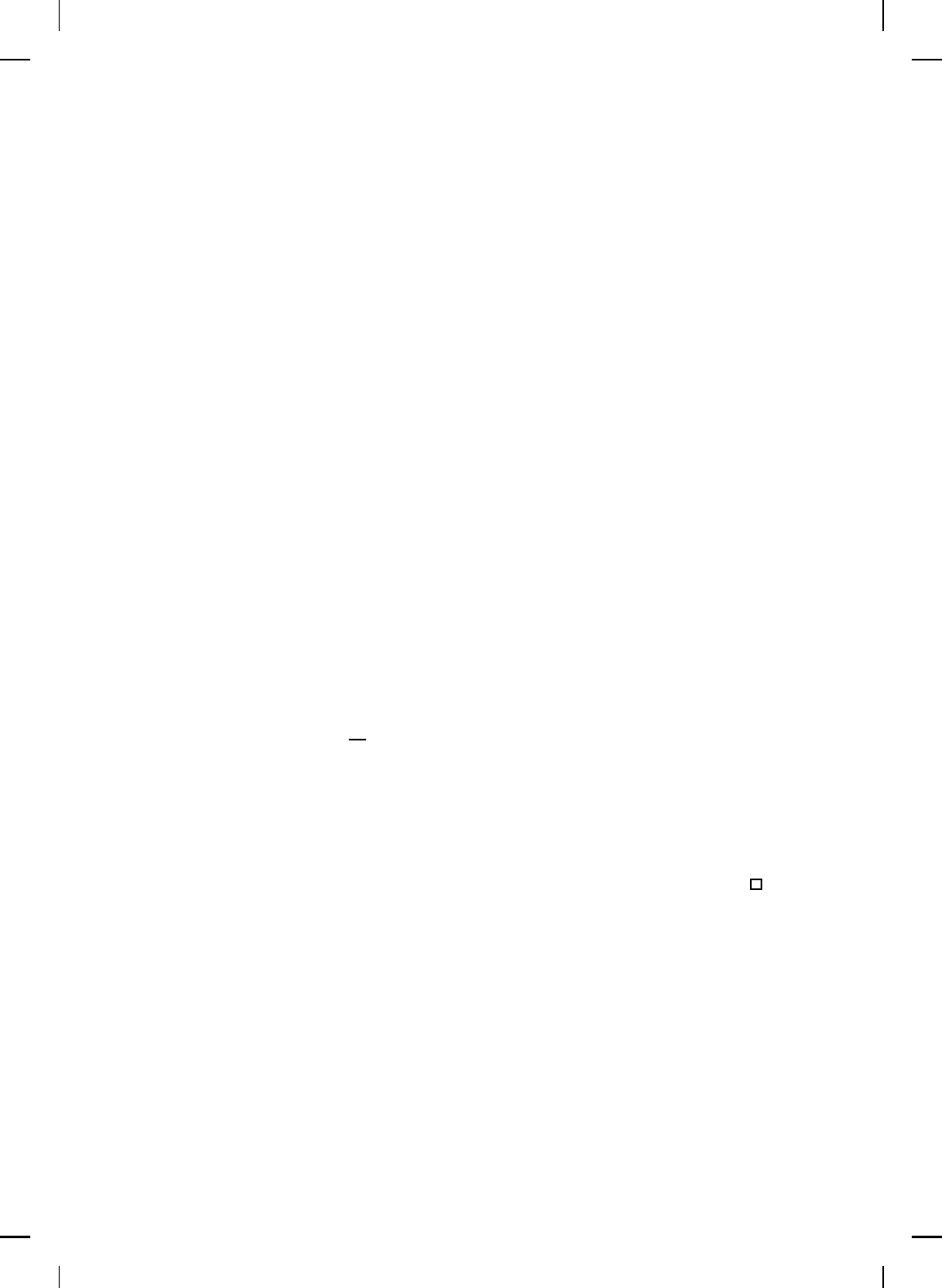
NonlinearBook10pt November 20, 2007

STABILITY THEORY FOR NONLINEAR SYSTEMS 169

the zero solution (x

(t), x

(t)) ≡ (0, 0) to (3.131) and (3.132) is unstable.△

Theorem 3.13 (Lyapunov’s Second Instability Theorem). Consider

the nonlinear dyn amical system (3.1). Assume that there exist a continu-

ously diﬀerentiable function V : D → R, a function W : D → R, and scalars

ε, λ > 0, such that

V (0) = 0, (3.134)

W (x) ≥ 0, x ∈ B

(0), (3.135)

′

(x)f(x) = λV (x) + W (x). (3.136)

Furth ermore, assume that for every suﬃciently small δ > 0 there exists

∈ D such that kx

k < δ and V (x

) > 0. Then the zero solution x(t) ≡ 0

to (3.1) is unstable.

Proof. Suppose ad absurdum, that there exists δ > 0 such that if

∈ B

(0), then x(t) ∈ B

(0), t ≥ 0. By assumption, there exists x

∈ B

(0)

such that V (x

) > 0. It follows from (3.135) and (3.136) that

′

(x)f(x) ≥ λV (x), x ∈ B

(0),

or, equivalently,

′

(x)f(x) −λV (x) ≥ 0, x ∈ B

(0). (3.137)

Next, forming e

−λt

(3.137), t ≥ 0, yields

−λt

′

(x(t))f(x(t)) − λe

−λt

V (x(t)) ≥ 0, t ≥ 0, (3.138)

and h en ce,

−λt

V (x(t))] ≥ 0, t ≥ 0, (3.139)

where x(t), t ≥ 0, denotes the solution to (3.1) with initial cond ition x

Integrating both sides of (3.139) yields e

−λt

V (x(t))−V (x(0)) ≥ 0, t ≥ 0, and

hence, V (x(t)) ≥ e

λt

V (x(0)), t ≥ 0. Thus , since V (x

) > 0, x(t) 6∈ B

(0) as

t → ∞, which is a contradiction. Hence, the zero solution x(t) ≡ 0 to (3.1)

is unstable since there does not exist δ > 0 such that if x

∈ B

(0), then

x(t) ∈ B

(0), t ≥ 0.

Example 3.13. Consider the nonlinear d ynamical system

˙x

(t) = x

(t) + 2x

(t) + x

(t)x

(t), x

(0) = x

, t ≥ 0, (3.140)

˙x

(t) = 2x

(t) + x

(t) −x

(t)x

(t), x

(0) = x

. (3.141)

To examine the stability of this system consider the function V (x

, x

) =

− x

. Note that on the line x

= 0, V (x

, x

) > 0 at points arbitrarily

close to the origin. Evaluating

V (x

, x

) yields

V (x

, x

) = 2x

˙x

− 2x

˙x

NonlinearBook10pt November 20, 2007

170 CHAPTER 3

= 2x

− 2x

+ 4x

= 2V (x

, x

) + 4x

. (3.142)

Now, w ith W (x

, x

)

△

= 4x

≥ 0, (x

, x

) ∈ R × R, it follows that

the conditions of Theorem 3.13 are satisﬁed, and hence, the zero solution

(t), x

(t)) ≡ (0, 0) to (3.140) and (3.141) is unstable. △

The ﬁnal instability theorem is due to Chetaev [92] and is appropri-

ately known as Chetaev’s instability theorem.

Theorem 3.14 (Chetaev’s Instability Theorem). Consider the non -

linear dynamical system (3.1) and assume that there exist a continuously

diﬀerentiable f unction V : D → R, a scalar ε > 0, and an open set Q ⊆ B

(0)

such that

V (x) > 0, x ∈ Q, (3.143)

sup

x∈Q

V (x) < ∞, (3.144)

0 ∈ ∂Q, (3.145)

V (x) = 0, x ∈ ∂Q ∩B

(0), (3.146)

′

(x)f(x) > 0, x ∈ Q. (3.147)

Then the zero solution x(t) ≡ 0 to (3.1) is unstable.

Proof. Let x

∈ Q and suppose, ad absurdum, that there exists a

closed set P s uch that x(t) ∈ P ⊂ Q ⊆ B

(0), t ≥ 0. Hence, it follows from

(3.147) that

V (x(t)) = V (x(0)) +

V (x(s))ds

= V (x(0)) +

′

(x(s))f(x(s))ds

≥ V (x(0)) + αt,

where α = min

x∈P

′

(x)f(x) > 0, which implies that V (x(t)) → ∞ as

t → ∞, contradicting (3.144). Thus, there exists T > 0 such that either

x(T ) ∈ ∂Q or x(t) → ∂Q as t → ∞. First, consider the case in which

x(T ) ∈ ∂Q. In this case, since (3.147) implies that V (x(t)), t ≥ 0, is strictly

increasing for all x(t) ∈ Q it follows that V (x(T )) > 0. Hence, since V (x) =

0, x ∈ ∂Q ∩ B

(0), it follows that x(T ) 6∈ ∂Q ∩ B

(0), wh ich implies that

x(T ) ∈ ∂Q\B

(0). Next, since ∂Q = Q ∩ ∂Q and Q ⊆ B

(0) it follows that

∂Q\B

(0) = (Q ∩∂Q)\B

(0) ⊆ (B

(0) ∩∂Q)\B

(0) = ∂Q ∩ ∂B

(0). Hence,

x(T ) ∈ ∂B

(0). Similarly, if x(t) → ∂Q as t → ∞ , then x(t) → ∂B

(0) as

t → ∞. Thus, there does not exist δ > 0 such that if x

∈ B

(0), then

x(t) ∈ B

(0), t ≥ 0. Hence, the zero solution x(t) ≡ 0 to (3.1) is uns table.

NonlinearBook10pt November 20, 2007

STABILITY THEORY FOR NONLINEAR SYSTEMS 171

Unlike Theorems 3.12 and 3.13 requiring that V (·) and

V (·) satisfy

certain conditions at all points in a neighborhood of D, Chetaev’s instability

theorem requires that V (·) and

V (·) satisfy certain conditions in a subregion

Q of D.

Example 3.14. Consider the nonlinear d ynamical system

˙x

(t) = x

(t) + x

(t)x

(t), x

(0) = x

, t ≥ 0, (3.148)

˙x

(t) = −x

(t) + x

(t), x

(0) = x

. (3.149)

To examine the stability of this system consider the function V (x

, x

) =

−

and the open set Q

△

= {(x

, x

) ∈ B

(0) : x

> x

> −x

}. Note

that V (x

, x

) > 0, (x

, x

) ∈ Q, and V (x

, x

) = 0, x ∈ ∂Q ∩ B

(0), where

(0) is a suﬃciently small neighborh ood of the origin. Next, evaluating

V (x

, x

) yields

V (x

, x

) = x

˙x

−x

˙x

= x

− x

) + x

. (3.150)

Now, there exist a neighborhood N of the origin and δ ∈ [0, 1) such that

V (x

, x

) ≥ x

− (1 + δ)|x

+ x

, (x

, x

) ∈ N (3.151)

which s hows that

V (x

, x

) > 0, (x

, x

) ∈ Q ∩ N. Hence, it follows from

Theorem 3.14 that the zero solution (x

(t), x

(t)) ≡ (0, 0) to (3.148) and

(3.149) is unstable. △

3.7 Stability of Linear Systems and Lyapunov’s

Linearization Method

In this section, we consider linear time-invariant dynamical systems of the

form

˙x(t) = Ax(t), x(0) = x

, t ≥ 0, (3.152)

where x(t) ∈ R

, t ≥ 0, and A ∈ R

n×n

. Note that the equilibrium solution

x(t) ≡ x

to (3.152) corresponds to x

∈ N(A), and hence, every point in

the null space of A is an equilibrium point for the linear dynamical system

(3.152). In the case where det A 6= 0, A has a trivial null space, and hence,

= 0. To examine the stability of (3.152) recall that the s olution to (3.152)

is given by x(t) = e

x(0), t ≥ 0. The structure of e

can be understood by

considering the Jordan form of A. In particular, it follows from the Jordan

decomposition [201] that A = SJS

−1

, where S ∈ C

n×n

is a nonsingu lar

matrix an d J = block−diag[J

, . . . , J

] is the Jordan form of A. Hence,

= Se

−1

, where e

= block−diag[e

, . . . , e

]. The structure of e

can thus be determined by considering each Jordan block J

. Recall that J

is of the form J

= λ

+ N

, where, for i ∈ {1, . . . , m}, n

is the order of

the ith Jordan block, λ

∈ spec(A), and N

is an n

× n

nilpotent matrix

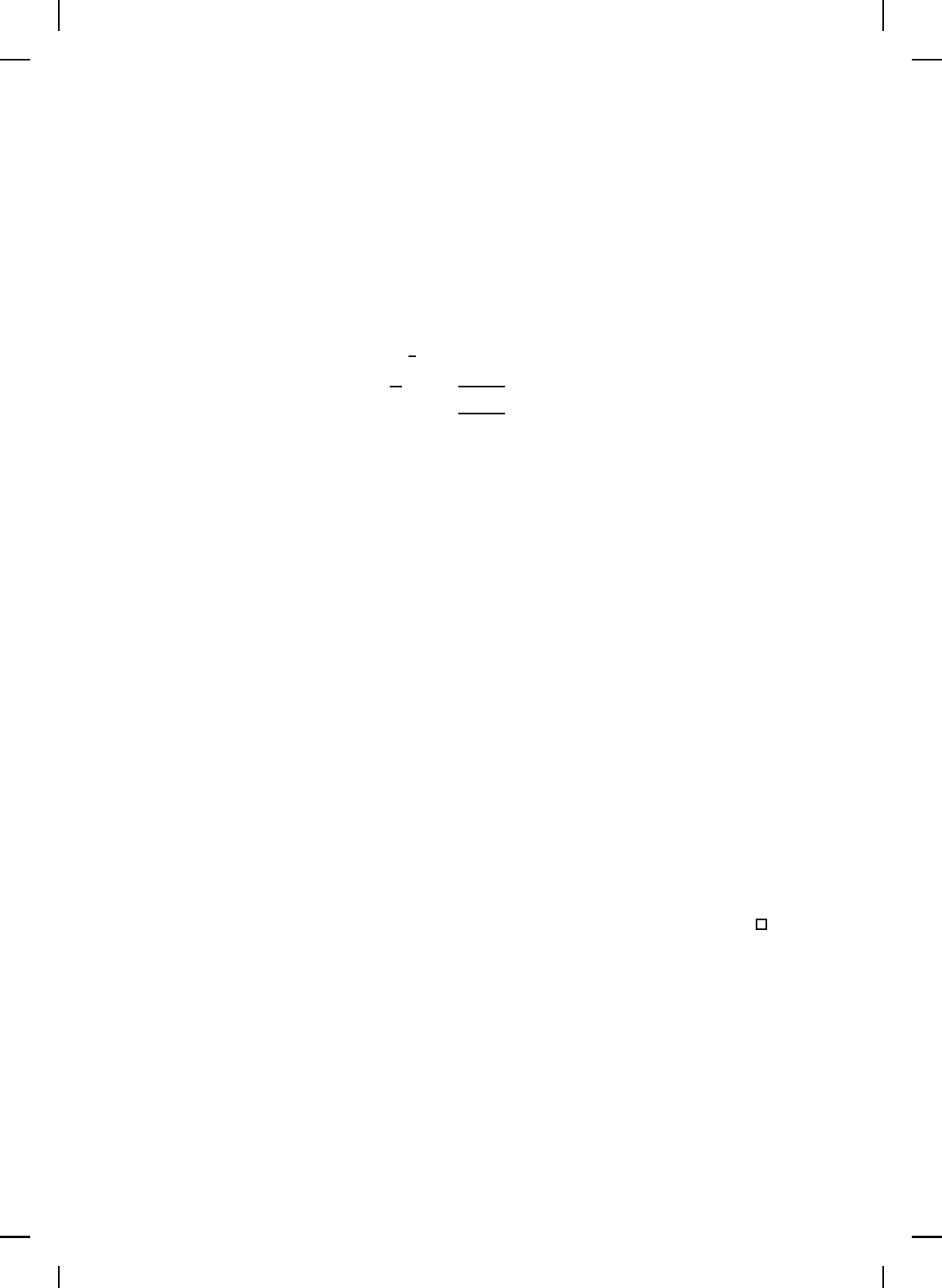
NonlinearBook10pt November 20, 2007

172 CHAPTER 3

which has ones on the superdiagonal and zeros elsewhere. By convention,

△

= 0

1×1

. Also note that N

= 0.

Now, since λ

and N

commute, it follows that

= e

(λ

= e

. (3.153)

Furth ermore, since N

= 0, it follows that e

is a ﬁnite sum of powers

of N

t. Speciﬁcally,

= I

+ N

t +

+ ··· + [(n

− 1)]!]

−1







1 t

···

−1

−1)!

0 1 t

−2

−2)!

0 ··· ··· 1







. (3.154)

Hence,

= Se

−1

i=1

j=1

j−1

(A), (3.155)

where m is th e number of distinct eigenvalues of A and p

(A) are constant

matrices. The following theorem gives necessary and suﬃcient conditions

for Lyapunov stability as well as global asymptotic stability of (3.152).

Theorem 3.15. Consider the linear dynamical system (3.152). The

zero solution x(t) ≡ 0 to (3.152) is Lyapunov stable if and only if for every

λ ∈ spec(A), either Re λ < 0, or both R e λ = 0 and λ is semisimple.

Alternatively, the zero solution x(t) ≡ 0 to (3.152) is globally asymptotically

stable if and only if Re λ < 0.

Proof. Since x(t) = e

x(0), t ≥ 0, it follows that the zero solution

to (3.152) is Lyapunov stable if an d only if there exists α > 0 such that

k < α, t ≥ 0. Now, it f ollows from (3.155) that e

, t ≥ 0, is bounded

if and only if either Re λ < 0, or both Re λ = 0 and λ is semisimple.

Alternatively, asymptotic stability is immediate since it follows from (3.155)

that e

→ 0 as t → ∞ if and only if Re λ < 0. Finally, since x(t), t ≥ 0,

depends linearly on x(0), asymptotic stability is global.

Theorem 3.15 gives necessary and suﬃcient conditions for Lyapunov

and asymptotic stability for the zero solution x(t) ≡ 0 of the linear system

(3.152) by examining the eigenvalues of A. In this book we say A is Lyapunov

stable if and only if every eigenvalue of A has nonpositive real part and

every eigenvalue of A with zero real part is semisimple. In addition, we

say A is asymptotically stable or Hurwitz if and only if every eigenvalue of

A has negative real part. Lyapunov’s stability theorem can also be u sed

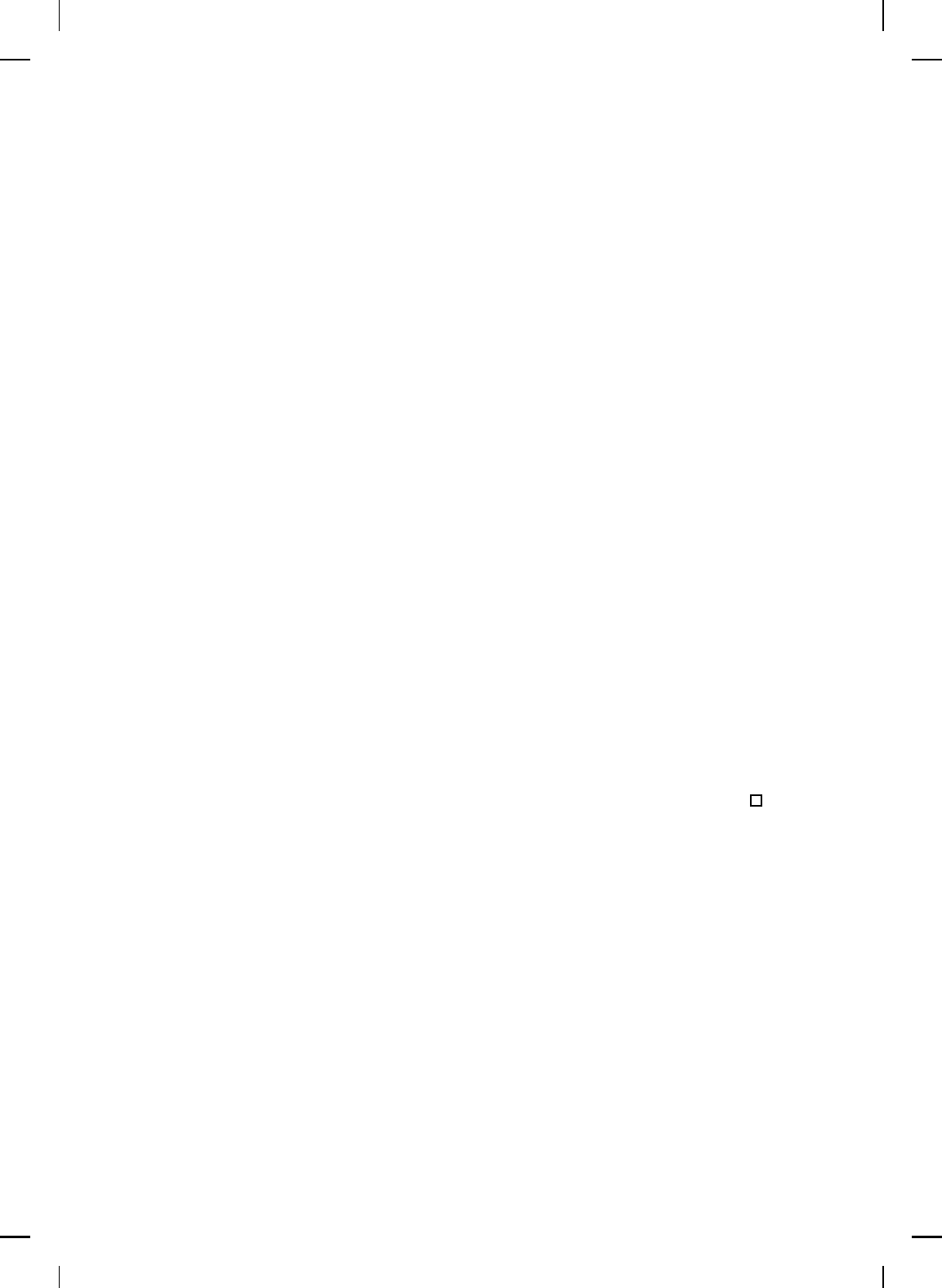
NonlinearBook10pt November 20, 2007

STABILITY THEORY FOR NONLINEAR SYSTEMS 173

to develop necessary and suﬃcient conditions for the stability of the zero

solution x(t) ≡ 0 to (3.152). In particular, to apply Theorem 3.1 to (3.152),

consider the Lyapunov function candidate V (x) = x

P x, where x ∈ R

and

P ∈ R

n×n

is positive deﬁnite. Next, note that

V (x) = V

′

(x)f(x) = 2x

P Ax = x

P + P A)x. (3.156)

Now, (3.4) will be satisﬁed if there exists a nonn egative-deﬁnite matrix R ∈

n×n

such that A

P + P A = −R so that V

′

(x)f(x) = −x

Rx ≤ 0, x ∈ R

Hence, we wish to determine the existence of a positive-deﬁnite matrix P

satisfying

0 = A

P + P A + R. (3.157)

Equation (3.157) is appropriately called a Lyapunov equation. The next

result addresses existence and un iqueness of solutions for the Lyapunov

equation (3.157).

Lemma 3.2. Let A ∈ R

n×n

. Then there exists a unique matrix P ∈

n×n

satisfying (3.157) if and only if

(A) + λ

(A) 6= 0, i, j = 1, . . . , n, (3.158)

where λ

(A) ∈ spec(A), k = 1, . . . , n.

Proof. Rewriting (3.157) as

vec(A

P ) + vec(P A) = −vec(R), (3.159)

where vec: R

n×n

→ R

denotes the column stacking operator, and using

the identities vec(XY Z) = (Z

⊗X)vec Y and I ⊗ X + Y

⊗I = Y

⊕X

[45, pp. 249 and 251], it follows that (A

⊕ A

)vec P = −vec R. Hence,

there exists a unique solution P ∈ R

n×n

satisfying (3.157) if and only if

det(A

⊕A

) 6= 0. Now, the result follows from the fact that spec(X ⊕Y ) =

{λ + µ : λ ∈ spec(X), µ ∈ spec(Y )} [45, p. 251].

It follows from Lemma 3.2 that if for some R ∈ R

n×n

, (3.157) does not

have a un ique solution, then the zero solution x(t) ≡ 0 to (3.152) is not an

asymptotically stable equilibrium. The following theorem gives necessary

and suﬃcient conditions for global asymptotic stability of (3.152) in terms

of the solution of the Lyapunov equation (3.157).

Theorem 3.16. Consider the linear dynamical system (3.152). The

zero solution x(t) ≡ 0 to (3.152) is globally asymptotically stable if and

only if for every positive-deﬁnite matrix R ∈ R

n×n

there exists a unique

positive-deﬁnite matrix P ∈ R

n×n

satisfying (3.157).

Proof. Suﬃciency follows from Theorem 3.1 by noting that if there

exists a positive-deﬁnite matrix P ∈ R

n×n

and a positive-deﬁnite matrix R ∈

NonlinearBook10pt November 20, 2007

174 CHAPTER 3

n×n

such that (3.157) holds, then global asymptotic stability is immediate

with Lyapunov function V (x) = x

P x. To show necessity, suppose A is

asymptotically stable, th at is, R e λ < 0, λ ∈ spec(A), and deﬁne

△

∞

dt. (3.160)

Note that the integral in (3.160) is well deﬁned since the integrand involves

a sum of terms of the from t

j−1

, where Re λ

< 0, and i = 1, . . . , m,

j = 1, . . . , n

. Next, note that

P + P A =

∞

dt +

∞

Adt

∞

)dt

= e



∞

= −R, (3.161)

which shows that there exists a matrix P ∈ R

n×n

satisfying (3.157).

To s how P > 0, let C ∈ R

p×n

be such that R = C

C and n ote that

for x ∈ R

P x =

∞

xdt

∞

kCe

dt, (3.162)

which shows that P = P

and P ≥ 0. Now, suppose, ad absurdum, that P is

not positive deﬁnite. Then, there exists x ∈ R

, x 6= 0, such that x

P x = 0,

which implies that Ce

x = 0 for all t ≥ 0. For t = 0 it follows that Cx = 0,

which implies C

Cx = 0, and hence, x = 0, which is a contradiction. Hence,

P > 0.

Finally, un iqueness of P follows from Lemma 3.2. Alternatively,

suppose, ad absurdum, th at there exist two solutions P

and P

to (3.157).

Then

0 = A

+ P

A + R, (3.163)

0 = A

+ P

A + R. (3.164)

Subtracting (3.164) from (3.163) yields

0 = A

− P

) + (P

− P

)A. (3.165)

Next, forming e

[(3.165)]e

, t ≥ 0, yields

0 = e

− P

) + (P

− P

)A]e