Greenwood D.T. Advanced Dynamics

Подождите немного. Документ загружается.

49 Work, energy and momentum

45°

Figure 1.21.

for all virtual displacements consistent with the constraints. Any such static equilibrium

conﬁguration is stable if it occurs at a local minimum of the potential energy, considered

as a function of the qs.

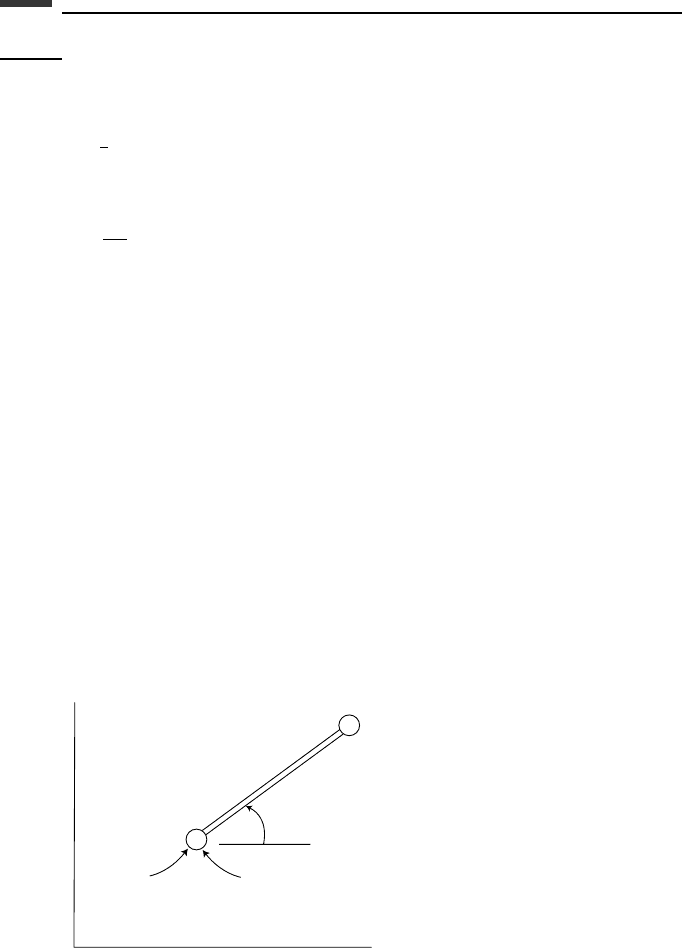
Example 1.11 Particles of mass m and 2m can slide freely on two rigid rods inclined at

◦

with the horizontal (Fig. 1.21). They are connected by a linear spring of stiffness k.We

wish to solve for the inclination θ of the spring and its tensile force F at the position of

static equilibrium.

Let us use the principle of virtual work. The applied forces acting on the system are the

spring force F and those due to gravity. The virtual work is

δW =



√

− F cos



+ θ





δx



√

2 mg − F cos



− θ



δx

= 0 (1.277)

Since δx

and δx

are independent virtual displacements, their coefﬁcients must each be

zero. Thus we obtain

F =

√

2 cos



+ θ



√

2 mg

cos



− θ



(1.278)

Hence, at equilibrium,

cos



− θ



= 2 cos



+ θ



(1.279)

cos θ + sin θ = 2

(

cos θ − sin θ

)

(1.280)

Thus,

tan θ =

or θ = 18.43

◦

(1.281)

50 Introduction to particle dynamics

Then, from (1.278), the tensile force F is

F =

2mg

cos θ + sin θ

√

10mg

= 1.5811mg (1.282)

Notice that, at the equilibrium conﬁguration, the angle θ and force F do not depend

on the spring stiffness k. So let us consider the case in which k is inﬁnite; that is, replace

the spring by a massless rigid rod of length L. Then δx

and δx

are not longer indepen-

dent. The force F becomes a constraint force which does not enter into the calculation

of θ.

The principle of virtual work gives the equilibrium condition

δV =−

√

(

δx

+ 2δx

)

= 0 (1.283)

The constraint relation between δx

and δx

can be found by noting that the length of the

rod is unchanged during a virtual displacement.

δx

cos



+ θ



+ δx

cos



− θ



= 0

(

cos θ − sin θ

)

δx

(

cos θ + sin θ

)

δx

= 0 (1.284)

We need to express an arbitrary virtual displacement in terms of δθ which is unconstrained.

To accomplish this, consider a small rotation of the system about its instantaneous center.

This results in

δθ =

√

2 δx

(

cos θ − sin θ

)

−

√

2 δx

(

cos θ + sin θ

)

(1.285)

Then the equilibrium condition of (1.283) can be written in the form

δV =

mgL

(

3 sin θ − cos θ

)

δθ = 0 (1.286)

since δθ = 0, in general, we conclude that

tan θ =

(1.287)

as we obtained previously. The expression for δV can be integrated to yield the potential

energy.

V =−

mgL

(

sin θ + 3 cos θ

)

(1.288)

Then we ﬁnd that

∂

∂θ

mgL

(

sin θ + 3 cos θ

)

√

2.5mgL (1.289)

Since this result is positive, the equilibrium is stable for the system consisting of two particles

connected by a rigid rod.

51 Work, energy and momentum

Generalized momentum

The kinetic energy of a system of particles, written as a function of (q,

q, t), has the form

of (1.258) which, in detail, is

T =



i=1



j=1

(q, t)



i=1

(q, t)

+ T

(q, t) (1.290)

The generalized momentum associated with the generalized coordinate q

∂T

∂

(1.291)



j=1

+ a

(1.292)

For a simple system such as a single particle whose position is given by the Cartesian

coordinates (x , y), the x-component of generalized momentum is just the x -component of

linear momentum m

x. On the other hand, if the position of the particle is given by the

polar coordinates (r,θ), then p

is equal to mr

θ, which is the angular momentum about

the origin. For more general choices of coordinates, the generalized momentum may not

have an easily discerned physical meaning. Because the generalized coordinates associated

with a given system do not necessarily have the same units or dimensions, neither will the

corresponding generalized momenta. However, the product p

will always have the units

of energy.

Example 1.12 Two particles, each of mass m are connected by a rigid massless rod of

length l to form a dumbbell that can move in the xy-plane. The position of the ﬁrst particle

is (x, y) and the direction of the second particle relative to the ﬁrst is given by the angle θ

(Fig. 1.22). We wish to ﬁnd the kinetic energy and the generalized momenta.

(x, y)

Figure 1.22.

52 Introduction to particle dynamics

Choose the ﬁrst particle as the reference point P and use the general kinetic energy

expression

T =



i=1

˙ρ

· m ˙ρ

(1.293)

which is of the form

T = T

+ T

(1.294)

Here T

, the kinetic energy due to the reference point motion, is

(1.295)

where m is the total mass. The kinetic energy due to motion relative to the reference point



i=1

˙ρ

(1.296)

Finally, the kinetic energy due to coupling between the two previous motions is

· m ˙ρ

(1.297)

If these general equations are applied to the present system, we obtain

T = m(

) +

+ ml

θ(

y cos θ −

x sin θ ) (1.298)

Then, using (1.291), we ﬁnd that

∂T

∂

= 2m

x −ml

θ sin θ (1.299)

∂T

∂

= 2m

y + ml

θ cos θ (1.300)

These are the x and y components of the total linear momentum.

The generalized momentum associated with θ is

∂T

∂

= ml

θ + ml(

y cos θ −

x sin θ ) (1.301)

This is equal to absolute angular momentum about P, that is, ml times the velocity com-

ponent of particle 2 which is perpendicular to the rod. Note that p

is not equal to H

as it

is ordinarily deﬁned. To obtain H

we use the relative kinetic energy T

∂T

∂





= ml

θ (1.302)

This is the angular momentum relative to the reference point P.

53 Impulse response

On the other hand, if we use (x

, y

,θ) as generalized coordinates, where (x

, y

) are the

Cartesian coordinates of the center of mass, the total energy of the system is, in accordance

with Koenig’s theorem,

T = m





(1.303)

The generalized momenta

∂T

∂

= 2m

, p

∂T

∂

= 2m

(1.304)

are once again the x and y components of the total linear momentum. The third generalized

momentum is

∂T

∂

θ (1.305)

This is the angular momentum about the center of mass.

1.5 Impulse response

Linear impulse and momentum

Consider a system of N particles whose position vectors r

are measured relative to an

inertial frame. Newton’s law of motion for the system is

p = F (1.306)

where F is the total external force and p is the total linear momentum.

p =



i=1

= m

(1.307)

where m is the total mass and r

is the position vector of the center of mass.

Now integrate (1.307) with respect to time over an interval t

to t

. We obtain

p = p

− p

F (1.308)

where the linear impulse

F is given by

F =



Fdt (1.309)

Equation (1.308) is a statement of the principle of linear impulse and momentum: The

change in the total linear momentum of a system of particles over a given time interval is

equal to the total impulse of the external forces acting on the system. Notice that the interval

is arbitrary and not necessarily small.

Since a vector equation is involved in this principle, a similar scalar equation must apply to

each component, provided that the component represents a ﬁxed direction in inertial space.

54 Introduction to particle dynamics

If any component of the total impulse is zero, the change in the corresponding component

of linear momentum is also zero. If F or one of its components is continuously equal to

zero, the corresponding linear momentum is constant. In this case, there is conservation of

linear momentum.

Angular impulse and momentum

Again consider a system of N particles and take a reference point P which is either (1) a

ﬁxed point, or (2) at the center of mass. The differential equation for the angular momentum

has the simple form

H = M (1.310)

Integrating this equation with respect to time over the interval t

to t

, we obtain

H = H

− H

M (1.311)

where the angular impulse

M due to the external forces is

M =



Mdt (1.312)

Note that the internal forces between particles occur in equal, opposite and collinear pairs.

Hence, they do not contribute to M or

Equation (1.311) is a statement of the principle of angular impulse and momentum.

Note that H can be zero even if M is not continuously zero. On the other hand, if M

is continuously zero, then

M = 0 over any interval, and there is conservation of angular

momentum. Similarly, if a certain component of M remains equal to zero, even though M

itself is not zero, then there is conservation of that component of H. Here we assume that

the direction of the component is ﬁxed in inertial space.

Now let us choose an arbitrary reference point P for angular momentum. The equation

of motion obtained earlier has the form

= M

− ρ

× m

(1.313)

Assume that very large forces are applied to the system of particles over an inﬁnitesimal

time t = t

− t

and integrate (1.313) with respect to time over this interval. We obtain



+t

dt =



+t

− ρ

× m

) dt (1.314)

H

− ρ

× m

(1.315)

During the interval t the conﬁguration is unchanged but M

and

may be very large,

resulting in ﬁnite

and 

. But if the reference point P is chosen such that

ﬁnite during the impulse, and therefore 

is zero, then the simpler form given in (1.311)

applies.

55 Impulse response

Figure 1.23.

Example 1.13 Three particles, each of mass m, are connected by rigid massless rods in

the form of an equilateral triangle (Fig. 1.23). The system is motionless with particle A

directly above particle B when a horizontal impulse

F is suddenly applied to particle A,

causing particle B to slide without friction on the horizontal ﬂoor. Solve for the values of

x and

θ immediately after the impulse. Evaluate the constraint impulse

N .

First let us use the linear impulse and momentum principle in the x direction to obtain

x +

θ =

F (1.316)

where we use (x,θ) as generalized coordinates and consider the θ rotation to take place

about particle B. If we choose a ﬁxed point that is initially coincident with particle B as the

reference point for angular momentum, we can ﬁnd

x and

θ without having to solve for the

constraint impulse

N . Thus, we can write

H =

M (1.317)

x +2ml

θ =

Fl (1.318)

Solving (1.316) and (1.318) we obtain

x =

15m

θ =

5ml

(1.319)

To ﬁnd the value of

N we use linear impulse and momentum in the vertical direction,

noting that C is the only particle with vertical motion. We obtain

√

θ =

N (1.320)

56 Introduction to particle dynamics

giving

N =

√

F (1.321)

Gravitational forces don’t affect the impulse response because they are ﬁnite and there-

fore result in a negligible impulse during the inﬁnitesimal interval t of the applied

impulse

Collisions

An important mode of interaction between bodies is that of collisions. We shall introduce

the theory by considering the special case of impact involving two smooth uniform spheres

(Fig. 1.24).

v′

Before impact

After impact

Figure 1.24.

Assume that the spheres are moving in the plane of the ﬁgure just before impact. At the

moment of impact, the interaction forces are normal to the tangent plane at the point of

contact, and therefore are directed along the line of centers. Let us assume that these forces

are impulsive in nature, that is, they are very large in magnitude and very short in duration.

As a result, there can be sudden changes in translational velocities, but rotational motion is

not involved.

Let v

and v

represent the velocity components along the line of centers just before

impact, and assume that v

. Let v

and v

be the corresponding tangential compo-

nents; that is, they are perpendicular to the line of centers. Because the spheres are smooth,

there are no tangential interaction forces, and therefore no changes in tangential velocities.

So we obtain



= v

(1.322)



= v

(1.323)

which implies the conservation of tangential momentum of each particle.

The interaction impulses along the line of centers are equal and opposite, so there is

conservation of the total normal momentum. Thus we have



+ m



= m

+ m

(1.324)

Now let us introduce the coefﬁcient of restitution e in accordance with the equation



− v



= e(v

− v

)(0≤ e ≤ 1) (1.325)

57 Impulse response

In other words, considering normal components, the relative velocity of separation after

impact is equal to e times the relative velocity of approach before impact. Solving (1.324)

and (1.325) for v



and v



, we obtain the ﬁnal normal velocity components.



− em

+ m

(1 +e)m

+ m

(1.326)



(1 +e)m

+ m

− em

+ m

(1.327)

If e = 0, termed inelastic impact, we see that v



= v



and the normal separation velocity

is zero. On the other hand, if e = 1, we have perfectly elastic impact, and the normal relative

velocities of approach and separation are equal. There is conservation of energy during

perfectly elastic impact, but there is some energy loss for 0 ≤ e < 1.

During the impact of two smooth spheres, there are equal and opposite impulses acting

on the spheres along the line of centers. These impulses are of magnitude

F = m

− v



) = m



− v

) (1.328)

Now suppose that Coulomb friction with a coefﬁcient µ is introduced at the impacting

surfaces. If sliding occurs throughout the impact, there will be equal and opposite friction

impulses µ

F acting to oppose the relative sliding motion. In other words, the total interaction

impulse of each body will lie along a cone of friction. It may turn out, however, that the

magnitude µ

F of the calculated friction impulse is larger than that required to stop the

relative sliding motion. In this case, the relative sliding motion will not reverse, but will

stop at zero with the friction impulse reduced accordingly.

We have assumed that the initial and ﬁnal velocity vectors, as well as the line of centers,

all lie in the same plane. If this is not true, one can choose an inertial frame translating with

sphere 2 just before impact as the reference frame. Then, relative to this second reference

frame, v

and v

are both equal to zero. The relative velocity vector of particle 1 and

the line of centers determine the plane in which the action takes place and for which the

standard equations such as (1.326) and (1.327) apply. After solving for the primed velocity

vectors relative to the second reference frame, one can ﬁnally transform to the original

inertial frame by adding vectorially the velocity of this second frame.

Example 1.14 A dumbbell is formed of two particles, each of mass m, which are connected

by a rigid massless rod of length l (Fig. 1.25). The dumbbell, with θ = 45

◦

and

θ = 0, is

falling vertically downwards with velocity v

when it has inelastic impact with a smooth

horizontal surface. We wish to ﬁnd the angular velocity

θ and the velocity v

of particle 1

immediately after impact.

First method Let us take the reference point P ﬁxed in particle 1 and use (1.315). Any

impulse acting on the system must be applied at P,so

= 0 (1.329)

58 Introduction to particle dynamics

(a)

(b)

45°

∆v

Figure 1.25.

The initial angular momentum about P is zero since

θ = 0 before impact. The ﬁnal angular

momentum is

H

= ml

θk (1.330)

where k is a unit vector in the z direction.

The impulse applied to particle 1 by the surface must be vertical since the horizontal

surface is frictionless. Hence there is no horizontal impulse applied to the system, and

its linear momentum in the x direction remains equal to zero. This implies that the x-

components of velocity of particles 1 and 2 are equal and opposite. Hence the velocity of

particle 1 immediately after impact is

√

i (1.331)

The vertical velocity of particle 1 after impact is zero because of the assumption of inelastic

impact. Thus, we ﬁnd that change in velocity of particle 1 is



√

i + v

j (1.332)

Note that the horizontal velocity component of the center of mass is continuously zero.

The position of the center of mass relative to the reference point P at particle 1 is

√

(i + j) (1.333)

The total mass of the system is 2m, so the term ρ

× m

in (1.315) is given by

× m

= 2m



√

−



k (1.334)