Gockenbach M.S. Partial Differential Equations. Analytical and Numerical Methods
Подождите немного. Документ загружается.

18
Chapter
2.
Models
in one
dimension
the
meaning
of
this
assumption
is
that
there
exists
a
constant
6
D > 0
such
that,
at
time
t,
the
chemical moves across
the
cross-section
at x at a
rate
of
This same quantity
can be
computed
from
the fluxes at the two
cross-sections
at x
and x + Ax. At the
left,
mass
is
entering
at a
rate
of
while
at the
right
it
enters
at a
rate
of
We
therefore have
(mass
per
unit
time—/
has
units
of
mass
per
volume
per
time).
We
then obtain
the
inhomogeneous
diffusion
equation:
The
units
of
this mass
flux are
mass
per
area
per
time (for example,
g/cm
2
s).
Since
the
units
of
du/dx
are
mass/length
4
,
the
diffusion coefficient
D
must have unit
of
area
per
time (for example,
cm
2
/s).
With this assumption,
the
equation modeling
diffusion
is
derived exactly
as
was
the
heat
equation, with
a
similar result.
The
total
amount
of the
chemical
contained
in the
part
of the
pipe between
x and x + Ax is
given
by
(2.12),
so the
rate
at
which
this
total
mass
is
changing
is
The
result
is the
diffusion
equation:
If
the
chemical
is
added
to the
interior
of the
pipe,
this
can be
accounted
for by a
function
/(x,
t),
where mass
is
added
to the
part
of the
pipe between
x and x + Ax
at a
rate
of
6
This
constant varies with
temperature
and
pressure;
see the CRC
Handbook
of
Chemistry
and
°hysics
[35],
page 6-179.
18
Chapter 2. Models
in
one dimension
the
meaning of this assumption
is
that
there exists a constant
6
D > 0 such
that,
at
time t,
the
chemical moves across the cross-section
at
x
at
a rate of
au
-D
ax
(x, t).
The
units
ofthis
mass flux are mass per area per time (for example,
g/cm
2
s). Since
the
units of
au/ax
are mass/length
4
,
the diffusion coefficient D must have unit of
area per time (for example, cm
2
/s).
With this assumption, the equation modeling diffusion
is
derived exactly as
was the heat equation, with a similar result. The total amount of the chemical
contained in
the
part
of
the
pipe between x
and
x + 6.x
is
given by (2.12), so the
rate
at
which this total mass
is
changing is
a [r+!::..x 1
r+!::..x
au
at
ix
Au(s, t)
ds
=
ix
A
at
(s, t) ds.
This same quantity can be computed from
the
fluxes
at
the two cross-sections
at
x
and x + 6.x. At the left, mass
is
entering
at
a
rate
of
au
-AD
ax
(x, t),
while
at
the right
it
enters
at
a
rate
of
au
AD
ax
(x + 6.x, t).
We
therefore have
r+!::..x
au au au
ix
A
8t
(s, t)
ds
=
-AD
8x
(x, t) +
AD
8x
(x + 6.x, t)
{x+!::..x
82u
=
ix
AD
8x
2
(s,
t)
ds.
The result is
the
diffusion equation:
8u = D 8
2
u 0 < X <
e,
t > to.
8t
8x
2
'
If
the
chemical
is
added
to
the interior of
the
pipe, this can be accounted for by a
function
f(x,
t), where mass
is
added to
the
part
of the pipe between x and x + 6.x
at
a rate of
r+!::..x
ix
Af(s,
t) ds
(mass per unit
time-f
has units of mass per volume per time).
We
then obtain
the inhomogeneous diffusion equation:
8u
8
2
u
8t
- D
8x
2
=
f(x,
t), 0 < x <
e,
t>
to·
6This
constant
varies
with
temperature
and
pressure; see
the
CRC
Handbook
of
Chemistry and
Physics
[35],
page 6-179.
2.1. Heat
flow
in a
bar; Fourier's
law 19
Just
as in the
case
of
heat
flow, we can
consider
steady-state
diffusion.
The
result
is the ODE
Temp.
(K)
c
(J/mole
• K)
200
21.59
250
23.74
300
25.15
350
26.28
400
27.39
500
29.70
600
32.05
As
this table indicates,
the
specific
heat
of a
material depends
on its
tempera-
ture.
How
would
the
heat equation change
if we did not
ignore
the
dependence
of
the
specific
heat
on
temperature?
3.
Verify
that
the
integral
in
(2.1)
has
units
of
energy.
4.
Suppose
u
represents
the
temperature distribution
in a
homogeneous bar,
as
discussed
in
this section,
and
assume
that
both ends
of the bar are
perfectly
insulated.
(a)
What
is the
IBVP
modeling this situation?
(b)
Show (mathematically)
that
the
total heat energy
in the bar is
constant
with
respect
to
time.
(Of
course, this
is
obvious
from
a
physical point
of
view.
The
fact
that
the
mathematical model implies
that
the
total
heat
energy
is
constant
is one
confirmation
that
the
model
is not
completely
divorced
from
reality.)
5.
Suppose
we
have
a
means
of
"pumping" heat energy into
a bar
through
one
of
the
ends.
If we add r
Joules
per
second through
the end at x =
I,
what
would
the
corresponding boundary condition
be?
6.
In our
derivation
of the
heat equation,
we
assumed
that
temperature
was
measured
on the
Kelvin scale. Explain what changes must
be
made
(in the
PDE, initial condition,
or
boundary conditions)
to use
degrees Celsius instead.
7.
The
thermal conductivity
of
iron
is
0.802
W/(cm
K).
Consider
an
iron
bar of
length
1 m and
radius
1 cm,
with
the
lateral side completely insulated,
and
assume
that
the
temperature
of one end of the bar is
held
fixed at 20
degrees
Celsius,
while
the
temperature
of the
other
end is
held
fixed at 30
degrees.
with
appropriate boundary conditions. Boundary conditions
for the
diffusion
equa-
tion
are
explored
in the
exercises.
Exercises
1.
Determine
the
units
of the
thermal conductivity
K
from
(2.6).
2.
In the CRC
Handbook
of
Chemistry
and
Physics [35], there
is a
table labeled
"Heat Capacity
of
Selected Solids,"
which
"gives
the
molar heat capacity
at
constant pressure
of
representative metals
...
as a
function
of
temperature
in
the
range
200 to 600 K"
([35],
page 12-190).
For
example,
the
entry
for
iron
is
as
follows:
2.1.
Heat flow
in
a
bar;
Fourier's law
19
Just
as in
the
case of
heat
flow, we
can
consider
steady-state
diffusion.
The
result is
the
ODE
d
2
u
-D
dx
2
=
f(x),
0 < x <
f,
with
appropriate
boundary
conditions.
Boundary
conditions for
the
diffusion equa-
tion
are
explored in
the
exercises.
Exercises
1. Determine
the
units
of
the
thermal
conductivity /'i, from (2.6).
2.
In
the
CRC
Handbook
of
Chemistry and Physics
[35],
there
is
a
table
labeled
"Heat
Capacity
of Selected Solids," which "gives
the
molar
heat
capacity
at
constant
pressure
of
representative metals
...
as a function
of
temperature
in
the
range
200
to
600 K" ([35], page 12-190). For example,
the
entry
for iron
is
as follows:
Temp.
(K)
c
(J(mole·
K)
As
this
table
indicates,
the
specific
heat
of
a
material
depends
on
its
tempera-
ture.
How would
the
heat
equation
change if we did
not
ignore
the
dependence
of
the
specific
heat
on
temperature?
3. Verify
that
the
integral in (2.1) has
units
of energy.
4.
Suppose u represents
the
temperature
distribution in a homogeneous
bar,
as
discussed
in
this
section,
and
assume
that
both
ends
of
the
bar
are perfectly
insulated.
(a)
What
is
the
IBVP
modeling this
situation?
(b) Show (mathematically)
that
the
total
heat
energy in
the
bar
is
constant
with
respect
to
time.
(Of
course,
this
is obvious from a physical point of
view.
The
fact
that
the
mathematical
model implies
that
the
total
heat
energy
is
constant
is
one confirmation
that
the
model
is
not
completely
divorced from reality.)
5. Suppose we have a means of "pumping"
heat
energy
into
a
bar
through
one
of
the
ends.
If
we
add
r Joules
per
second
through
the
end
at
x = f,
what
would
the
corresponding
boundary
condition be?
6.
In
our
derivation of
the
heat
equation, we assumed
that
temperature
was
measured
on
the
Kelvin scale. Explain
what
changes
must
be
made (in
the
PDE,
initial condition, or
boundary
conditions)
to
use degrees Celsius instead.
7.
The
thermal
conductivity of iron is 0.802 W
((
cm K). Consider
an
iron
bar
of
length 1 m
and
radius
1 cm, with
the
lateral
side completely insulated,
and
assume
that
the
temperature
of one
end
of
the
bar
is held fixed
at
20 degrees
Celsius, while
the
temperature
of
the
other
end
is held fixed
at
30 degrees.
20
Chapter
2.
Models
in one
dimension
Assume
that
no
heat energy
is
added
to or
removed
from
the
interior
of the
bar.
(a)
What
is the
(steady-state) temperature distribution
in the
bar?
(b)
At
what
rate
is
heat
energy
flowing
through
the
bar?
8. (a)
Show
that
the
function
is
a
solution
to the
homogeneous
heat
equation
(b)
What values
of 0
will
cause
u to
also
satisfy
homogeneous Dirichlet con-
ditions
at x = 0 and x =
tl
9.
In
this exercise,
we
consider
a
boundary condition
for a bar
that
may be
more
realistic
than
a
simple Dirichlet condition. Assume
that,
as
usual,
the
side
of a
bar is
completely insulated
and
that
the
ends
are
placed
in a
bath
maintained
at
constant temperature. Assume
that
the
heat
flows out of or
into
the
ends
in
accordance with Newton's
law of
cooling:
the
heat
flux is
proportional
to
the
difference
in
temperature between
the end of the bar and the
surrounding
medium.
What
are the
resulting boundary conditions?
10.
Derive
the
heat
equation
from
Newton's
law of
cooling (cf.
the
previous exer-
cise)
as
follows:
Divide
the bar
into
a
large number
n of
equal pieces, each
of
length
Ax.
Approximate
the
temperature
in the
«th
piece
as a
function
Ui(t)
(thus assuming
that
the
temperature
in
each piece
is
constant
at
each point
in
time). Write down
a
coupled system
of
ODEs
for
ui(t),
u^t],
• • •
,u
n
(t)
by
applying Newton's
law of
cooling
to
each piece
and its
nearest
neighbors.
Assume
that
the bar is
homogeneous,
so
that
the
material properties
p,
c, and
K
are
constant. Take
the
limit
as
Aa;
—>
0, and
show
that
the
result
is the
heat
equation.
11.
Suppose
a
chemical
is
diffusing
in a
pipe,
and
both ends
of the
pipe
are
sealed.
What
are the
appropriate boundary conditions
for the
diffusion
equation?
What
initial conditions
are
required? Write down
a
complete
IBVP
for the
diffusion
equation under these conditions.
12.
Suppose
that
a
chemical contained
in a
pipe
of
length
I
has an
initial concen-
tration
distribution
of
w(or,0)
=
ip(x}.
At
time zero,
the
ends
of the
pipe
are
sealed,
and no
mass
is
added
to or
removed
from
the
interior
of the
pipe.
(a)
Write down
the
IBVP describing
the
diffusion
of the
chemical.
(b)
Show
mathematically
that
the
total
mass
of the
chemical
in the
pipe
is
constant. (Derive this
fact
from
the
equations rather than
from
common
sense.)
20
Chapter
2.
Models
in
one
dimension
Assume
that
no heat energy
is
added
to
or removed from the interior of the
bar.
(a)
What
is
the (steady-state) temperature distribution in the bar?
(b) At what
rate
is
heat energy flowing through the bar?
8.
(a) Show
that
the function
u(x, t) =
e-~IPt/(pc)
sin
(Ox)
is
a solution
to
the homogeneous heat equation
au
a
2
u
pc
at - /'i,
ax
2
=
0,
0 < x <
l,
for all t.
(b)
What
values of 0 will cause u
to
also satisfy homogeneous Dirichlet con-
ditions
at
x = 0 and x = f?
9.
In this exercise,
we
consider a boundary condition for a bar
that
may be more
realistic
than
a simple Dirichlet condition. Assume
that,
as usual, the side of a
bar
is
completely insulated
and
that
the
ends are placed in a
bath
maintained
at
constant temperature. Assume
that
the heat
flows
out of or into the ends
in accordance with
Newton's law
of
cooling: the heat flux
is
proportional
to
the
difference in temperature between the end of the
bar
and the surrounding
medium.
What
are the resulting boundary conditions?
10. Derive
the
heat equation from Newton's law of cooling
(cf.
the previous exer-
cise) as follows: Divide the
bar
into a large number n of equal pieces, each of
length
Llx. Approximate the temperature in
the
ith
piece as a function
Ui(t)
(thus assuming
that
the
temperature in each piece
is
constant
at
each point
in time). Write down a coupled system of ODEs for
Ul
(t),
U2
(t),
...
,
Un
(t)
by applying Newton's law of cooling
to
each piece and its nearest neighbors.
Assume
that
the bar
is
homogeneous, so
that
the
material properties p,
c,
and
/'i, are constant. Take the limit as
Llx
-+
0, and show
that
the
result
is
the
heat equation.
11. Suppose a chemical
is
diffusing in a pipe, and
both
ends of the pipe are sealed.
What
are the appropriate boundary conditions for the diffusion equation?
What
initial conditions are required? Write down a complete IBVP for
the
diffusion equation under these conditions.
12. Suppose
that
a chemical contained in a pipe of length l has
an
initial concen-
tration
distribution of u(x,
0)
=
'IjJ(x).
At time zero, the ends of
the
pipe are
sealed, and no mass
is
added to or removed from the interior of the pipe.
(a) Write down the IBVP describing
the
diffusion of the chemical.
(b) Show
mathematically
that
the total mass of the chemical in
the
pipe
is
constant. (Derive this fact from the equations rather
than
from common
sense.)

2.2.
The
hanging
bar 21
(c)
Describe
the
ultimate steady-state concentration.
(d)
Give
a
formula
for the
steady-state
concentration
in
terms
of
if).
13.
Suppose
a
pipe
of
length
t and
radius
r
joins
two
large reservoirs, each con-
taining
a
(well-mixed) solution
of the
same chemical.
Let the
concentration
in
one
reservoir
be
MO
and in the
other
be
ui,
and
assume
that
w(0,£)
=
MO
and
u(l,t)
= ut.
(a)
Find
the
steady-state
rate
at
which
the
chemical
diffuses
through
the
pipe
(in
units
of
mass
per
time).
(b)
How
does this
rate
vary with
the
length
i and the
radius
r?
14.
Consider
the
previous exercise,
in
which
the
chemical
is
carbon monoxide
(CO)
and the
solution
is CO in
air. Suppose
that
MO
=
0.01
and
ui
=
0.015,
and
that
the
diffusion
coefficient
of CO in air is
0.208
cm
2
/s.
If the bar is 1
m
long
and its
radius
is
2cm,
find the
steady-state
rate
at
which
CO
diffuses
through
the
pipe
(in
units
of
mass
per
time).
15.
Verify
that
Theorem
2.1
holds
for (a, 6) x (c, d) =
(0,1)
x
[0,1]
and F
defined
by
F(x,y)
=
cos(xy}.
2.2 The
hanging
bar
Suppose
that
a
bar, with
uniform
cross-sectional
area
A and
length
i,
hangs verti-
cally,
and it
stretches
due to a
force
(perhaps gravity) acting upon
it. We
assume
that
the
deformation occurs only
in the
vertical direction; this assumption
is
reason-
able only
if the bar is
long
and
thin. Normal materials tend
to
contract horizontally
when
they
are
stretched vertically,
but
both this contraction
and the
coupling
be-
tween
horizontal
and
vertical motion
are
small compared
to the
elongation when
the bar is
thin (see
Lin and
Segel
[36],
Chapter
12).
With
the
assumption
of
purely vertical deformation,
we can
describe
the
move-
ment
of the bar in
terms
of a
displacement function
u(x,t).
Specifically,
suppose
that
the top of the bar is fixed at x = 0, and let
down
be the
positive
x-direction.
Let
the
cross-section
of the bar
originally
at x
move
to x +
u(x,t)
at
time
t
(see
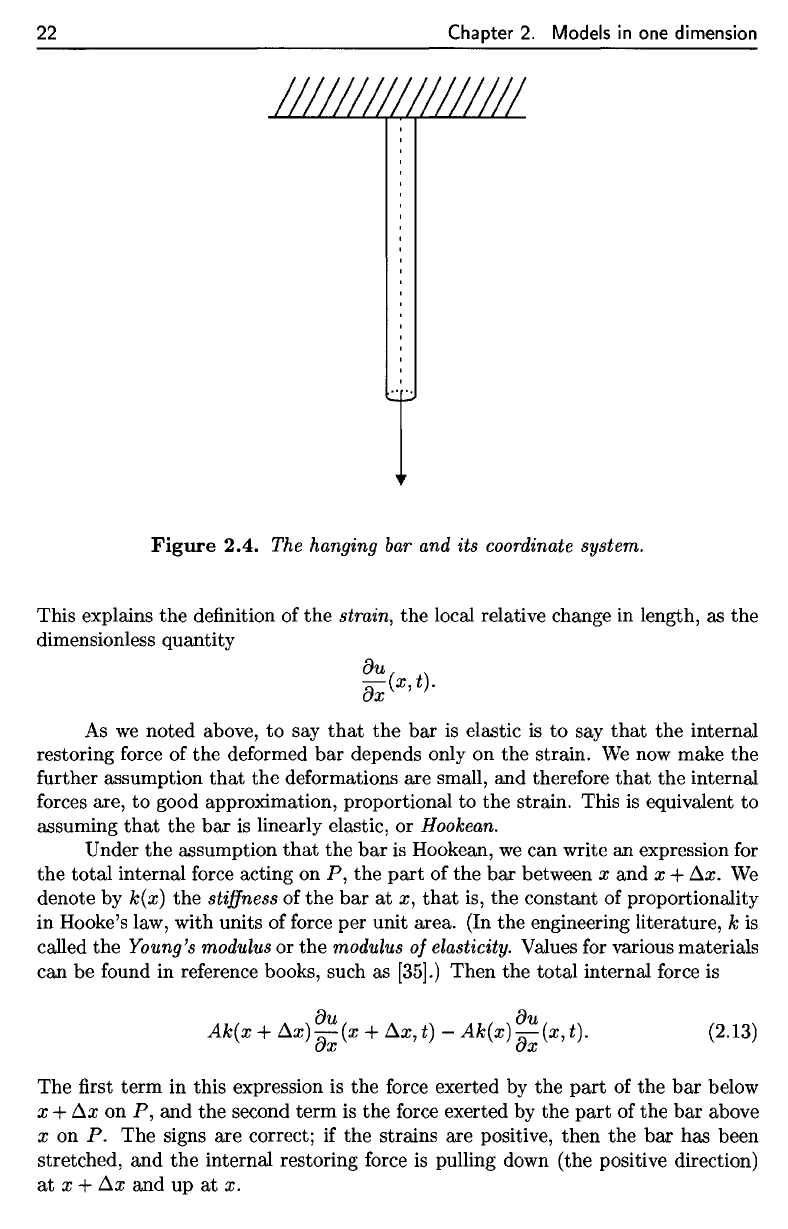
Figure 2.4).
We
will
derive
a PDE
describing
the
dynamics
of the bar by
applying
Newton's
second
law of
motion.
We
assume
that
the bar is
elastic, which means
that
the
internal
forces
in the
bar
depend
on the
local relative
of
change
in
length.
The
deformed length
of the
part
of the bar
originally between
x and x + Ax (at
time
t}
is
Since
the
original length
is Ax, the
change
in
length
of
this part
is
and the
relative change
in
length
is
2.2. The hanging bar
21
(c) Describe
the
ultimate
steady-state concentration.
(d) Give a formula for
the
steady-state concentration in terms of
'IjJ.
13. Suppose a pipe of length f
and
radius r joins two large reservoirs, each con-
taining a (well-mixed) solution of
the
same chemical. Let
the
concentration
in one reservoir
be
Uo
and
in
the
other
be
Uf,
and
assume
that
u(O,
t) =
Uo
and
u(f,
t) =
Uf.
(a)
Find
the
steady-state
rate
at
which
the
chemical diffuses
through
the
pipe (in units of mass
per
time).
(b) How does this
rate
vary with
the
length f
and
the
radius r?
14. Consider
the
previous exercise, in which
the
chemical is carbon monoxide
(CO)
and
the
solution
is
CO in air. Suppose
that
Uo = 0.01
and
Uf
= 0.015,
and
that
the
diffusion coefficient of CO in air
is
0.208 cm
2
Is.
If
the
bar
is
1 m
long
and
its radius
is
2 cm, find
the
steady-state
rate
at
which CO diffuses
through
the
pipe (in units
of
mass
per
time).
15.
Verify
that
Theorem
2.1
holds for
(a,
b)
x
(c,
d)
= (0,1) x [0,1]
and
F defined
by F(x, y) = cos (xy).
2.2 The hanging
bar
Suppose
that
a
bar,
with uniform cross-sectional
area
A
and
length
f,
hangs verti-
cally,
and
it stretches due
to
a force (perhaps gravity) acting
upon
it.
We
assume
that
the
deformation occurs only in
the
vertical direction; this assumption is reason-
able only if
the
bar
is long
and
thin. Normal materials
tend
to
contract horizontally
when
they
are stretched vertically,
but
both
this contraction
and
the
coupling be-
tween horizontal
and
vertical motion are small compared
to
the
elongation when
the
bar
is
thin
(see Lin
and
Segel
[36],
Chapter
12).
With
the
assumption of purely vertical deformation,
we
can describe
the
move-
ment of
the
bar
in
terms of a displacement function
u(x,
t). Specifically, suppose
that
the
top
of
the
bar
is
fixed
at
x =
0,
and
let down
be
the
positive x-direction.
Let
the
cross-section of
the
bar
originally
at
x move
to
x +
u(x,
t)
at
time t (see
Figure 2.4).
We
will derive a
PDE
describing
the
dynamics of
the
bar
by applying
Newton's second law
of
motion.
We
assume
that
the
bar
is elastic, which means
that
the
internal forces in
the
bar
depend on
the
local relative
of
change in length.
The
deformed length
of
the
part
of
the
bar
originally between x
and
x +
6.x
(at time t)
is
(x +
6.x
+ U (x + 6.x,
t))
- (x +
u(x,
t)) =
6.x
+
u(x
+ 6.x, t) -
u(x,
t).
Since
the
original length
is
6.x,
the
change in length of this
part
is
u(x
+ 6.x, t) -
u(x,
t),
and
the
relative change in length is
u(x+6.x,t)
-u(x,t)
~
ou(
)
6.x
-
ax
x,
t .
22
Chapter
2.
Models
in one
dimension
Figure
2.4.
The
hanging
bar and its
coordinate
system.
As
we
noted above,
to say
that
the bar is
elastic
is to say
that
the
internal
restoring
force
of the
deformed
bar
depends only
on the
strain.
We now
make
the
further
assumption
that
the
deformations
are
small,
and
therefore
that
the
internal
forces
are,
to
good approximation, proportional
to the
strain. This
is
equivalent
to
assuming
that
the bar is
linearly elastic,
or
Hookean.
Under
the
assumption
that
the bar is
Hookean,
we can
write
an
expression
for
the
total
internal
force
acting
on P, the
part
of the bar
between
x and x + Ax. We
denote
by
k(x)
the
stiffness
of the bar at x,
that
is, the
constant
of
proportionality
in
Hooke's law, with units
of
force
per
unit
area.
(In the
engineering literature,
k is
called
the
Young's modulus
or the
modulus
of
elasticity. Values
for
various materials
can be
found
in
reference books, such
as
[35].)
Then
the
total
internal
force
is
The first
term
in
this expression
is the
force
exerted
by the
part
of the bar
below
x + Ax on P, and the
second
term
is the
force
exerted
by the
part
of the bar
above
x on P. The
signs
are
correct;
if the
strains
are
positive, then
the bar has
been
stretched,
and the
internal restoring
force
is
pulling down (the positive direction)
at x + Ax and up at x.
This explains
the
definition
of the
strain,
the
local relative change
in
length,
as the
dimensionless quantity
22
Chapter
2.
Models
in
one
dimension
Figure
2.4.
The hanging
bar
and its coordinate system.
This explains the definition of
the
strain, the local relative change in length, as the
dimensionless quantity
au
ax
(x, t).
As
we
noted above,
to
say
that
the
bar
is
elastic
is
to
say
that
the
internal
restoring force of
the
deformed
bar
depends only on the strain.
We
now make
the
further assumption
that
the deformations are small, and therefore
that
the internal
forces are,
to
good approximation, proportional to the strain. This
is
equivalent to
assuming
that
the
bar
is
linearly elastic, or Hookean.
Under the assumption
that
the
bar
is
Hookean,
we
can write an expression for
the
total
internal force acting on
P,
the
part
of the bar between x and x +
~x.
We
denote by k(x)
the
stiffness of
the
bar
at
x,
that
is, the constant of proportionality
in Hooke's law, with units offorce per unit area. (In the engineering literature,
k
is
called the Young's modulus or the modulus
of
elasticity. Values for various materials
can be found in reference books, such as
[35].)
Then the total internal force is
au au
Ak(x
+
~x)
ax
(x +
~x,
t) -
Ak(x)
ax
(x, t).
(2.13)
The first
term
in this expression
is
the force exerted by the
part
of the
bar
below
x +
~x
on
P,
and the second
term
is
the
force exerted by
the
part
of
the
bar above
x on P. The signs are correct; if the strains are positive, then the
bar
has been
stretched,
and
the internal restoring force
is
pulling down (the positive direction)
at
x +
~x
and up
at
x.
(note
how the
factor
of A
cancels). This integral must
be
zero
for
every
x
e
[0,£)
and
every
Ax > 0. It
follows
(by the
reasoning introduced
on
page
12)
that
the
integrand must
be
identically zero;
this
gives
the
equation
The PDE
(2.14)
is
called
the
wave
equation.
If
the bar is in
equilibrium, then
the
displacement does
not
depend
on
t,
and
we
can
write
u =
u(x].
In
this case,
the
acceleration
d
2
u/dt
2
is
zero,
and the
forcing
function
/
must also
be
independent
of
time.
We
then obtain
the
following
ODE
for
the
equilibrium displacement
of the
bar:
2.2.
The
hanging
bar 23
Now,
(2.13)
is
equal
(by the
fundamental theorem
of
calculus)
to
We
now
assume
that
all
external
forces
are
lumped into
a
body
force
given
by a
force
density
/
(which
has
units
of
force
per
unit
volume).
Then
the
total
external
force
on P (at
time
t]
is
and the sum of the
forces
acting
on
part
P is
Newton's
second
law
states
that
the
total
force
acting
on P
must equal
the
mass
of P
times
its
acceleration.
This
law
takes
the
form
where
p(x)
is the
density
of the bar at x (in
units
of
mass
per
volume).
We can
rewrite
this
as
This
is the
same equation
that
governs steady-state
heat
flow!
Just
as in the
case
of
steady-state
heat
flow, the
resulting BVPs
can be
solved with
two
integrations
(see Examples
2.2 and
2.3).
2.2.
The hanging bar
23
Now,
(2.13)
is
equal (by the fundamental theorem of calculus)
to
r+l:J.x a (
au
)
ix
A
ax
k(s)
ax
(s, t) ds.
We
now assume
that
all external forces are lumped into a body force given by a force
density
f (which has units offorce per unit volume). Then the total external force
on
P (at time t)
is
{x+l:J.x
ix
f(s,
t)Ads,
and
the
sum of
the
forces acting on
part
P
is
{x+l:J.x
a (
au
) r+l:J.x
ix
A
ax
k(s)
ax
(s, t) ds +
ix
Af(s,
t) ds.
Newton's second law states
that
the total force acting on P must equal the
mass of
P times its acceleration. This law takes the form
r+l:J.x a (
au
) l
x
+l:J.X
l
x
+l:J.X
a
2
u
ix
A
ax
k(s)
ax
(s, t) ds + x
Af(s,
t) ds = x Ap(s)
at
2
(s, t) ds,
where p(x)
is
the density of the bar
at
x (in units of mass per volume).
We
can
rewrite this as
l
x
+l:J.X
[a
2
u a (
au)
]
x p(s)
at
2
(s, t) -
ax
k(s)
ax
(s, t) -
f(s,
t) ds = 0
(note how the factor of A cancels). This integral must be zero for every x E
[0,
£)
and every
~x
>
O.
It
follows (by the reasoning introduced on page
12)
that
the
integrand must be identically zero; this gives the equation
a
2
u a (
au)
p(x)
at
2
-
ax
k(x)
ax
-
f(x,
t) = 0,
or
(2.14)
The
PDE
(2.14) is called the wave equation.
If
the bar
is
in equilibrium, then the displacement does not depend on t, and
we
can write u =
u(x).
In this case,
the
acceleration a
2
u/at
2
is
zero, and
the
forcing
function
f must also be independent of time.
We
then obtain the following ODE
for the equilibrium displacement of
the
bar:
d (
dU)
--
k(x)-
=
f(x).
dx dx
(2.15)
This
is
the same equation
that
governs steady-state heat
flow!
Just
as in the case
of steady-state heat
flow,
the resulting BVPs can be solved with two integrations
(see Examples 2.2 and 2.3).
24
Chapter
2.
Models
in one
dimension
If
the bar is
homogeneous,
so
that
p and k are
constants, these
last
two
differential
equations
can be
written
as
respectively.
2.2.1 Boundary conditions
for the
hanging
bar
Equation (2.15)
by
itself does
not
determine
a
unique displacement;
we
need bound-
ary
conditions,
as
well
as
initial conditions
if the
problem
is
time-dependent.
The
statement
of the
problem explicitly gives
us one
boundary
condition:
u(0)
=0
(the
top end of the bar
cannot
move).
Moreover,
we can
deduce
a
second boundary
condition
from
force
balance
at the
other
end of the
bar.
If the
bottom
of the bar
is
unsupported, then there
is no
contact
force
applied
at x = t. On the
other hand,
the
analysis
that
led to
(2.13) shows
that
the
part
of the bar
above
x
—
t
(which
is
all of the
bar) exerts
an
internal
force
of
and
or
simply
Since
the
wave equation involves
the
second
time derivative
of
u,
we
need
two
initial
conditions
to
uniquely determine
the
motion
of the
bar:
the
initial
displace-
ment
and the
initial velocity.
We
thus arrive
at the
following
IBVP
for the
wave
equation:
on
the
surface
at x = t.
Since there
is
nothing
to
balance this
force,
we
must have
24 Chapter
2.
Models
in
one dimension
If
the
bar
is
homogeneous, so
that
p
and
k are constants, these last two
differential equations can be written as
and
J2u
-k
dx
2
=
I(x),
respectively.
2.2.1 Boundary conditions for the hanging bar
Equation (2.15) by itself does not determine a unique displacement;
we
need bound-
ary conditions, as well as initial conditions if
the
problem is time-dependent.
The
statement
of
the
problem explicitly gives us one boundary condition:
u(O)
= 0 (the
top
end of
the
bar
cannot move). Moreover,
we
can deduce a second boundary
condition from force balance
at
the
other
end of
the
bar.
If
the
bottom
of
the
bar
is unsupported,
then
there is no contact force applied
at
x =
e.
On
the
other
hand,
the
analysis
that
led
to
(2.13) shows
that
the
part
of
the
bar
above x = e (which
is
all of
the
bar) exerts
an
internal force of
on
the
surface
at
x =
e.
Since there is nothing
to
balance this force,
we
must have
-Ak(f)
~~
(e)
= 0,
or simply
~~(f)=O.
Since
the
wave equation involves
the
second time derivative of
u,
we
need two
initial conditions
to
uniquely determine
the
motion of
the
bar:
the
initial displace-
ment and
the
initial velocity.
We
thus arrive
at
the
following IBVP for
the
wave
equation:
a
2
u a (
au)
p(x)
at
2
-
ax
k(x)
ax
=
I(x,
t), 0 < x <
e,
t>
to,
u(x,
to) =
'I/J(x),
0 < x <
e,
au
at
(x, to) =
"Y(x),
0 < x <
e,
(2.16)
u(O,
t) = 0,
t>
to,
au
ax
(e,
t)
= 0, t > to·
2.2.
The
hanging
bar 25
The
corresponding
steady-state
BVP
(expressing mechanical equilibrium)
is
There
are
several other sets
of
boundary conditions
that
might
be of
interest
in
connection with
the
differential
equations
(2.14)
or
(2.15).
For
example,
if
both
ends
of the bar are fixed
(not allowed
to
move),
we
have
the
boundary conditions
(recall
that
u
is the
displacement,
so the
condition
u(i]
= 0
indicates
that
the
cross-
section
at the end of the bar
corresponding
to x = t
does
not
move
from
its
original
position).
If
both ends
of the bar are
free,
the
corresponding boundary conditions
are
Any
of the
above boundary conditions
can be
inhomogeneous.
For
example,
we
could
fix one end of the bar at x = 0 and
stretch
the
other
to x = t +
A^.
This
experiment corresponds
to the
boundary conditions
w(0)
= 0,
u(t]
=
A£
As
another
example,
if one end of the bar
(say
x = 0) is fixed and a
force
F is
applied
to the
other
end (x =
£),
then
the
applied
force
determines
the
value
of
du/dx(i}.
Indeed,
as
indicated above,
the
restoring
force
of the bar on the x = t
cross-section
is
and
this must balance
the
applied
force
F:
This
leads
to the
boundary condition
and the
quantity
F/A
has
units
of
pressure
(force
per
unit
area).
For
mathematical
purposes,
it is
simplest
to
write
an
inhomogeneous boundary condition
of
this type
as
but for
solving
a
practical problem,
it is
essential
to
recognize
that
2.2. The hanging bar
25
The
corresponding
steady-state
BVP
(expressing mechanical equilibrium) is
d (
dU)
- dx k(x)
dx
=
f(x),
0<
x <
C,
u(O)
= 0,
(2.17)
~~(C)
=
o.
There
are several
other
sets
of
boundary
conditions
that
might be of interest
in
connection
with
the
differential equations (2.14) or (2.15). For example, if
both
ends of
the
bar
are
fixed (not allowed
to
move), we have
the
boundary
conditions
U(O)
= 0,
u(C)
= 0
(recall
that
u
is
the
displacement, so
the
condition
u(C)
= ° indicates
that
the
cross-
section
at
the
end
of
the
bar
corresponding
to
x = C does
not
move from its original
position).
If
both
ends of
the
bar
are
free,
the
corresponding
boundary
conditions
are
du
du
dx
(0)
=
0,
dx
(C)
=
o.
Any of
the
above
boundary
conditions
can
be inhomogeneous. For example,
we could
fix
one end of
the
bar
at
x = 0
and
stretch
the
other
to
x = C +
I::!.L
This
experiment corresponds
to
the
boundary
conditions
u(O)
= 0,
u(C)
=
tJ..£.
As
another
example, if one end of
the
bar
(say x =
0)
is fixed
and
a force F is applied
to
the
other
end (x =
C),
then
the
applied force determines
the
value of du/dx(C).
Indeed,
as
indicated above,
the
restoring force of
the
bar
on
the
x = C cross-section
is
-Ak(C)
~~
(C),
and
this
must
balance
the
applied force F:
du
-Ak(C)
dx
(C)
+ F =
O.
This
leads
to
the
boundary
condition
and
the
quantity
F / A has
units
of pressure (force
per
unit
area). For
mathematical
purposes,
it
is
simplest
to
write
an
inhomogeneous
boundary
condition
of
this
type
as
:~
(C)
=
c,
but
for solving a practical problem, it is essential
to
recognize
that
F
C = Ak(C)"

26
Chapter
2.
Models
in one
dimension
Exercises
1.
Consider
the
following
experiment:
A bar is
hanging with
the top end fixed
at x = 0, and the bar is
stretched
by a
pressure
(force
per
unit area)
p
applied
uniformly
to the
free
(bottom) end. What
are the
boundary conditions
describing this situation?
2.
Suppose
that
a
homogeneous
bar
(that
is, a bar
with constant
stiffness
A;)
of
length
i has its top end fixed at x = 0, and the bar is
stretched
to a
length
1+A£.
by a
pressure
p
applied
to the
bottom end. Take down
to be the
positive
x-direction.
(a)
Explain
why p and
A^
have
the
same
sign.
(b)
Explain
why p and
\t
cannot both
be
chosen arbitrarily (even subject
to the
requirement
that
they have
the
same sign). Give both physical
and
mathematical reasons.
(c)
Suppose
p is
specified.
Find
A^
(in
terms
of
p,
k,
and i).
(d)
Suppose
Al
is
specified.
Find
p (in
terms
of
A£,
fc, and
t}.
3. A
certain type
of
stainless steel
has a
stiffness
of 195
GPa.
(A
Pascal
(Pa)
is
the
standard
unit
of
pressure,
or
force
per
unit
area.
The
Pascal
is a
derived
unit:
one
Pascal
equals
one
Newton
per
square meter.
The
Newton
is the
standard
unit
of
force:
one
Newton equals
one
kilogram meter
per
second-
squared. Finally,
GPa is
short
for
gigaPascal,
or
10
9
Pascals.)
(a)
Explain
in
words (including units) what
a
stiffness
of 195 GPa
means.
(b)
Suppose
a
pressure
of 1 GPa is
applied
to the end of a
homogeneous,
circular
cylindrical
bar of
this stainless steel,
and
that
the
other
end is
fixed.
If
the
original length
of the bar is 1 m and its
radius
is 1 cm,
what
will
its
length
be, in the
equilibrium
state,
after
the
pressure
has
been
applied?
(c)
Verify
the
result
of 3b by
formulating
and
solving
the
boundary value
problem
representing this experiment.
4.
Consider
a
circular cylindrical bar,
of
length
1 m and
radius
1 cm,
made
from
an
aluminum alloy with
stiffness
70
GPa.
If the top end of the bar (x = 0)
is
fixed,
what
total
force
must
be
applied
to the
other
end (x = 1) to
stretch
the bar to a
length
of
1.01
m?
5.
Write
the
wave equation
for the bar of
Exercise
3,
given
that
the
density
of
the
stainless steel
is 7.9
g/cm
3
.
(Warning:
Use
consistent units!) What must
the
units
of the
forcing
function
/ be?
Verify
that
the two
terms
on the
left
side
of the
differential
equation have
the
same units
as /.
6.
Suppose
that
aim
bar of the
stainless steel described
in
Exercise
3,
with
density
7.9g/cm
3
,
is
supported
at the
bottom
but
free
at the
top.
Let the
cross-sectional
area
of the bar be
O.lm
2
.
A
weight
of
1000
kg is
placed
on
26
Chapter
2.
Models
in
one
dimension
Exercises
1. Consider the following experiment: A
bar
is
hanging with the top end fixed
at
x =
0,
and
the
bar
is
stretched by a pressure (force per unit area) p
applied uniformly
to
the free (bottom) end.
What
are
the
boundary conditions
describing this situation?
2.
Suppose
that
a homogeneous
bar
(that
is, a
bar
with constant stiffness
k)
of
length
£ has its top end fixed
at
x = 0, and the bar
is
stretched
to
a length
£
+ Il£ by a pressure p applied
to
the
bottom end. Take down
to
be the positive
x-direction.
(a) Explain why
p and Il£ have the same sign.
(b) Explain why p
and
Il£ cannot
both
be chosen arbitrarily (even subject
to
the
requirement
that
they have the same sign). Give both physical
and mathematical reasons.
(c)
Suppose p
is
specified. Find Il£ (in terms of p, k, and
C).
(d) Suppose Il£
is
specified. Find p (in terms of Il£, k, and
C).
3.
A certain type of stainless steel has a stiffness of
195
GPa.
(A
Pascal (Pa)
is
the
standard
unit of pressure, or force per unit area. The Pascal
is
a derived
unit: one Pascal equals one Newton per square meter. The
Newton
is
the
standard unit of force: one Newton equals one kilogram meter per second-
squared. Finally,
GPa
is
short for gigaPascal, or
10
9
Pascals.)
(a) Explain in words (including units) what a stiffness of
195
GPa
means.
(b) Suppose a pressure of 1
GPa
is applied to the end of a homogeneous,
circular cylindrical
bar
of this stainless steel, and
that
the other end
is
fixed.
If
the original length of
the
bar
is
1 m and its radius
is
1 cm, what
will its length be, in the equilibrium state, after the pressure has been
applied?
(c)
Verify the result of 3b by formulating and solving the boundary value
problem representing this experiment.
4.
Consider a circular cylindrical bar, of length 1 m
and
radius 1 cm, made from
an aluminum alloy with stiffness
70
GPa.
If
the top end of the
bar
(x =
0)
is fixed, what total force must be applied
to
the other end (x =
1)
to
stretch
the
bar
to
a length of 1.01 m?
5.
Write the wave equation for the
bar
of Exercise
3,
given
that
the density of
the stainless steel
is
7.9
g/cm
3
.
(Warning: Use consistent units!)
What
must
the units of the forcing function
f be? Verify
that
the two terms on the left
side of
the
differential equation have the same units as f.
6.
Suppose
that
aIm
bar of the stainless steel described in Exercise
3,
with
density 7.9
g/
cm
3
,
is
supported
at
the bottom
but
free
at
the
top. Let the
cross-sectional area of the
bar
be 0.1 m
2
•
A weight of 1000 kg
is
placed on

2.3.
The
wave
equation
for a
vibrating
string
27
top of the
bar,
exerting pressure
on it via
gravity (the gravitational
constant
is
9.8m/s
2
).
The
purpose
of
this problem
is to
compute
and
compare
the
effects
on the bar
of
the
mass
on the top and the
weight
of the bar
itself.
(a)
Write down three BVPs:
i.
First,
take
into account
the
weight
of the bar
(which means
that
gravity induces
a
body
force),
but
ignore
the
mass
on the top (so the
top end of the bar is
free—the
boundary condition
is a
homogeneous
Neumann
condition).
ii.
Next, take into account
the
mass
on the
top,
but
ignore
the
effect
of
the
weight
of the bar (so
there
is no
body
force).
iii.
Last, take both
effects
into account.
(b)
Explain
why the
third
BVP can be
solved
by
solving
the first two and
adding
the
results.
(c)
Solve
the first two
BVPs
by
direct integration. Compare
the two
dis-
placements.
Which
is
more significant,
the
weight
of the bar or the
mass
on
top?
(d)
How
would
the
situation change
if the
cross-sectional
area
of the bar
were
changed
to
0.2m
2
?
7.
(a)
Show
that
the
function
2.3 The
wave
equation
for a
vibrating
string
We
now
present
an
argument
that
the
wave equation (2.14) also describes
the
small
transverse vibrations
of an
elastic string (such
as a
guitar string).
In the
course
of
the
following
derivation,
we
make several
a
priori
unjustified
assumptions which
are
significant enough
that
the end
result ought
to be
viewed with some skepticism.
However,
a
careful
analysis leads
to the
same model (see
the
article
by
Antman
[1]).
For
simplicity,
we
will assume
that
the
string
in
question
is
homogeneous,
so
that
any
material properties
are
constant throughout
the
string.
We
suppose
that
the
string
is
stretched
to
length
I
and
that
its two
endpoints
are not
allowed
to
move.
We
further
suppose
that
the
string vibrates
in the
xy-plane,
occupying
the
interval
[0,1]
on the
x-axis
when
at
rest,
and
that
the
point
at
(x,0)
in the
(b)
What values
of 9
will
cause
u
to
also
satisfy
homogeneous Dirichlet con-
ditions
at x = 0 and x =
tl
is
a
solution
to the
homogeneous wave equation
2.3. The
wave
equation for a vibrating string
27
top of
the
bar, exerting pressure on it via gravity (the gravitational constant
is
9.8m/s
2
).
The purpose of this problem
is
to compute and compare
the
effects on the
bar
of the
maBS
on the
top
and
the
weight of the
bar
itself.
(a) Write down three BVPs:
1. First, take into account the weight of
the
bar (which means
that
gravity induces a body force),
but
ignore
the
maBS
on the top (so the
top
end of the
bar
is
free-the
boundary condition
is
a homogeneous
Neumann condition).
ii. Next, take into account the mass on
the
top,
but
ignore the effect of
the
weight of the bar (so there
is
no body force).
iii.
LaBt,
take
both
effects into account.
(b) Explain why the third BVP can be solved by solving the first two
and
adding the results.
(c)
Solve the first two BVPs by direct integration. Compare the two dis-
placements. Which
is
more significant, the weight of the
bar
or the
maBS
on top?
(d)
How
would the situation change
if
the cross-sectional area of the bar were
changed
to
0.2 m
2
?
7.
(
a)
Show
that
the function
u(x, t) = cos
(c(}t)
sin
((}x)
is
a solution
to
the homogeneous wave equation
(b)
What
values of
()
will cause u
to
also satisfy homogeneous Dirichlet con-
ditions
at
x = 0 and x =
£?
2.3
The
wave equation for a vibrating string
We
now present an argument
that
the wave equation (2.14) also describes
the
small
transverse vibrations of an elastic string (such
aB
a guitar string). In
the
course
of the following derivation,
we
make several a priori unjustified assumptions which
are significant enough
that
the end result ought
to
be viewed with some skepticism.
However, a careful analysis leads
to
the same model (see
the
article by Antman
[1]).
For simplicity,
we
will assume
that
the string in question
is
homogeneous,
so
that
any material properties are constant throughout the string.
We
suppose
that
the string
is
stretched
to
length £ and
that
its two endpoints are not allowed
to
move.
We
further suppose
that
the
string vibrates in the xy-plane, occupying
the
interval
[0,
£]
on
the
x-axis when
at
rest, and
that
the point
at
(x,O)
in the
