Galdi G.P. An Introduction to the Mathematical Theory of the Navier-Stokes Equations: Steady-State Problems
Подождите немного. Документ загружается.

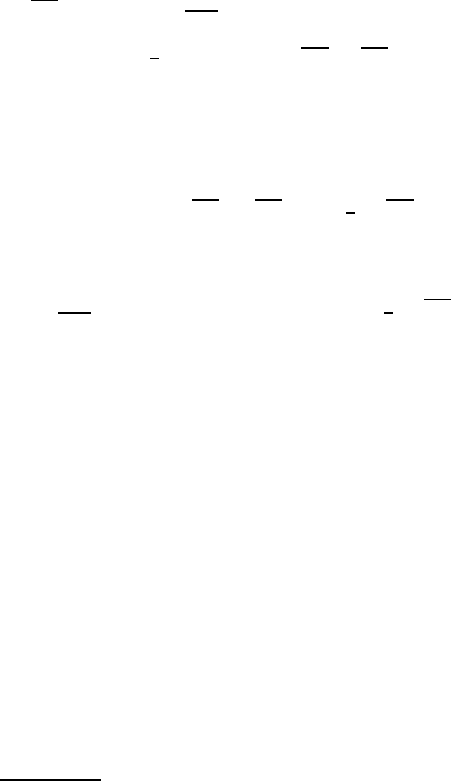
506 VIII Steady Generalized Oseen Flow in Exterior Domains
−∇ × (ψ
R
∇× v), and integrate over Ω. Since
1
−∇ × (ψ
R
∇ × v) = −ψ
R
∇× ∇ × v + (∇ × v) ×∇ψ
R
= ψ
R
∆v + (∇ × v) × ∇ψ
R
,
(VIII.2.4)
we obtain
2
k
√
ψ
R
∆vk
2
2
= −
R
∂v
∂x
1
+ f, ψ
R
∆v + (∇ × v) × ∇ψ
R
−
1
2
((∇ × v) × ∇(
√
ψ
R
),
√
ψ
R
∆v)
+T (e
1
× v, ∇× (ψ
R
∇ × v) − (e
1
× x · ∇v, ∇× (ψ
R
∇ × v)) .
(VIII.2.5)
By the Schwarz i nequality, the Cauchy inequali ty (II.2.5), and the properties
of ψ
R
, we obtain
− ((∇ × v) × ∇(
p
ψ
R
),
p
ψ
R
∆v) ≤
1
4
k
p
ψ
R
∆vk
2
2
+ M
2
|v|
2
1,2
(VIII.2.6)
and
−
R
∂v
∂x
1
+f , ψ
R
∆v+(∇ × v) × ∇ψ
R
≤
1
4
k
p
ψ
R
∆vk
2
2
+ c (|v|
2
1,2
+ kfk
2
2
) ,
(VIII.2.7)
where c = c(B, ρ, r). We need now to estimate the last two terms on the
right-hand side of (VIII.2.5). Employing the well-known identities
∇ · (A ×B) = B · ∇ ×A − A · ∇ × B ,
∇ × (a × B) = a ∇ · B − a ·∇B ,
(VIII.2.8)
where A, B are vector fields and a is a constant vector, along with the prop-
erties of ψ
R
, we obtain
(e
1
× v, ∇ × (ψ
R
∇ × v)) = −
Z
Ω
∇ · [ψ
R
(e
1
×v) × (∇ × v)]
+ (ψ
R
∇× v, ∇ × (e
1
×v))
= −(ψ
R
e
1
· ∇v, ∇×v) ≤ 2|v|
2
1,2
.
(VIII.2.9)
Furthermore, a gain with the help of (VIII.2.8)
1
, and by the properties of ψ
R
,
we deduce
1
This vector identity as well as the others used throughout the proof is known for
“smooth” vector fields. However, by a simple approximating procedure based on
Theorem II.3.1, we can show that they continue to hold a.e. in Ω
ρ
also if the
fields merely belong to W
2,2
loc
(Ω
ρ
).
2
Throughout t he proof, for simplicity, we set k ·k
2,Ω
ρ
≡ k·k
2
, and (·, ·)
Ω
ρ
≡ (·, ·).
VIII.2 Generalized Solutions. Uniqueness 507
−(e
1
× x · ∇v, ∇× (ψ
R
∇ × v)) =
Z
Ω
∇ · [ψ
R
(e
1
× x · ∇v) × (∇×v)]
−(ψ
R
∇ × v, ∇× (e
1
×x ·∇v))
= −(ψ
R
∇ × v, ∇× (e
1
×x ·∇v)) .
(VIII.2.10)
In order to estimate the term on the right-hand side of (VIII.2.10), we recall
the following classical form o f ∇ × A, and that of the cross product of two
vectors, a, b in terms of their components:
∇× A = ε
ijk
D
j
A
k
e
i
, a × b = ε
ijk
a
j
b
k
e
i
,
where ε
ijk
is the alternating symbol.
3
We thus have, for i = 1, 2, 3,
[∇× (e
1
×x · ∇v)]
i
= ε
ijk
D
j
(e
1
× x · ∇v
k
)
= [e
1
×x ·∇(∇ × v)]
i
+ ε
ijk
ε
lmj
e
m
D
l
v
k
,
and so, employing the well- known identities (see, e.g., Evett (1966))
ε
ijk
ε
lmj
= δ
im
δ
kl
− δ
il
δ
km
,
and recalling (VIII.2.3)
2
, we deduce
∇ × (e
1
× x · ∇v) = e
1
× x · ∇(∇ × v) − ∇v · e
1
.
Taking into account that e
1
×x ·∇ψ
R
(|x|) = 0, for all x ∈ R
3
, from this latter
relation and again from the properties of ψ
R
, we obtain
−(ψ
R
∇ × v, ∇×(e
1
× x · ∇v)) = −
1
2
Z
Ω
∇ · [ψ
R
(∇ × v)
2
e
1
×x]
+ (ψ
R
∇ × v, ∇v · e
1
)
= (ψ
R
∇ × v, ∇ v ·e
1
) ≤ 2|v|
2
1,2
.
(VIII.2.11)
Collecting (VIII.2.5)–(VIII.2.7), (VII I.2.9), and (VIII.2.11), we thus obtain
k
p
ψ
R
∆vk
2
2
≤ c (|v|
2
1,2
+ kfk
2
2
) , (VIII.2.12 )
and so, recalling that ψ
R
(|x|) = 1, for x ∈ Ω
r,R
, we conclude, in particular,
that
k∆vk
2
2,Ω
r,R
≤ c (| v|
2
1,2
+ kfk
2
2
)
where c is independent of R. Letting R → ∞ in this relation gives ∆v ∈
L
2
(Ω
r
), for all r > ρ, and moreover,
k∆vk
2,Ω
r
≤ c (|v|
1,2
+ kfk
2,Ω
ρ
) . (VIII.2.13)
3
See footnote 4 in Section VIII.1.
508 VIII Steady Generalized Oseen Flow in Exterior Domains
From (VIII.2.13) and from its proof, it immediately follows that in the par-
ticular case Ω = R
3
and f ∈ L
2
(R
3
), we have ∆v ∈ L
2
(R
3
) and
k∆vk
2,R
3 ≤ c
1
(|v|
1,2
+ kfk
2,R
3 ) , (VI II.2.14)
with c
1
= c
1
(B). We now observe that as a consequence of this property and
the Helmholtz–Weyl decomposition theorem, Theorem III.1.1 , v satisfies the
following Stokes system:
∆v = F + ∇ζ
∇ · v = 0
)
in Ω
r
,
where F ∈ H(Ω
r
) ⊂ L
2
(Ω
r
) and ζ ∈ D
1,2
(Ω
r
). Moreover, v ∈ D
1,2
(Ω
r
) by
assumption, and so f rom Theorem V.5.3, we readily obtain v ∈ D
2,2
(Ω
r
), and
the property stated in the lemma follows. Clearly, if Ω = R
3
and f ∈ L
2
(R
3
),
this argument furnishes D
2
v ∈ L
2
(R
3
). Therefore, since
v ∈ D
2,2
(R
3
) ∩ D
1,2
0
(R
3
) ,
by Theorem II.7.6 it easily follows that v ∈ D
2,2
0
(R
3
). Thus, by Exercise II.7.4,
we have
kD
2
vk
2
= k∆vk
2
,
and from (VIII.2.14) we also prove (VIII.2.2). The case Ω 6= R
3
can be treated
by similar considerations. In fact, l et ζ = ζ(x) a smooth function that is 1
for | x| ≥ r and is 0 for |x| ≤ ρ, and set w = ζ v. Then, by the property
of generalized solutions and with the help of Theorem II.7.6 we prove that
w ∈ D
2,2
0
(R
3
), and so, as before, we find that kD
2
wk
2
= k∆wk
2
. However,
by a simple calcula tion, we show that
kD
2
vk
2,Ω
r
≤ k∆wk
2
≤ c
1
(r) (k∆vk
2,Ω
r
+ kvk
1,2,Ωρ,r
)
≤ c
2
(r) (k∆vk
2,Ω
r
+ |v|
1,2
) ,
(VIII.2.15)
where in the last step, we have used the H¨o lder inequality together with
inequality kvk
6
≤ c| v|
1,2
. The relation (VIII.2.1) is then a consequence of
(VIII.2.13) and (VIII.2.15).
ut
The next result furnishes a representation of the pressure field under very
general assumptions on f .
Lemma VIII.2.2 Let v be a generalized soluti on to (VIII.0.2), (VIII.0.7)
corresponding to f =
e
f + ∇ · F , where
e
f ∈ L
2
(Ω
ρ
) ∩ L
q
(Ω
ρ
), for some ρ > δ(Ω
c
) and q ∈ (1, ∞) ,
and F is a second-order tensor field such that

VIII.2 Generalized Solutions. Uniqueness 509
∇· F ∈ L
2
(Ω
ρ
) , F ∈ L
t
(Ω
ρ
) ∩ L
2
(Ω
r
) ∩ L
q
(Ω
r
) , for some t ∈ (1, ∞).
Then, there exists p
∞
∈ R such that the pressure p associated to v by L emma
VIII.1.1 admits the following decomposition:
p = p
∞
+ p
1
+ p
2
, (VIII.2.16 )
where
p
1
∈ L
6
(Ω
r
) ∩D
1,2
(Ω
r
) ∩ D
1,q
(Ω
r
) ,
p
2
∈ L
6
(Ω
r
) ∩L
t
(Ω
r
) ∩ D
1,2
(Ω
r
) .
(VIII.2.17)
Moreover, if q ∈ (1, 3), we have al so
p
1
∈ ∩L
3q/(3−q)
(Ω
r
) . (VIII. 2.18)
Finally, if
(f, ∇ ψ) = 0 for all ψ ∈ C
∞
0
(Ω
ρ
) , (VIII.2.19)
namely, ∇ ·f = 0 in the generalized sense, and q ∈ (1, 3/2], t ∈ (1, 3], then
D
α
(p(x) − p
∞
) = χ
α
(x) , for all |α| ≥ 0, |x| > r , (VIII.2.20)
where
χ
α
(x) = O(|x|
−2−|α|
) .
Proof. By the Helmholtz–Weyl decomposition theorem, Theorem III.1.2, we
may write
e
f = f
∗
+ ∇p
∗
,
where f
∗
, ∇p
∗
∈ L
2
(Ω
ρ
) ∩L
q
(Ω
ρ
). By Theorem II.6.1, we can add a constant
to p
∗
in such a way that the modified function, which we continue to denote
by p
∗
, belongs to L
6
(Ω
ρ
)∩L
3q/(3−q)
(Ω
ρ
). We set ep = p+p
∗
, so that (VIII.2.3)
becomes
∆v + R
∂v
∂x
1
+ T (e
1
× x · ∇v − e
1
× v) = ∇ep + f
∗
+ ∇· F
∇· v = 0
a.e. in Ω
ρ
.
(VIII.2.21)
We begin by proving the properties (VIII.2.16)–(VIII.2.18). Fix r > ρ, and
let ψ be a smooth “cut-off” f unction that is 1 if |x| ≥ r, and 0 if |x| ≤ ρ.
Moreover, let
σ(x) := −
Z
∂B
r
v · n
∇E , (VIII.2.22)
where E is the (three-dimensional) fundamental Laplace solution (II.9.1), and
consider the following problem:

510 VIII Steady Generalized Oseen Flow in Exterior Domains
∇ · H = ∇ · [ψ(v + σ)] in B
r
,
H ∈ W
3,2
(R
3
) , supp (H) ⊂ B
r
.
(VIII.2.23)
Since
Z
B
r
∇ · [ψ(v + σ)] =
Z
∂B
r
ψ(v + σ) · n = 0 ,
we know, by the properties of v and Theorem II I.3.1, that (VIII.2.23) has at
least one solution. Put
w = ψv + ψσ −H , π = ψ ep.
Taking into account (VIII.1.8), from (VIII. 2.21) by a direct calculation we
obtain
4
∆w +
∂w
∂x
1
+ (e
1
× x · ∇w − e
1
×w) = ∇π + ψf
∗
+ ψ∇·F + G
∇ · w = 0
a.e. in R
3
,
(VIII.2.24)
where G ∈ L
2
(R
3
), with supp (G) ⊂ B
r
. Now, for all ψ ∈ C
∞
0
(Ω
ρ
),
(∆w, ∇ψ) = −(∇ · w, ∆ψ) = 0 ,
∂w
∂x
1
, ∇ψ
=
∇ · w,
∂ψ
∂x
1
= 0 .
(VIII.2.25)
Also, since
∇ · (e
1
×x ·∇Φ − e
1
× Φ) = e
1
× x · ∇(∇ · Φ) = 0 ,
for all Φ ∈ W
2,2
loc
(Ω) with ∇ ·Φ = 0 ,
(VIII.2.26)
we obtain, in particular,
(e
1
×x·∇w−e
1
×w, ∇ψ) = −(∇·(e
1
×x·∇ w−e
1
×w), ψ) = 0 . (VIII.2.27 )
Using (VIII.2.25) and (VIII.2.27) from (VIII.2.24)
1
, we deduce that π is a
generalized solution to the foll owing problem:
− ∆π = ∇ ·(ψf
∗
) + ∇ · (ψ∇ · F ) + ∇ · G in R
3
. (VIII.2.28)
A solution to (VIII. 2.28) is given by
π = −∇E ∗ (ψf
∗
) −∇E ∗ G + ∇E ∗ (∇ψ · F ) − ∇E ∗ [∇· (ψF )]
≡
4
X
i=1
π
i
,
(VIII.2.29)
4
Since their values are irrelevant to the proof, we set throughout R = T = 1.
VIII.2 Generalized Solutions. Uniqueness 511
where E i s the fundamental solution of Laplace’s equation (II.9.1). We recall
that |∇E(ξ)| ≤ c|ξ|
−2
, and that D
ij
E is a singular kernel, for all i, j = 1, 2, 3
(see Exercise II.11.7). Therefore, ta king into account that ψf
∗
, G, ∇ψ · F ∈
L
q
(R
3
) ∩ L
2
(R
3
), q ∈ (1, 3), from Theorem II.11.3 and Theorem II. 11.4 we
obtain
π
i
∈ L
6
(R
3
) ∩ D
1,q
(R
3
) ∩ D
1,2
(R
3
), q ∈ (1, ∞)
π
i
∈ L
3q/(3−q)
(R
3
) ∩ L
6
(R
3
) ∩ D
1,q
(R
3
) ∩ D
1,2
(R
3
), q ∈ (1, 3)
)
i = 1, 2, 3,
(VIII.2.30)
and by the same token,
π
4
∈ L
t
(R
3
) ∩ L
6
(R
3
) ∩ D
1,2
(R
3
) ; (VIII.2.31)
see Exercise VIII.2.2 . L et us show that (up to an additive constant) π = π in
Ω
r
. To this end, we observe that clearly, Ψ := π − π is harmonic in R
3
, for
which the fol lowing local representation holds (see (VI I.4. 57)):
Ψ(x) = −
Z
R
3
H
(R)
(x − y)Ψ(y)dy x ∈ R
3
,
with H
(R)
satisfying (V. 3.11)–(V.3.13). Therefore, by differentiating bo th
sides of this relation and using the Schwarz and H¨older inequalities, we deduce
|∇Ψ (x)| ≤
Z
R
3
|y|
2
|H
(R)
(x − y)|
2
dy
1/2
∇π
y
2
+k∇H
(R)
k
3q/(4q−3)
kπk
3q/(3q−3)
.
(VIII.2.32)
Also, recalling the properties (V. 3.11), (V.3.13) of the function H
(R)
and
noticing that |y|
2
≤ 2(|x − y|
2
+ |x|
2
), from (VIII.2 .32) we obtain
|∇Ψ (x)| ≤ c
1
1 + | x|
√
R
∇π
y
2
+ c
2
R
−5+4q/3
kπk
3q/(3q−3)
, (VIII.2.33)
for a ll fixed x ∈ R
3
and all R > 0 . On the other ha nd, dividing b oth sides of
(VIII.2.21)
1
by |x|, squaring the resulting equation and integrating over Ω
r
,
r > ρ, we deduce
∇ep
|x|
2,Ω
r
≤ c
"
k∆vk
2,Ω
r
+ |v|
1,2,Ω
r
+ kfk
2,Ω
r
+
v
|x|
2,Ω
r
#
, (VIII.2.34)
where c = c(r). From Lemma VIII.2.1 and the assumption on f , we infer that
∆v ∈ L
2
(Ω
r
). Therefore, taking into account that v ∈ D
1,2
(Ω), we deduce
that the first three terms on the right-hand side of (VIII.2.34) are finite.
Moreover, by Theorem II.6.1(i) and property (iv) in Definition VIII.1.1, we
have also that the last term on the right-hand side of (VIII.2.34) is finite, so
that we conclude, in particular, that
512 VIII Steady Generalized Oseen Flow in Exterior Domains
Z
Ω
r
∇ep
x
2
< ∞,
namely, since π = ψep and ep ∈ L
2
loc
(Ω
ρ
),
Z
R
3
∇π
x
2
< ∞.
If we take into account this conditio n al ong with (VIII.2.30), and pass to the
limit R → ∞ in (VIII.2 .33), we conclude that ∇Ψ(x) = 0, for all x ∈ R
3
,
that is, π = π + const. Therefore, since π(x) = ep(x) ≡ p(x) + p
∗
(x), x ∈
Ω
r
, the desired summability property (VIII.2.16)–(VIII.2.17) for p fol lows
from thi s latter, the analogous property of p
∗
, and (VIII.2.29)–(VIII.2.31). We
shall now complete the pro of of the theorem. Using (VIII.2 .25), (VIII.2.27)
(with w ≡ v), and bearing in mind (VIII.2.19), from (VIII.2.2 1) we obtain
(∇p, ∇ψ) = 0, for a ll ψ ∈ C
∞
0
(Ω
ρ
), whi ch, i n turn, by well-known results
of Ca ccioppoli (1937), Cimmino (1 938a, 19 38b), and Weyl (1940), i mplies
that p is harmonic in Ω
ρ
and belongs to C
∞
(Ω
ρ
). Recalling that p satisfies
(VIII.2.16)–(VIII.2.17), we may use the results of Exercise V.3.6(i) to obtain
the following representation for ep(x), for al l x ∈ Ω
r
:
D
α
p(x) = −
Z
∂B
r
∂ep
∂n
`
n
`
D
α
E(x) + χ
α
(x) , for all |α| ≥ 0. (VIII.2.35)
Take α = 0 in this relation. Then, if q ∈ (1, 3/2], t ∈ (1, 3], by (VIII. 2.16),
(VIII.2.17) both p and χ
0
are summable in Ω
r
with some exponent s ∈
(3/2, 3], and since E 6∈ L
s
(Ω
r
), this impl ies that the surface integral in
(VIII.2.35) must vanish, which concludes the proof of the lemma. ut
Remark VI II.2.1 As the reader may have noticed, the proof of the previous
lemma remains una ltered if we take T = 0.
We are now in a position to prove the following.
Lemma VIII.2.3 Let Ω be locally Lipschitz and let u be a generalized so-
lution to (VI II.0.2), (VIII.0.7) correspondi ng to f ≡ v
∗
≡ 0. Moreover, de-
note by p the associated pressure field from Lemma VIII.1.1. Then u ≡ 0,
p ≡ p
1
+ const.
Proof. By assumption, we have
(∇u, ∇ψ)−R
∂u
∂x
1
, ψ
−T (e
1
×x·∇u−e
1
×u, ψ) = (π, ∇·ψ) , (VI II.2.36)
for all ψ ∈ C
∞
0
(Ω). By Theorem I II.5.1, we obtain that u ∈ D
1,2
0
(Ω), whi le
by Theorem VIII.1.1, it also follows that u, p ∈ C
∞
(Ω). Employing this latter
property and (VIII.2.36), we deduce that
VIII.2 Generalized Solutions. Uniqueness 513
∆u + R
∂u
∂x
1
+ T (e
1
×x · ∇u −e
1
×u) = ∇π
∇ · u = 0
in Ω . (VIII.2.37)
Now by Lemma VI II.2.2, we have
π(x) − π
∞
= O(|x|
−2
) , as |x| → ∞, (VIII.2.38)
for some π
∞
∈ R. Since (VIII.2.36) remains unchanged if we replace π with
π −π
∞
, we will take, without loss, π
∞
= 0. Our next objective i s, basically, to
replace ψ in (VIII.2.36) with u. In order to reach this goal, we begin to notice
that since D
1,2
0
(Ω) ⊂ W
1,2
(Ω
R
), for all R > δ(Ω
c
), one can show, by a simple
density argument, that (VIII.2.36) continues to hold for ψ ∈ W
1,2
0
(Ω
R
), for
all R > δ(Ω
c
). We next choose ψ = ψ
4,R
u, where ψ
α,R
is the “cut-off”
function determined in Lemma II.6.4. We recall that by that lemma, we have,
in particular,
supp (ψ
4,R
) ⊂ Ω
R
√
2
,
lim
R→∞
ψ
4,R
(x) = 1 uni formly pointwise ,
∂ψ
4,R
∂x
1
3/2
≤ C
1
, ku |∇ψ
4,R
|k
2
≤ C
2
|u|
1,2,Ω
R
√
2
,
(e
1
× x) · ∇ψ
4,R
(x) = 0 for all x ∈ R
3
.
(VIII.2.39)
Thus, replacing ψ
4,R
u for ψ in (VIII.2.36), we obtain after a few integrations
by parts (for notati onal simplicity, we drop the subscript “
4
”),
0 = (ψ
R
∇u, ∇u) + (∇ψ
R
· ∇u, u) +
R
2
u
2
,
∂ψ
R
∂x
1
+
T
2
(e
1
×x · ∇ψ
R
, u
2
) − (π, ∇ψ
R
· u)
= (ψ
R
∇u, ∇u) + (∇ψ
R
· ∇u, u) +
R
2
u
2
,
∂ψ
R
∂x
1
−(π, ∇ψ
R
· u) .
From this relation, with the help of (VIII. 2.39) and the Schwarz and H¨older
inequalities, we derive
k
p
ψ
R
∇uk
2
2
≤ c
|u|
2
1,2,Ω
R
√
2
+ Rkuk
2
6,Ω
R
√
2
+ kπk
2
|u|
1,2,Ω
R
√
2
, (VIII.2.40)
with c independent of R. From (VIII.2.38 ) we k now that π ∈ L
2
(Ω), whereas
by Theorem II.7.5, we have u ∈ L
6
(Ω). Therefore, in v iew of these properties,
we may let R → ∞ in (VIII.2 .40) to deduce
lim
R→∞
k
p
ψ
R
∇uk
2
= 0 ,

514 VIII Steady Generalized Oseen Flow in Exterior Domains
which, in turn, by (VIII.2.39)
2
and by the Lebesgue dominated convergence
theorem of Lemma II.2.1, shows that k∇uk
2
= 0, that is, u ≡ 0 in Ω. The
proof of the theorem is thus completed. ut
From the previous lemma, we at once deduce the following uniqueness
theorem, which constitutes the main accomplishment of this section.
Theorem VIII.2.1 Let Ω be locally Lipschitz and let v be a generalized
solution to (VIII.0.2), (VIII.0 .7) corresponding to f ∈ W
−1,2
0
(Ω
0
), Ω
0
any
bounded subdomain with Ω
0
⊂ Ω, and to v
∗
∈ W
1/2,2
(∂Ω). Moreover, denote
by p the asso ciated pressure field from Lemma VIII.1.1. Then, if w is another
generalized solution corresponding to the same data, with associated pressure
field p
1
, we have v ≡ w, p ≡ p
1
+ const.
Exercise VIII.2.1 Let v be a generalized soluti on to (VIII.0.2), (VIII.0.7), and
suppose that f satisfies the assumption of Lemma VIII .2.1. Show that
lim
|x|→∞
v(x) = 0 ,
uniformly pointwise. Hint: Couple the results of Lemma VIII.2.1 with those of The-
orem II.9.1.
Exercise VIII.2.2 Prove properties (VIII. 2.30), (VIII.2.31).
VIII.3 The Fundamental Solution to the
Time-Dependent Oseen Problem and Related Properti es
As we observed in the Introduction to thi s chapter, the asymptotic proper-
ties o f generalized solutions to (VIII.0.7), (VII I.0.2) will be established by
first proving similar properties for the solutions to an associated i nitial-value
problem, and then by showing that in the limit as t → ∞, the latter converge
to the former, uniformly in suitable no rms.
Actually, as we shall show later on, in order to achieve this goal, it suffices
to investigate the above properties for solutions to the following Oseen initial-
value problem:
∂u
∂t
= ∆u + R
∂u
∂x
1
−∇φ + f
∇ · u = 0
in R
3
× (0, ∞)
u(x, 0) = u
0
,
(VIII.3.1)
where f = f (x, t) and u
0
= u
0
(x) are given vector fields, with u
0
solenoidal,
satisfying appropriate assumptions. In turn, the study of the asymptotic prop-
erties (in space and time) of solutions to probl em (VIII.3.1) is more conve-
niently done by means of the integral representation of the solutions through
VIII.3 Fundamental Solution to the Time-Dependent Oseen Problem 515
the fundamental tensor solution o f the Oseen system (VIII.3.1)
1,2
. Such a
solution is well known, and was originally introduced by Oseen (1927, §5).
The objective of this section will thus be to recall some of the relevant
properties of the Oseen fundamental solution, and to establish certain uniform
properties concerning its asymptotic behavior i n space and ti me.
Since the study of all principal properties of this solution will be performed
in great depth in Volume II,
1
we shall restrict ourselves to state those we need
here, without detailed proofs, referring the reader to the existing literature for
all the missing details.
Following Oseen (1927, §5), for all R ≥ 0 we introduce tensor and vector
fields, Γ and γ, respectively, defined by
2
Γ
ij
(x −y, t −τ; R) = −δ
ij
∆Ψ(|x + R(t − τ )e
1
− y|, t − τ )
+
∂
2
∂y
i
∂y
j
Ψ(|x + R(t − τ )e
1
−y|, t − τ )
γ
j
(x − y, t −τ) =
∂
∂y
j
(∆ +
∂
∂τ
)Ψ(|x + R(t − τ )e
1
−y|, t − τ ) ,
(VIII.3.2)
where Ψ = Ψ (r, s) is any real function that is defined and smooth for all
r ≥ 0 and all s > 0. By a straightforward calculation, we show that the fields
(VIII.3.2) satisfy the following equations:
∂Γ
ij
∂τ
− R
∂Γ
ij
∂y
1
+ ∆Γ
ij
−
∂γ
j
∂y
i
= −δ
ij
∂
∂τ
+ ∆
∆Ψ ,
∂Γ
ij
∂y
i
= 0 ,
(VIII.3.3)
where “∆” operates on the y-variables. The idea is now to choose Ψ in such
a way that
∆Ψ(r, s) = −W (r, s) , (VII I.3.4)
where W = W (r, s) is the three-dimensional Weierstrass kernel, namely, the
fundamental solution to the three-dimensional heat equation:
W =
(
(4π s)
−3/2
e
−
r
2
4s
, s > 0 ,
0 , s ≤ 0 .
(VIII.3.5)
This choice will then ensure that the right-hand side of (VIII.3.3)
1
vanishes
for x, y ∈ R
3
and t > τ, and moreover, that when τ → t
−
, it becomes
“appropriately singular,” in a way that will be clarified later on, in Lemma
VIII.3.1. The requirement (VIII.3.4) means that
1
Actually, in any space dimensions n ≥ 2.
2
The properties that we will state in this section continue to hold also for R < 0.
However, for the application we have in mind, we suppose that R is nonnegative.
