Galdi G.P. An Introduction to the Mathematical Theory of the Navier-Stokes Equations: Steady-State Problems
Подождите немного. Документ загружается.


456 VII Steady Oseen Fl ow in Exterior Domains
kGk
s
1
≤ ckGk
1,q
, s
1
=
(n + 1)q
n + 1 − q
, 1 < q < n + 1, (VII.4 .49)
and so, from (VII.4.41)
2,3
, (VII. 4.48), and (VII.4.49) it foll ows that
kwk
1,q
+ kτ k
q
≤ c
10
(kgk
q
+ |g|
−1,q
) , 1 < q < ∞,
kwk
s
1
≤ c
11
(kgk
q
+ |g|
−1,q
) , s
1
=
(n + 1)q
n + 1 − q
, 1 < q < n + 1.
(VII.4.5 0)
In view of (VII.4.9), (VII.4.44)–(VII.4.47), (VII. 4.50), and using the replace-
ments (VII.4.3 ) we may conclude that, for given f and g from C
∞
0
(R
n
) prop-
erties stated previously, there exists a q-weak solution to (VII.4.1) satisfy-
ing to gether w ith the corresponding pressure p, the following estimates: if
1 < q < ∞
|v|
1,q
+ kpk
q
≤ c (R|f|
−1,q
+ R|g|
−1,q
+ kgk
q
)
Rkv
2
k
q
+ |v|
1,q
+ kpk
q
≤ c (R|f|
−1,q
+ R|g|
−1,q
+ kgk
q
) ( if n = 2)
(VII.4.5 1)
and if 1 < q < n + 1
R
1/(n+1)
kvk
s
1
+ |v|
1,q
+ kpk
q
≤ c (R|f |
−1,q
+ R|g|
−1,q
+ kgk
q
) , s
1
=
(n + 1)q
n + 1 − q
Rkv
2
k
q
+ R
1/3
kvk
3q/(3−q)
+ |v|
1,q
+ kpk
q
≤ c (R|f |
−1,q
+ R|g|
−1,q
+ kgk
q
) ( if n = 2)
(VII.4.5 2)
with c = c(n, q).
Starting from (VII.4.51) and (VII.4.52), we may use a now standard den-
sity argument to extend the above results to the case when f and g merely
satisfy the assumptions
f ∈ D
−1,q
0
(R
n
), g ∈ L
q
(R
n
) ∩D
−1,q
0
(R
n
).
However, to do this we need a result that ensures us that we can approximate
g by functions from C
∞
0
(R
n
) in the norm of L
q
(R
n
) ∩D
−1,q
0
(R
n
). This is the
content of the following.
Lemma VII.4.3 Let
g ∈ L
q
(R
n
) ∩ D
−1,q
0
(R
n
), 1 < q < ∞.
Then, for any η > 0 there exists g
(η)
∈ C
∞
0
(R
n
) such that
|g
(η)
−g|
−1,q
+ kg
(η)
− gk
q
< η.
VII.4 Existence, Uniqueness, and L
q
-Estimates in the Whole Space 457
Proof. Consider the problem
∆Ψ = g in R
n
. (VII. 4.53)
In view of the assumption made on g, by Exercise II.11.9 there exist two (a
priori distinct) solutions Ψ
1
and Ψ
2
to (VII.4.53) such that
|Ψ
1
|
2,q
≤ ckgk
q
|Ψ
2
|
1,q
≤ c|g|
−1,q
.
(VII.4.5 4)
It is not difficult to show that
D
2
(Ψ
1
− Ψ
2
) ≡ 0. (VII.4.55)
In fact, the difference Ψ = Ψ
1
−Ψ
2
satisfies
∆Ψ = 0 in R
n
. (VII. 4.56)
We may now represent Ψ by means of (V.3.14). Taking into account (VII.4.56)
and that H
(R)
is of bounded support, this latter leads, in particular, to the
following
D
i
D
j
Ψ(x) = −
Z
R
n
H
(R)
(x − y)D
i
D
j
Ψ(y)dy . (VII.4.57)
From (VII. 4.57) we infer
D
i
D
j
Ψ(x) = −
Z
R
n
H
(R)
(x − y)D
i
D
j
Ψ
1
(y)dy
+
Z
R
n
D
i
H
(R)
(x − y)D
j
Ψ
2
(y)dy,
and so, by the H¨older inequality, we obta in
|D
i
D
j
Ψ(x)| ≤ kH
(R)
k
q
0
|Ψ
1
|
2,q
+ |H
(R)
|
1,q
0
|Ψ
2
|
1,q
.
Letting R → ∞ into this relation and using (VII.4.54) and (V.3.13) proves
(VII.4.5 5). We may then state that problem (VII.4.53) with g verifying the
assumptions of the lemma admits a unique solution Ψ ∈ D
1,q
0
(R
n
) ∩D
2,q
(R
n
)
and that this solution satisfies
|Ψ|
2,q
≤ ckgk
q
|Ψ|
1,q
≤ c|g|
−1,q
.
(VII.4.5 8)
Given ρ > 0, let us denote by ζ
ρ
= ζ
ρ
(x) a nonincreasing, smooth function
that equals 1 for |x| ≤ ρ and 0 for |x| ≥ 2ρ and
|∇ζ
ρ
| ≤ c/ρ. (VII.4.5 9)

458 VII Steady Oseen Fl ow in Exterior Domains
Set
u = ∇Ψ, u
ρ,ε
= ζ
ρ
∇Ψ
ε
, g
ρ,ε
= ∇ · u
ρ,ε
, (VII.4.60 )
where Ψ
ε
is the regularizer of Ψ. Evi dently
g
ρ,ε
∈ C
∞
0
(R
n
).
Let us next show that g
ρ,ε
approaches g simultaneously in L
q
(R
n
) and
D
−1,q
0
(R
n
) as ρ → ∞ and ε → 0. By (VII.4.53), (VII.4.59), and (VII.4.60), by
the Minkowski inequality, property (II.2.9)
2
of the regularizer and by Exercise
II.3.2 we find
kg
ρ,ε
−gk
q
= k∇· u
ρ,ε
−∇ · uk
q
≤k∇ζ
ρ
· ∇Ψ
ε
k
q
+ kζ
ρ
g
ε
−gk
q
≤
C
ρ
|g|
−1,q
+ k(ζ
ρ
− 1)gk
q
+ kg
ε
− gk
q
.
This inequality, along with (I I.2.9)
2
, establishes that for all η > 0 we m ay find
ρ
1
= ρ
1
(η, g) > 0 and ε
1
= ε
1
(η, g) > 0 such that
kg
ρ,ε
−gk
q
< η/2, for all ρ > ρ
1
, ε > ε
1
. (VII.4.6 1)
Furthermore, from (VII.4.53), (VI I .4.60) and the Minkowski inequali ty, we
have
|g
ρ,ε
−g|
−1,q
≤ ku
ρ,ε
− uk
q
≤ |Ψ
ε
− Ψ|
1,q
+ k(ζ
ρ
− 1)∇Ψk
q
and so, again by Exercise II.3.2 and (VII.4.58)
2
, it follows that for al l η > 0
we may find ρ
2
= ρ
2
(η, g) > 0 and ε
2
= ε
2
(η, g) > 0 such that
|g
ρ,ε
−g|
−1,q
< η/2, for all ρ > ρ
2
, ε > ε
2
. (VII.4.62)
The lemma then fol lows from (VII.4.61 ) and (VII.4.62). ut
We shall finally show tha t the q-generalized solutions just obtained are in
fact unique among their class of existence.
2
Actually, supp ose w, τ is another
solution corresponding to the same data, with
w ∈ D
1,q
0
(R
n
), τ ∈ L
q
loc
(R
n
).
3
The differences
z = w −v ∈ D
1,q
0
(R
n
), s = τ −p ∈ L
q
loc
(R
n
)
satisfy the identity
2
We observe that uniqueness is an immediate consequence of Theorem VII.6.2,
which wi ll be proved in Section VII.6. Here, we prefer to give another proof,
which makes all treatment self-contained.
3
This assumption on τ is a consequence of t hat made on w, see Lemma VII.1.1.
Notice that we need no global summability assumption on τ.

VII.5 Existence of Plane Flow in Exterior Domains 459
(∇z, ∇ψ) − R(
∂z
∂x
1
, ψ) = (s, ∇ ·ψ) (VII.4.63)
for all ψ ∈ C
∞
0
(R
n
). From Theorem VI I.1.1 we deduce z, s ∈ C
∞
(R
n
) and
so, in particular, using (VII.4.63), we find
∆z + R
∂z
∂x
1
− ∇s = 0
∇ · z = 0
in R
n
z ∈ D
1,q
0
(R
n
).
(VII.4.6 4)
Using Theorem V.5.3 (with f ≡ −R(∂z/∂x
1
)) it follows that
z ∈ D
2,q
(R
n
), s ∈ D
1,q
(R
n
).
Operating with ∇· o n both sides of (VII.4.64)
1
we find that s is harmonic
throughout R
n
and, therefore, by Exercise II.11.11 we deduce ∇ s ≡ 0 in
R
n
. Relation (VII. 4.64) then furnishes that each component z of z satisfies
(VII.4.3 8) and si nce z ∈ D
1,q
0
(R
n
) we may use Exercise VII.4.1 to deduce
z = 0 in D
1,q
0
(R
n
) and uniqueness is proved.
The results just shown are summarized in the foll owing.
Theorem VII.4.2 Given
f ∈ D
−1,q
0
(R
n
), g ∈ L
q
(R
n
) ∩ D
−1,q
0
(R
n
), 1 < q < ∞,
there exists at least one q-generalized solution v to (VII.4.1). Moreover, the
pressure field p associ ated to v satisfies
p ∈ L
q
(R
n
)
and v, p verify the estimate (VII.4.51). Also, if 1 < q < n + 1, then
v ∈ L
s
1
(R
n
), s
1
=
(n + 1)q
n + 1 − q
,
with
v
2
∈ L
q
(R
2
), if n = 2,
and estimate (VII. 4.52) holds. Finally, if w is another q-generali zed solution to
(VII.4.1 ) corresponding to the same data f and g, then w ≡ v +c
1
, τ ≡ p+c
2
,
for some constants c
i
, i = 1, 2, where τ is the pressure field associated to w
by Lemma VII.1.1. If q < n + 1, we may take c
1
= 0.
VII.5 Existence of Generalized Soluti ons for Plane Flows
in Exterior Domains
In the previous section we proved, among other things, existence of pla ne flow
in the whole space tending to a prescrib ed value at infinity. In this section
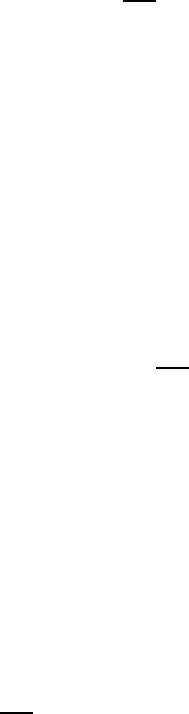
460 VII Steady Oseen Fl ow in Exterior Domains
we shall establish the same result in the more general case when the relevant
region of flow is an exterior domain. This is in sharp contrast with the Stokes
approximation, where we know that the problem is resolvable if and only if
certain restrictions are imposed on the data, see Section V. 7. However, as we
already observed in Remark VII.2.1, to show existence we cannot use sic et
simpliciter the technique of Theorem VII.1.1, for then we would not be able
to control the behavior of the solution at large distances. Consequently, we
have to employ a different approach.
To this end, following Finn & Smith (19 67a), we begin to consider the
modified problem
∆v + R
∂v
∂x
1
−εv = ∇p + Rf
∇ · v = 0
in Ω
v = v
∗
at ∂Ω
lim
|x|→∞
v(x) = 0
(VII.5.1 )
with ε ∈ (0, 1] and prove for it existence of solutions and suitable L
q
-estimates
for any value of ε. Successively, using such estimates, we show that in the limit
ε → 0 these solutions converge in a well-defined sense to a generalized solution
of the original problem (VII.0.2), (VII.0.3).
The above assertions will be proved through several steps. First of all we
consider existence of a solution to the following nonhomogeneous approximat-
ing system in R
2
:
∆u + R
∂u
∂x
1
−εu = ∇π + RF
∇ · u = g
(VII.5.2 )
Specifically, we have
Lemma VII.5.1 Let F ∈ L
q
(R
2
), g ∈ W
1,q
(R
2
), 1 < q < 3/2. Then for all
ε ∈ (0, 1] there exists a solution u
ε
, π
ε
to (VII .5.2) such that
u
ε
∈ W
2,q
(R
2
) ∩ D
1,3q/(3−q)
0
(R
2
) ∩ L
3q/(3−2q)
(R
2
)
π
ε
∈ D
1,q
(R
2
)
u
ε
2
∈ L
2q/(2−q)
(R
2
) ∩ D
1,q
0
(R
2
)
∂u
1
∂x
1
∈ L
q
(R
2
)
and satisfying the estimate

VII.5 Existence of Plane Flow in Exterior Domains 461
Rku
ε
2
k
2q/(2−q)
+R|u
ε
2
|
1,q
+ R
2/3
ku
ε
k
3q/(3−2q)
+ R
1/3
|u
ε
|
1,3q/(3−q)
+R
∂u
ε
1
∂x
1
q
+ |u
ε
|
1,q
+ |π
ε
|
1,q
≤ c (R|f|
q
+ |g|
1,q
+ Rkgk
q
) ,
(VII.5.3 )
where c = c(q). Moreover, if w, τ are such that
(a) w ∈ W
1,2
(R
2
), τ ∈ L
loc
(R
2
);
(b) (∇w, ∇ψ) −R(
∂w
∂x
1
, ψ) + ε(w, ψ) = (τ, ∇ · ψ) − R[F , ψ],
(w, ∇· φ) = −(g, φ), for all ψ, φ ∈ C
∞
0
(R
2
),
necessarily w = u
ε
and τ = π
ε
+ const a.e. in R
2
.
Proof. The proof of the existence of u
ε
, π
ε
satisfying (VII.5.3) is entirely anal-
ogous to that of Theorem VII. 4.1. The only point that deserves a littl e care is
to show tha t the constant c in (VII.5.3) can be taken independent of ε. To see
how this can be done, we shall sketch the proof of (VII.5.3) when F ∈ C
∞
0
(R
2
)
and g ≡ 0, leaving to the reader the simple tasks of giving details and extend-
ing the result to the general case. As in Section VII.4, we first ma ke a change
of variable of the type (VII. 4.3), which formally brings (VII.5.2) into a similar
system having R = 1 and εR in place of ε. L et us denote by (VII. 5.2
0
) this
latter system. We next construct a solution to (VII.5.2
0
) by Fourier transform,
that is,
u
ε
(x) =
1
2π
Z
R
2
e
ix·ξ
U
ε
(ξ)dξ
π
ε
(x) =
1
2π
Z
R
2
e
ix·ξ
Π
ε
(ξ)dξ,
where
U
ε
m
(ξ) =
ξ
m
ξ
k
−ξ
2
δ
mk
ξ
2
(ξ
2
+ εR + iξ
1
)
b
F
k
(ξ), m = 1, 2
Π
ε
(ξ) = i
b
F
k
(ξ)ξ
k
ξ
2
,
and
b
F is the Fourier transform of F . Setting
Ψ
mk
(ξ) =
ξ
m
ξ
k
− ξ
2
δ
mk
ξ
2
(ξ
2
+ εR + iξ
1
)
, (VII.5.4)
using the same reasonings as in the proo f of Lemma VII.4.2 one shows with
no difficulty that the functions
Ψ
mk
(ξ), ξ
`
Ψ
mk
(ξ), ξ
`
ξ
s
Ψ
mk
(ξ), `, s, m, k = 1, 2,
satisfy the assumptio n of Lemma VII.4.1 with β = 2/3, β = 1/3 and β = 0,
respectively, with a constant M independent of εR. The same is true for
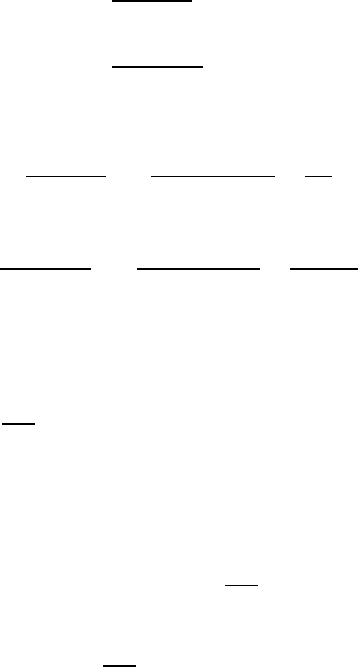
462 VII Steady Oseen Fl ow in Exterior Domains
Ψ
2k
(ξ), with β = 1/2 , and for ξ
`
Ψ
2k
(ξ), with β = 0, k = 1, 2. As a consequence,
arguing exactly as in the proof of Theorem VII.4.1, we obtain (VII.5.3) with
a constant c independent of ε. Let us next show
u
ε
∈ W
1,q
(R
2
). (VII.5.5)
By Lemma VII.4.1, it suffices to prove, for some C = C(ε, R) and all `, m, k =
1, 2
|ξ
κ
1
1
| |ξ
κ
2
2
|
∂
κ
Ψ
mk
∂ξ
κ
1
1
∂ξ
κ
2
2
≤ C
|ξ
κ
1
1
| |ξ
κ
2
2
|
∂
κ
(ξ
`
Ψ
mk
)
∂ξ
κ
1
1
∂ξ
κ
2
2
≤ C
with κ
1
, κ
2
= 0, 1 and κ
1
+ κ
2
= κ. We now have, wi th c = c(κ
1
, κ
2
),
|ξ
κ
1
1
| |ξ
κ
2
2
|
∂
κ
Ψ
mk
∂ξ
κ
1
1
∂ξ
κ
2
2
≤ c
1
ξ
2
+ εR + |ξ
1
|
≤
c
εR
and
|ξ
κ
1
1
| |ξ
κ
2
2
|
∂
κ
(ξ
`
Ψ
mk
)
∂ξ
κ
1
1
∂ξ
κ
2
2
≤ c
|ξ|
ξ
2
+ εR + |ξ
1
|
≤
c
(εR)
1/2
and (VII.5 .5) follows. Finally, let w, τ satisfy conditio ns (a) and (b) stated in
the lemma and set v = w −u
ε
, p = τ −π
ε
. We then obtain that v obeys the
identity
(∇v, ∇ϕ) −R(v,
∂ϕ
∂x
1
) + ε(v, ϕ) = 0, for all ϕ ∈ D(Ω). (VII. 5.6)
However, by embedding Theorem II.3.2 , it follows that u
ε
∈ W
1,2
(R
2
) and
since ∇ · v = 0 we conclude v ∈ H
1
(R
2
). By continuity, we may then extend
identity (VII.5.6) to all ϕ ∈ H
1
(R
2
) and, in particular, we may take ϕ = v
to deduce
(∇v, ∇v) + ε(v, v) = R(v,
∂v
∂x
1
). (VII.5.7)
Since
(v,
∂v
∂x
1
) = 0,
(VII.5.7 ) yields v = 0 a.e. in R
2
and, consequently, from (b) and the a nalog ous
identity written for u
ε
, we have
(p, ∇ · ψ) = 0, for all ψ ∈ C
∞
0
(R
2
),
implying τ = π
ε
+ const a.e. in R
2
. The pro of of the lemma is therefore
complete. ut
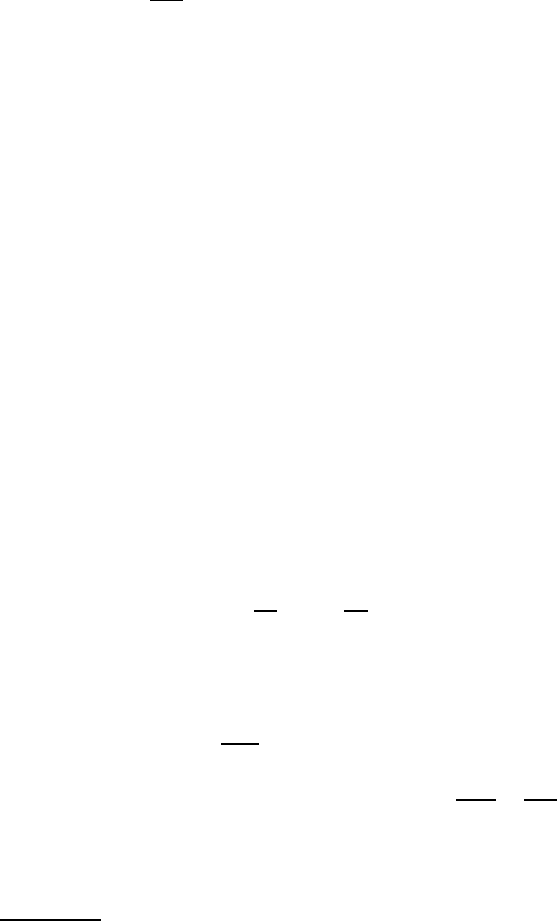
VII.5 Existence of Plane Flow in Exterior Domains 463
The second step consists in proving the existence of a generalized solution
to (VII.5.1), that is, of a field v : Ω → R
2
satisfying the requirements (i)-(iv)
of Definition VII.1.1 with q = 2 , along with the identity
(∇v, ∇ϕ) − R(
∂v
∂x
1
, ϕ) + ε(v, ϕ) = −R[f , ϕ], for all ϕ ∈ D(Ω). (VII.5.8)
We have
Lemma VII.5.2 Let Ω be a locally Lipschitz, exterior domain of R
2
, and l et
f ∈ D
−1,2
0
(Ω), v
∗
∈ W
1/2,2
(∂Ω).
Then, for all ε ∈ (0, 1] there exists a generalized solution v
ε
to (VII.5.1). This
solution verifies the estimate
εkv
ε
k
2
+ kv
ε
k
2,Ω
R
+ |v
ε
|
1,2
≤ c
1
R|f|
1,2
+ (1 + R)kv
∗
k
1/2,2(∂Ω)
(VII.5.9 )
for all R > δ(Ω
c
) and with a constant c
1
= c
1
(R, Ω). Moreover, denoting by
p
ε
∈ L
2
loc
(Ω) the corresponding pressure field,
1
we have
kp
ε
k
2,Ω
R
/ R
≤ c
2
{R|f|
−1,2
+ (1 + R)|v
ε
|
1,2
} (VII.5.10)
for a ll R > δ(Ω
c
) and with a constant c
2
= c
2
(R, Ω).
Proof. Again, the proof is compl etely analogous to that given in Theorem
VII.2.1. Actually, we look for a solution v
ε
of the form
v
ε
= w
ε
+ V
1
+ σ,
where V
1
is a suitable extension of v
∗
constructed exactly as in the proof of
Theorem VII.2.1 . In addition,
σ = −
1
4π
∇
log
1
|x|
Z
∂Ω
v
∗
· n
(the origin of coo rdinates having been taken in
˙
Ω
c
) and w
ε
∈ H
1
(Ω) verifies
the identity
(∇w
ε
, ∇ϕ) −R(
∂w
ε
∂x
1
, ϕ) + ε(w
ε
, ϕ)
= −R[f , ϕ] − (∇V
1
, ∇ϕ) − R(
∂V
1
∂x
1
+
∂σ
∂x
1
, ϕ),
for all ϕ ∈ D(Ω). Using the Galerkin method and exploiting the properties
of V
1
, σ, a nd a , we easily show the existence of w
ε
obeying the previous
identity and the following inequality
1
Associated to v
ε
in a way completely analogous to that used in Lemma VII.1.1
for generalized solutions with ε = 0.
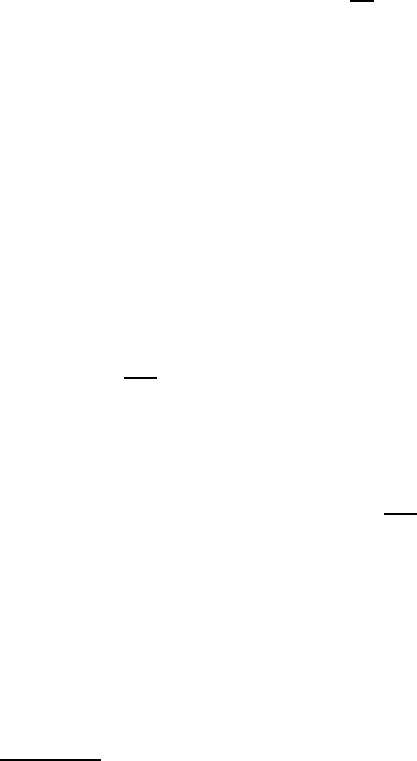
464 VII Steady Oseen Fl ow in Exterior Domains
εkw
ε
k
2
+ |w
ε
|
1,2
≤ c
3
R|f|
−1,2
+ (1 + R)kv
∗
k
1/2,2(∂Ω)
(see the proof of Theorem VI I.2.1). From this we deduce the existence of a
generalized solution v
ε
satisfying (VII.5.9). The estimate for p
ε
is obtained
again as in the proof of Theorem VII.2.1. No tice that this time, si nce ε 6= 0,
we are abl e to control the behavior of v
ε
at infinity and to show, in particular,
the validity of condition (iv) of Definition VII.1. 1. Actually, for ε > 0, we have
v
ε
∈ W
1,2
(Ω) and so, putting |x| = r and
I(r) =
Z
2π
0
|v
ε
(r, θ)|
2
dθ, r > δ(Ω
c
),
we recover
I(r) ∈ L
1
(0, ∞),
dI
dr
∈ L
1
(0, ∞),
which implies I(r) = o(1) as r → ∞. ut
The third step is to prove that if, in addition to the assumptions made in
Lemma VII.5.2, f belongs to L
q
(Ω) for some q ∈ (1, 3/2), then v
ε
and its
derivatives belong to suitable Lebesgue spaces and satisfy there an estimate
in terms of the data uniformly in ε ∈ (0, 1]. Specifically, we have
Lemma VII.5.3 Let Ω, f , and v
∗
satisfy the hypotheses of Lemma VII.5.2.
Suppose, further, f ∈ L
q
(Ω), 1 < q < 3/2. Then, the generalized solution v
ε
determined in Lemma VII.5.2 and the corresponding pressure field p
ε
verify,
in addition, for all R > δ(Ω
c
)
v
ε
∈ D
2,q
(Ω
R
) ∩ D
1,3q/(3−q)
(Ω
R
) ∩ L
3q/(3−2q)
(Ω)
v
ε
2
∈ L
2q/(2−q)
(Ω) ∩ D
1,q
(Ω)
∂v
ε
1
∂x
1
∈ L
q
(Ω)
p
ε
∈ D
1,q
(Ω
R
)
along with the estimate
R
kv
ε
2
k
2q/(2−q)
+ |v
ε
2
|
1,q
+
∂v
ε
1
∂x
1
q
!
+bkv
ε
k
3q/(3−2q)
+ R
1/3
|v
ε
|
1,3q/(3−q),Ω
R + |v
ε
|
2,q ,Ω
R + |p
ε
|
1,q,Ω
R
≤ c
R(kfk
q
+ (1 + R)|f |
−1,2
) + (1 + R)
2
kv
∗
k
1/2,2(∂Ω)
,
(VII.5.1 1)
where b = min{1, R
2/3
} and c = c(q, Ω, R).
Proof. Let χ ∈ C
∞
(R
2
) be zero in B
R/2
and one in B
c
R
, for some arbitrarily
fixed R > δ(Ω
c
). Setting u
ε
= χv
ε
, π
ε
= χp
ε
it is easy to show that u
ε
, π
ε
satisfy (VII.5.2)
2
with
2
In the generalized sense.

VII.5 Existence of Plane Flow in Exterior Domains 465
RF = ∆χv
ε
+ 2∇χ · ∇v
ε
+ Rv
∂χ
∂x
1
+ p
ε
∇χ + Rχf
g = ∇χ · v
ε
.
By the properties of χ, we readily deduce the estimates
RkF k
q
≤c
1
[Rkfk
q
+ (1 + R)kv
ε
k
1,2,Ω
R
+ kp
ε
k
2,Ω
R
]
kgk
q
≤c
2
kv
ε
k
2,Ω
R
|g|
1,q
≤c
3
kv
ε
k
1,2,Ω
R
(VII.5.1 2)
with c
i
= c
i
(χ), i = 1, 2. Using these inequalities along with Lemma VII.5.2
we deduce tha t
F ∈ L
q
(R
2
), g ∈ W
1,q
(R
2
), 1 < q < 3/2
and that
RkF k
q
+ |g|
1,q
+ Rkgk
q
is increased through the right-hand side of (VII.5.11). From Lemma VI I.5.1
we then deduce
u
ε
∈ W
2,q
(R
2
) ∩ D
1,3q/(3−q)
0
(R
2
) ∩L
3q/(3−2q)
(R
2
)
π
ε
∈ D
1,q
(R
2
)
u
ε
2
∈ L
2q/(2−q)
(R
2
) ∩D
1,q
0
(R
2
)
∂u
ε
1
∂x
1
∈ L
q
(R
2
)
and that u
ε
, π
ε
satisfy (VII.5.3). The pro of then follows from (VII.5.9) and
(VII.5.1 2), and by recalling that χ = 1 in B
c
R
and that
kv
ε
2
k
2q/(2−q),Ω
R
+ |v
ε
2
|
1,q,Ω
R
+
∂v
ε
1
∂x
1
q,Ω
R
+ kv
ε
k
3q/(3−2q),Ω
R
≤ ckv
ε
k
1,2,Ω
R
.
ut
We are now in a position to prove the following.
Theorem VII.5.1 Let Ω be a two-dimensional, locally Lipschitz exterior
domain. Then, given
f ∈ D
−1,2
0
(Ω) ∩ L
q
(Ω), 1 < q < 3/2,
v
∗
∈ W
1/2,2
(∂Ω) ,
there exists a unique generalized solution v to (VII.0.2) and (VII.0.3). More-
over, for all R > δ(Ω
c
) this solution verifies
