Galaktionov V.A., Svirshchevskii S.R. Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and Physics
Подождите немного. Документ загружается.


5 Quasilinear Wave and Boussinesq Models. Systems 273
This system, which was introduced by Manin and Radul in 1985 [410] (who also proposed
a supersymmetric extension to the whole KP hierarchy), is integrable and, for ξ = 0, yields
the KdV equation. Earlier Kupershmidt’s super KdV-version [361] is not invariant under a
space supersymmetric transformation. For several supersymmetric systems, the Baker–Hirota
bilinear method applies and soliton solutions can be constructed; see references and results in
[101, 251], though existence of higher-order N-solitons is not guaranteed. For systems of two
semilinear equations with rather general cubic nonlinearities, the system
u
t
= u
xx
− 2u
2
v + 2ku,
v
t
=−v
xx
+ 2uv
2
− 2kv,
(5.114)
where k is a constant, is the only one admitting infinite-dimensional prolongation Lie algebras
[7]. See [512] and references therein for a complete classification of two-component homo-
geneous polynomial symmetry-integrable systems (i.e., admitting a generalized symmetry of
infinitely many orders). System (5.114), also emerging in the gauge gravity formulation, ad-
mits a Lax pair and B¨acklund transformation, and affords soliton-like solutions that are called
dissipatons; see references in [7]. Note also the Thirring equations, [551] representing the
most famous solvable field theory model,
−iu
x
+ 2v + 2|v|
2
u = 0,
−iv
t
+ 2u + 2|u|
2
v = 0,
possess exact solitons constructed by using Baker’s hyperelliptic functions generated by even
hyperelliptic curves (similarto the KP equation; recall that the KdV one needs odd curves, [22,
415]); see results and earlier references in [166]. Baker’s hyperelliptic functions associated
with algebraic curves of genus two were used in [126] to construct traveling wave periodic
and quasi-periodic solutions of two coupled nonlinear Schr¨odinger equations
iu
t
+ u
xx
+ (κuu
∗
+ χvv
∗
)u = 0,
iv
t
+v
xx
+ (χuu
∗
+ ρvv
∗
)v = 0,
in the case where κ = χ = ρ, corresponding to the integrable Manakov system [409].
A large number of parabolic systems admitting second and third-order differential con-
straints were introduced in [324], where some of the examples deal with linear invariant sub-
spaces. A classification of first-order differential constraints and substitutions was performed
in an earlier paper by Kaptsov [318]. For systems, determining exact solutions via known dif-
ferential constraints is often a difficult problem. Consider [318] the reaction-diffusion system
u
t
= u
xx
+ µ(u − v) + ν(u − v)e
u+v
− e
2(u+v)
,
v
t
= d v
xx
+ ν(u − v)e
u+v
− µ(u − v) − 2de
2(u+v)
,
(5.115)
where d is constant (d = Le, the Lewis number in reaction-diffusion theory, [383]). The
functions µ(u −v) and ν(u − v) are arbitrary. The system is compatible with two differential
constraints u
x
= v
x
= e
u+v
, which on integration imply the following solutions:
u(x, t) =
1
2
[C
1
(t) − ln(2x + C
2
(t))],v(x, t) =
1
2
[−C
1
(t) − ln(2x + C
2
(t))],
so u − v = C
1
(t), as the structure of the system suggests. Plugging into (5.115) yields the
DS C
1
= 2µ(C
1
), C
2
=−2ν(C
1
). One may observe a direct similarity of nonlinearities in
(5.115) and that of the hyperbolic Tzitz´eica equation (sometimes also called the Mikhailov–
Dodd–Boullough equation) u
tt
− u
xx
= e
u
+ e
2u
, or of the corresponding elliptic nonlinear
Poisson equation, u
tt
+u
xx
= e
u
+e
2u
, describing vortical structure in inviscid fluid, where u
is the stream function; see [29]. Both PDEs admit Lie–B¨acklund symmetries [10, p. 205] and
possess extra solutions on 1D subspaces; see the generalized separation of variables (GSV)
© 2007 by Taylor & Francis Group, LLC

274 Exact Solutions and Invariant Subspaces
method in [316] and [13, Ch. 5]. Application of the GSV to the corresponding parabolic PDE
u
t
= u
xx
+e
u
−e
2u
gives a simple traveling wave solution u(x, t) =−
1
2
ln[
√
2(x −
√
2t)].
A classical example of quadratic systems is the completely anisotropic XY Z Landau–
Lifshitz (LL) equations [369], [371, p. 417]
S
t
= S × S
xx
+ S × JS, (5.116)
where × denotes the vector product in IR
3
, S = (S
1
, S
2
, S
3
)
T
(x, t ) is a vector-function, and
J = diag {j
1
, j
2
, j
3
} is a constant diagonal 3×3 matrix. The scalar form of (5.116) contains
quadratic operators,
S
1t
= S
2
S
3xx
− S
3
S
2xx
+ ( j
3
− j
2
)S
2
S
3
,
S
2t
= S
3
S
1xx
− S
1
S
3xx
+ ( j
1
− j
3
)S
1
S
3
,
S
3t
= S
1
S
2xx
− S
2
S
1xx
+ ( j
2
− j
1
)S
1
S
2
.
This model was derived for magnetic crystals as a modification of the Hamiltonian Heisenberg
spin equation S
t
= S ×S
ss
, S
2
= 1(t is time, s is the arc length) from solid state physics. The
LL equations describe perturbations propagating in a direction orthogonal to the anisotropy
axis in a ferromagnet (the anisotropy parameters satisfy j
1
< j
2
< j
3
), and has since been
identified as a soliton-bearing system possessing 1, 2, and N-soliton solutions constructed by
the Baker–Hirota method; see references in [539, 507] and [35, p. 218] for finite-gap peri-
odic solutions. Existence, uniqueness, and regularity results (solutions are smooth, except, at
most, finitely many points) for equations such as (5.116) have been studied in a number of
papers; see [106] and [105], where the Maximum Principle and comparison aspects for the LL
equations are pointed out. Some exceptional symmetries exist for the radially symmetric LL
equations for the N -dimensional magnetic spin system with an external magnetic field
Z
t
= Z ×
N
Z + Z × H,
where
N
denotes the radial Laplacian in IR
N
,andH = (0, 0, h)
T
is a constant vector. For
instance, there exist exact solutions
Z = c(r)(k
2
cosk sinm, k
2
sink sinm, k
1
cosm)
T
,
where k
1,2
are constants, c(r) is an arbitrary function, and k = k(r, t), m = m(r, t ) satisfy
sinm
c
k
t
=
N
m +
2c
c
m
r
− sinm cosm (k
r
)
2
−h
sinm
c
,
sinm
c
m
t
=−
N
k −
2c
c
k
r
−
2cosm
sinm
m
r
k
r
.
(5.117)
This system generates a number of explicit solutions, including those with blow-up, [398]. In
the case where N = 2andh = 0, there exist blow-up similarity solutions [269]
Z(r, t) =
1
√
T −t
f (y), y =
x
(T −t)
1/4
, f (y) =−
1
y
2
(
1
4
, cos
y
4
4
, sin
y
4
4
)
T
(similar solutions can be derived from system (5.117)), and the following global non-scaling
invariant solutions:
Z(r, t) =
1
Cϕ
C
(t)
(cos
r
2
4ϕ
C
(t)
, sin
r
2
4ϕ
C
(t)
, Ct)
T
, with ϕ
C
(t) =
1
C
2
+ t
2
(C =∞yields similarity solutions), [270]. Some of these solutions can be associated with
invariant subspaces or sets.
Open problems
• In particular, these appeared in Examples 5.1, 5.5, 5.7, 5.9, 5.14, 5.16, Sections
5.3.3, 5.3.4, and in Remark 5.35.
© 2007 by Taylor & Francis Group, LLC

CHAPTER 6
Applications to Nonlinear Partial Differential
Equations in IR
N
We present invariant subspace methods and results that are applied to nonlinear second and
higher-order differential operators in
IR
N
. The analysis of invariant conditions is now associ-
ated with systems of nonlinear elliptic PDEs, and results of the generality and completeness
achieved earlier for
N = 1
in the three previous chapters are difficult to obtain. Nevertheless,
we give a number of examples of invariant subspaces in radial and non-radial geometry in
IR
N
, especially when dealing with polynomial and trigonometric functions.
6.1 Second-order operators and some higher-order extensions
6.1.1 Basic polynomial subspaces
As in 1D geometry, we begin with a class of quadratic second-order operators admit-
ting polynomial subspaces. Consider a family of operators given by
F[u] = α(u)
2
+ βuu + γ |∇u|
2
in IR
N
, (6.1)
where, as usual, α, β,andγ are arbitrary parameters. Here x = (x
1
, x
2
, ..., x
N
)
T
is
a vector in IR
N
. Obviously, F preserves the subspace of linear functions
W
lin
N
= L{x
1
, ..., x
N
}. (6.2)
This defines a simple map F : W
lin
N
→ L{1}. The next observation is also easy.
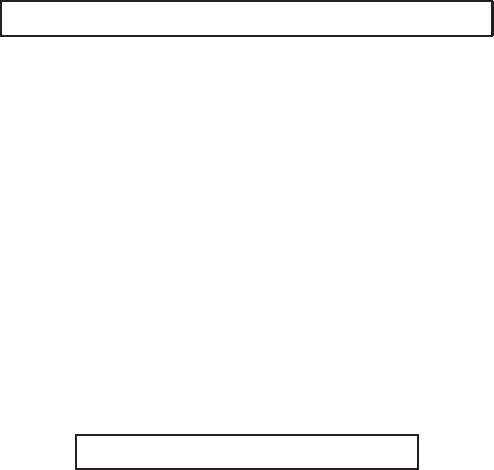
Proposition 6.1
Operator
(6.1)
preserves:
(i)
the 2D subspace of radial functions
W
r
2
= L{1, |x |
2
}; (6.3)
(ii)
the
(N+1)
-dimensional subspace of diagonal quadratic forms
W
q
N+1
= L
1, x
2
1
, ..., x
2
N
; (6.4)
(iii)
the subspace of arbitrary quadratic forms
W
q
n
= L{1, x
i
x
j
, 1 ≤ i, j ≤ N}
n = 1 +
N(N+1)
2
;
and
(6.5)
(vi)
the direct sum of subspaces
(6.2)
and
(6.5)
,
W
q
n
⊕ W
lin
N
. (6.6)
Let us perform basic computations for the general case (6.6).
© 2007 by Taylor & Francis Group, LLC
276 Exact Solutions and Invariant Subspaces
Example 6.2 Consider the quasilinear PDE with the operator (6.1),
u
tt
= F[u] ≡ α(u)
2
+ βuu + γ |∇u|
2
in IR
N
× IR
+
, (6.7)
which is formally hyperbolic in the domain {αu +βu > 0}. Using subspace (6.6),
let us look for solutions
u(x , t) = C(t) + D(t)x + x
T
A(t)x , (6.8)
where C(t) is a function, D
T
(t) = (d
1
(t), ..., d
N
(t))
T
∈ IR
N
,andA(t) =#a
i, j
(t)#
is an N × N symmetric matrix with elements a
i, j
(t) = a
j,i
(t) for all i, j. Then,
u = 2
(i)
a
i,i
≡ 2(tr A),
and substituting (6.8) into (6.7), after some manipulations, we obtain the DS
C
= 4α(trA)
2
+ 2β(tr A) C + γ |D|
2
,
D
= 2β(tr A) D + 4γ DA,
A
= 2β(tr A) A + 4γ A
2
.
(6.9)
In general, (6.9) is a difficult DS even in lower-dimensional cases N = 3or2;see
references and related results in Remarks.
Example 6.3 (Quadratic wave equation)ThePDE
u
tt
=∇·(u∇u) + a + bu in IR
2
× IR
is hyperbolic in {u > 0} and admits solutions
u(x , t) = C(t) + a
1,1
(t)x
2
1
+ 2a
1,2
(t)x
1
x
2
+ a
2,2
(t)x
2
2
∈ W
q
4
. (6.10)
The corresponding DS is eighth-order,
C
= 2(a
1,1
+ a
2,2
)C + a + bC,
a
1,1
= 6a
2
1,1
+ 2a
1,1
a
2,2
+ 4a
2
1,2
+ ba
1,1
,
a
1,2
= 6(a
1,1
+ a
2,2
)a
1,2
+ ba
1,2
,
a
2,2
= 4a
2
1,2
+ 2a
1,1
a
2,2
+ 6a
2
2,2
+ ba
2,2
,
(6.11)
so the set of solutions is 8D. Setting a
1,2
(t) ≡ 0 givesa sixth-orderDS for {C, a
1,1
, a
2,2
}
and a 6D manifold of solutions
u(x , t) = C(t) + a
1,1
(t)x
2
1
+ a
2,2
(t)x
2
2
∈ W
q
3
= L{1, x
2
1
, x
2
2
}.
Therefore, W
q
4
contains new solutions, which cannot be obtained from those on W
q
3
despite the fact that the quadratic form in (6.10) is diagonalizable by an orthogonal
transformation in IR
2
. Clearly, this reflects the fact that, for hyperbolic(second-order
in t) PDEs, non-diagonal terms in solutions can occur evolutionarily, even if initial
data u(x, 0) was given by a diagonal quadratic form. For parabolic (first-order in t)
PDEs, initially diagonal forms remain diagonal for all times.
Example 6.4 (PME-type equations) Next, consider solutions on W
q
N+1
of a quasi-
linear parabolic equation. The pressure transformation u = v
σ
, σ = 0, in the PME
with extra reaction-absorption terms
v
t
=∇·(v
σ
∇v) + αv
1−σ
+ βv (v > 0)
© 2007 by Taylor & Francis Group, LLC
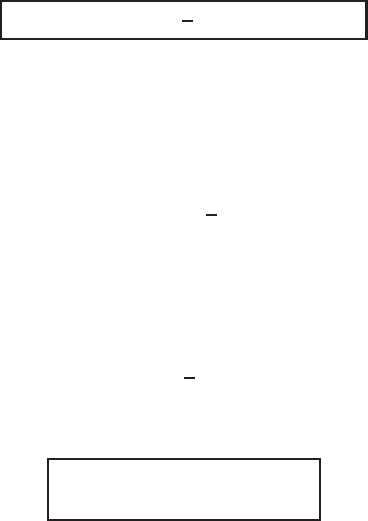
6 Applications to Nonlinear PDEs in IR
N
277
yields the PDE with quadratic operators
u
t
= F[u] ≡ uu +
1
σ
|∇u|
2
+ σα + σβu.
We use the subspace (6.4) and take
u(x , t) = C(t) +
(i)
a
i,i
(t) x
2
i
,
where a
i,i
(t) for i = 1, 2, ..., N are the only non-zero diagonal terms of the matrix
A(t). This gives the following (N+1)-dimensional DS:
C
= 2(tr A) C +σα + σβC,
a
i,i
= 2(tr A) a
i,i
+
4
σ
a
2
i,i
+ σβa
i,i
.
(6.12)
For equal coefficients a
1,1
= ... = a
N,N
= a, i.e., for radially symmetric solutions
u(r, t) = C(t) + a(t)r
2
(r =|x|)
on the subspace (6.3), the DS (6.12) reduces to a simple explicitly solvable system
C
= 2NaC + σα + σβC,
a
= 2(N +
2
σ
)a
2
+ σβa.
Example 6.5 (Ernst equation from general relativity) To illustrate application to
systems, consider the nonstationary version of the Ernst equation
f
t
= f f −|∇f |
2
+|∇g|
2
,
g
t
= f g − 2∇ f ·∇g.
(6.13)
For time-independentfunctions in IR
3
, setting E = f +ig yields the stationary Ernst
equation
(ReE)E =∇E ·∇E
that describe stationary axisymmetric space-times in general relativity, [171]. Using
the polynomial subspace (6.4) yields solutions of (6.13)
f (x, t) = C
0
(t) +
N
i=1
C
i
(t)x
2
i
, g(x, t) = D
0
(t) +
N
i=1
D
i
(t)x
2
i
,
where the expansion coefficients solve the DS
C
0
= 2
C
k
C
0
, C
i
= 2
C
k
C
i
+ 4
D
2
i
− C
2
i
,
D
0
= 2
D
k
C
0
, D
i
= 2
D
k
C
i
− 8C
i
D
i
.
In radial setting for solutions
f (x, t) = C
0
(t) + C(t)|x|
2
, g(x, t) = D
0
(t) + D(t)|x |
2
,
using |x|
2
= 2N , we obtain a simpler DS,
C
0
= 2NC
0
C, C
= 4D
2
+ 2(N −2)C
2
,
D
0
= 2NC
0
D, D
= 2(N − 4)CD.
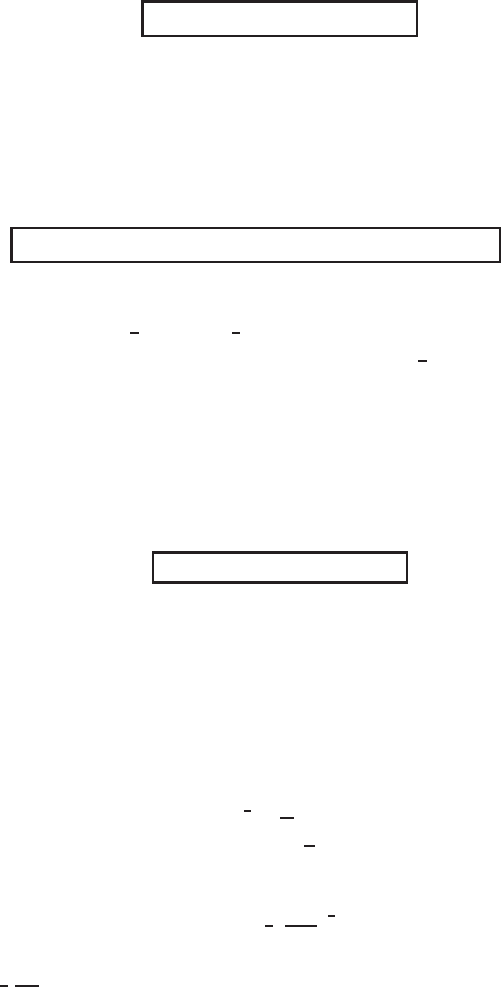
Example 6.6 These polynomial subspaces can be used for another class of second-
order parabolic equations. Consider the following homogeneous quadratic PDE:
vv
t
= αvv + β|∇v|
2
+ γv
2
in IR
N
× IR
+
,
© 2007 by Taylor & Francis Group, LLC
278 Exact Solutions and Invariant Subspaces
where, unlike many previous examples, the left-hand side is also quadratic. By the
exponential change v = e
u
, this PDE reduces to the standard form
u
t
= αu + (α +β)|∇u|
2
+ γ,
containing the quadratic Hamilton–Jacobi operator from the class (6.1). By Proposi-
tion 6.1, we can construct various solutions on the subspaces listed therein.
6.1.2 Two quadratic water wave models
Example 6.7 (Benney–Luke equation for water waves) The isotropic Benney–
Luke (BL) equation in IR
3
× IR
u
tt
− u + µ(a
2
u − bu
tt
) + ε[u
t
u + (|∇u|
2
)
t
] = 0, (6.14)
which describes the evolution of long water waves with small amplitude, was derived
in [40] as an approximation of the full water wave problem. The original model
includes ε = µ, a =
1
6
,andb =
1
2
in the absence of surface tension (in general,
a and b are positive constants satisfying a − b = σ −
1
3
,whereσ is the Bond
number proportional to the coefficient of surface tension). In appropriate limits, the
BL equation (6.14) can be reduced to the KdV and the KP equation; see details and
references in [458]. Both the quadratic and the linear operators in (6.14) preserve
the polynomial subspace (6.4) for N = 3 (or the more general one (6.5)), so the
restriction to W
q
4
yields an eighth-order DS (or higher-order for W
q
n
).
Let us point out another reduction of (6.14) describing interesting mathematical
features. In 1D, neglecting the linear terms gives the quadratic model
u
tt
+ ε(u
t
u
xx
+ 2u
x
u
xt
) = 0. (6.15)
In the domain {εu
t
< 0}, it is formally hyperbolic, for which an FBP can be posed,
exhibiting the free boundaries on the set {u
t
= 0}, where the PDE is degenerate. We
discuss the interface propagation by using the elementary exact solutions
u(x , t) = C
1
(t) + C
2
(t)x
2
,
C
1
=−2εC
2
C
1
,
C
2
=−10εC
2
C
2
.
Taking ε =−1, consider a particular explicit solution of the form
u(x , t) =−t
3
5
−
1
5t
x
2
for t > 0. (6.16)
It follows that u
t
= 0onthecurves(t) =
√
3t
4/5
, so that (6.16) is a solution of an
FBP in {x > s(t)} with the following free-boundary conditions:
u
t
= 0andu =
8
5
2
5u
xx
3
5
at x = s(t).
These are easily derived from (6.16) by differentiating, with the t-parameterization
t =−
2
5
1
u
xx
. Similar to FBPs for the TFEs in Example 3.10, one way to justify the
well-posedness of such FBPs is by using the von Mises transformation X = X (z, t),
© 2007 by Taylor & Francis Group, LLC

6 Applications to Nonlinear PDEs in IR
N
279
z = u
t
> 0, in a neighborhood of the interface. This is a hard OPEN PROBLEM for
a singular non-local-in-time PDE with a non-local Neumann-type condition at the
origin z = 0.
Example 6.8 (Boussinesq system) Consider the Boussinesq system that generates
the BL equation in Example 6.7,
I −
µ
2
η
t
=− − ε∇·(η∇) +
2µ
3
2
,
−
I −
µ
2
t
= η − µσ η +
ε
2
|∇|
2
,
(6.17)
where η is the surface elevation with the velocity potential on the free surface
ξ = φ
z=h
0
+η
= −
µ
2
+ O(µ
2
,µε);
see details in [458, pp. 481–483]. The system (6.17) is close to that derived by
Boussinesq in 1877 [76, p. 314]. It is not difficult to find the DS reduction of (6.17),
e.g., on the subspace (6.4). Both PDEs in (6.17) are degenerate at the interface sur-
face {∇ = 0}on which certain free-boundaryconditions should be posed. Solvabil-
ity analysis, even in 1D, becomes more involved than that for (6.15); cf. the FFCH
equation in Example 4.43.
6.1.3 Invariant subspaces driven by elliptic equations
Linear elliptic equations. Consider other types of subspaces governed by linear
elliptic PDEs for fully nonlinear second-orderoperators
F[u] = α(u)
2
+ βuu + εu
2
in IR
N
. (6.18)
Proposition 6.9
Operator
(6.18)
admits
W
2
= L{1, f (x )},
where
f
satisfies the
linear elliptic equation
f + µf = 0
in
IR
N
,
with
αµ
2
− βµ + ε = 0. (6.19)
Proof. Setting u = C
1
+ C
2
f ∈ W
2
yields
F[u] = εC
2
1
+ (2ε f +βf )C
1
C
2
+ F[ f ]C
2
2
,
where f =−µf ∈ W
2
and F[ f ] = (αµ
2
− βµ + ε) f
2
= 0 by (6.19).
Here, can be replaced by any homogeneoushigher-order operator,
L =
(|σ |≥1)
a
σ
(x )D
σ
(e.g., L =
m
for m ≥ 2).
Example 6.10 (Semilinear wave equation) Consider a fourth-ordersemilinear wave
equation with nonlinearities from the Boussinesq PDEs,
u
tt
= a
2
u + bu + βuu + εu
2
+ cu +d. (6.20)
It admits solutions
u(x , t) = C
1
(t) + C
2
(t) f (x), (6.21)
where f solves the linear elliptic PDE
βf +ε f = 0inIR
N
.
© 2007 by Taylor & Francis Group, LLC
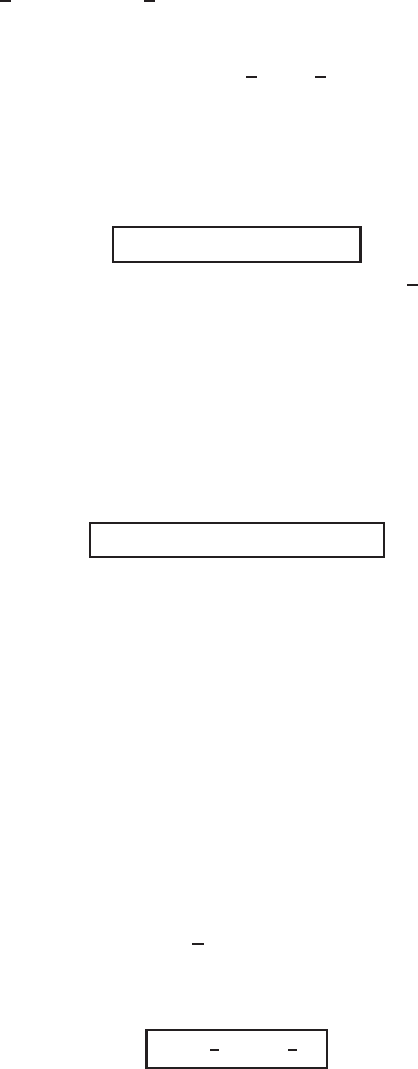
280 Exact Solutions and Invariant Subspaces
So f =−
ε
β
f and
2
f =
ε
β
2
f , and expansion coefficients satisfy the DS
C
1
= εC
2
1
+ cC
1
+ d,
C
2
= εC
1
C
2
+
a
ε
β
2
− b
ε
γ
+ c
C
2
.
Example 6.11 (Quasilinear heat equation) Consider the following quasilinear
parabolic equation with exponential nonlinearities:
v
t
= β∇·(e
v
∇v) + e
−v
(εe
2v
+ ce
v
+ d).
By the pressure-type change u = e
v
, it reduces to the quadratic PDE
u
t
= βuu + εu
2
+ cu +d.
There exist solutions (6.21), where f satisfies (6.19) with µ =
ε
β
,andtheDSis
C
1
= εC
2
1
+ cC
1
+ d,
C
2
= εC
1
C
2
+ cC
2
.
Example 6.12 (Fourth-order diffusion equation) Keeping the same exponential
nonlinearities, let us introduce a fourth-order parabolic PDE (we write principal op-
erators only)
v
t
= α∇·(e
v
∇v) + β∇·(e
v
∇v) + εe
v
+ ... ,
which on substitution u = e
v
yields
u
t
= αu
2
u + βuu + εu
2
+ ... .
For solutions (6.21), f solves (6.19).
Nonlinear elliptic systems. We consider families of quadratic evolution PDEs for
which it is convenient to deal with subspaces defined on the functions of the time-
variable t. This leads to systems of elliptic PDEs. Let us study two main cases, cor-
responding to parabolic and hyperbolic PDEs. The proofs are elementary and have
applicationsto a number of classical models. We begin by describingthe polynomial
subspaces.
Proposition 6.13
The following holds:
(i) F[u] = uu
t
admits
W
2
= L{1, t};
(ii) F[u] = uu
tt
admits
W
3
= L{1, t, t
2
};
(iii) F[u] = u
t
u
tt
admits
W
4
= L{1, t, t
2
, t
3
}.
Example 6.14 (2mth-order parabolic equation) This construction is associated
with the Oron–Rosenau solutions in Example 1.9. Consider a quasilinear parabolic
equation,
v
t
= L[
√
v] + p(x)(v≥ 0), (6.22)
where L is a linear 2mth-order elliptic operator with coefficients independent of t,
and p(x) is a given function. Setting v = u
2
yields
uu
t
=
1
2
L[u] +
1
2
p. (6.23)
© 2007 by Taylor & Francis Group, LLC
6 Applications to Nonlinear PDEs in IR
N
281
By Proposition 6.13(i), there exist solutions
u(x , t) = C
1
(x ) + C
2
(x )t ∈ W
2
. (6.24)
Substituting this into (6.23) yields a system of elliptic PDEs in IR
N
,
C
1
C
2
=
1
2
L[C
1
] +
1
2
p,
C
2
2
=
1
2
L[C
2
].
For instance, the fast diffusion equation with reaction or absorption terms
v
t
= (
√
v) + δ
√
v + p
possesses solutions v = u
2
,whereu is given by (6.24) with the elliptic system
C
1
C
2
=
1
2
(C
1
+ δC
1
+ p),
C
2
2
=
1
2
(C
2
+ δC
2
).
Example 6.15 (Hyperbolic equation) It follows from Proposition 6.13(ii) that the
hyperbolic PDE
uu
tt
+ µu
t
= L[u] + p(x )
has solutions
u(x , t) = C
1
(x ) + C
2
(x )t + C
3
(x )t
2
∈ W
3
,
2C
1
C
3
+ µC
2
= L[C
1
] + p,
2C
2
C
3
+ 2µC
3
= L[C
2
],
2C
2
3
= L[C
3
].
(6.25)
For instance, such solutions exist for the quasilinear hyperbolic(in {u > 0}) equation
with damping,
uu
tt
+ µu
t
= u + p(x)(L = ).
Note also that W
3
is admitted by the operator (uu
t
)
t
. Therefore, setting v = u
2
(v ≥ 0) in the quasilinear hyperbolic PDE yields
v
tt
= L[
√
v] + p(x) ⇒ 2(uu
t
)
t
= L[
√
u] + p,
possessing solutions on W
3
.
Example 6.16 Using Proposition 6.13(iii), consider the PDE
u
t
u
tt
= L[u] + p(x ),
possessing solutions on W
4
,
u(x , t) = C
1
(x ) + C
2
(x )t + C
3
(x )t
2
+ C
4
(x )t
3
, (6.26)
2C
2
C
3
= L[C
1
] + p,
4C
2
2
+ 6C
2
C
4
= L[C
2
],
18C
3
C
4
= L[C
2
],
18C
2
4
= L[C
4
].
© 2007 by Taylor & Francis Group, LLC
282 Exact Solutions and Invariant Subspaces
Example 6.17 (Forced quasilinear wave equation) It is not difficult to extend
this approach to other classes of PDEs. For instance, setting v = u
3
in the forced
quasilinear wave equation yields a cubic equation,
v
tt
=∇·
v
−
2
3
∇v
+ µv
1
3
+ ν ⇒ (u
2
u
t
)
t
= u +
µ
3
u +
ν
3
.
Since the operator on the left-hand side admits W
2
= L{1, t}, there exist solutions
(6.24) with the coefficients satisfying the elliptic system
2C
1
C
2
2
= C
1
+
µ
3
C
1
+
ν
3
,
2C
3
2
= C
2
+
µ
3
C
2
.
We next consider trigonometric, exponential, and other subspaces.
Proposition 6.18
The following holds:
(i) F[u] = uu
t
− u
2
admits
W
2
= L{1, e
t
};
(ii) F[u] = uu
tt
− u
2
admits
W
3
= L{1, e
t
, e
−t
};
(iii) F[u] = uu
tt
+ u
2
admits
W
3
= L{1, cost, sin t};
(iv) F[u] = u
t
u
tt
− u
2
admits
W
4
= L{1,ϕ(t)},
where
ϕ
ϕ
= ϕ
2
.
Concerning case (ii), see more general Proposition 4.23.
Example 6.19 Consider the PDE (6.22) with an extra linear term,
v
t
= L[
√
v] + p(x) + 2v,
so that v = u
2
yields
F[u] ≡ uu
t
− u
2
=
1
2
L[u] +
1
2
p.
Using Proposition 6.18(i) yields the exact solutions
u(x , t) = C
1
(x ) + C
2
(x )e
t
,
−C
2
1
=
1
2
L[C
1
] +
1
2
p,
−C
1
C
2
=
1
2
L[C
2
].
Example 6.20 As a new second-order PDE, consider
v
tt
= L[
√
v] + p(x) + 4v.
Setting v = u
2
again yields
F[u] ≡ (uu
t
)
t
− 2u
2
=
1
2
L[u] +
1
2
p.
This operator F admits the exponential subspace W
3
in (ii), so there exists
u(x , t) = C
1
(x ) + C
2
(x )e
t
+ C
3
(x )e
−t
,
−2C
2
1
− 4C
2
C
3
=
1
2
L[C
1
] +
1
2
p,
−3C
1
C
2
=
1
2
L[C
2
],
−3C
1
C
3
=
1
2
L[C
3
].
© 2007 by Taylor & Francis Group, LLC
