Furusawa A., van Loock P. Quantum Teleportation and Entanglement: A Hybrid Approach to Optical Quantum Information Processing

Подождите немного. Документ загружается.

60 1 Introduction to Quantum Information Processing

tal evolution is completely speciﬁed through 2N

real parameters. For the more

interesting case of physical stabilizer states corresponding to Gaussian states (see

Chapters 2 and 3), instead of the 2N

real coefﬁcients, 2N

complex coefﬁcients are

needed, corresponding to 4N

real parameters. The formalism of complex-valued

stabilizers for physical qumode stabilizer states and their Clifford evolution will

be discussed in Chapters 2 and 3. The Gottesmann–Knill theorem for qumodes

then states that Gaussian operations on Gaussian states can be efﬁciently simulat-

ed classically [90].

Similar to the qubit case, one may also consider a CNOT gate for qumodes. This

is deﬁned as CNOT D exp(2i Ox

˝Op

) D X

( Ox

) D Z

(Op

), corresponding to an

x-controlled x-displacement of mode 2 and a p-controlled p-displacement of mode

1: Ox

!Ox

COx

, Op

!Op

Op

, Ox

!Ox

,and Op

!Op

. As opposed to the C

gate,

CNOT is no longer symmetric under exchange of the two modes. The CNOT gate

can be obtained from the C

gate through local Fourier transforms,

(1 ˝ F

†

)exp(2iOx

˝Ox

)(1 ˝ F) D exp(2i Ox

˝Op

) . (1.133)

Universal sets

Qubits

fH, Z

π/2

, Z

π/4

, C

single-qubit gates: Z-Pauli (phase ﬂip):

Zj˙i D ji , ZjkiD(1)

jki

general Z-rotation:

D exp(iθ Z/2)

π/4-phase gate:

†

π/2

D Z , Z

†

π/2

DY (Clifford)

π/8-phase gate:

†

π/4

D Z , Z

†

π/4

(X  Y ) (non-Clifford)

Hadamard:

HjkiD

j0iC(1)

j1i

, HXH D Z , HZH D X (Clifford)

X-Pauli (bit ﬂip):

X jkiDjk ˚1i , X j˙i D ˙j˙i

1.8 Quantum Computation 61

two-qubit gate:

jki˝jliD(1)

jki˝jli (Clifford)

¬¬¬¬ Qumodes

fF, Z(s), D

(t), D

(), C

single-mode gates: WH-momentum shift:

Z(s)jpiDjp C si , Z(s)jxiDe

2isx

jxi

general phase (momentum) gate:

D D exp[i f ( Ox)] , for example D

(t) D exp(it Ox

)

quadratic gate:

†

(t) OxD

(t) DOx , D

†

(t) OpD

(t) DOp C t Ox (Clifford)

cubic gate:

†

(t) OxD

(t) DOx , D

†

(t) OpD

(t) DOp C

t Ox

(non-Clifford)

Fourier:

Fjxi

pos

dy e

2ixy

jy i

pos

Djxi

mom

†

OpF DOx , F

†

OxF DOp (Clifford)

WH-position shift:

X(s)jxiDjx C si , X(s)jp iDe

2isp

jpi

two-mode gate:

D exp(2i Ox ˝Ox) W C

jxi

pos

jpi

mom

Djxi

pos

jp C xi

mom

†

1,2

DOx

1,2

, C

†

1,2

DOp

1,2

COx

2,1

(Clifford)

Again, similar to the qubit case, the “magic state” for qumodes is obtained by

applying the non-Clifford, cubic phase gate upon a zero-momentum eigenstate,

(t)jp D 0iDe

it Ox

dxjxiD

dxe

itx

jxi . (1.134)

This is the so-called cubic phase state [28].

62 1 Introduction to Quantum Information Processing

In the current section, we attempted to give an overview of various important no-

tions in quantum computation including those of universality and scalability in the

context of both qubit and qumode approaches. Universality in either approach will

require some form of nonlinearity which may only be indirectly incorporated into a

quantum computation through measurements or directly through some effective-

ly enhanced weak nonlinear interaction. In the former scenario, a measurement-

based model of quantum computation is applied, as we shall discuss in the context

of experimental implementations in Chapters 6 and 7. The idea of weak nonlin-

ear interactions is most intuitively realized in hybrid protocols in which both qubit

and qumode systems participate (see Chapter 8). Once universality is achieved, in-

cluding non-Clifford gates, in principle, a quantum computation can no longer be

simulated classically in an efﬁcient way.

Even when universality can be attained in principle, scalability remains a subtle

issue. This issue will be part of the subsequent discussions on optical approaches

to quantum computation.

Another topic of great importance is fault tolerance. Without some form of (con-

catenated) quantum error correction, a quantum computer will remain a theoreti-

cal construct. As we discussed before, quantum communication too must rely upon

some form of quantum error detection when it is to be extended over larger dis-

tances. A complete treatment of fault tolerance for quantum information process-

ing and computation is beyond the scope of this introductory chapter on quantum

information. Nonetheless, in the n ext section, we shall at least mention the basic

concepts of quantum error correction.

1.9

Quantum Error Correction

Quantum information processing and computation became an area of practical

interest with potential real-world applications only after the discovery of quantum

error correction (QEC) codes [5, 21, 91, 92]. Shor’s code [21] was proposed at a

time when people believed that QEC unlike classical error correction would be

impossible. These initial doubts originated mainly from two supposed obstacles.

First, in classical error correction, the most natural way for protecting informa-

tion against errors is to use redundancy. However, to create redundancy in the quan-

tum case (by encoding qubits into multiple copies of the same qubits) appeared to

be forbidden even in principle by the quantum mechanical no-cloning theorem

(recall Section 1.1). Further, a second complication seemed to exist, following from

the fundamental nature of quantum information: encoded into complex-amplitude

superposition states, as opposed to classical digital information, quantum informa-

tion is inherently continuous.Thisevenholdsforjustasinglequbit.

Despite these initial doubts, Shor’s discovery and the many subsequent results

on QEC demonstrated that there are two speciﬁc solutions to the two main prob-

lems mentioned in the preceding paragraph. A kind of redundancy can be obtained

in the quantum case by encoding quantum information globally into entangled

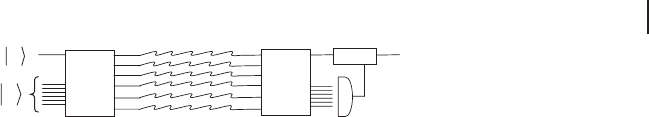
1.9 Quantum Error Correction 63

Correction

Encoding

Decoding

Syndrome

Figure 1.12 Basic elements of quantum error

correction. Most commonly, the signal state

jψi and a set of ancillae in some standard

initial state jAi are unitarily transformed into

an encoded state. After the effect of the errors,

typically assumed to occur individually and

independently on every subsystem, a unitary

decoding circuit and a subsequent syndrome

measurement of the ancillae reveal the type

and location (and, for example, for qumodes,

also the size) of the error. A ﬁnal correction

operation on the signal system will then recov-

er the original state with a ﬁdelity greater than

that for an unprotected signal state, depend-

ing on the correctable set of errors for the

speciﬁc code and on the actual error model.

states that are deﬁned in a larger Hilbert space than the original signal space. These

encoded states do not correspond to multiple copies of the original state and so do

not violate no-cloning. For example, an arbitrary qubit state, jψiDaj0iCbj1i,

may be encoded into an entangled state of three physical qubits as

47)

jψi˝j0i˝j0i!aj000iCbj111i¤jψi˝jψi˝jψi . (1.135)

This encoding can be achieved by pairwise applying two CNOT gates upon the

signal qubit together with the ﬁrst ancilla qubit as well as with the second one.

Eventually, local bit-ﬂip errors occurring on exactly one of the three qubits can be

detected and corrected, as we shall discuss in more detail shortly. The detection

of the error will depend on some form of measurement, and it i s this so-called

syndrome measurement step which enables one to correct arbitrary, even continu-

ous errors. This effect is called discretization of errors because a continuous error

is reduced to a ﬁnite, discrete set of Pauli errors. We will illuminate this essential

feature of QEC in the following section. Figure 1.12 shows the basic elements of

QEC as applicable to both qubits and qumodes.

1.9.1

Discretization

In the preceding section, we wrote universal sets for qubits and qumodes in terms

of single-variable gates, that is, gates diagonal in the computational variables Pauli

Z and position Ox, respectively. For universality, at least one diagonal gate needed to

have a rotation angle ¤ kπ/2 on the Bloch sphere for qubits and a Hamiltonian of

> quadratic order for qumodes. In addition, the Hadamard and the Fourier gates

were required in order to affect multi-variable gates.

The simplest manifestation of a QEC code also works with single-variable gates.

However, quite remarkably, universality, that is, universal protection against ar-

bitrary single-variable errors (including non-Clifford-type errors) follows directly

47) Recall the discussion of the preceding section. The state in Eq. (1.135) may as well be interpreted

as a certain superposition state of an eight-level particle. However, in this case, encoding,

occurrence of local errors, and syndrome identiﬁcation lack the nice physical and operational

meaning of the multi-particle scenario.

64 1 Introduction to Quantum Information Processing

from the ability of a code to correct the simplest single-variable errors, for instance,

Pauli X bit-ﬂip errors for qubits and WH X(s) position shift errors for qumodes.

Let us see how this works.

Consider a single qubit in an arbitrary state, jψiDaj0iCbj1i. First, the error

model shall be described by a simple one-qubit bit ﬂip Pauli channel, with

E ( O) D

(1  p) O C pXOX (see Section 1.4.1): with probability p a bit ﬂip occurs; otherwise,

the state remains unchanged. So the error set is discrete and ﬁnite, consisting only

of Pauli X errors. Hence, the correctable error set should contain at least one-qubit

X errors. Using the encoded state in Eq. (1.135) and applying the channel map

upon every physical qubit independently gives the output density operator,

(1  p)

O

enc

C p (1  p )

kD1

O

enc

C p

(1  p)

l<kD2

˝ X

) O

enc

˝ X

) C p

˝3

O

enc

˝3

, (1.136)

with O

enc

 (aj000iCbj111i)(a



h000jCb



h111j). Now, if we were able to discrim-

inate the orthogonal subspaces spanned by fj000i, j111ig and fX

j000i, X

j111ig

with k D 1, 2, 3, without changing the original amplitudes of the corresponding

terms, we could at least identify the errors up to O(p

). In fact, the three-qubit code

achieves exactly this. It uses four orthogonal subspaces, each two-dimensional with

enough space to preserve the original qubit, which correspond to the four cases of

no error at all and a bit-ﬂip error occurring on any one of the three qubits. As a

result, through the three-qubit repetition code, the effective error probability is re-

duced from p to p

. Higher repetitions may lead to even better error suppression.

From this, it also becomes clear why two physical qubits are not enough for

such a bit-ﬂip code: in the four-dimensional physical Hilbert space of two qubits,

there are only two possible orthogonal, two-dimensional subspaces; not enough

for obtaining and discriminating all the three cases of an error occurring on either

qubit (fX

j00i, X

j11ig), with k D 1, 2, and no error happening at all (fj00i, j11ig),

which would require six physical dimensions. However, i f we are satisﬁed with

only detecting whether an error occurred ( without correcting it), two qubits would

be enough since the no-error subspace fj00i, j11ig can still be discriminated from

the error subspaces fX

j00i, X

j11ig. In general, this dimensional argument tells

us how many physical qubits will be needed for a given error model and a desired

correctable error set.

Now, let us consider a channel which is more general than the bit-ﬂip channel

and allow for an arbitrary X-error, that is, an arbitrary X-rotation X

D e

iθ X/2

cos(θ /2)1  isin(θ /2) X . In this case, using again the three-qubit code, we would

still be able to correct the dominating errors by discriminating the orthogonal sub-

spaces fj000i, j111ig and fX

j000i, X

j111ig with k D 1, 2, 3. In fact, the syndrome

measurements that achieve this discrimination will reduce the total density oper-

ator again to terms which have no error at all or a bit ﬂip on exactly one qubit in

the leading order. More precisely, only terms like p (1 p)

cos

(θ /2) O

enc

and p(1

1.9 Quantum Error Correction 65

sin

(θ /2) X

O

enc

with k D 1, 2, 3 will remain after the syndrome detection,

and the off-diagonal terms like, for instance, p (1  p)

icos(θ /2) sin(θ /2)1 O

enc

vanish. In other words, even though the original error is a continuous X-rotation,

due to the syndrome measurement, this error will become asimplePauliX error or

result in no error at all. The ﬁnal correction operation then works as before by just

unﬂipping the corrupted qubit.

Now, consider a single qumode in an arbitrary state, jψiD

dx ψ(x)jxi.A

(perfectly) repetition-encoded three-qumode state in this case becomes

dx ψ(x)jxi˝jxi˝jxi . (1.137)

Now, whenever exactly one qumode is subject to an arbitrary Ox-error, acting as

i f ( Op)

, the syndrome detection discriminating between the subspaces fX

(s)jxxxij

8x 2 Rg with k D 1, 2, 3 and s 2 R would result in a state where exactly one

qumode is corrupted by a simple position shift. Since the location and the size of

this position shift will be known from the syndrome measurement, the original,

uncorrupted state can be recovered through a simple displacement operation on

the corresponding qumode. For example, e

i f ( Op)

acting upon qumode 1 leads to

i f ( Op

)

dx ψ(x)jxxxiDe

i f ( Op

)

dx ψ(x)

dpe

2ixp

jpxxi

dxdp ψ(x)e

2ixp

i f (p )

jpxxi

dxdy dp ψ(x)e

2i(y x)p

i f (p )

jyxxi . (1.138)

The syndrome identiﬁcation amounts to projecting qumodes 1 and 2 as well as

qumodes 2 and 3 onto the two-qumode projectors

dzjz, z  u

ihz, z  u

j with

syndromes u

and u

. In terms of the position operators, this corresponds to mea-

surements of the relative positions Ox

Ox

and Ox

Ox

with outcomes u

and u

respectively. When the error e

i f ( Op)

occurredonqumode1,wewillalwaysobtain

D 0, whereas the other projector gives

dzjz, z  u

ihz, z  u

dxdy dp ψ(x)e

2i(y x)p

i f (p )

jyxxi

dxdydp ψ(x)e

2i(y x)p

i f (p )

δ(y  u

 x)jy, y  u

, xi

dxdp ψ(x)e

2iu

i f (p )

jx C u

, x, xi

D g(u

)

dx ψ(x)jx C u

, x, xi . (1.139)

Though the function g(u

)  (1/π

dpe

2iu

i f (p )

) is a measurement-dependent

prefactor, the conditional state for every syndrome u

becomes

dx ψ(x)jx C

, x, xi which can be corrected as described above. Note that for simplicity,

we have used unnormalized states here and syndrome detections with inﬁnite

66 1 Introduction to Quantum Information Processing

resolution. In the realistic case, the encoded state would correspond to a three-

mode Gaussian state

48)

producible with two squeezed-state ancillary qumodes

using beam splitters (see Chapters 2 and 5). The inﬁnitely precise measurement

should be more realistically described by a ﬁnite syndrome window with projec-

tors

∆

dzjz, z  u

ihz, z  u

j. So when, for instance, g(u

) D δ(u

)for

the no-error case with e

i f (p )

 1, we would obtain

∆/2

∆/2

g(u

)

dx ψ(x)jx C

, x, xiD

dx ψ(x)jx, x, xi as the ﬁnal state.

To summarize, the mechanism for correcting arbitrary single-variable errors is

very similar for qubits and for qumodes. In either case, even when an arbitrary er-

ror diagonal in, for example, X (qubits) and Op (qumodes) may disturb a quantum

state in inﬁnitely many ways, the syndrome detection will map the original error

onto a simpler error from a smaller error set: for qubits, this would be a ﬂip in the

Z basis; for qumodes, a shift in the Ox basis. Although this guarantees that even

non-Clifford-type single-variable errors can be corrected by simple means, it does

not yet allow for the correction of multi-variable errors including two or more non-

commuting variables such as X and Z for qubits, and Ox and Op for qumodes. Such

full QEC codes, however, can be constructed by concatenating a single-variable

code using Hadamard and Fourier gates. The ﬁrst and certainly most famous full

QEC code is Shor’s nine-qubit code [21]. A qumode version of this code and its

experimental realization will be discussed in Chapter 5.

On the level of arbitrary channel (CPTP) maps, the effect of discretization in a

QEC protocol can be understood by expanding an arbitrary qubit Kraus operator

in the Pauli matrix basis as in Eq. (1.77). Similarly, the WH shift operators serve

as a complete basis for arbitrary qumode CPTP maps, see Eq. (1.78). In either

case, syndrome detections of Pauli and WH errors will then always remove the

offdiagonal terms of the channel output matrix and the remaining terms can be

easily corrected. In the qumode case, the reduced error set is, of course, not really

discrete. It is, nonetheless, smaller and simpler, containing only phase-space shift

errors.

Although universal QEC of arbitrary, multi-variable errors occurring on a subset

of the physical qubits or qumodes is possible, a subtlety remains when compar-

ing qubit and qumode QEC. This complication arises for the realistic scenario of

multi-channel errors. Typically, not only a single qubit or qumode will be subject to

an error. Usually, every subsystem will be corrupted, and so a hierarchy of errors

in terms of the frequency of their occurrence or their size will become important.

For instance, as we have seen for qubits, multiple-qubit bit-ﬂip errors may simply

be neglected when their probability scales as p

compared to the single-qubit error

probability p. Similarly, an amplitude damping error may be corrected up to an or-

der O(γ

) in the damping parameter (see Section 1.4.1 and Chapter 2) [5]. Howev-

er, for qumodes, amplitude damping becomes a Gaussian channel (see Chapter 2)

and, as such, it may simply no longer be correctable when the damping occurs

on every encoded qumode in every channel [93]. Nonetheless, whenever a stochas-

tic channel leads to a hierarchy of errors, arbitrary multi-variable, multi-channel

48) When the signal state jψiD

dx ψ(x)jxi is a Gaussian state, which is not a requirement here.

1.9 Quantum Error Correction 67

errors can be suppressed through the standard QEC codes, both for qubits and

qumodes [94]. In either case, whether a QEC code is useful at all and whether it

is efﬁcient depends on the correctable error set (for instance, the set of arbitrary

single-channel errors) and the given channel error model. The only basic assump-

tion typically is that the errors act independently on the individual subsystems.

1.9.2

Stabilizer Codes

A particularly important class of QEC codes is that of so-called stabilizer codes [95,

96], the quantum analogue of classical additive codes. Stabilizer codes are general-

izations of stabilizer states. This shall become clear in the present section.

In the DV setting, through an [N, k]stabilizercode,k logical qubits are encod-

ed into N physical qubits. The stabilizer group S, an abelian subgroup of the N-

qubit Pauli group

49)

with (N  k)stabilizergeneratorshg

, g

,...,g

Nk

i,deﬁnes

the codespace which is spanned by the set of simultaneous C1eigenvectorsofS.

Measuring the N  k stabilizer generators, yielding 2

Nk

classical syndrome bit

values, reveals which orthogonal error subspace an encoded input state is mapped

onto. Signal recovery is then achieved by mapping the state back into the codespace

with stabilizer eigenvalues C1.

Let us illustrate these deﬁnitions and notions for the three-qubit code of the pre-

ceding section. This code represents a very simple example of a stabilizer code. In

this case, k D 1 logical qubit is encoded into N D 3 physical qubits. The corre-

sponding [3, 1] code is deﬁned through the minimal set of N  k D 2 independent

stabilizer generators hg

 Z ˝ Z ˝1, g

 1 ˝ Z ˝Zi. This set uniquely deﬁnes

the stabilizer group S for the corresponding stabilizer code with a two-dimensional

codespace spanned by fj000i, j111ig.SinceS is abelian, and we have [g

, g

] D 0,

the basis vectors j000i and j111i can be simultaneous eigenvectors of g

and g

with eigenvalue C1.

The effect of the bit-ﬂip channel on the three physical qubits of the repetition

code, as described in the preceding section, can now be equivalently expressed in

terms of the stabilizers. Up to order O(p

), including only linear terms in p,we

obtain the following stochastic transformations of the stabilizer generators,

, Z

i!hZ

, Z

iI with probability (1  p)

, Z

i!hZ

, Z

iI p(1  p)

, Z

i!hZ

, Z

iI p (1  p )

, Z

i!hZ

, Z

iI p(1  p)

. (1.140)

The ﬁrst case in the top row corresponds to the no-error case; the encoded state

remains in the original codespace. In the other three cases, the encoded state is

subject to a bit ﬂip on any one of the three qubits; hence, the encoded state is

49) Which itself is formed by a tensor product of the one-qubit Pauli group. Recall from footnote 14

on page 19 that we omit all unnecessary prefactors of Pauli operators such as (˙i).

68 1 Introduction to Quantum Information Processing

mapped into one of the orthogonal subspaces fX

j000i, X

j111ig with k D 1, 2, 3.

These three error subspaces are each uniquely determined through the new sta-

bilizer generators, as shown in Eq. (1.140), and are each spanned by a new two-

dimensional set of simultaneous C1 eigenvectors. Th e syndrome measurement

will then reveal the change of the eigenvalues with respect to the original stabiliz-

ers, that is, those of the codespace, g

and g

. There are four syndrome outcomes

corresponding to the four cases of no error at all (g

DC1, g

DC1), a bit ﬂip on

qubit 1 (g

D1, g

DC1), a bit ﬂip on qubit 2 (g

D1, g

D1), and a bit

ﬂip on qubit 3 (g

DC1, g

D1). Thus, measuring the N  k D 2stabilizers

of the code uniquely determines the error. Mapping the state from one of the or-

thogonal error subspaces back into the original codespace enables one to recover

an uncorrupted version of the encoded state. This is a general feature of stabilizer

codes.

The three-qumode repetition code can be similarly expressed in terms of sta-

bilizers. In this case, we need N  k D 2 products of WH operators, hg

(s) 

Z(s) ˝ Z(s) ˝ 1, g

(s)  1 ˝ Z(s) ˝ Z(s)i, in order to represent the stabilizer

group and uniquely deﬁne a one-qumode codespace as a subspace of the whole

three-qumode space. This inﬁnite-dimensional subspace is spanned by the basis

vectors fjxxxij8x 2 Rg,whicharesimultaneousC1 eigenvectors of the stabiliz-

ers g

(s)andg

(s). More conveniently expressed in terms of the WH generators Ox

and Op,wehaveN k D 2 so-called nulliﬁer conditions, Ox

Ox

D 0and Ox

Ox

D 0

since these combinations must have fjxxxij8x 2 Rg as their simultaneous zero-

eigenvectors. The syndrome information now becomes continuous, corresponding

to the eigenvalues of Ox

Ox

D u

and Ox

Ox

D u

after an error occurred on

any one of the three qumodes. Every pair of these eigenvalues uniquely determines

one of the orthogonal error subspaces, fX

(s)jxxxij8x 2 Rg with k D 1, 2, 3 and

s 2 R, into which the encoded state is mapped by the channel. Compared with the

qubit case in Eq. (1.140), the stabilizer map now becomes

(s)Z

(s), Z

(s)Z

(s)i

2isu

(s)Z

(s), e

2isu

(s)Z

(s)

, (1.141)

with the syndrome information contained in the phase factors e

2isu

and e

2isu

Though the syndrome is now continuous, the QEC mechanism is very similar to

the qubit case; however, the stochastic nature of the qubit channels, as illustrated

by Eq. (1.140), will be missing in the most important examples of qumode channels

(see Chapter 2).

We have used the notion of stabilizers and stabilizer states already at various

times. A stabilizer is a (not necessarily unitary) operator M that, for some vector

jψi, has the property M jψiDjψi. If there is a commuting set of such stabilizers

g such that M

jψiDjψi, 8i, jψi may be a unique state vector or an arbitrary

vector in a uniquely deﬁned subspace. In fact, the former case is a special case of

the latter one.

For instance, for N qubits, N  k Pauli generators will deﬁne a 2

-dimensional

subspace

C of the 2

-dimensional N-qubit space. This subspace C represents a

1.9 Quantum Error Correction 69

stabilizer code, and the stabilizer condition becomes M

jψiDjψi, 8i and 8jψi2

C.Now,thespecialcasewithk D 0meansthatC is speciﬁed through N Pauli

generators. In this case,

C has a dimension such that M

jψiDjψi, 8i uniquely

deﬁnestherank-1projectorjψihψj corresponding to a pure N-qubit state. These

deﬁnitions are similar for qumodes. Later, we shall use full sets of N Pauli and WH

stabilizers in order to deﬁne mul ti-party entangled N-qubit and N-qumode graph

states, respectively.

Stabilizers and stabilizer codes

stabilizer: any operator M such that MjψiDjψi

stabilizer code: any subspace deﬁned by a commuting stabilizer set fM

stabilizer state: any such 1-dimensional subspace (pure-state projector)

Qubits

stabilizer codes:

any 2

-dimensional subspace C of the 2

-dimensional N-qubit space de-

ﬁned through N  k Pauli stabilizer generators hg

, g

,...,g

Nk

i such that

, g

] D 0andg

jψiDjψi, 8i, j and 8jψi2C

stabilizer states:

any 2

D 1-dimensional subspace jψihψj of the 2

-dimensional N-qubit

space deﬁned through N Pauli stabilizer generators hg

, g

,...,g

i such that

, g

] D 0andg

jψiDjψi, 8i, j

¬¬¬¬ Qumodes

stabilizer codes:

any k-qumode subspace

C of the inﬁnite-dimensional N-qumode space de-

ﬁned through N  k WH stabilizers hg

(s), g

(s),...,g

Nk

(s)i such that

(s), g

(s)] D 0andg

(s)jψiDjψi, 8i, j ; s 2 R,and8jψi2C

stabilizer states:

any 1-dimensional subspace jψihψj of the inﬁnite-dimensional N-qumode

space deﬁned through N WH stabilizers hg

(s), g

(s),...,g

(s)i such that

(s), g

(s)] D 0andg

(s)jψiDjψi, 8i, j ; s 2 R

A great advantage of QEC schemes is that they are deterministic which makes

them directly applicable to quantum computation. However, this comes at a price.

Encoding logical quantum information into a sufﬁciently large physical system

will require expensive resources. Alternatively, probabilistic quantum error detec-

tion and, in particular, entanglement puriﬁcation schemes [22] may be employed

in order to reduce the (spatial) resource consumption and the complexity of the

quantum circuits for implementing the protocol. This would then be more useful

for quantum communication applications, as described in Section 1.7.2. We shall