Furusawa A., van Loock P. Quantum Teleportation and Entanglement: A Hybrid Approach to Optical Quantum Information Processing
Подождите немного. Документ загружается.

180 4 Quantum Teleportation
4.1
Qubit Quantum Teleportation
As we explained in Section 1.6, through DV quantum teleportation, an arbitrary,
unknown qubit state can be transferred using shared entanglement and classical
communication. If instead, without using shared entanglement, Alice and Bob at-
tempted to transfer the qubit state, A lice would have to measure the state in order
to gain as much information as possible and to convey this classical information
to Bob who would eventually prepare a quantum state according to that informa-
tion. This classical teleportation of an unknown qubit state achieves a fidelity of
F D 2/3 at most. This value represents the boundary between classical and quan-
tum teleportation when unknown qubit states are to be transmitted [193]. Let us
now discuss various qubit teleportation experiments.
4.1.1
Experiment: Qubit Quantum Teleportation
One of the most famous experiments of qubit teleportation was performed by
Bouwmeester et al. [194]. In this section, we shall explain it in detail.
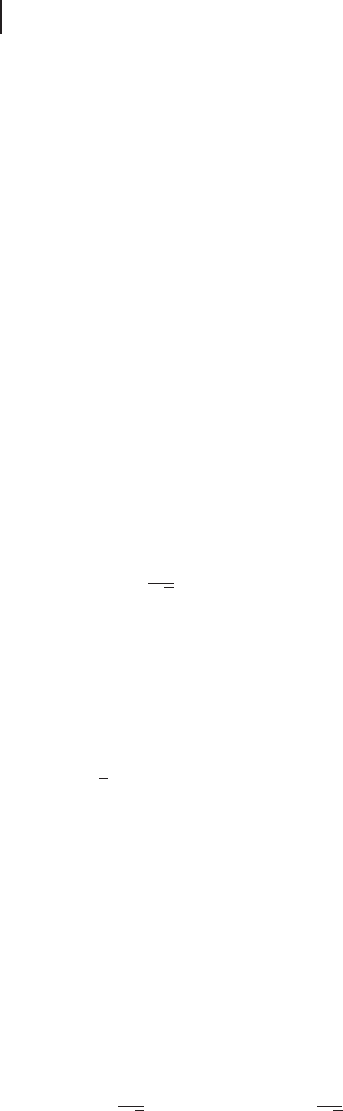
Figure 4.1 shows the scheme of qubit teleportation as done by Bouwmeester
et al. [194]. First, Alice and Bob share a polarization entangled photon pair,
jΨ
()
iD
1
p
2
(
j$i
A
˝jli
B
jli
A
˝j$i
B
)
, (4.1)
which can be created by the method shown in Section 3.1.3.1.
Alice now performs a Bell measurement on the input c
0
j$i
in
C c
1
jli
in
and her
entangled photon. This Bell measurement can be understood from the following
decomposition (recall Section 1.6),
(
c
0
j$i
in
C c
1
jli
in
)
˝
ˇ
ˇ
Ψ
()
˛
AB
D
1
2
h
ˇ
ˇ
Ψ
()
˛
inA
˝
(
c
0
j
$
i
B
c
1
j
l
i
B
)
C
ˇ
ˇ
ˇ
Ψ
(C)
E
inA
˝
(
c
0
j
$
i
B
C c
1
j
l
i
B
)
C
ˇ
ˇ
Φ
()
˛
inA
˝
(
c
0
j
l
i
B
C c
1
j
$
i
B
)
C
ˇ
ˇ
ˇ
Φ
(C)
E
inA
˝
(
c
0
j
l
i
B
c
1
j
$
i
B
)
i
, (4.2)
where jΨ
(˙)
i and jΦ
(˙)
i are the Bell states defined in Eq. (3.19).
In the experiment of Bouwmeester et al.,onlythecaseofjΨ
()
i
inA
can be detect-
ed. This is because their scheme of Bell measurement corresponds to simultaneous
detection of photons at detectors f1 and f2 as follows.
Simultaneous detection of photons at detectors f1 and f2 may be represented by
h0jOa
j
f1
Oa
k
f2
[195], where j, k D$, l and,
Oa
j
f1
D
1
p
2
Oa
j
in
COa
j
A
, Oa
j
f2
D
1
p
2
Oa
j
in
Oa
j
A
. (4.3)
4.1 Qubit Quantum Teleportation 181
U
BSM
EPR-
source
Teleported state
Teleported
state
ALICE
ALICE
f1
BS
f2
Initial
state
Initial
state
UV-pulse
p
Classical
information
Classical
information
“coincidence”
2
Entangled pair
EPR-source
BOB
BOB
Analysis
d1
d2
PBS
2
1
1
3
3
4
(a)
(b)
Figure 4.1 Scheme of qubit quantum teleportation demonstrated by Bouwmeester et al. [194].
(a) the concept, (b) the experimental setup.
Here, the relations of Eq. (4.3) come from a hal f beam splitter at A lice. Therefore,
h0jOa
j
f1
Oa
k
f2
becomes
h0jOa
j
f1
Oa
k
f2
D
1
2
h0j
Oa
j
in
Oa
k
in
COa
j
A
Oa
k
in
Oa
j
in
Oa
k
A
Oa
j
A
Oa
k
A
. (4.4)
If we neglect the probability of two photons in the input and Alice’s mode, we can
omit Oa
j
in
Oa
k
in
and Oa
j
A
Oa
k
A
in Eq. (4.4). Thus, the final expression of h0jOa
j
f1
Oa
k
f2
becomes
h
0
j
Oa
j
f1
Oa
k
f2
D
(
˙
1
p
2
inA
˝
Ψ
()
ˇ
ˇ
j ¤ k
0 j D k .
(4.5)
182 4 Quantum Teleportation
From this consideration, simultaneous detection of photons at detectors f1 and f2
is physically equivalent to projection onto jΨ
()
i
inA
.
With the scheme of Bell measurement, one can detect the case of jΨ
()
i
inA
in
Eq. (4.2). So when detectors f1 and f2 have simultaneous “clicks”, the overall state
of the input and an entangled photon pair of Alice and Bob in Eq. (4.2) shrinks into
jΨ
()
i
inA
˝
(
c
0
j$i
B
c
1
jli
B
)
DjΨ
()
i
inA
˝
(
c
0
j$i
B
C c
1
jli
B
)
. (4.6)
Since we can neglect overall phase, Bob can recover the input state at his place
(mode).
Note that this scheme depends on post-selection and only the case of jΨ
()
i
inA
can be determined from the four Bell states jΨ
(˙)
i
inA
and jΦ
(˙)
i
inA
.Thismeans
that 3/4 of events are discarded and so the success probability is 1/4.
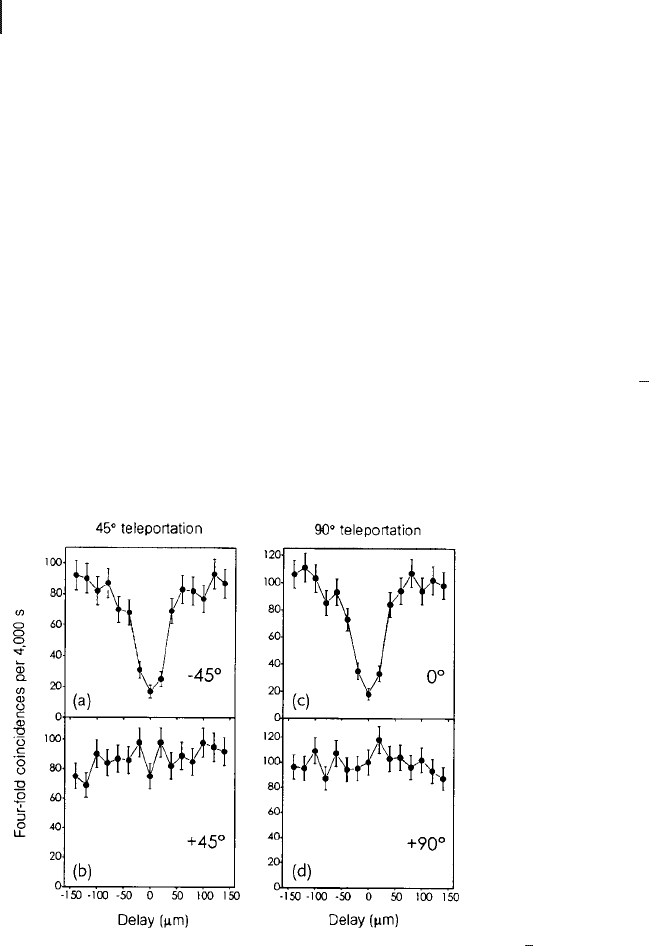
1)
Figure 4.2 shows the experimental results for teleportation of (j$i
in
Cjli
in
)/
p
2
and jli
in
demonstrated by Bouwmeester et al. [194]. It was checked with four-fold
coincidence of detection of photons at detector p, f1, f2, and d1 (d2), where “click”
at detector p shows the existence of input photon, simultaneous “clicks” at detec-
tors f1 and f2 correspond to projection onto jΨ
()
i
inA
, and “click” at detector d1
Figure 4.2 Experimental results of qubit
quantum teleportation demonstrated by
Bouwmeester et al. [194]. They checked four-
fold coincidence of detection of photons at de-
tector p, f1, f2, and d1 (d2). (a) and (b) reflect
thecaseof45
ı
input ((1/
p
2)(j$i
in
Cjli
in
)),
(c) and (d) reflect the case of 90
ı
input
(jli
in
). (a) and (c) are the reference experi-
ments.
1) Which is only half as big as the maximal success probability of 1/2 for a Bell measurement using
linear optics without ancilla photons and feedforward [196] (see Chapter 2).

4.1 Qubit Quantum Teleportation 183
(d2) shows the proper output. Thus, four-fold coincidences around zero delay in
Figure 4.2 mean the success of teleportation (Figure 4.2a and c are references).
Braunstein and Kimble pointed out the disadvantage of this scheme [197]. It
comes from the indistinguishability between a single photon and two photons in
the input using an on-off detector without resolving photon numbers. In the case
of two photons in the input, simultaneous detection of photons at detectors p, f1
and f2 occurs as the case of success of teleportation without entangled photons of
Alice and Bob. It is because we cannot neglect Oa
j
in
Oa
k
in
in Eq. (4.4) and then simulta-
neous detection of photons at detectors f1 and f2 no longer means projection onto
jΨ
()
i
inA
. Therefore, one must detect a photon at the output (detector d1 or d2) to
verify the success of teleportation. In other words, four-fold coincidence is a neces-
sary condition here for the success of teleportation. However, in the teleportation
scheme described here, a survival and a potentially further expl oitation of the tele-
ported state by avoiding post-selection is possible when photon-number resolving
detectors are used.
4.1.2
Experiment: Qubit Telecloning
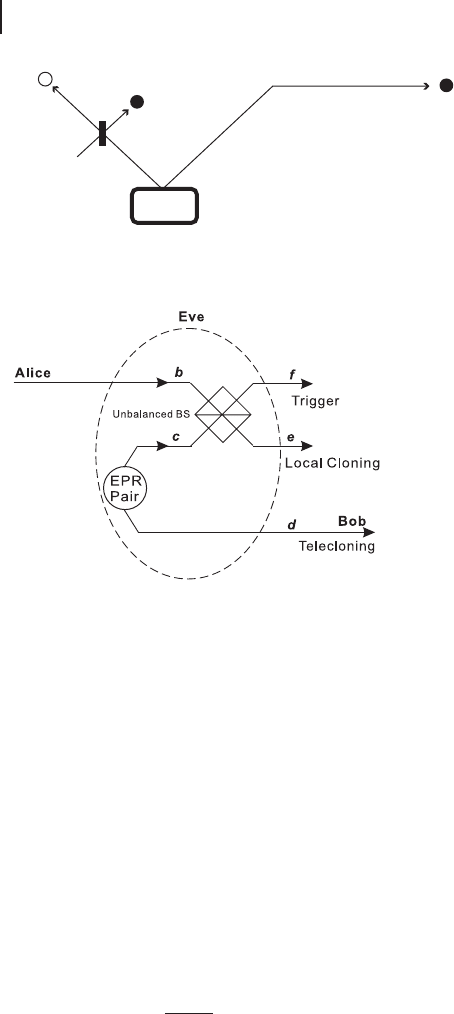
Telecloning is a generalized version of teleportation where n senders send the
same quantum information to m receivers ( n-to-m telecloning) [198]. Since the no-
cloning theorem [14, 15] prohibits the receivers to have perfect clones of the input
state, the clones are approximate ones which have higher fidelities to the original
than the classical limit [25]. Although complete telecloning for qubits is not re-
alized so far, “partial” telecloning is proposed by Filip [199] and demonstrated by
Zhao et al. [200]. In this section, we will explain the experiment in detail. Again,
this is also a post-selection experiment with polarization qubits (single photons).
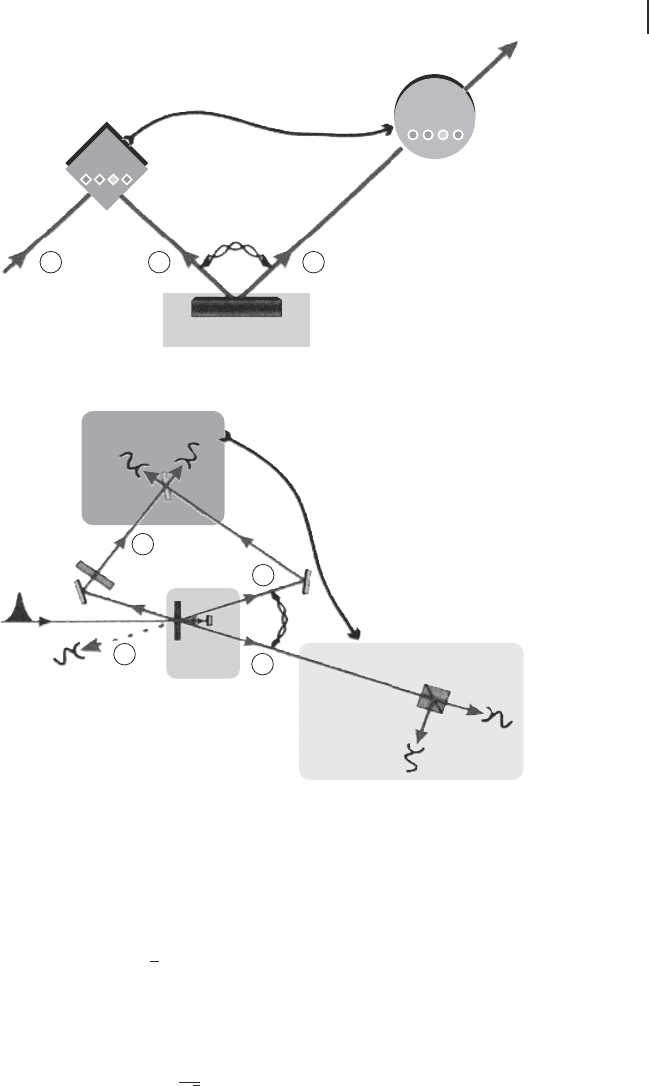
Figure 4.3 shows the proposed scheme of “partial” telecloning by Filip [199]
which was actually proposed as “partial teleportation”. Zhao et al. used an anti-
clone in Figure 4.3 as a trigger, and demonstrated the “partial” telecloning with the
scheme shown in Figure 4.4 [200]. In these schemes, an input is cloned to two,
one is a local clone and the other is distant one (teleclone). In any case, the scheme
is very similar to the one for qubit teleportation explained in the previous section.
The only difference is that a half beam splitter for Alice’s Bell measurement in
Figure 4.1 is replaced by an asymmetrical (unbalanced) beam splitter as shown in
Figures 4.3 and 4.4.
Figure 4.5 shows the experimental setup of “partial” telecloning demonstrated
by Zhao et al. [200]. In Figure 4.5, an asymmetric beam splitter is realized by a
Mach–Zehnder interferometer [201].
The asymmetric beam splitter transforms modes b and c to modes e and f as
follows,
2)
b ! t e Cir f , c ! ir e C t f , (4.7)
2) Here, we drop the hats on annihilation operators and use “mode” and “mode operator”
synonymously.
184 4 Quantum Teleportation
ALICE
BOB
clone
distant clone
anti-clone
R
ψ
Ψ
–
I
S
S’
Figure 4.3 Proposed scheme of “partial” telecloning as partial teleportation by Filip [199]. S, S
0
are clones, I is an anti-clone. R: reflectivity of beam splitter.
Figure 4.4 Experimental scheme of “partial” telecloning demonstrated by Zhao et al. [200].
where R D r
2
,1R D t
2
,andR is the reflectivity of the asymmetric beam splitter.
If an input to the cloner is in the state jψiDαjli C βj$i and the outputs from
the asymmetric beam are single photons in modes e and f, the overall state jΨ i
all
can be written as follows [199],
jΨ i
all
D α(1 2R)jli
e
jli
f
j$i
d
β(1 2R)j$i
e
j$i
f
jli
d
α(1 R) jli
e
j$i
f
jli
d
C αRj$i
e
jli
f
jli
d
C β(1 R)j$i
e
jli
f
j$i
d
βRjli
e
j$i
f
j$i
d
, (4.8)
where modes c and d are originally in the entangled state jΨ
()
i
cd
defined in
Eq. (3.19).
When detectors D
2
and D
4
detect photons as j$i
f
jli
d
,thestateofmodee be-
comes α(1R) jli
e
Cβ(12R)j$i
e
, whose fidelity to the input jψiDαjliCβj$i
is [199],
F
local clone
D
1
2P(R)
(1 2R)
2
C (1 R)
2
, (4.9)
where P(R) D 13R C3R
2
. Similarly, when detectors D
2
and D
4
detect photons as
jli
f
j$i
d
,thestateofmodee (local clone) becomes α(1 2R)jli
e
Cβ(1R)j$i
e
,
whose fidelity is the same as Eq. (4.9).
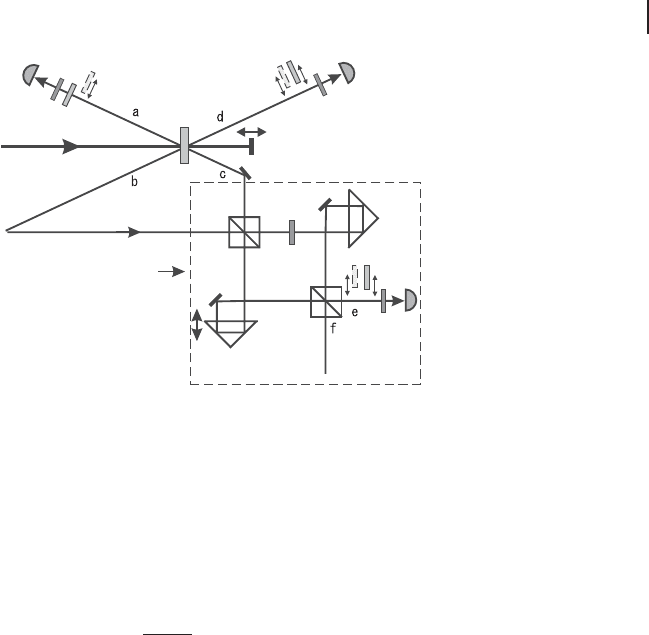
4.1 Qubit Quantum Teleportation 185
Mach-Zehnder
Interferometer
M
UV
BBO
F
F
F
λ/4 λ/4
λ/4
P
1
P
4
P
3
D
4
D
3
DL
1
BS
1
BS
2
C
Figure 4.5 Experimental setup of “partial”
telecloning demonstrated by Zhao et al. [200].
P: pola rizer, F: bandpath filter, DL: delay line,
BBO: second-order nonlinear crystal (beta-
barium borate), BS: beam splitter, D: photon
detector, λ/4: quarter wave plate. The Mach–
Zehnder interferometer acts as a variable
beam splitter. C is compensator to equalize
the path length of orthogonal polarizations.
When detectors D
2
and D
3
detect photons as jli
e
j$i
f
,thestateofmoded (dis-
tant clone or teleclone) becomes α(1R)jli
d
β Rj$i
d
, whose fidelity to the input
jψiDαjli C βj$i is [199]
F
teleclone
D
1
2P(R)
R
2
C (1 R)
2
. (4.10)
Similarly , when detectors D
2
and D
3
detect photons as j$i
e
jli
f
,thestateofmode
d (distant clone or teleclone) becomes α Rjli
d
C β(1 R)j$i
d
,whosefidelityis
the same as in Eq. (4.10).
Here, classical limit of the fidelity for one-to-two cloning is 2/3. From Eqs. (4.9)
and (4.10), one can obtain the fidelity of 5/6 for both clones with R D 1/3, which is
higher than the classical limit and thus shows the success of “partial” telecloning.
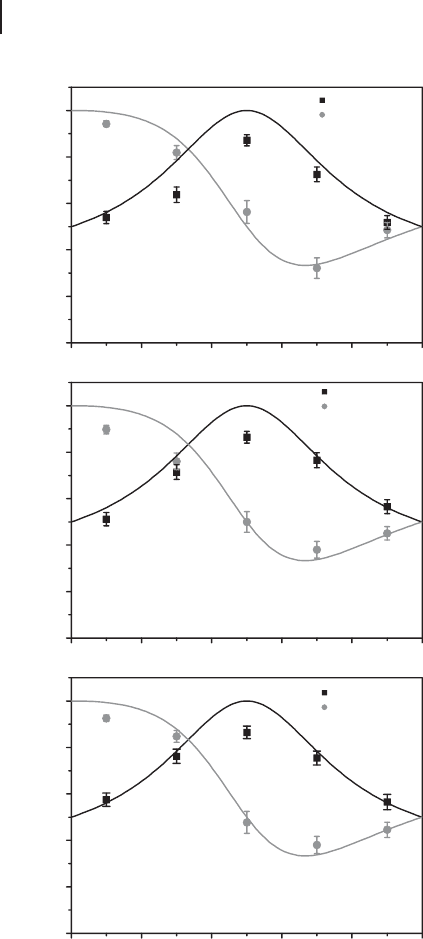
Zhao et al. checked the fidelities as a function of reflectivity R of the asymmetric
beam splitter as shown in Figure 4.6. Theoretical curves are calculated by Eqs. (4.9)
and (4.10). The experimental results agree well with the theoretical prediction and
show the higher fidelities than the classical limit of 2/3 with R D 1/3. Thus “par-
tial” telecloning is successfully demonstrated.
4.1.3
Experiment: Qubit Entanglement Swapping
With post-selection illustrated in the previous sections, entanglement swapping –
teleportation of entanglement – can be performed for polarization qubits. P an et al.
implemented this for the first time [202]. Here, we will explain their experiment in
detail.
186 4 Quantum Teleportation
Telecloning
Local-cloning
1.0
0.8
Fidelity
0.6
0.4
0.2
0.0
1.0
0.8
Fidelity
0.6
0.4
0.2
0.0
1.0
0.8
Fidelity
0.6
0.4
0.2
0.0
0.0 0.2 0.4 0.6
Reflectivity
0.8 1.0
Telecloning
Local-cloning
Telecloning
Local-cloning
(a)
(b)
(c)
Figure 4.6 Experimental fidelities of local and
telecloning as a function of the reflectivity R
of the asymmetric beam splitter [200]. Polar-
ization angle of polarizers P
1
,P
3
,andP
4
are
0
ı
(a), C45
ı
(b), and circularly polarized with
a quarter wave plate (c). Theoretical curves
are calculated by Eqs. (4.9) and (4.10).
4.1 Qubit Quantum Teleportation 187
Bell State
Measurement
EPR-source II
EPR-source I
12 34
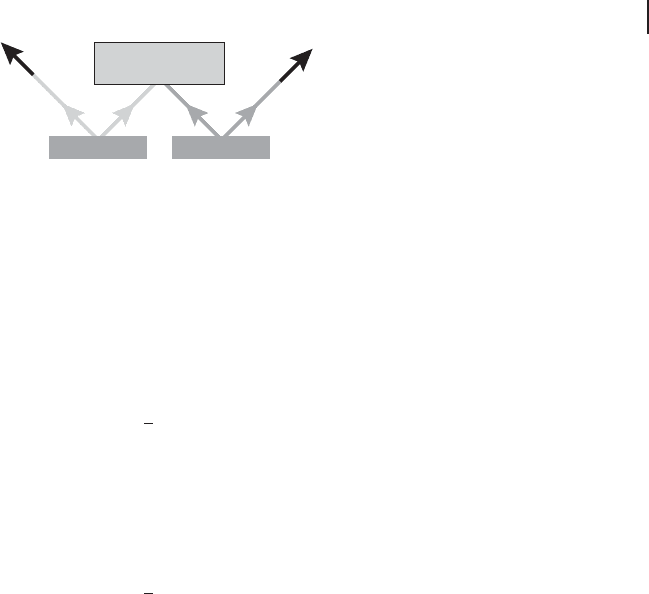
Figure 4.7 Principle of entanglement swap-
ping [202]. Two EPR sources produce two
pairs of entangled photons, pair 1–2 and pair
3–4. One photon from each pair (photons 2
and 3) is subjected to a Bell measurement.
This results in projecting the other two outgo-
ing photons 1 and 4 onto an entangled state.
Figure 4.7 shows the principle of entanglement swapping [202]. First, two EPR
sources each simultaneously emit a pair of entangled photons, pair 1–2 and pair
3–4. The overall state of photons 1–4 jΨ i
1234
is represented as follows:
jΨ i
1234
D
ˇ
ˇ
Ψ
()
˛
12
˝
ˇ
ˇ
Ψ
()
˛
34
D
1
2
(
j$i
1
jli
2
jli
1
j$i
2
)
˝
(
j$i
3
jli
4
jli
3
j$i
4
)
, (4.11)
with jΨ
()
i from Eq. (3.19). Next, a Bell measurement is performed on photons
2 and 3. After the Bell measurement, photons 2 and 3 are projected onto one of
the four Bell states jΨ
(˙)
i and jΦ
(˙)
i described in Eq. (3.19), and the overall state
jΨ i
1234
gets “rearranged” to
jΨ i
1234
D
1
2
ˇ
ˇ
ˇ
Ψ
(C)
E
14
ˇ
ˇ
ˇ
Ψ
(C)
E
23
C
ˇ
ˇ
ˇ
Ψ
()
E
14
ˇ
ˇ
ˇ
Ψ
()
E
23
C
ˇ
ˇ
ˇ
Φ
(C)
E
14
ˇ
ˇ
ˇ
Φ
(C)
E
23
C
ˇ
ˇ
ˇ
Φ
()
E
14
ˇ
ˇ
ˇ
Φ
()
E
23
. (4.12)
When we know the result of Bell measurement, the overall state shrinks to one of
the four cases as shown in Eq. (4.12). For example, in the case of jΨ
()
i
23
obtained
in the Bell measurement, the overall state shrinks to jΨ
()
i
14
jΨ
()
i
23
. In this state,
photons 1 and 4 are entangled and photons 2 and 3 are entangled. The situation is
similar for the other results of the Bell measurement, that means photons 1 and 4
are entangled and photons 2 and 3 are entangled in all cases. Thus, entanglements
in 1–2 and 3–4 are swapped, and 1–4 and 2–3 become entangled.
Figure 4.8 shows the experimental setup of entanglement swapping demonstrat-
ed by Pan et al. [202], which is very similar to the one shown in Figure 4.1 [194].
EPR sources are the same ones illustrated in Section 3.1.3.1. The trick for the Bell
measurement is the same as the one in teleportation, which is explained in Sec-
tion 4.1.1. With this trick, one can detect only jΨ
()
i
23
with simultaneous detec-
tion of photons at detectors D
2
and D
3
. Moreover, as the case of the teleportation
experiment by Bouwmeester et al. [194], simultaneous detection of four photons
at detectors D
1
–D
4
is the necessary condition for success of the experiment. To
check the entanglement between photons 1 and 4, correlation measurement was
performed by changing the polarizer angle Θ before detector D
4
in Figure 4.1.
Figure 4.9 shows the experimental results. Counting rates of four-fold coinci-
dence are shown as a function of polarizer angle Θ before detector D
4
.Sincethe
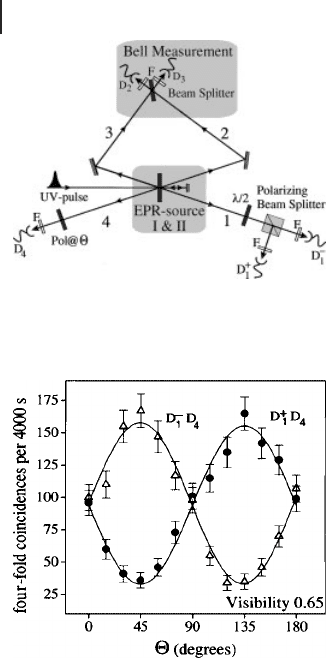
188 4 Quantum Teleportation
Figure 4.8 Experimental setup of entanglement swapping demonstrated by Pan et al. [202].
The half wave plate (λ/2) just before a polarization beam splitter was set at 22.5
ı
.Thepolarizer
angle before detector D
4
was scanned.
Figure 4.9 Results of the correlation measurement to check the entanglement between photons
1and4[202].
angle of the half wave plate before the polarization beam splitter was set at 22.5
ı
,
detector D
C
1
detects a C45
ı
-polarized photon and detector D
1
detects a 45
ı
-
polarized photon. From Figure 4.9, we can clearly see the correlation between
detectors D
C
1
and D
4
and between detectors D
1
and D
4
, which are the proof of
jΨ
()
i-type entanglement between photons 1 and 4. Since four-fold coincidence al-
so guarantees jΨ
()
i
23
, we can conclude that th e overall state is jΨ
()
i
14
jΨ
()
i
23
,
meaning success of entanglement swapping.
4.2
Qumode Qua ntum Teleporta tion
By using an optical field, qumode quantum teleportation has been experimental-
ly realized for G aussian input states – a coherent state [177, 203–205], a squeezed
state [206], and an EPR state, for so-called entanglement swapping [205, 207]. All

4.2 Qumode Quantum Teleportation 189
these experiments are based on the well-developed techniques of optical Gaussian
operations consisting of beam splitter, phase shifting, and squeezing transforma-
tions as well as phase-space displacements and homodyne detection. Those CV
quantum protocols implemented so far operated only with Gaussian states and op-
erations. However, non-Gaussian states or non-Gaussian operations are needed to
potentially achieve universal CV quantum information processing (see Chapters 6
and 7). Quantum teleportation of a non-Gaussian state would become the next im-
portant challenge (see Chapter 8).
In Section 1.6, we presented an idealized version of CV quantum teleportation
using unphysical, infinitely squeezed states. In the following reports on experimen-
tal implementations of CV quantum teleportation, of course, a realistic, physical
description must be employed. For this purpose, it is most convenient to use the
Heisenberg representation for the quadrature operators. Alternative formal isms
for CV quantum teleportation include using the Wigner [163] and the Fock repre-
sentations [208, 209]. These are also both useful to describe quantum teleportation
of non-Gaussian states (see Chapter 8).
Note that unlike the discussion in Section 1.6 on idealized CV quantum telepor-
tation, Alice does gain partial information about the input state through her Bell
measurement on the input state and one half of the finitely squeezed EPR state, and
as a consequence, perfect state transfer is no longer achievable. Criteria in order to
assess the nonclassicality of CV quantum teleportation were derived in [210–212].
4.2.1
Experiment: Qumode Quantum Teleportation
In this section, we first explain the CV teleportation experiment demonstrated by
Furusawa et al. [203] in detail.
Figure 4.10 shows the experimental setup of CV teleportation demonstrated by
Furusawa et al. [203]. First, Alice and Bob share the EPR beams that are created
with the technique explained in Section 3.2.3.1 where two squeezed vacua with
squeezing parameter r are combined at a half beam splitter. The state of the elec-
tromagnetic field to be teleported ( Ox
in
, Op
in
) is created by Victor, which for the exper-
iment here, is more precisely a particular set of modulation sidebands (coherent
state). The beam to be teleported is combined with Alice’s EPR beam ( Ox
A
, Op
A
)by
using a half beam splitter. This process creates states described by the quadrature
amplitudes ( Ox
u
, Op
u
)and(Ox
v
, Op
v
)where
3)
Ox
u
D
1
p
2
Ox
in
1
p
2
Ox
A
, Op
u
D
1
p
2
Op
in
1
p
2
Op
A
,
Ox
v
D
1
p
2
Ox
in
C
1
p
2
Ox
A
, Op
v
D
1
p
2
Op
in
C
1
p
2
Op
A
. (4.13)
Alice measures both quadratures Ox
u
and Op
v
using two homodyne detectors and
obtains the classical results x
u
and p
v
. This measurement corresponds to a CV
3) Compared to Eq. (3.38), the number subscripts for the two EPR modes are replaced by “A” and
“B”, indicating that those beams are in Alice’s and Bob’s possession, respectively.
