Furusawa A., van Loock P. Quantum Teleportation and Entanglement: A Hybrid Approach to Optical Quantum Information Processing

Подождите немного. Документ загружается.

150 3 Entanglement

set of basis states for N qumodes. Note that for two qumodes, N D 2, this basis

is just the CV Bell basis of Eq. (1.95). For the case with N D 3qumodes,v 

C p

D 0, u

 x

 x

D 0, and u

 x

 x

D 0, we obtain the state

jΨ (0, 0, 0)iD

1

dxjxi˝jxi˝jxi . (3.55)

This is the CV version of the three-qubit GHZ state in Eq. (3.6) with stabilizer

generators given by Eq. (3.10). Correspondingly, the CV stabilizer for this state,

expressed in terms of WH operators, is

S D

Z(s) ˝ Z

†

(s) ˝ 1 , X(s) ˝ X(s) ˝ X(s), 1 ˝ Z(s) ˝ Z

†

(s)

. (3.56)

Generalizing the two-mode CV Bell-basis stabilizer derived in Section 1.5.1.2 to a

three-mode CV GHZ-basis stabilizer gives the nonlocal stabilizer generators

2isu

Z(s) ˝ Z

†

(s) ˝ 1 ,e

C2isv

X(s) ˝ X(s) ˝ X(s),

2isu

1 ˝ Z(s) ˝ Z

†

(s)

. (3.57)

Because this constitutes a full set of stabilizer generators for three qumodes (recall

Section 1.9), the eigenvalues v, u

,andu

completely and uniquely determine a

particular GHZ basis state. Instead of the WH stabilizers, again, the qumode states

are more conveniently expressed in terms of x and p linear combinations.

In an N-qumode “CV GHZ-state analyzer”, determining the stabilizer eigenval-

ues v  p

CCp

, u

 x

x

, u

 x

x

,...,andu

N1

 x

N1

x

means projecting onto the CV GHZ basis fjΨ (v, u

, u

,...,u

N1

)ig. Similar to the

two-party two-mode case from the preceding section, these stabilizers correspond

to a set of observables that can be used as a multi-party multi-mode entanglement

witness. Before discussing such a witness in more detail, let us ﬁrst explain how to

actually generate an approximate version of a CV GHZ state using squeezed light

and beam splitters [161].

The simplest way to generate a tripartite entangled state of three qumodes is

to split a single-mode squeezed vacuum state jx  0i (idealized by an x zero-

eigenstate) subsequently at two beam splitters, as shown in Figure 3.14 [161]. In

this case, the inputs of the two unused ports of the beam splitters are vacuum

states. This is practically easy to implement, but when applied to a quantum pro-

tocol, the performance would be o f only limited quality due to the vacuum input

states. For example, in the qumode teleportation network described in Chapter 4,

the maximum ﬁdelity between any pair will be only 1/

2, even in the limit of

inﬁnite squeezing.

H owever, the CV GHZ state in Eq. (3.55) can be generated by sending a

momentum-squeezed vacuum state, jp  0i

, and two position-squeezed vacuum

states, jx  0i

and jx  0i

, in to a “tritter”, consisting of two beam splitters with

transmissivity/reﬂectivity of 1/2 and 1/1, as shown in Figure 3.15. Applying ﬁrst

4) Excluding additional local squeezers [162] by means of which the one-squeezer GHZ-type state

can be locally converted into a three-squeezer GHZ-type state.

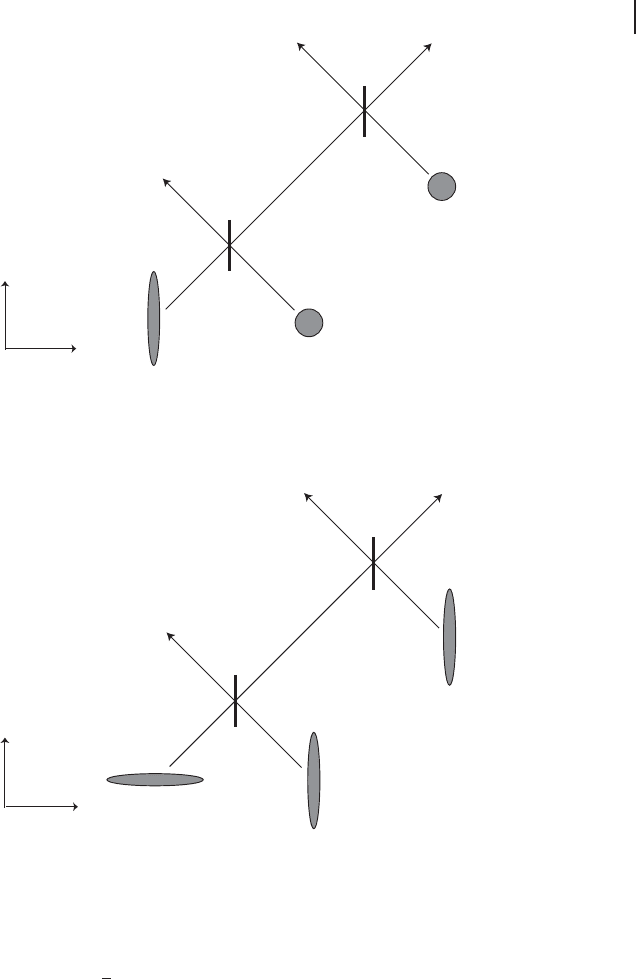
3.2 Qumode Entanglement 151

Figure 3.14 Creation of a CV tripartite en-

tangled state with a single-mode squeezed

vacuum state, jx  0i. The ellipse represents

the squeezed vacuum and the circles corre-

spond to the vacua. The beam splitter labeled



is a 1/2 beam splitter and the other one

is a half (1/1) beam splitter.

Figure 3.15 Creation of a CV GHZ-type state

by combining a momentum-squeezed vacuum

state jp  0i

and two position-squeezed

vacuum states jx  0i

and jx  0i

at two

beam splitters with transmissivity/reﬂectivity

of 1/2 and 1/1. The ellipses represent the

squeezed vacua. The beam splitter labeled by



is a 1/2 beam splitter and the other one is a

half (1/1) beam splitter.

(cos

1

3) and then

(π/4) to the input state jp D 0i

jx D 0i

yields the state in Eq. (3.55), using phase-free beam splitting operators

(θ )

corresponding to the linear transformations in Eq. (2.108). The realistic ﬁnite-

squeezing case will be described in Section 3.2.3.3.

Let u s now see how one can ﬁnd entanglement witnesses for states with three

parties and three qumodes [165]. The goal is to extend the simple two-party two-

152 3 Entanglement

mode entanglement check of the preceding section to a simple test for genuine

three-party three-mode entanglement. The criteria are to be expressed in terms of

variances of quadrature linear combinations for those qumodes involved. Deﬁning

Ou  h

C h

, Ov  g

C g

, (3.58)

a fairly general ansatz is

h(∆ Ou)



Ch(∆ Ov)



 f (h

, h

, g

) , (3.59)

as a potential necessary condition for an at least partially separable state. The posi-

tion and momentum variables Ox

and Op

are the quadratures of the three qumodes.

The h

and g

are arbitrary real parameters. We will prove the following state-

ment(s) for (at least partially) separable states,

O D

O

i,km

˝O

i,n

) f (h

, h

, g

) (3.60)

D (jh

jCjh

C h

j)/2 . (3.61)

Here, O

i,km

˝O

i,n

indicates that the three-party density operator is a mixture of

states i where parties (qumodes) k and m may be entangled or not, but party n is

not entangled with the rest, and where (k, m, n) i s any triple of (1, 2, 3). Hence, also

the fully separable state is included in the above statements. In fact, for the fully

separable state, we have

O D

O

i,1

˝O

i,2

˝O

i,3

) f (h

, h

, g

) (3.62)

D (jh

jCjh

j)/2 , (3.63)

which is always greater or equal than any of the boundaries in Eq. (3.61). For the

proof, let us assume that the relevant state can be written as in Eq. (3.60). For the

combinations in Eq. (3.58), we ﬁnd

(∆ Ou)



(∆ Ov)



˝



hOui



hOvi





C h

C g

C 2



C h





C g



hOui



hOv i



3.2 Qumode Entanglement 153



(∆ Ox

)

C h

(∆ Ox

)

C h

(∆ Ox

)

C g

(∆ Op

)

C g

(∆ Op

)

C g

(∆ Op

)

C 2h

(

hOx

hOx

hOx

)

C 2h

(

hOx

hOx

hOx

)

C 2h

(

hOx

hOx

hOx

)

C 2g

(

hOp

hOp

hOp

)

C2g

(

hOp

hOp

hOp

)

C 2g

(

hOp

hOp

hOp

)



hOui



hOu i

hOvi



hOvi

(3.64)

where hi

represents the expectation value in the state O

i,km

˝O

i,n

.Notethatin

the derivation so far, we have not used the particular form in Eq. (3.60) yet. Exploit-

ing this form of the state, we obtain hOx

DhOx

hOx

, hOx

DhOx

hOx

and similarly for the terms involving p.Becausemodesk and m may be entangled

in the states i, we cannot replace hOx

by hOx

hOx

, and so on. By applying the

Cauchy–Schwarz inequality as in the two-party derivation of [159],

hOu i



(

jhOui

, we see that the last two lines in Eq. (3.64) are bounded below by

zero.Hence,inordertoproveh(∆ Ou)



Ch(∆ Ov)



 (jh

jCjh

C h

j)/2,

it remains to be shown that for any i [recall that the mixture in Eq. (3.60) is a convex

sum with

D 1],

(∆ Ox

)

C h

(∆ Ox

)

C h

(∆ Ox

)

C g

(∆ Op

)

C g

(∆ Op

)

C g

(∆ Op

)

C 2h







C 2g







 (jh

jCjh

C h

j)/2 . (3.65)

By rewriting the left-hand side of Eq. (3.65) in terms of variances only, indeed we

ﬁnd

h(∆ Ox

)

C g

h(∆ Op

)

[

∆

(

C h

)

]



∆

(

C g

)





˝

, g

˛

˝

C h

, g

C g

˛

D (jh

jCjh

C h

j)/2 , (3.66)

using the sum uncertainty relation h(∆

iCh(∆

ijh[

B]ij and [ Ox

, Op

] D

i δ

/2. Hence, the statements in Eq. (3.61) with Eq. (3.60) are proven for all per-

mutations of ( k, m, n) D (1, 2, 3). The inequalities Eq. (3.59) with Eqs. (3.61) and

(3.60) represent necessary conditions for all kinds of (partial) separability in a tri-

partite three-mode state. One may then prove the presence of genuine tripartite

154 3 Entanglement

entanglement through violations of these inequalities, thus ruling out any (partial-

ly) separable form.

A particularly useful example for these conditions is the GHZ-type witness [165]

as expressed by the inequalities in Eq. (3.86), directly related with the CV GHZ

stabilizers discussed above. Since the three-mode GHZ state is determined by three

stabilizer combinations, the witness also only requires three such combinations

and hence it is sufﬁcient (as well as it is necessary) to consider only (at least) two of

the three inequalities in Eq. (3.86).

Besides the above entanglement witnesses, the CV partial transpose criterion

can be utilized to decide on the separability properties of three-party three-mode

Gaussian states [166], provided the correl ation matrix of the state in question is

available. For three-party three-mode Gaussian states, the only partially separable

forms are those with a bipartite splitting of 1  2modes.Inthiscase,theNPT

criterion is necessary and sufﬁcient.

In Section 3.2.3.3, we shall describe an experiment in which tripartite CV entan-

glement was created and veriﬁed. Moreover, in another recent experiment, tripar-

tite Gaussian states with entanglement between three qumodes of three different

frequencies (colors) was demonstrated [167]. In other more recent experiments, as

we will also discuss below, entanglement between more than three parties was pro-

duced and detected in multi-mode CV graph and cluster states. In the next section,

we shall deﬁne and discuss such CV qumode graph states.

3.2.2

Cluster and Graph States

Similar to the operational deﬁnition of qubit graph states in terms of C

-gate edges

pairwise acting upon jCi-state nodes (Section 3.1.2), we may deﬁne CV graph states

on qumodes (see Figure 3.16). For this purpose, we use the CV analogues for the

basis states, stabilizers, and gates as presented in Chapter 1, especially, in Sec-

tions 1.8 and 1.9.

≈p

xxi

ˆˆ

2 ⊗

Figure 3.16 An approximate, Gaussian CV

cluster state built from momentum-squeezed

states of light and Gaussian, CV versions of

the C

gate, e

2iOx˝Ox

.InChapter7,weshall

see that such a cluster state becomes a re-

source for universal quantum computing on

qumodes in the limit of inﬁnite squeezing.

In this case, an arbitrary multi-mode state

jψi attached from the left can be universally

transformed into the output state

Ujψi ap-

pearing on the most right column when all

the remaining qumodes are measured out in

suitable bases.

3.2 Qumode Entanglement 155

Let us start with every qumode representing a single node of the graph in an

inﬁnitely squeezed zero-momentum eigenstate jp D 0i. The initial N-qumode

product state, jp D 0i

˝N

,isstabilizedby

(s)

, with the WH operators X

(s)for

qumode k D 1,...,N and s 2 R. The canonical generation of CV cluster states

in terms of CV C

gates, e

2i Ox˝Ox

, pairwise acting upon the input qumodes in state

jp D 0i, leads to the following evolution of the stabilizer generators,



(s) ˝ 1

N1



†

D X

(s)

l2N(k)

(s) D X

(s)

l¤k

(s)

 K

(s), 8k 2 G , 8s 2 R , (3.67)

where

U describes all the C

gates pairwise acting upon all qumodes of the graph

G according to the adjacency matrix elements A

D A

which are one when the

qumodes become connected and zero otherwise. This set of stabilizer generators

can be rewritten as

(s)

l2N(k)

(s) D e

2is Op

l2N(k)

2is Ox

D e

2is



l2N(k)

. (3.68)

Now, applying the stabilizer conditions to a given graph state jGi,

2is



l2N(k)

jGiDjG i , 8s 2 R , 8k 2 G , (3.69)

immediately leads to the deﬁnition



l2N(k)

! 0, 8k 2 G . (3.70)

Hence, we deﬁne cluster-type (graph-type) states as those multi-mode Gaussian

states for which certain quadrature correlations, as expressed by Eq. (3.70), become

perfect in the limit of inﬁnite squeezing. More precisely, in this limit, the N-mode

graph state becomes a simultaneous zero-eigenstate of the N linear combinations

in Eq. (3.70). We therefore name these combinations nulliﬁers, uniquely deﬁning

the corresponding CV graph state.

This deﬁnition covers all Gaussian states which, in the inﬁnite-squeezing lim-

it, become zero-eigenstates of all those quadrature combinations that generate the

stabilizer group of the corresponding graph. Here, the zero-eigenstates are the rep-

resentatives for a given graph. More generally, one may deﬁne CV graph states

as common eigenstates of the corresponding quadrature combinations. Howev-

er, eigenvalues other than zero correspond to simple phase-space displacements

which have no effect on the entanglement properties of the graph states. This def-

inition then still means that the variance of the quadrature combinations vanishes

in the limit of inﬁnite squeezing, Var[ Op



l2N(k)

] ! 0, 8k 2 G.

156 3 Entanglement

A simple example for a CV graph state is the tripartite GHZ-type state deﬁned

in the preceding section corresponding to a linear three-mode graph up to local

Fourier transforms. The graph stabilizer in this case is

S DhX(s) ˝ Z(s) ˝ 1 , Z(s) ˝ X(s) ˝ Z(s), 1 ˝ Z(s) ˝ X(s)i , (3.71)

differing from that in Eq. (3.56) only by local Fourier rotations on qumodes 1 and 3.

More generally, GHZ-type graph states correspond to star graphs, as illustrated in

Figure 3.1b, differing from standard GHZ-type states only by local Fourier rotations

acting upon all nodes except the central one.

The CV graph states discussed so far are only deﬁned in the unphysical limit of

inﬁnite squeezing as expressed by Eq. (3.70), which we may rewrite as

p  A

x ! 0 . (3.72)

Here,

p  ( Op

, Op

,..., Op

)

and

x  ( Ox

, Ox

,..., Ox

)

are the vectors of position

and momentum operators, and A is the corresponding adjacency matrix of the

graph. Thus, Eq. (3.72) represents the entire set of N stabilizer/nulliﬁer conditions

from Eq. (3.70). Any multi-mode Gaussian state satisfying this set of nulliﬁer rela-

tions in the limit of inﬁnite squeezing belongs to the same class of CV graph states

with matrix A. This matrix is symmetric (so the graph is “undirected”), has all di-

agonal entries zero (so the graph has no “self-loops”), and whenever the graph has

an edge, the corresponding element of A is one, otherwise it is zero.

There is now one straightforward and conceptually distinct generalization of the

notion of CV graph states as deﬁned above. First, we may consider arbitrary real

elements in A instead of only zeros and ones. This gives rise to the notion of weight-

ed CV graph states. In this case, one can think of a network of “weighted” CV C

gates, e

2ig

˝Ox

, again, pairwise acting upon the input qumodes in state jp D 0i

with real-valued “gains” A

 g

2 R for each quadratic interaction. This leads to

a set of more general nulliﬁers which still satisfy Eq. (3.72) in the unphysical limit

of inﬁnite squeezing. Thus, these weighted graph states remain stabilizer states

which are deﬁned through idealized stabilizer conditions. We note that for qubits,

weighted graph states are no longer stabilizer states. While the additional coefﬁ-

cients in the CV nulliﬁer conditions still give quadrature linear combinations, an

extra weight in the qubit C

gates would result in non-stabilizer states.

The most general manifestation of a CV graph state, however, allows for complex-

weighted edges of the graph and correspondingly complex nulliﬁer conditions [168].

In this case, the complex adjacency matrix shall be denoted by Z, this time with

nonzero diagonal entries including self-loops in the graph. We deﬁne the complex

nulliﬁer conditions

p  Z

x D 0 , (3.73)

now representing a set of exact eigenvalue equations satisﬁed by the corresponding

complex-weighted graph state jGi,(

p  Z

x)jGiD0. What are these generalized

graph states? One can show that every N-mode Gaussian pure state can be uniquely

3.2 Qumode Entanglement 157

represented as a graph state with complex adjacency matrix Z,uptophase-space

displacements. In this case, ﬁnite squeezing and phase rotations such as Fourier

transforms are incorporated into Z. In other words, the complex-weighted graph

states are the physical, properly normalized versions of CV graph states.

In Section 1.8, we implicitly used the fact that all Gaussian pure states are stabi-

lizer states in order to show that their manipulation through Gaussian operations

can be efﬁciently classically simulated. For this CV Gottesman–Knill theorem to

hold in the regime of physical states, we needed to keep track of complex eigen-

values of non-Hermitian operators. Similarly, in Section 2.2.8.1, we used complex-

valued stabilizers (nulliﬁers) to uniquely represent arbitrary single-qumode Gaus-

sian pure states. Here, in the context of CV graph states, we may generalize these

results to the multi-mode case (for which we shall omit representing phase-space

displacements).

Let us see how the canonical CV cluster states, operationally deﬁned and cre-

ated by pairwise applying the controlled Z gates, C

(where we deﬁne C

 C

here), are represented when the stabilizers for physical squeezed states are used

from the beginning (see Section 2.2.8.1). The N stabilizers of the initial N momen-

tum-squeezed modes as derived in Eq. (2.84), e

e

2r

(s)Z

(ie

2r

s), k D

1,2,...,N, are then transformed for each interaction with neighbor l as

e

2r

(s)C

†

(ie

2r

s)C

†

D e

e

2r

(s)Z

(ie

2r

s) . (3.74)

Eventually, by collecting all these interactions, we obtain the N new stabilizers

e

2r

(s)Z

(ie

2r

l2N(k)

(s) . (3.75)

In the limit of inﬁnite squeezing r

!1, we get back the ideal CV cluster sta-

bilizers from Eq. (3.68). However, this time, the above stabilizers also do the job

for ﬁnite squeezing and uniquely represent the corresponding approximate cluster

state. The nulliﬁers can be derived from

e

2r

(s)Z

(ie

2r

l2N(k)

(s)

D e

e

2r

2is

(

ie

2r

)

2r

l2N(k)

2is Ox

D e

2is

(

ie

2r



)

8k D 1,2,...,N , 8s 2 R , (3.76)

and become

 ie

2r



l2N(k)

D 0,8k . (3.77)

This result corresponds to the complex nulliﬁer conditions in Eq. (3.73), with a

complex adjacency matrix Z having imaginary diagonal entries ie

2r

and the re-

158 3 Entanglement

maining entries being either zero or one depending on the particular graph state

with unweighted edges.

Now, we also know that any pure N-mode Gaussian state can be built from N

squeezed vacua through passive linear optics (modulo phase-space displacements,

see Chapter 2). In terms of stabilizers, this means that without loss of generality,

the stabilizers of N momentum-squeezed states are transformed as

e

2r

(s)U

†

(ie

2r

s)U

†

D e

2is

(

ie

2r

)

. (3.78)

Here, the Op

and Oq

are the linearly transformed momentum and position op-

erators after the corresponding (inverse) unitary transformation U.Providedthis

U represents a Gaussian (Clifford) transformation, we will always obtain linear

combinations in terms of the generators on the right-hand side of Eq. (3.78). This

would include the canonical C

interactions, as discussed before. However, now

we shall restrict ourselves to only passive, number-preserving unitaries U,without

loss of generality (recall the Bloch–Messiah decomposition discussed in Chapter 2).

The canonical case woul d then requi re that the squeezing parts of the C

gates be

absorbed into the ofﬂine momentum squeezers corresponding to Bloch–Messiah

reduction [169].

For the case of a passive linear transformation, we can write Oa

,and

so Ox

(ReU

 ImU

)and Op

(ImU

 ReU

), with some

unitary N  N matrix U,(U)

 U

.Finally,throughEq.(3.78),wearriveatthe

new stabilizers

2is

[

(

ImU

ie

2r

ReU

)

(

ReU

Cie

2r

ImU

)

]

. (3.79)

For the nulliﬁers, we then obtain

(

p C B

)

jGiD0 , (3.80)

which we may rewrite as



1

p C A

1



jGiD

(

p  Z

)

jGiD0 , (3.81)

with Z A

1

B,(A)

 ReU

C ie

2r

ImU

,and(B)

 ImU



2r

ReU

. Thi s gives us the complex adjacency matrix for an arbitrary pure

Gaussian N-modestate.Wenotethatthereareatmost4N

parameters to deter-

mine the stabilizer/nulliﬁer (see Section 1.8). These, however, are not independent,

as U must be unitary and B follows from A. A general LUBO transformation has

C N free parameters without displacements, which is the same number for

representing a symplectic transformation from Sp(2N, R ). For representing pure

Gaussian N-mode states (modulo displacements), it is enough to apply a general

LUBO transformation to an N-mode vacuum state where after Bloch–Messiah re-

duction, the ﬁrst passive transformation has no effect on the vacuum [169]. Thus,

N real squeezing parameters r

and N

parameters for the remaining passive

transformation U sufﬁce to uniquely determine the matrices A and B, and hence

the state through Z.

3.2 Qumode Entanglement 159

Stabilizer and graph states

Qubits

unweighted graph state  any stabilizer state: (up to local Cliffords)

l2N(k)

jGiDX

l¤k

jGiDjGi , 8k 2 G , A

D 0, 1

¬¬¬¬ Qumodes

real-weighted graph state  any unphysical 1-squeezing stabilizer state: (up

to local Gaussian unitaries)

p  A

x ! 0 ,



l2N(k)

! 0, 8k 2 G

complex-weighted graph state  any Gaussian pure state:

(up to local WH unitaries)

p  Z

x D 0 ,

canonical graph:

 ie

2r



l2N(k)

D 0, 8k 2 G

Even though every Gaussian pure state is effectively a graph state, these would

include trivial graphs such as products of vacuum states with an imaginary, diago-

nal Z matrix only describing self-loops. Note that, for universal quantum informa-

tion processing, not even GHZ-type star graphs would sufﬁce as a resource. Other

nontrivial, highly entangled, two-dimensional CV graphs would be needed.

Before we start describing various experiments in which optical qumode en-

tanglement was generated, including various CV Gaussian graph states, let us

mention that there are currently ﬁve distinct proposals for preparing CV graph

states optically. The canonical generation method [171] is in one-to-one corre-

spondence with the operational deﬁnition of CV cluster states and uses CV C

gates for every link of the cluster. These gates are rather hard to achieve online,

that is, upon non-vacuum states such as the initial squeezed-state cluster nodes.

A second, more practical scheme would shift every squeezer to the very begin-

ning of the cluster generation circuit such that only ofﬂine, single-mode vacuum

squeezing is required and the rest is just interferometry with a suitable network of

beam splitters [169]. This works for arbitrary graph states and hence is the chosen

technique for all those experiments conducted so far, including those described

below.