Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

8.6. Applications: Extensions of the Notion of Properly Posed Cauchy Problem 497

8.6.

APPLICATIONS; EXTENSIONS OF

THE NOTION OF PROPERLY POSED

CAUCHY PROBLEM

There exist abstract differential equations for which the Cauchy problem,

although perfectly natural, does not fit into the mold of properly posed

problems introduced in Chapter 1. We examine below an instance of this

situation.

8.6.1 Example (Beals [1972: 2]).

Consider the following system of partial

differential equations for two functions u(x, t), v(x, t):

Dtu = Dxu +Dxv, Dv=Dv (x E R,t >,0)

(8.6.1)

with initial conditions

u(x,0) = uo(x), v(x,0) = vo(x). (8.6.2)

We can write (8.6.1) as a differential equation

u'(t) = %u (t) (8.6.3)

in the space L2(R)2; in matrix notation the operator 91 is

t=lax

DD:1

(8.6.4)

whose domain D(11) consists of all u = (u, v) E L2(R)2 such that u', v' E

L2(R) (that is, D(A) = H'(R)2 = H'(R)x H'(R)). We analyze (8.6.3) using

Fourier transforms as in the examples in Chapter 1. The operator it_

s?1

' in L2 (R a) 2 is the operator of multiplication by the matrix

ie[0

(8.6.5)

with domain D(i1) consisting of all (0,0) E L2(R a)2 with au, a13 E-= L2(R a).

In view of the isometric character of IF, we may examine

u'(t) _ itit(t)

(8.6.6)

instead of (8.6.3). We have

exp(tit(a))=

(-iat)k

k=o

e-lat

- late

tat

e-'at

(8.6.7)

If u= (u, i3)ED(!12)=((u,t7)EL2(Ra)2, o2u,a21b EL2(Ra)), then fi(t)=

(t)(u, v) = exp(t !t(a))(u, v) is a genuine solution of (8.6.6) in t > 0 (in

fact, in - oo < t < oo), although the operator C (t) is not bounded in

L2(R a)2 if t - 0. However, it is plain that there exists a constant C such that

Ilu(t)II<CO +ItI)(Ilull+Ilifull)

(-oo<t<oo).

(8.6.8)

498

The Cauchy Problem in the Sense of Vector-Valued Distributions

Obviously, the same conclusion holds for the original equation (8.6.3): the

assumption on the initial condition u = (u, v) is that u, v E H2(R).

We take this example as motivation for the following definition,

where additional generality is attempted.

Let A be a densely defined operator in an arbitrary Banach space E.

The Cauchy problem for the equation

u'(t) = Au(t) (8.6.9)

is mildly well posed in t >_ 0 if and only if

(a) The subspace D = D(Ao") = n I ID(A") is dense in E and for

each uo E D there exists a solution'

of (8.6.9) in t >, 0 with

u(0) = ua. (8.6.10)

(b) For every T > 0 there exists an integer p = p(T) and a constant

C = C(T) such that

11u(t)II < C(Ilu(0)II+IIAPu(0)II)

(8.6.11)

for any solution

of (8.6.9) defined in 0 < t< T and such that

u(0) E D(AP).

We note that the first assumption coincides with (a) in the definition

of well posed Cauchy problem in Section 1.2 (except for identification of

D). Note also that (b), unlike its counterpart in Section 1.2 applies to

solutions not necessarily defined in t >_ 0 but only in a finite subinterval

0 < t < T. Obviously, it implies uniqueness of any such solution u(-), at least

in 0 < t < T, if u(0) E D(AP), p the integer corresponding to T. If we assume

that p (A) 0, uniqueness follows in general: if

is an arbitrary solution

of (8.6.9) in 0 < t < T, then v(.) = R (A) P u

is a solution to which unique-

ness applies, and v(0) = 0 if u(0) = 0.

The mildly well posed Cauchy problem in - oo < t < oo is corre-

spondingly defined.

8.6.2

Theorem.

Let A be a densely defined operator with p(A) *®

and assume the Cauchy problem for (8.6.9) is mildly well posed in t >, 0. Then

the Cauchy problem for (D - A) U = F is well posed in the sense of distribu-

tions, that is, there exists S E 6D'((E; D(A))) such that

(8'®I-8®A)*S=8®I, S*(S'®I-S®A)=S®J,

(8.6.12)

where I (resp. J) is the identity operator in E (resp. D(A)). Conversely, let the

Cauchy problem for a closed, densely defined operator A be well posed in the

sense of distributions. Then the Cauchy problem for (8.6.9) is mildly well posed

int>, 0.

7SolUtions

are defined as in Chapter I.

8.6. Applications; Extensions of the Notion of Properly Posed Cauchy Problem 499

Proof.

Assume the Cauchy problem for (8.6.9) is mildly well posed

in t > 0 with p (A) * 0. As in Section 1.2 we define the propagator of

(8.6.9) for u E D by

S(t)u = u(t),

where u(.) is the solution of (8.6.9) with u(0) = u provided by assumption

(a). Unlike in Section 1.2, however, S(t) is in general unbounded and not

defined in all of E.

We begin by observing that if X E p (A), then the norms I I u I I + I I AP u II

and

I I(X I - A) P u I I are equivalent in the space D(AP). Accordingly, it

follows from (b) that IIS(t)uII <CII(XI - A)PuII (0 < t <T, u E D). Hence

S(t) R (X) P is bounded in D, uniformly in 0 < t < T. We note next that

R(X)PS(t)u=S(t)R(X)Pu

(8.6.13)

for 0 < t < T, u e D. To prove this equality we observe that the right-hand

side of (8.6.13) is a solution of (8.6.9) in t >_ 0, and so is R (a)

u (note

that we obviously have (XI - A) PD = D). Since both solutions have the

same initial value, they must coincide everywhere and (8.6.13) results. Using

the uniform boundedness of the right-hand side and the continuity of S(-) u

for u E D, we deduce that R(X)PS(t) has an extension R (X) PS(t ), uni-

formly bounded and strongly continuous in 0 < t < T. We shall use this fact

to show that

(YP*Su)(t)=

1 ft(t-s)P-'S(c)uds (8.6.14)

(p-1)! 0

(in principle defined only for u E D) admits as well a uniformly bounded

and strongly continuous extension (YP * S) (t) in 0 < t < T. To see this we

write equation (8.6.9) for u(s) = S(s)u (u e D), subtract XS(s)u from both

sides and premultiply by R(JR), obtaining

(Y, * Su)(t) = f 1S(s)uds

=x f1R(X)S(s)uds-R(X)S(t)u+R(X)u,

(8.6.15)

which proves our claims about YP * S in the event that p = 1. An obvious

iteration argument takes care of the case p > 1.

The distribution S E 6DD0'((E; X)) promised in the statement of Theo-

rem 8.6.2 is defined as follows: if q) is a test function with support in t < T

and u E E, we set

S(9))u

(-1)P+,f

(YP+, *

S)(t)uq)cP`>(t) dt.

(8.6.16)

-00

500

The Cauchy Problem in the Sense of Vector-Valued Distributions

If u E D, we have

A(Yp+t

* S)(t)u = (Yp+, *

AS)(t)u

= (Yp+t * S')(t)u

= (Yp *

S)(t)u-Yp+i®u.

An approximation argument then shows that

(Yp+l * S)(t)E C D(A)

with

A (Yp+i * S)(t)E = (Y, * S)(t) u -Yp+1®I

so that (8.6.16), used for arbitrary T > 0 (and p depending on T) defines a

distribution in 6D'((E; X)); that the definition is consistent for different

values of T (and p) is a simple matter of integration by parts.

Finally, we verify both equations (8.6.12). To show the first we check

that (S' (DI - S ®A) * Su = 80 u for u E D and extend to u e E by denseness

of D. The second equation reduces in an entirely similar fashion to

S * (8'(9 u - S (&Au) = S ®u for u E D, and this can be shown proving that

AS(t)u = S(t)Au for u E D. This is done arguing as in the proof of (8.6.13)

and we omit the details.

We prove now the converse. Assume that S' ®I - S ® A E

6 o((E; X))-t and let S E 6 o((E; X)) be the solution of (8.6.12). We begin

by checking assumption (a). To show denseness of D, we consider S-as we

may-as a distribution in 61o((E; E)) and define the adjoint distribution

S* E 6D ((E*; E*)) by

S*((p) = S(p)*

(q) E 6D).

(8.6.17)

Since AS(gp) = S(cp)A, it follows that S((p)A is a bounded operator (with

domain D(A)) for each qp E 6D, thus the second equality (4.8) implies that

A*S(pp)* = (S(g))A)* is bounded. Accordingly S(p)* E (E*; D(A*)), D(A*)

endowed with the graph norm. Also, it follows that S(p)* and A*S(q))*

depend continuously on p as (E*; E*)-valued functions, thus S* E

6D ((E*; D(A*)). Taking adjoints in (8.6.12), we obtain the corresponding

adjoint versions:

(6'®I*-S®A*)*S*=S®I*, S**(S'®I*-6®A*)=S®J*,

(8.6.18)

where I* (resp. J*) is the identity operator in E* (resp. in D(A*)). We note,

incidentally, that D(A*) may not be dense in E*.

Let (E be the subspace of E consisting of all elements of the form

S(p)u, 9 E 6D ((0, cc)), u e E. Since AS((p)u = - S(99') u, it is clear that

G 9 D(A°°). Assume (F is not dense in E. Then there exists u* - 0 in E*

8.6. Applications; Extensions of the Notion of Properly Posed Cauchy Problem 501

such that (S*((v)u*, u) = (u*, S(99)u) = 0 for all qi E 6 ((0, oo)), u E E, so

that S*(qq)u* = 0 for all such qq. This means that S*u* = 0 in t > 0; since S*

itself has support in t > 0, it follows that supp S*u* = (0). Applying Corollary

8.1.7, we deduce that

S*u* = S®uo + ... -4' 8(-)(& U*

,,,

where uo,...,u*, E D(A*) (m >_ 0). Replacing this expression in the first

equality (8.6.18), we deduce that

(6'®I*-60A*)*S*u*=8'®u*+

+8(m+1)®u*

- S®A*uo -

6(m)®A*um = 8(&u*.

Equating coefficients we obtain A*uo = - u*, A*u* = uo,...,A*um =

um = 0, which implies that un,_ 1 _ = u* = u* = 0, whence a contradic-

tion results. This proves that Cs, thus D, is dense in E.

To construct the solutions postulated in (a) we use Theorem 8.4.8.

Let A = A (a, $) be the logarithmic region where R (X) exists and satisfies

(8.4.18), r the boundary of A oriented clockwise with respect to A. For

p > m + 1, we define

p-1

up(t)

jEo

j! Ajuo + 2Iri

fA-peatR(X

)Apuo (8.6.19)

in 0 < t < tp = (p - m -2)/a. (See Example 2.5.1). We check that up(-) is a

solution of (8.6.9) in 0 < t < tp with u(0) = uo. To prove (as we must) that

up(t) = u(t) if t < tp when p'> p, it is obviously enough to show that any

solution of (8.6.9) in a finite interval 0 < t < T with u(0) = 0 must

vanish identically. This is done observing that the function satisfies

(S' ®I - S®A) * u(t) = 8(t)®u(0) and convolving both sides on the left by

S; the formula

u(t)=S(t)u(0) (8.6.20)

results.

We shall use (8.6.20) as well to prove (b). Let T > 0, p an integer,

such that T < tp = (p - m -2)/a, u(t) an arbitrary solution of (8.6.9) in

0 < t < T with u(0) E D(AP ), up

(t) the solution provided by (8.6.19). By the

uniqueness result (8.6.20), u(t) = up(t) and the estimate (8.6.11) can be

instantly obtained from (8.6.19). We omit the remaining details.

We note the following particular case of Theorem 8.6.1, which is

proved using essentially the same arguments.

8.6.3

Theorem.

Let A be a closed, densely defined operator such that

8'(& I - S®A E S'((E; X))-'. Then the Cauchy problem for (8.6.9) is mildly

well posed in t > 0. Moreover, for each w'> w there exists a constant C and an

502

The Cauchy Problem in the Sense of Vector-Valued Distributions

integer p such that any solution of (8.6.9) in t > 0 with u(0) E D(AP) satisfies

jju(t)II S Cew"(jIuII+jIAPujI)

(t > 0). (8.6.21)

In the following result another relation between the Cauchy problem in the

sense of distributions and the ordinary Cauchy problem is established. Although the

latter will be properly posed, we need to use here linear topological spaces.

Let A be an arbitrary operator in E such that p (A) - 0. We introduce a

translation invariant metric in D(A°°) as follows:

d(u,v)= Y_ 2-"IIA"u-A"vII(l+jjA"u-A"vII)-1.

n=0

It can be easily shown that existence of R(X) implies that all powers A" are closed,

thus d makes D(A°°) a Frechet space (Dunford-Schwartz [1957: 1]). We note that a

sequence (u",) in D(A°°) converges to u E D(A°°) if and only if

A"u as

m --> oo for n = 0,1,.... Note also that the operator A is continuous in D(A°°): in

fact, d(Au, Av) < 2d(u, v).

96.4 Theorem.

Let A be a closed, densely defined operator in E such that

8'®I - 80A E 6 Do((E; D(A))-'. Then the Cauchy problem for

u'(t)=Au(t) (t>0) (8.6.22)

(A the restriction of A to D(A°°)) is well posed; more precisely, for every u E D(A°°)

there exists a solution of (8.6.22) and solutions depend continuously on their initial data

(equivalently, A generates a uniformly continuous semigroup in D(A°°)). Conversely,

let A be an operator with p (A) *0 and D(A°°) dense in E, and assume the Cauchy

problem for (8.6.22) is well posed with every u E D(A°°) as initial datum of a solution.

Then S'®I - S®A E 6 Do((E; D(A))-'.

This result is little more than a restatement of Theorem 8.6.2. Assume that

8'®I - S®A e °1 o((E; X)), and let S be the solution of (8.6.12). Let u0 E D(A°°)

and let u(t) be the solution of (8.6.9) constructed "by pieces" using formula

(8.6.19). An examination of this formula shows that u(t) E D(A°°) for t > 0;

moreover, given an arbitrary integer q we can take p large enough in (8.6.19) and

show that lim Aj(h-'(u(t + h)- u(t)) exists and Aju'(t) is continuous in 0 < t <T

for j = 0, 1, ... , q. This covers the existence statement (a) in the definition of properly

posed Cauchy problems. The continuous dependence assumption (b) follows essen-

tially as in the proof of Theorem 8.6.2.

We must finally show that the propagator S(t) of (8.6.22) is continuous in

the topology of (D(A°°)) (that is, that S(t)u- u in D(A°°) uniformly for u in

bounded sets of D(A°°)). This is a rather obvious consequence of the equality

S(t)u - u= f `S(s),4uds

and of the fact that a set `3C is bounded in the space D(A°°) when and only when

(A"u; u E `3C) is bounded in n for each n = 0,1,2,...

To prove the converse, let S(t) be the semigroup generated by ,4 in D(A').

It follows from the definition of the topology of D(A°°) that, given T> 0, there

8.6. Applications; Extensions of the Notion of Properly Posed Cauchy Problem

503

exists an integer p and a constant C such that

IIS(t)uII

CII(XI-A)'uII (uED(A°°),0

t<T). (8.6.23)

We check easily that A commutes with S(t); thus if X E p(A), R(X) commutes with

A, and we deduce from (8.6.23) that R(X)PS(t)-a priori only defined in

D(A°°)-possesses an extension R(X)°S(t), uniformly bounded and strongly

continuous in 0 < t < T. After this is established the proof proceeds in essentially

the same way as that of the corresponding portion of Theorem 8.6.2 (see the

comments following (8.6.13)). We omit the details.

8.6.5 Remark. In some situations where A is a differential operator (such

as the one examined in Example 8.6.1) the norm of the space D(AP) is

equivalent, say, to a Sobolev norm involving norms of partial derivatives of

order less than or equal to a multiple of p. In these instances, the mildly

well-posed Cauchy problem is related to the Cauchy problem in the sense of

Hadamard (see Section 1.7 for a thorough discussion). However, the equiva-

lence is not complete in that the use of Banach spaces imposes global rather

than local convergence (see again Section 1.7). In other cases, the norm of

D(AP) may not be equivalent to a Sobolev norm.

We close this section with an example where the framework of mildly

well-posed Cauchy problems proves too restrictive to handle the initial value

problem for a partial differential equation.

8.6.6

Example (Beals (1972: 21).

We consider the following lower order

perturbation of the system (8.6.1):

Dtu=Dxu+DXv, Dtv=DXv+u (xE118,t>0) (8.6.24)

with initial conditions (8.6.2) and write it as an abstract differential equa-

tion

u'(t) = 8u(t) (8.6.25)

in L2(R)2, with

The Fourier analysis of (8.6.25) is

essentially the same as that of (8.6.3): this time the equivalence is with

u'(t) _ f3 u(t),

(8.6.26)

where 8 is the operator of multiplication by

Vi(a)=r-ia

-ial

1 -iaJ

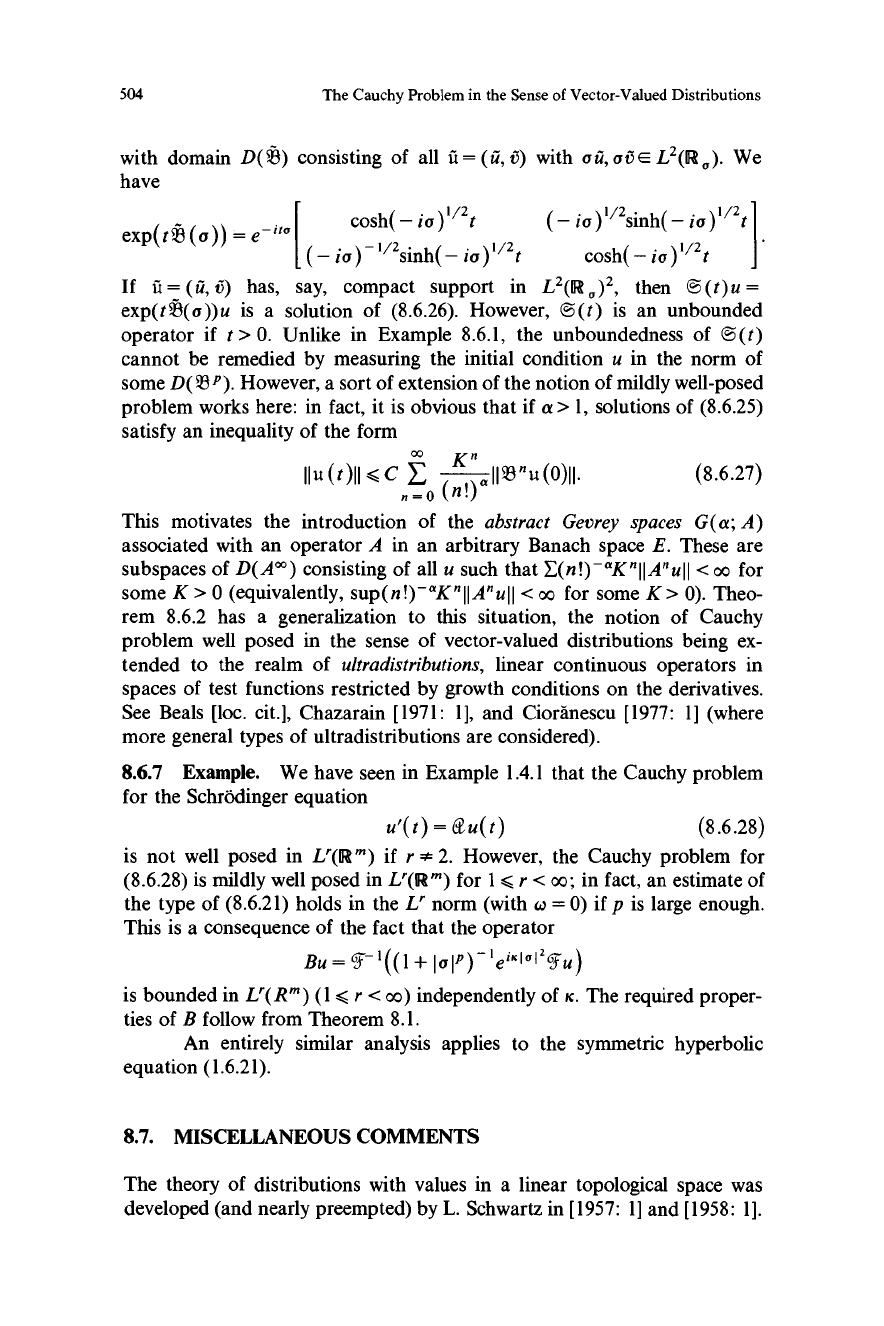
504

The Cauchy Problem in the Sense of Vector-Valued Distributions

with domain D( ) consisting of all u = (u, v) with a u, av E L2 (!!8 (,). We

have

tta

cosh(- w

)1,12

t (- l a )1/2 sinh(- i s

)1,12

exp(t 8(a)) = e

(- i a) -

"2sinh(-

i a)

cosh(- j a )"/12

If u = (u, v)

has, say, compact support in L2(R ")2,

then C5(t)u =

exp(t B(a )) u is a solution of (8.6.26). However, C5 (t) is an unbounded

operator if t > 0. Unlike in Example 8.6.1, the unboundedness of C5 (t)

cannot be remedied by measuring the initial condition u in the norm of

some D(

P). However, a sort of extension of the notion of mildly well-posed

problem works here: in fact, it is obvious that if a > 1, solutions of (8.6.25)

satisfy an inequality of the form

11u (t)II < C

Kn« Ii93 nu (0)11.

(8.6.27)

n=0

(n!)'

This motivates the introduction of the abstract Gevrey spaces G(a; A)

associated with an operator A in an arbitrary Banach space E. These are

subspaces of D(A°°) consisting of all u such that E(n!)-"K"II A"uII < oo for

some K> 0 (equivalently, sup(n!)-°K"IIA"uII <00 for some K> 0). Theo-

rem 8.6.2 has a generalization to this situation, the notion of Cauchy

problem well posed in the sense of vector-valued distributions being ex-

tended to the realm of ultradistributions, linear continuous operators in

spaces of test functions restricted by growth conditions on the derivatives.

See Beals [loc. cit.], Chazarain [1971: 11, and Cioranescu [1977: 11 (where

more general types of ultradistributions are considered).

8.6.7 Example. We have seen in Example 1.4.1 that the Cauchy problem

for the Schrodinger equation

u'(t) _ ¢u(t)

(8.6.28)

is not well posed in L"(R ') if r

2. However, the Cauchy problem for

(8.6.28) is mildly well posed in L"(R"') for I < r < oo; in fact, an estimate of

the type of (8.6.21) holds in the Lr norm (with w = 0) if p is large enough.

This is a consequence of the fact that the operator

Bu =

f-'((1+IaI°)-'e"1.12

is bounded in L'(Rm) (1 < r < oo) independently of K. The required proper-

ties of B follow from Theorem 8.1.

An entirely similar analysis applies to the symmetric hyperbolic

equation (1.6.21).

8.7.

MISCELLANEOUS COMMENTS

The theory of distributions with values in a linear topological space was

developed (and nearly preempted) by L. Schwartz in [1957: 1] and [1958: 1].

8.7. Miscellaneous Comments

505

Almost all the definitions and results here can be found there in enormously

general versions. Theorem 8.3.1 is due to Lions [1960: 2], who also intro-

duced the notion of the Cauchy problem in the sense of distributions for the

equation

U'- AU= F. (8.7.1)

The generalization to the convolution equation (8.4.1) is due to the author

[1976: 1]. In [1960: 2] Lions defines a distribution semigroup as an operator-

valued distribution S E 6D'((O, oo); (E; E)) satisfying the generalized semi-

group equation

S(p*0=S(p)S( )

(8.7.2)

for arbitrary test functions q), E 6D'((O, oo)). This is the analogue of the

second equation (2.3.1); semigroup distributions are thus natural generaliza-

tions of the semigroups in Section 2.5(a), where no specific behavior near

t = 0 is postulated. If certain assumptions regarding that behavior are

introduced the distribution semigroup in question is called regular by Lions

and an infinitesimal generator A can be defined (roughly, as the closure of

lim S(p, ), where

C 6 ((0, oo )) and %, - 8). The distribution S can be

shown to belong to' '((E; D(A)) and to satisfy

S'-AS=8®I, S'-SA=S®J. (8.7.3)

Conversely, any solution of (8.7.3) is a regular distribution semigroup with

infinitesimal generator A, thus establishing a relation between the abstract

differential equation (8.7.1) and distribution semigroups very similar to that

between the equation (2.1.1) and strongly continuous semigroups. The

central problem of the theory is of course, the identification of the genera-

tors A or equivalently of those operators A such that 8'0 I - 8 ®A possesses

a convolution inverse. This was done by Lions [1960: 2] only in the case

where S grows exponentially at infinity. At the same time, Foias [1960: 11

coinsidered semigroup distributions of normal operators in Hilbert space,

identified in general their infinitesimal generators and gave growth condi-

tions on S based on the location of the spectrum of A in subregions of

logarithmic regions. The general case was handled by Chazarain [1968: 11,

[1971: 1], who also extended Lions' theory to the case of ultradistribution

semigroups (where S belongs to a space of vector valued Roumieu-Gevrey

ultradistributions) in [1968: 2], [1971:

1]; the corresponding results for

Beurling ultradistributions and for Sato hyperfunctions are respectively due

to Emami-Rad [1973: 11 and to Ouchi [1971: 1]. Semigroup distributions

that are smooth in t > 0 have been studied by Da Prato-Mosco [1965: 1],

[1965: 2] in the case where S coincides with a vector-valued analytic

function in a sector containing the half axis t > 0 and by Barbu [1968: 2]

[1969: 1] when S is infinitely differentiable in t > 0; we note that his result

generalizes the result of Pazy (Theorem 4.1.3) commented on in Section

4.11. Barbu also gives necessary and sufficient conditions on A in order that

506

The Cauchy Problem in the Sense of Vector-Valued Distributions

S possess different "degrees of smoothness" for t > 0 related to a priori

bounds on its derivatives; in particular, a characterization is obtained for

those A that make S real analytic in t > 0. These theorems are new even in

the semigroup case.

Among other results we mention an "exponential formula" of the

type of the Yosida approximation due to the author [1970: 1] (for additional

material on this score see Cioranescu [1972:

11, who provided as well a

perturbation result in [1973: 2]). The connection between distribution

semigroups and ordinary semigroups in the Frechet space D(A°°) (Theorem

8.6.3) was established by Fujiwara [1966: 1] for distribution semigroups of

exponential growth and by Ujishima [1972: 1] in the general case (see also

Oharu [1973: 1] and Guillement-Lai [1975: 1]). The extension to ultradistri-

bution semigroups is due to Cioranescu [1977: 1]. An earlier and somewhat

different (but essentially equivalent) treatment is due to Beals [1972: 1],

[1972: 2] (see Example 8.6.6). This is an abstract version of a method due to

Ohya [1964: 1] and Leray-Ohya [1964: 1] for the treatment of hyperbolic

systems with multiple characteristics of which those in Examples 8.6.5 and

8.6.6 are particular cases. For additional results on distribution semigroups

and on the equation (8.7.1) in the sense of distributions see Cioranescu

[1974:

11, Emami-Rad [1975:

11, Da Prato [1966: 3], Krabbe [1975: 1],

Ujishima [1969: 1], [1970: 1], Malik [1971: 1], [1972: 1], [1975: 2], Larsson

[1967: 1], Shirasai-Hirata [1964: 1], Mosco [1965: 1], [1967: 1], Yoshinaga

[1963: 1], [1964: 11, [1965: 1], [1971: 1]. Several portions of the theory have

been generalized to distribution semigroups with values operators in a linear

topological space: we mention the results of Vuvunikjan [1971: 3] and

[1972: 11 (see also the reviews in MR) where generation theorems are given

using "resolvent sequences" in the style of T. Komura, Dembart, and

Okikiolu (see Section 2.5(d)); moreover, extensions of the results of Barbu

on smoothness of S can be found as well. See also Vuvunikjan-Ivanov

[1974: 1].

Many of the results above on distribution semigroups do not involve

in any essential way the difference between the equation (8.4.6) and its

generalization (8.4.1); for instance, Chazarain's results on characterization

of generators are in fact stated for a P involving combinations of (possibly

fractional) derivatives. It was shown by Cioranescu [1974: 2] that Chazarain's

theorems extend to an arbitrary P having compact support, both in the

distribution and ultradistribution case; in fact, Cioranescu coinsiders also

the case of several time variables. A somewhat different proof (Theorem

8.4.8) was given by the author [1976: 11, where the restriction that P have

compact support is removed (see also Corollary 8.4.9). Theorem 8.5.1 is a

generalization (due to the author) of Barbu's result mentioned above for

infinitely differentiable distribution semigroups. The rest of Barbu's results

on quasianalytic and analytic classes was also generalized by the author

([1980: 1]). Other results for the equation (8.4.6) (such as the Trotter-Kato