Eschrig H. Topology and Geometry for Physics

Подождите немного. Документ загружается.

In a sense inverse to the above is the following theorem:

Each connected Lie group G has a simply connected covering space



G which is

again a Lie group and the covering p :



G ! G is a Lie group homomorphism the

kernel of which is a discrete subgroup of



Proof It was already seen that G has a simply connected covering space



Choose an arbitrary element of p

1

ðeÞ (where e is the unit of G) to be the unit



e of



G: Let



and



be two paths in



G from



e to arbitrarily chosen points



g and



respectively. Let F

¼ pð



Þ; F

¼ pð



Þ; g ¼ pð



gÞ and h ¼ pð



hÞ: Let F

be the path in G obtained by concatenation of F

and the g-translated image

of F

and let



be a path in



G starting at



e and being projected by p onto F

: Its

end point



k depends only on



g and



h and not on the particular paths chosen. Indeed,

let



g

and



alternatively chosen paths, let F

and F

be their projections, and

let



be a path starting at



e and being projected onto F

¼ gF

: Since

½F

g

; ½F

h

2qðp;



eÞ and ðFF



¼ F



; it follows that F



¼ðgF

ðgF

Þ¼F

g

h

ﬃðF

g

ÞðF

h

Þ; and hence ½F



2qðp;



eÞ with

the consequence that F

and F

; both starting at



e; have the same end point



k: On

this basis, the product



h ¼



k in



G is correctly deﬁned, and by considering cor-

responding paths associativity of this product, unit property of



e and the existence



1

is demonstrated. Furthermore, pð



hÞ¼pð



gÞpð



hÞ was underlying the con-

struction of the product. Hence,



g is a group and the covering p is a group

homomorphism.

It remains to show that the product



1

is smooth in



G: This is straightfor-

wardly demonstrated with the help of paths F

ðtÞ¼FðstÞ smoothly depending on s

in G and using the fact that p is a local diffeomorphism.

Finally, since p is a covering, there is a neighborhood U of e in G the preimage

of which consists of disjoint open sets of



G homeomorphic with U: In particular,

the preimage of e which is the kernel of the homomorphism p is discrete. h

Hence, for every connected Lie group G there exists a simply connected Lie

group



G which is a covering of G: The natural question arises, whether and in

which sense



G is unambiguously determined. It was already demonstrated that

simply connected coverings of G are diffeomorphic as manifolds. That they are

also isomorphic as groups follows from the connection between the Lie groups and

their Lie algebras.

Let G and H be connected Lie groups, and let F : G ! H be a Lie group

homomorphism. Then F is a covering, iff F



: g ! h is a Lie algebra isomorphism.

Proof Suppose that F is a covering. Then F



must be injective. Otherwise F



ðGÞ!T

FðgÞ

ðHÞ would have a non-trivial kernel at every point g: These kernels

form an involutive distribution having an integral manifold (Frobenius theorem)

which is mapped into a point of H by F; and F could not be a local homeomor-

phism. F



must also be surjective, since otherwise F would deﬁne a proper

6.4 Simply Connected Covering Group 187

submanifold of H: Being an injective and surjective homomorphism, F



is a Lie

algebra isomorphism.

Suppose now that F



is a Lie algebra isomorphism. Then, by the inverse function

theorem, p. 74 f, F is everywhere a local diffeomorphism, and, since FðeÞ¼

e; FðGÞ contains a neighborhood of the unit e 2 H and hence, by (6.8), FðGÞ¼H:

It remains to show that a neighborhood of every point of H is evenly covered by G:

Observe, since F is a local homeomorphism, that F

1

ðeÞ¼Ker F ¼ K is a discrete

normal subgroup of G: Therefore, there exists a small enough neighborhood U of

e 2 G such that ðU

1

UÞ\K ¼feg: Using the continuous group operations it is not

difﬁcult to show that FðUÞ is a neighborhood of e 2 H evenly covered by F: This

even cover may be translated to every point of H. h

Let G and H be Lie groups, and let G be simply connected. Let

F : g ! h be a

homomorphism. Then there exists a unique homomorphism F : G ! H such that



F: In particular, if simply connected Lie groups have isomorphic Lie alge-

bras, then they are isomorphic.

Uniqueness was proved at the end of Sect. 6.2. The proof of existence which

uses considerations similar to those of Sect. 6.3 is skipped, see for instance

[1, p. 101].

Now, for any Lie group G the Lie algebra g is isomorphic to the tangent space

ðGÞ (p. 175). If G and H are diffeomorphic (as manifolds) Lie groups and g and

h are elements mapped to each other by the diffeomorphism, then the tangent

spaces T

ðGÞ and T

ðHÞ are isomorphic (inverse function theorem, p. 74 f).

Hence, from the above it follows that connected Lie groups have (up to isomor-

phism) uniquely deﬁned simply connected covering groups, which are called

universal covering groups. Just to mention an important simple example from

physics: the group of transformations of spinors SUð2Þ is the universal covering

group of the group of rotations in 3-space SOð3Þ: (See Sect. 6.6 for details.)

6.5 The Exponential Mapping

Recall the formal Taylor expansion of a real function f of a real variable, analytic

in a neighborhood of x:

f ðx þtÞ¼e

f ðxÞ¼f ðxÞþt

ðxÞþ

ðxÞþ ð6:9Þ

The real line G

¼ R is a simple case of a simply connected Lie group with respect

to addition as group operation. Its Lie algebra consists of the one-dimensional

vector ﬁelds tðd=dxÞ ; t 2 R; that is g

¼ R:

Consider any Lie group G and its Lie algebra g: Let X 2 g be a left invariant

vector ﬁeld on G; then exp



ðXÞ : g

¼ R ! g : t 7!tX is a Lie algebra homo-

morphism. (The notation exp will become evident soon.) According to the end of

last section there is a unique Lie group homomorphism expðXÞ : G

¼ R ! G :

188 6 Lie Groups

t 7!expðXÞ

for every X 2 g from R onto a 1-parameter subgroup of G: On the

other hand, the tangent vector ﬁelds ðd=dxÞ and X are expðXÞ-related (see p. 78)

and hence, according to the Frobenius theorem, there is a unique integral curve of

X in the manifold G for which expð XÞ

¼ e; the latter since expðXÞ is a Lie group

homomorphism. Moreover, since X is left invariant, there are unique integral

curves of X for which l

 expðXÞ

¼ g for every g 2 G; in particular for every

g 2 expðXÞ

¼fexpðXÞ

jt 2 Rg: Thus, the 1-parameter subgroup expðXÞ

consists of the integral curve of X through e 2 G; and the left invariant tangent

vector ﬁelds on a Lie group are always complete. (See p. 81.)

Note that this is a global statement. Locally, one could introduce a local

coordinate system in G like (3.33) and argue with (6.9). Now, with the help of left

translations one easily ﬁnds globally, that is for all t; t

; t

2 R;

expðtXÞ¼expðtXÞ

¼ expðXÞ

;

expððt

þ t

ÞXÞ¼expðt

XÞexpðt

XÞ;

expðtXÞ¼ðexpðtXÞÞ

1

ð6:10Þ

So far, expðXÞ for every ﬁxed X 2 g was a mapping t 7!expðXÞ

from R to G:

With the relations (6.10) one may put expðXÞ

¼ expðXÞ and consider exp as a

mapping from g to G:

As a mapping from g to G; exp maps a sufﬁciently small neighborhood u of the

origin of g homeomorphic to a neighborhood U of e in G; and, according to (6.8)

as a whole is obtained by all kinds of products of factors out of U: However, the

mapping exp : g ! G

need not be onto (compare the exercise on p. 200) nor need

it be one–one (compare the mapping exp : R ¼ s

! S

: t 7!e

i2pt

). It can be

shown that exp is a smooth map. Moreover, it can be shown for every Lie group

that there exists a unique complete analytic atlas (that is, all transition functions

between charts are analytic) so that the group operations and the mapping exp are

analytic. (See for instance [2].)

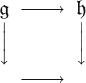
With the help of the exponential mapping the interrelation between a Lie group

and its Lie algebra can further be explored. If F : G ! H is a Lie group homo-

morphism, then the following diagram is commutative:

exp exp

ð6:11Þ

Indeed,

FðexpðXÞÞ ¼ expðF



ðXÞÞ: ð6:12Þ

Since F is a homomorphism, FðexpðXÞ

Þ is a 1-parameter subgroup of H: On the

other hand, expðF



ðXÞÞ

is an integral curve of F



ðXÞ in H; and both are uniquely

6.5 The Exponential Mapping 189

deﬁned by F



ðXÞ at e 2 H: Left translation from e to g 2 H and left invariance of



ðXÞ prove their equality.

If, in particular, FðGÞ is a Lie subgroup of H and X 2 F



ðgÞ; then expðtXÞ2

FðGÞ for all t 2 R: If expðtXÞ2FðGÞ for some interval of values t; then

expðt

XÞ¼FðgÞ for an inner value t

of that interval and some g 2 G; and

expððt  t

ÞXÞ¼expðtXÞðFðgÞÞ

1

2 FðGÞ is a local 1-parameter group through e

in FðGÞ; hence ðt  t

ÞX 2 F



ðgÞ implying X 2 F



ðgÞ:

The following basic results can be proved with the help of the exponential

mapping [1]:

Let H be a Lie group, and let G be an algebraic subgroup of H closed in the

topology of H: Then there is a unique complete atlas of G making it into a Lie

subgroup of H:

Let F : G ! H be a Lie group homomorphism, and let K ¼ Ker F; k ¼ Ker F



Then K is a closed Lie subgroup of G with Lie algebra k:

6.6 The General Linear Group Gl(n,K)

A most important case of a Lie algebra is formed by the n

-dimensional real vector

space of all n n-matrices A; B; ... with the multiplication (commutator)

½A; B¼AB BA ð6:13Þ

where AB is the ordinary matrix multiplication. This Lie algebra is called the

general linear algebra glð n; RÞ:

A base X

ðijÞ

; i; j ¼ 1; ...; n may be introduced consisting of matrices X

ðijÞ

having

unity as matrix element of the ith row and jth column and zeros at all other entries.

Then, obviously

½X

ðijÞ

; X

ðklÞ

¼d

ðilÞ

 d

ðkjÞ

; ð6:14Þ

and comparison with (6.1) yields the structure constants

ðpqÞ

ðijÞðklÞ

¼ d

 d

: ð6:15Þ

Any matrix A can be expanded in this base as A ¼

i;j¼1

ðijÞ

where the vector

components of A in the vector space glðn; RÞ are the ordinary matrix elements A

row i and column j: As a topological vector space, glðn; RÞ is homeomorphic to

The complex general linear algebra glðn; CÞ is obtained just by replacing the

real components A

with complex ones. Hence, it has the same base and structure

constants as glðn; RÞ; but is homeomorphic to C

R

: The following consid-

eration is the same for both cases.

190 6 Lie Groups

Let jAj¼max

j; then it is easily seen that jA

jn

m1

jAj

holds for the mth

power of A: Denote the n  n unit matrix by 1 and consider the series

expðAÞ¼1 þA þ

þþ

þ¼

m¼0

: ð6:16Þ

Its partial sums are n  n-matrices, and for all A with jAj\c it converges uni-

formly for any ﬁxed positive constant c; since the absolute value of each matrix

element of the mth item is bounded by n

m1

=m! and

ðncÞ

=m! ¼ e

con-

verges. Since the matrix multiplication is continuous in glðn; KÞ; K ¼ R or C;

m¼0

ð6:17Þ

and accordingly for the right multiplication, and hence

1

¼ e

ðBAB

1

ð6:18Þ

for any B 2 glðn; KÞ: Now, for any matrix A there is a matrix B such that BAB

1

upper-triangular, and the product of two upper-triangular matrices is again an

upper-triangular matrix. Hence, the right hand side of (6.18) for such a B is an

upper-triangular matrix, and, if a

; ...; a

are the diagonal elements of BAB

1

; then

; ...; e

are the diagonal elements of that right hand side. In particular, no

matter what the numbers a

are,

det e

¼ detðBe

1

Þ¼det e

ðBAB

1

i¼1

¼ e

trðBAB

1

¼ e

tr A

6¼ 0: ð6:19Þ

Here, the simple matrix rules detðABÞ¼det A det B and trðABCÞ¼trðCABÞ were

used.

At the beginning of this chapter it was already mentioned that the general

linear group

Glðn; KÞ¼fA jdet A 6¼ 0gð6:20Þ

is a Lie group. Consider for any matrix A

the 1-parameter subgroup t 7!e

; t 2

R: Its tangent vector at t ¼ 0; that is, for e

¼ 1; is A

: It is simply obtained by

term wise differentiating the uniformly converging power series for e

with

respect to t: This proves

expðA

Þ¼e

; e

glðn;KÞ

generates ðGlðn; KÞÞ

: ð6:21Þ

The general linear algebra is the Lie algebra of the general linear group, the

exponential mapping coincides with the ordinary matrix exponentiation and yields

the component containing the unity of the general linear group. Glðn; KÞ is

pathwise connected for K ¼ C: It is not difﬁcult to see that a path from any

6.6 The General Linear Group Gl(n, K) 191

non-singular matrix A

to any other non-singular matrix A

in C

can always be

inﬁnitesimally deformed into a path avoiding det A ¼ 0 (for instance encircling

det A ¼ 0 in the complex plane always in the positive sense). This does not hold

for K ¼ R for which there are two components with det A ? 0: Glðn; CÞ is not

simply connected, which is most easily seen for Glð1; CÞ¼C n 0: However,

ðGlðn; RÞÞ

is simply connected: Let A

and A

be two arbitrary matrices both with

positive (negative) determinant. There are paths B

ðtÞ; B

ðtÞ; t 2½0; 1 ; B

ð0Þ¼

1; det B

ðtÞ¼1 which continuously transform B

ðtÞA

1

ðtÞ into upper triangular

matrices without changing the determinant. Having determinants of the same sign,

the signs of diagonal elements of both triangular matrices can only differ in an

even number of them. Group neighboring diagonal elements not having the same

signs into pairs and consider a ð2  2Þ-matrix. Put a

ðtÞ¼ta

; a

ðtÞa

ðtÞ¼

ðt

 1Þa

; a

ð1Þ¼a

ð1Þ¼0 and let t run from 1 to 1: It reverses the

signs of diagonal elements without changing the determinant on a path from upper

triangular form to upper triangular form. It does also not change the determinant of

the full matrix which is up to the considered ð2 2Þ-block upper triangular. (This

can directly be inferred from the Laplace expansion with respect to the two rows

containing the ð2 2Þ-block.) In this way successively all diagonal elements of

ð1ÞA

1

ð1Þ differing in sign from those of B

ð1ÞA

1

ð1Þ can be moved

together and then sign reverted. A further continuous path brings the absolute

values of the diagonal elements into coincidence without changing signs. Con-

catenation of all changes completes the path from A

to A

in Glðn; RÞ: Since any

path from det A [ 0 to det A\0 must unavoidably cross det A ¼ 0; this proves that

the polynomial condition det A ¼ 0 deﬁnes a smooth hypersurface in R

dividing

it into just two connected components. Consider any loop in the component with

det A [ 0; and take the point on it with minimal det A as base point of the loop.

(The minimum exists since a loop is compact.) Transform every point of the loop

by the above transformation into the base point. This transformation may be

chosen as a continuous function of the points of the loop and keeps det A above the

value at the base point, contracting the loop into the base point. Hence, ðGlðn; RÞÞ

is simply connected.

Any n n-matrix may be considered as a linear mapping of the n-dimensional

vector space K

over the ﬁeld K into itself (endomorphisms Endð K

Þ). Likewise, a

non-singular matrix may be considered as the transformation matrix of an auto-

morphism of K

: Hence, one has also

exp : EndðK

Þ!AutðK

Þ: ð6:22Þ

Again, this mapping need not be surjective, for instance, if AutðK

Þ is not con-

nected. If G is any Lie group and F : G ! AutðK

Þ is a representation of G; and if

X 2 g; then it follows immediately from (6.11) that

Fðexp XÞ¼expðF



ðXÞÞ ¼ 1 þ F



ðXÞþ



ðXÞ

þ ð6:23Þ

where F



ðXÞ2glðn; KÞ is a matrix obtained from ½dFðexpðtXÞÞ=dt

t¼0

192 6 Lie Groups

It has been shown (theorem by Ado) that every ﬁnite-dimensional Lie algebra

is isomorphic to a subalgebra of glðn; RÞ for some n: (That means that also

glðn

; CÞ is isomorphic to such a subalgebra, of course with n [ n

:) By expo-

nentiation, every subalgebra of glðn; RÞ generates a connected Lie group to which

this subalgebra is the corresponding Lie algebra. Every such Lie group has a

uniquely (up to Lie group isomorphism) determined simply connected covering

group. This provides a one–one correspondence between Lie subalgebras of

glðn; RÞ and simply connected Lie groups.

(A complete classiﬁcation of all Lie algebras has not yet been achieved, to say

nothing about a complete classiﬁcation of all Lie groups.)

Some important Lie subgroups of Glðn; KÞ are shortly considered:

The special linear group

Slðn; KÞ¼fA jdet A ¼ 1g; n [ 1; ð6:24Þ

is a closed connected Lie subgroup of Glðn; KÞ and has its Lie algebra

slðn; KÞ¼fA

jtr A

¼ 0g : ð6:25Þ

Indeed, if tr A

¼ 0; then det expðA

Þ¼1 follows directly from (6.19). Conversely,

det expðA

Þ¼1 implies tr A

¼ð2piÞk with some integer k; and only in the case

k ¼ 0 the A

may form a vector space over the ﬁeld K: This trace condition reduces

the number of independent diagonal elements of A by one, hence the dimension of

slðn; KÞ and of Slðn; KÞ is equal to n

 1; in the case of complex algebra for

K ¼ C; and slðn; CÞ and Slðn; CÞ have the dimension 2n

 2 in real algebra.

Slðn; KÞ is closed since det A ¼ 1 is a polynomial equation in K

: Let A

and A

two arbitrary elements of Slðn; CÞ: Since Glðn; CÞ is connected, there is a path BðtÞ

in Glð n; CÞ connecting A

¼ Bð0Þ with A

¼ Bð1Þ: For every t there is a path

Dðu; tÞ BðtÞ; where Dðu; tÞ¼kðu; tÞ1 and kðu; tÞ is a continuous non-zero complex

scalar function with kð0; tÞ¼1; kð1; tÞ¼ðdet BðtÞÞ

1

(It is continuous in both

variables u and t and may for instance be chosen always to go around the origin of

the complex plane in the positive sense). Now, Dðu; tÞBðtÞ continuously deforms

the path BðtÞ into a path AðtÞ2Glðn; CÞ (in the relative topology from C

) from

to A

; where now AðtÞ is in Slðn; CÞ: Hence, Slðn; CÞ is connected. In fact it is

even simply connected. An analogous argument shows that Slðn; RÞ¼Slðn; CÞ\

Glðn; RÞ is a connected subspace of ðGlðn; RÞÞ

: (Slð1; KÞ¼f1g is trivial.)

The unitary group

UðnÞ¼fA jA

¼ A

1

gð6:26Þ

is a connected compact (closed bounded) Lie subgroup of Glðn; CÞ: A

means the

Hermitian conjugate of the matrix A: Indeed, from 1 ¼ðAA

it fol-

lows that jA

j1; and hence UðnÞ is bounded in C

: It is closed since the

condition A

¼ A

1

implies det A 6¼ 0; and hence this former condition can be

expressed as a set of polynomial equations in the matrix elements. One further has

6.6 The General Linear Group Gl(n, K) 193

expðA

Þ¼1 þ A

þ¼ð1 þA

þÞ

¼ðexpðA

ÞÞ

¼ðexpð A

ÞÞ

1

¼ expðA

from which chain it is seen that the commutator algebra of skew-Hermitian

matrices

uðnÞ¼fA

þ A

¼ 0gð6:27Þ

is the Lie algebra of UðnÞ: Since the matrices A

2 uðnÞ necessarily have a van-

ishing real part of the diagonal matrix elements, it is an algebra over K ¼ R:

Although the matrices themselves may be complex, UðnÞ is a real Lie group and

uðnÞ is a real Lie algebra, both with real dimension n

: Uð1Þ is the unit circle in the

complex plane (which is not simply connected). The angle / may be taken as its

real coordinate.

Let D

p;q

be the diagonal matrix with the ﬁrst p diagonal entries equal to 1 and

the last q diagonal entries equal to 1; p; q 1; p þq ¼ n: The generalized

unitary group

Uðp; qÞ¼fA jD

p;q

¼ A

1

gð6:28Þ

is a real subgroup of Glðn; CÞ with the real Lie algebra

uðp; qÞ¼fA

p;q

þ A

¼ 0g: ð6:29Þ

Uðp; qÞ is not compact as the example

cosh h sinh h

sinh h cosh h



2 Uð1; 1Þ; h 2 R ð6:30Þ

shows. The real dimension is again n

The orthogonal group

Oðn; KÞ¼fA jA

¼ A

1

gð6:31Þ

is a closed Lie subgroup of Glðn; KÞ: Here, A

means the transposed of the matrix

A: For K ¼ R; it is compact by the same argument as in the unitary case. However,

since 1 ¼ detðAA

1

Þ¼det Adet A

¼ðdet AÞ

; it consists of the two components

with det A ¼1 and is not connected. A chain of equations analogous to the

unitary case shows that the commutator algebra of skew-symmetric matrices

oðn; KÞ¼fA

þ A

¼ 0gð6:32Þ

is the Lie algebra of Oðn; KÞ: Since all matrices of oðn; CÞ have zero diagonal

elements, complex coefﬁcients will not violate the skew-symmetry condition;

oðn; CÞ is a complex Lie algebra and Oðn; CÞ a complex Lie group, both with

complex dimension nðn 1Þ=2 because of the vanishing diagonal of A

2 oðn; CÞ

194 6 Lie Groups

and the skew-symmetry of the off-diagonal elements. The corresponding real

dimension is nðn  1Þ: Oðn; RÞ¼OðnÞ¼UðnÞ\Glðn; RÞ and oðn; RÞ¼oðnÞ¼

uðnÞ\glðn; RÞ consist of real matrices and are of real dimension nðn 1Þ=2:

(Oð1; KÞ¼Oð1Þ is discrete and consists of the two elements 1 and 1; hence its

Lie algebra is trivial, oð1Þ¼f0g:)

The generalized orthogonal group (again p; q 1; p þ q ¼ n)

ðp; qÞ¼fA jD

p;q

¼ A

1

gð6:33Þ

is a non-compact non-connected Lie subgroup of Glðn; RÞ with the Lie algebra

oðp; qÞ¼fA

p;q

þ A

¼ 0g: ð6:34Þ

It is not difﬁcult to see that Oðp; qÞ has the four components ðOðp; qÞ

ðp; qÞ; 1O

ðp; qÞ; D

p;q

ðp; qÞ and D

p;q

ðp; qÞÞ: The matrix (6.30)is

obviously also an element of Oð1; 1Þ: The real dimension of Oðp; qÞ is again

nðn 1Þ=2:

The special unitary group

SUðnÞ¼UðnÞ\Slðn; CÞ¼fA jA

¼ A

1

; det A ¼ 1g; n [ 1; ð6:35Þ

is a simply connected compact real Lie subgroup of both UðnÞ and Slðn; CÞ with

the Lie algebra

suðnÞ¼uðnÞ\slðn; CÞ¼fA

þ A

¼ 0; tr A

¼ 0g : ð6:36Þ

Its (real) dimension is n

 1:

The generalized special unitary group

SUðp; qÞ¼Uð p; qÞ\SlðnCÞ; p; q 1; p þq ¼ n; ð6:37Þ

has also dimension n

 1; but is not compact. Again, (6.30) is also an element of

SUð1; 1Þ:

The special orthogonal group

SOðn; KÞ¼Oðn; KÞ\Slðn; KÞ¼fA jA

¼ A

1

; det A ¼ 1g; n [ 1; ð 6:38Þ

is a connected Lie subgroup of both Oðn; KÞ and Slðn; KÞ with the Lie algebra

soðn; KÞ¼oðn; KÞ; ð6:39Þ

since the skew-symmetry implies a vanishing diagonal and hence tracelessness.

SOðn; CÞis not compact and has complex dimension nðn 1Þ=2 and real dimension

nðn 1Þ: SOðn; RÞ¼SOðnÞ is compact and has real dimension nðn 1Þ=2:

The generalized special orthogonal group

SOðp; qÞ¼O

ðp; qÞ\Slðn; RÞ; p; q 1; p þ q ¼ n; ð6:40Þ

has also real dimension nðn 1Þ=2: It is again not compact, and (6.30) is also an

element of SOð1; 1Þ:

6.6 The General Linear Group Gl(n, K) 195

Finally, let

0 1

1



ð6:41Þ

be the matrix which replaces the ﬁrst n coordinates of K

with the second n

coordinates and the second ones with the negative ﬁrst ones. (For R

it just rotates

by p=2:) The symplectic group

Spð2nÞ¼fA jJ

¼ A

1

; A

¼ A

1

g; n [ 1; ð6:42Þ

is a simply connected compact real Lie subgroup of Uð2nÞ with the Lie algebra

spð2nÞ¼fA

þ A

¼ 0; A

þ A

¼ 0g: ð6:43Þ

Like UðnÞ it is a real group and algebra, although the elements are complex. It is a

simple exercise to see that its (real) dimension is nð2n þ1Þ: The elements A

of the

algebra spð2nÞ have two skew-Hermitian n n diagonal blocks being the negative

transposed of each other and two symmetric n n off-diagonal blocks being the

skew-Hermitian conjugate of each other. Spð2Þ¼SUð2Þ:

The symplectic K-group

Spð2n; KÞ¼fA jJ

¼ A

1

g; n [ 1; ð6:44Þ

is a connected (but non-compact and not simply connected) Lie subgroup of

Glð2n; KÞ with the Lie algebra

spð2n; KÞ¼fA

þ A

¼ 0g : ð6:45Þ

The K-matrices A

consist of two n n diagonal blocks being the negative

transposed of each other and two independent symmetric off-diagonal n  n

blocks. The K-groups and K-algebras have the K-dimension nð2n þ 1Þ:

Spð2; KÞ¼Slð2; KÞ:

The Lie groups SUðnÞ; n 2; Spð2nÞ; n 2; SOðnÞ; n 7; are compact simply

connected and as such universal covering groups corresponding to their respective

Lie algebras. If the Lie algebra g of a Lie group G is isomorphic to one of the

algebras suðnÞ; spð2nÞ; oðnÞ with integers n from above, then the corresponding

group SUðnÞ; Spð2n

Þ or SOðnÞ; respectively, is the universal covering group of G:

(soð3Þspð2Þsuð2Þ; soð 4Þsuð2Þsuð2Þ; soð5Þspð4Þ; soð6Þsuð4Þ:)

All compact, simply connected, simple Lie groups (Lie groups having simple Lie

algebras, see Compendium C.4) were classiﬁed by W. Killing and H. Cartan

(which leads in addition to the just mentioned classical groups to the ﬁve so-called

exceptional groups E

; E

; F

; G

; see again Compendium C.4).

The relevance of the general linear group and its subgroups lies in the fact

that they may be understood as transformation groups of an n-dimensional vec-

tor pace K

over the ﬁeld K: If x 2 K

with coordinates x

; i ¼ 1; ...; n; then

Ax 2 K

is the transformed vector with coordinates A

: The composition of two

196 6 Lie Groups