Emmrich E., Wittbold P. (editors) Analytical and Numerical Aspects of Partial Differential Equations: Notes of a Lecture Series
Подождите немного. Документ загружается.


Atomistic to continuum coupling methods 2
15
Lemma 4.14. Le
t g ∈ W
1,1
(0, L) with L = N h. Then
Z
L
0
g(x) dx −h
N
X
i=1
g(ih)
≤ hkg
′
k
L
1
(0,L)
.
Let ψ ∈ X
0h
c
. Recall that Ω
M
= (P h, L) with L = Nh. Then
B
c
(ψ) − B
µ
(Eψ) =
Z
Nh
P h
f(x) ψ(x) dx − h
N
X
i=P +1
f(ih) ψ(ih).
We want to use Lemma 4.14 with g = fψ. In view of assumption (H4), we have
f ∈ C
0
Ω
M
.
Since ψ ∈ X
0h
c
, we have ψ ∈ C
0
Ω
M
a
nd ψ
′
∈ L
1
(Ω
M
).
Let us now make the additional assumption that the body force f satisfies f
′
∈
L
1
(Ω
M
). Then
|B
c
(ψ) − B
µ
(Eψ)| ≤ hk(f ψ)
′
k
L
1
(Ω
M
)
≤ h
kf
′
ψk
L
1
(Ω
M
)
+ kfψ
′
k
L
1
(Ω
M
)
≤ h
kf
′
k
L
1
(Ω
M
)
kψk
L
∞
(Ω
M
)
+ kfk
L
∞
(Ω
M
)
kψ
′
k
L
1
(Ω
M
)
.
We work with ψ ∈ X
0h
c
, so that ψ(L) = 0. Therefore, we have kψk
L
∞
(Ω
M
)
≤
kψ
′
k
L
1
(Ω
M
)
≤
√
L kψ
′
k
L
2
(Ω
M
)
≤
√
L |ψ|
H
1
(X
c
)
,
and find
|B
c
(ψ) − B
µ
(Eψ)| ≤ h
√
L
kf
′
k
L
1
(Ω
M
)
+ kfk
L
∞
(Ω
M
)
|ψ|
H
1
(X
c
)
.
I
n view of the definition (4.37) of τ, this yields
τ ≤ h
√
L
kf
′
k
L
1
(Ω
M
)
+ kfk
L
∞
(Ω
M
)
. (
4.38)
We thus obtain the following result.
Theorem 4.15. Assume that (H3) and (H4) hold, and that the partition Ω = Ω
M
∪Ω
µ
is such that f
′
∈ L
1
(Ω
M
). We also assume that
Ω
µ
= (0, P h], Ω
M
= (P h, L),
for some P that depends on h such that P h is constant. Then
|φ
c
− Iφ
µ
|
H
1
(X
c
)
≤ C h
kf
′
k
L
1
(Ω
M
)
+ kfk
L
∞
(Ω
M
)
,
where C only depends on L, α and β.
Proof. In view of Theorem 4.13 and equation (4.38), we have
|φ
c
− Iφ
µ
|
H
1
(X
c
)
≤
h
√
L
α
kf
′
k
L
1
(Ω
M
)
+ kfk
L
∞
(Ω
M
)
+
2β
α
C hkφ
′′
c
k
L
2
(Ω
M
)
,

216 F
rédéric Legoll
where C o
nly depends on Ω = (0, L). In view of Lemma 4.9, we also have
kφ
′′
c
k
L
2
(Ω
M
)
= kψ
′′
c
k
L
2
(Ω
M
)
≤ kψ
c
k
H
2
(Ω
M
)
≤
C
α
kfk
L
2
(Ω
M
)
≤
C
√
L
α
kfk
L
∞
(Ω
M
)
,
w
here ψ
c
(x) = φ
c
(x) − ax/L, and where C only depends on Ω. We hence obtain the
claimed bound.
Theorem 4.15 provides a bound on the first derivatives of the deformations. This
is
hence a bound on strains, in a L
2
-kind of norm. Using a Poincaré inequality, we
deduce from it a bound on the deformations, e.g., a bound on kφ
c
−Iφ
µ
k
L
2
(X
c
)
, where
the norm k ·k
L
2
(X
c
)
is defined on X
c
by
∀ψ ∈ X
c
, kψk
2
L
2
(X
c
)
:=
Z
L
P h
ψ
2
(x) dx + h
P
X
i=0
ψ
i
2
.
Remark 4.16. We observe that, if we choose the partition according to the assump-
tions of Theorem 4.15 with f and f
′
small on Ω
M
(in the L
∞
- and the L
1
-norm,
respectively), then the coupled problem is a good (and converging, when h → 0) ap-
proximation of the microscopic problem. Hence, we can define an appropriate partition
based on the knowledge of the body forces only. This is related to the convexity as-
sumption. Indeed, due to the convexity of the problems, the Euler–Lagrange equations
of the problems are uniformly elliptic, hence their solutions are "singular" only where
the datum f is singular. In the nonconvex case, the situation is different, as is shown in
Section 4.3.
Remark 4.17. The above estimates involve L
2
-bounds on the deformation and its first
derivative. It is also possible to obtain L
∞
-bounds, as is shown in [23, 24], where a
different strategy is followed. See also [42].
4.3 A nonconvex case: the Lennard–Jones case
We now consider a particular example of nonconvex potential, namely the Len
nard–
Jones potential
V
LJ
(x) =
1
x
1
2
−
2
x
6
. (
4.39)
We observe that lim
x→0
V
LJ
(x) = +∞ and lim
x→+∞
V
LJ
(x) = 0, which are physically
relevant properties (see Remark 2.2). Actually, the property that we use in the sequel
is lim
x→+∞
V
LJ
(x)/x = 0, rather than lim
x→+∞
V
LJ
(x) = 0 (see Remark 4.19 for the
mechanical implication of this property). Note also that V
LJ
attains its unique minimum
at x = 1.
Remark 4.18. The particular choice of exponents in V
LJ
(6 and 12) has no influence
on the following analysis. Similar results hold for the potential V (x) =
q
x
p
−
p
x
q
with
p > q > 0.

Atomistic to continuum coupling methods 2
17
Remark 4.19. F
or a one-dimensional solid described by the continuum energy
E
M
(φ) =
Z
L
0
W (φ
′
(x)) dx,
the behaviour of W (x)/x when x → +∞ is related to the cost of making a fracture in
the material. Consider indeed a nonnegative energy density W that attains its unique
minimum at x = 1, and for which c = lim
x→+∞
W (x)/x exists, c ∈ [0, +∞]. Let
a > L and 0 < ℓ < L, and consider the deformations φ
n
defined by φ
n
(0) = 0 and
φ
′
n
(x) =
(
1 on
0, ℓ −
1
n
∪
(
ℓ
, L
)
,
n(a −L) + 1 on
ℓ −
1
n
,
ℓ
,
which all satisfy the same boundary conditions φ
n
(0) = 0 and φ
n
(L) = a. This
sequence represents configurations with a strain larger and more localized when n in-
creases. In the limit n → +∞, it represents a fractured material. The energy of the
configuration φ
n
is
E
M
(φ
n
) =
L −
1
n
W (1)
+
1
n
W (n(a − L)
+ 1),
hence
lim
n→+∞
E
M
(φ
n
) = LW (1) + c(a − L).
If c = +∞, then making a fracture costs an infinite energy. If c = 0, then making
a fracture costs no energy: lim
n→+∞
E
M
(φ
n
) is equal to the energy of the reference
configuration φ(x) = x. If c ∈ (0, +∞), then making a fracture costs a finite amount
of energy.
In this nonconvex case, we show that expression (4.13) for the coupled energy might
be inappropriate, and we describe several ways to circumvent this difficulty. The com-
plete analysis of this case can be read in [23]. Here, we briefly summarize it (see also
[155]).
We consider a material that occupies the domain Ω = (0, L) in the reference config-
uration and that is put in tension. We focus on the case when there are no body forces:
f ≡ 0. Taking into account body forces does not change the qualitative conclusions
of the analysis. If the material is compressed rather than extended, then the analysis is
very similar to the convex case analysis (see, for instance, [23, Theorem 2.1]).
We first look at the macroscopic problem (4.10) with the macroscopic energy
E
M
(φ) =
1
L
Z
L
0
V
L
J
(φ
′
(x)) dx
and the variational space
X
M
=
φ ∈ W
1,1
(0, L);
1
φ
′
(x)
∈ L
12
(0, L), φ(0) = 0, φ(L) = a
. (4.40)
We consider here the tension case: a > L.
218 F
rédéric Legoll
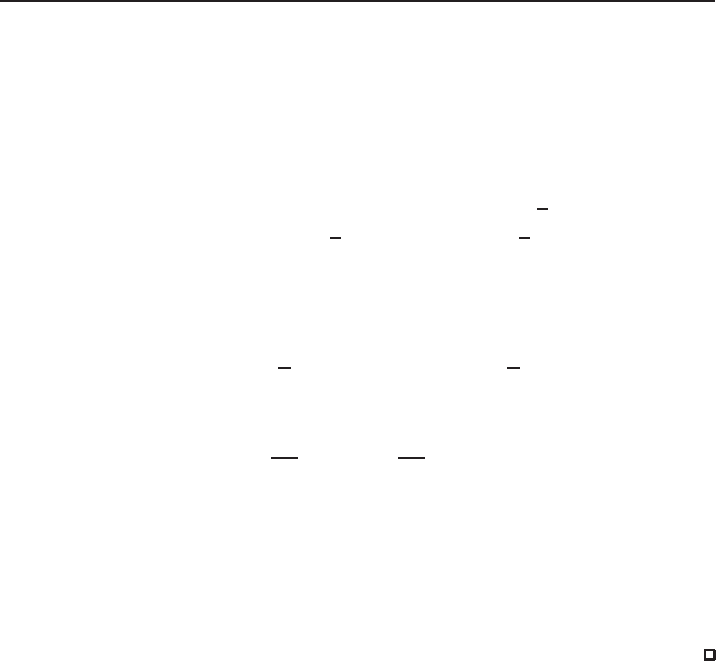
Lemma 4.20. Th
e minimum I
M
in (4.10) is equal to inf V
LJ
. In addition, the infimum
in the problem (4.10) is not attained: there exists no φ ∈ X
M
such that E
M
(φ) = I
M
.
Proof. For any φ ∈ X
M
, we have that E
M
(φ) ≥ infV
LJ
. Hence, E
M
is lower-bounded
and I
M
≥ infV
LJ
> −∞. Consider now the following sequence of continuous func-
tions:
φ
n
(x) =
(
x on
0, L −
1
n
,
a + n
a − L +
1
n
(x − L) o
n
L −
1
n
,
L
.
The function φ
n
is piecewise affine, satisfies the boundary conditions φ
n
(0) = 0,
φ
n
(L) = a, and
φ
′
n
(x) = 1 on
0, L −
1
n
,
φ
′
n
(x) = n
a −L +
1
n
e
lsewhere.
We find
E
M
(φ
n
) =
1 −
1
nL
in
fV
LJ
+
1
nL
V
L
J
(
n(a −L) + 1
)
.
Since lim
x→+∞
V
LJ
(x)/x = 0, we obtain lim
n→+∞
E
M
(φ
n
) = inf V
LJ
. As E
M
(φ
n
) ≥
I
M
, this yields infV
LJ
≥ I
M
. Therefore, we get I
M
= infV
LJ
.
Let us now assume that there exists φ ∈ X
M
such that E
M
(φ) = I
M
. This implies
R
L
0
V
LJ
(φ
′
(x)) dx = L infV
LJ
and thus V
LJ
(φ
′
(x)) = infV
LJ
almost everywhere on
(0, L). We conclude φ
′
(x) = 1 almost everywhere, which is in contradiction with the
boundary conditions φ(0) = 0, φ(L) = a > L. Hence, the infimum in the problem
(4.10) is not attained.
If the variational space is enlarged (in (4.40), replace W
1,1
(0,
L) by SBV (0, L),
whose definition is recalled below), then the problem (4.10) has an infinite number of
solutions with jumps. They are of the form
φ(x) = x +
X
j∈J
v
j
H(x − y
j
), (4.41)
where J ⊂ N, y
j
are any points in Ω = (0, L),
P
j
v
j
= a − L, and H is the Heaviside
function: H(t) = 1 if t ≥ 0, H(t) = 0 otherwise. This statement can be shown by
arguments similar to the ones used to prove Theorem 2.1 of [23]. We recall (see [3, 5])
that the set SBV (0, L) of special functions of bounded variation is
SBV (0, L) =
u ∈ D
′
(0, L); u
′
= D
a
u +
P
j∈N
v
j
δ
y
j
, D
a
u ∈ L
1
(0, L),
y
j
∈ (0, L),
P
j∈N
|v
j
| < +∞
,
where δ
y
j
is the Dirac distribution centered at y
j
(note that H
′
(· − y
j
) = δ
y
j
in the
distributional sense).

Atomistic to continuum coupling methods 2
19
Note that, with the above continuum model, neither the number nor the locations
o
f the fractures are determined. All the configurations (4.41) have the same energy,
whatever J, v
j
and y
j
are.
The fully atomistic model can be analyzed with methods similar to the ones used in
[23, Section 2.2]. It turns out that the minimizers of this model have a unique fracture,
whose location is not determined (the energy is the same whatever the fracture location
is).
Hence, working with the Lennard–Jones potential and tension boundary conditions
is interesting, since it leads to a deformation with a singularity (here, a jump, which
represents a fracture in the one-dimensional bar). Our aim is thus to design a multi-
scale method able to describe this singularity by an atomistic model, while keeping a
continuum description where it is accurate enough. Since the location of the fracture is
not prescribed by the atomistic model, we can afford to fix a priori the partition of Ω,
with the aim to find the singularity in Ω
µ
.
Let us now follow the strategy explained in Section 4.2. We consider a given parti-
tion of Ω, and, to simplify notations, we again assume that this partition is (4.12). In
the spirit of the coupled energy (4.13) and the coupled space (4.14), we consider the
energy
E
c
(φ) =
1
L
Z
L
P
h
V
LJ
(φ
′
(x)) dx +
1
N
P −1
X
i=
0
V
LJ
φ
i+1
− φ
i
h
, (
4.42)
defined on the variational space
X
c
=
(
φ; φ
|Ω
M
∈ SBV (Ω
M
), 1/φ
′
∈ L
12
(Ω
M
),
φ
|Ω
µ
= {φ
i
}
0≤i≤P
∈ R
P +1
, φ
0
= 0, φ(L) = a, φ
P
= φ(P h)
)
. (4.43)
We consider the tension case: a > L. Following the arguments of [23, Lemma 2.3],
one can show that the minimizers of
inf{E
c
(φ); φ ∈ X
c
}
are of the form
φ
i
= ih, 0 ≤ i ≤ P,
φ(x) = x +
X
j∈J
v
j
H(x −y
j
), ∀x ≥ P h,
where J ⊂ N, y
j
are any points in Ω
M
and
P
j
v
j
= a − L. Therefore, as in the com-
pletely macroscopic description, the material breaks. However, the fracture is always
in the domain Ω
M
, in which the deformation φ is assumed to be regular (since we use a
macroscopic model in that domain). Hence, we do not reach our aim with the coupled
method (4.42)–(4.43).
Proving the above statement is quite technical. The following calculations help
to understand why the fracture systematically occurs in the continuum domain. We
consider two configurations, the first one with a fracture in Ω
M
and the second one
with a fracture in Ω
µ
, and compare their energies.
220 F
rédéric Legoll
Ω
M
Ω
µ
x
P
h
φ
1
Ω
µ
x
Ω
M
φ
2
P
h
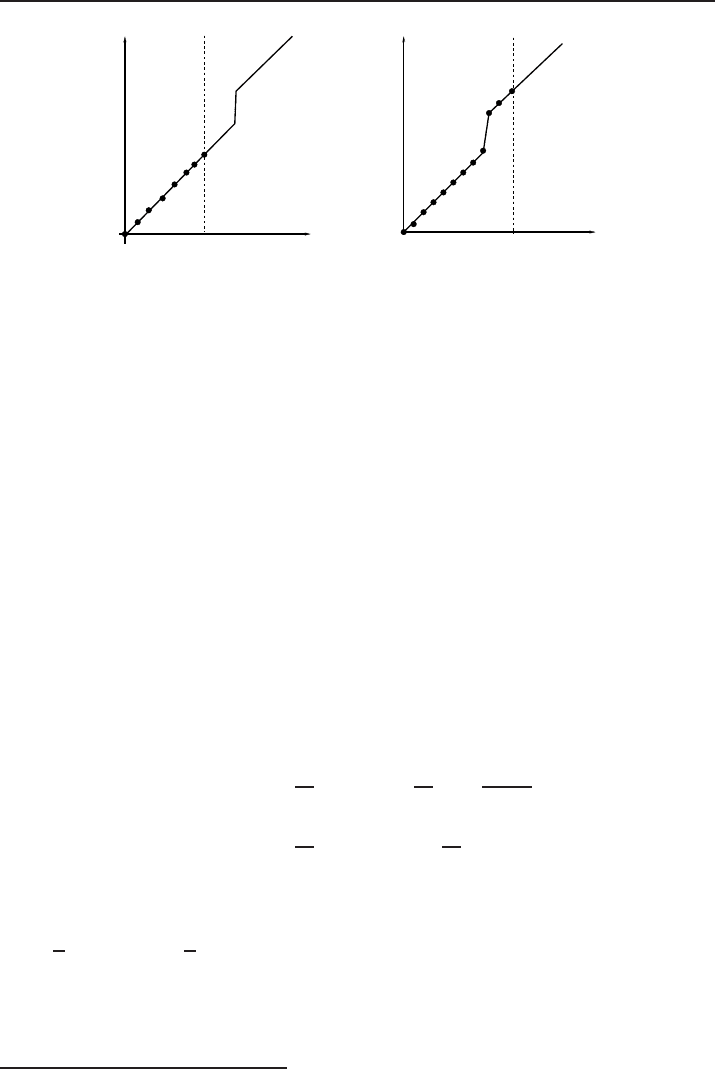
Figure 3. Configurations φ
1
(with a crack in Ω
M
) and φ
2
(with a crack in Ω
µ
).
Let us define φ
1
∈ X
c
by
φ
i
1
= ih, 0 ≤ i ≤ P,
φ
1
(x) = x + (a −L)H(x − y
0
), ∀x ≥ P h,
(4.44)
where y
0
is any real number, P h < y
0
< L (see Figure 3). The map φ
1
represents a
deformation with a fracture in Ω
M
, located at y
0
. We also consider φ
2
∈ X
c
defined by
φ
i
2
= ih, 0 ≤ i ≤ Q
0
,
φ
i
2
= ih + (a − L), 1 + Q
0
≤ i ≤ P,
φ
2
(x) = x + (a −L), ∀x ≥ P h,
(4.45)
which represents a deformation with a fracture in Ω
µ
on the atomic bond (Q
0
, 1+ Q
0
),
with arbitrary integer Q
0
∈ [0, P − 1].
Their energies are
E
c
(φ
1
) = V
LJ
(1),
E
c
(φ
2
) =
1 −
1
N
V
L
J
(1) +
1
N
V
L
J
a − L
h
+ 1
=
1 −
1
N
V
L
J
(1) + o
1
N
.
H
ence, E
c
(φ
2
) > E
c
(φ
1
): a configuration with a fracture in Ω
M
has a lower energy
than a configuration with a fracture in Ω
µ
. Actually, the energy cost for a fracture in
Ω
µ
is
h
L
|V
L
J
(1)| + o
h
L
,
whereas the cost for a fracture in Ω
M
is zero
10
. Hence, even
if the difference E
c
(φ
2
) −E
c
(φ
1
) is small, the minimization of E
c
leads to locating the
fracture in Ω
M
.
There are several ways out of this difficulty: First, it is possible to modify the
definition of the coupled energy. More precisely, the idea is to modifythe elastic energy
10
We define the cost of a fracture by the difference between the energy of a configuration with a fracture and
the energy of the reference configuration.
Atomistic to continuum coupling methods 2
21
Ω
M
Ω
µ
x
P
h
φ
1
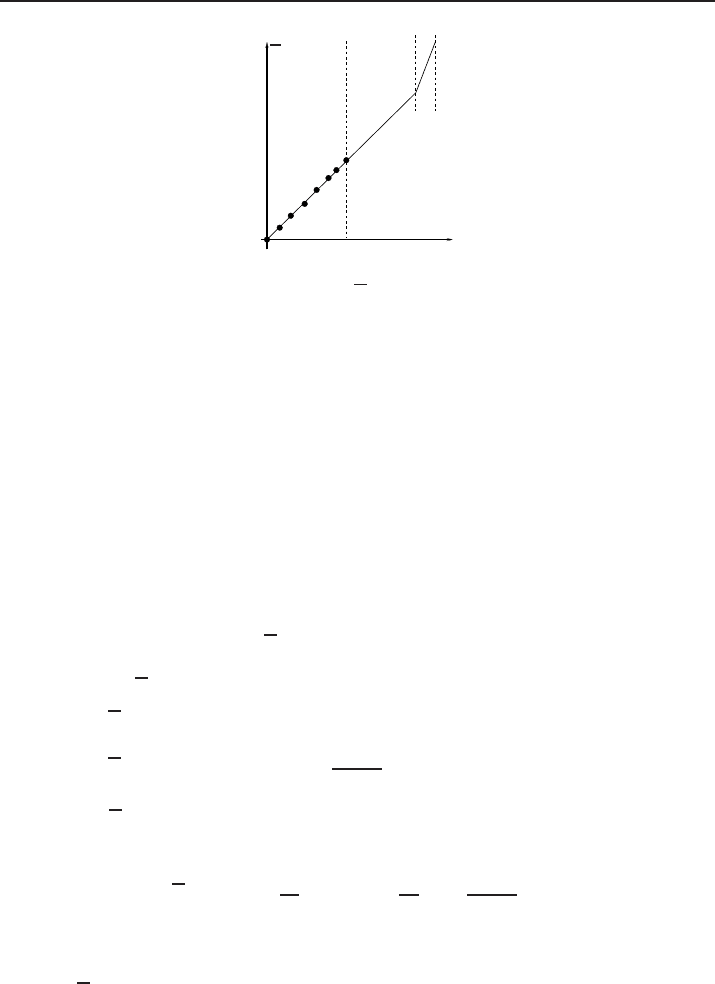
H
F
igure 4. Configuration
φ
1
(
with a crack in Ω
M
).
density that is used in Ω
M
in such a way as it remains consistent with the atomistic
model, and such that making a fracture in Ω
M
costs more than making a fracture in
Ω
µ
. See [23] (in particular, Section 2.4) for details. Introducing this modified coupled
energy is possible because we have a very detailed understanding of the difficulties
associated with the coupled energy (4.42).
Second, we notice that, in practice, one does not work with functions in the space
(4.43), but within a finite dimensional subset of it. Consider for instance the Galerkin
approximation
X
H
c
= {φ ∈ X
c
; φ is piecewise affine on Ω
M
on a mesh of size H}. (4.46)
Let us again compare the energies of cracked configurations. We see that φ
2
defined
by (4.45) belongs to X
H
c
. However, φ
1
defined by (4.44) does not belong to X
H
c
. Let
us define its discretized version
φ
1
∈ X
H
c
b
y
φ
i
1
= i
h, 0 ≤ i ≤ P,
φ
1
(x)
= x, P h ≤ x ≤ L −H,
φ
1
(x)
= a + (x − L)
a − L
H
+ 1
,
L −H ≤ x ≤ L.
The map
φ
1
b
elongs to X
H
c
and represents a deformation with a fracture in Ω
M
,
located in the last finite element (see Figure 4). We find
E
c
φ
1
=
1 −
H
L
V
L
J
(1) +
H
L
V
L
J
a −L
H
+ 1
.
I
f H is chosen such that H ≫ h (which makes the problem defined on X
H
c
cheaper
to solve than the fully atomistic problem, since it involves fewer degrees of freedom),
then E
c
φ
1
>
E
c
(φ
2
). Actually, one can prove that the minimizers of the coupled
energy E
c
on X
H
c
have a fracture which occurs in the atomistic domain Ω
µ
.
Let us summarize our observations:
•
Minimizing the coupled energy (4.42) on the space (4.43) leads to issues, since a
fracture appears in the continuum domain.

222 F
rédéric Legoll
•
I
ntroducing a (coarse enough) mesh in the continuum domain regularizes the
problem: when minimizing the coupled energy (4.42) on the space (4.46), a frac-
ture appears, which is contained in the atomistic domain.
The situation is hence saved by the fact that we look at a discretized version of the
problem, for a sufficiently large discretization parameter H.
This discussion shows that the nonconvex case is much more challenging than the
convex case, and that unexpected difficulties appear, although the general setting is
extremely simple (a one-dimensional system with nearest-neighbour interactions). We
expect to meet similar difficulties in more general settings (problems in higher dimen-
sion or long-range interactions).
5 Discussion
In this section, we review some general questions about the modelling and setting of
the problem. We also review some methods recently proposed and discuss some open
questions at the front of research.
5.1 The nearest-neighbour assumption
We gather here some remarks concerning the atomistic model (2.4) that we
used:
E
µ
(φ
1
, . . . , φ
N
) =
1
2
N
X
i=
1
X
j6=i
V (φ
j
− φ
i
).
In general, the potential energy V (φ
j
− φ
i
) tends to zero when the distance between
atoms i and j goes to +∞ (see Remark 2.2). Hence, one often simplifies the expres-
sion (2.4) (which is a sum of N
2
/2 terms) by introducing a cutoff r
cut
> 0 in V : we
approximate E
µ
(φ
1
, . . . , φ
N
) by
E
cut
µ
(φ
1
, . . . , φ
N
) =
1
2
N
X
i=
1
X
j6=i,kφ
j
−φ
i
k≤r
cut
V (φ
j
− φ
i
), (5.1)
which is cheaper to evaluate than (2.4), in general (indeed, only a finite number of
atoms j are within the cutoff radius of any atom i for reasonable deformations). Ana-
lyzing a model such as (2.4) is difficult due to the long-range interactions (whose range
increases when N increases!). Analyzing a model based on (5.1) is also challenging,
because we do not know a priori which atoms interact with each other. Hence, for
analysis purposes, a further simplification is often made: instead of considering the
energy (5.1), with a finite range of interaction in terms of the distance between atoms,
we consider an energy with a finite range of interaction in terms of atoms indices:
E
FR
µ
(φ
1
, . . . , φ
N
) =
1
2
N
X
i=1
X
j6=i,kj−ik≤I
R
V (φ
j
− φ
i
), (5.2)

Atomistic to continuum coupling methods 2
23
for some cutoff I
R
.
In a one-dimensional setting, the extreme case is I
R
= 1, corre-
sponding to nearest-neighbour interactions.
When making the above assumption of working with (5.2), we implicitly assume
that the ordering of the atoms in the deformed configuration is the same as the or-
dering in the reference configuration. Consider indeed the case of nearest-neighbour
interactions for a one-dimensional model:
E
FR
µ
(φ
1
, . . . , φ
N
) =
N−1
X
i=1
V (φ
i+1
− φ
i
). (5.3)
If the ordering in the current (deformed) configuration is not the original one, then we
have one of the following situations:
•
There exist indices i and j, 1 ≤ i, j ≤ N , with j > i+1, such that φ
i
≤ φ
j
≤ φ
i+1
.
The interaction between atoms i and j is not taken into account in (5.3), although
they are closer to each other than atoms i and i + 1, whose interaction is taken
into account in (5.3). This does not make sense.
•
There exist indices i and j, 1 ≤ i, j ≤ N , with j ≤ i−1, such that φ
i
≤ φ
j
≤ φ
i+1
.
Again, it does not make sense not to consider the couple (j, i + 1) whereas the
couple (i, i + 1) is considered.
So, when working with (5.2), a natural assumption is that the ordering of the atoms is
preserved by the deformation. A simple way to enforce this is to impose the constraint
φ
i+1
≥ φ
i
for all i in the variational space.
For pedagogical purposes, we have not included this constraint in the atomistic
variational space (4.8). See [23, 24] for an analysis of the same models as in Section
4, where the variational spaces include this ordering constraint. We show there that, if
the boundary value a is large enough (recall that, in (4.8), we impose φ
N
= a), then
the constraint is not attained, and the analysis is exactly the same as the one without
constraint that we perform here. On the other hand, if a is not large enough, then the
variational problems do not have any solution.
5.2 Beyond global minimization
In these notes, we have adopted a variational viewpoint: we have defined the
solution
of the various models as the global minimizer of the respective energies (as we did
in [23, 24]). This approach was also followed in [102], where problems similar to
the one studied here were studied. More precisely, a finite one-dimensional atomistic
chain is considered, with the energy (2.4), and the Lennard–Jones interaction potential
(4.39) for V . There are no body forces and no boundary conditions (except a Dirichlet
condition to remove translational invariance). The reference problem is hence written
as
inf
1
2
N
X
i=1
X
j6=i
V
LJ
(φ
j
− φ
i
); φ ∈ R
N
, φ
1
= 0, φ
i+1
> φ
i
.

224 F
rédéric Legoll
The influence, on the solution of the above variational problem, of introducing a cutoff
r
adius in V
LJ
, as well as the influence of making a QuasiContinuum like approximation
(see Section 5.3) are analyzed.
An alternative approach to global minimization is to look for local minimizers (see,
for instance, [63, 64, 66]). A difficulty linked to this approach is that there are in
general many local minimizers. In that setting, one often proves that, close to a given
atomistic solution, there exists a solution of the hybrid model.
Another possibility is to try and select one local minimizer by some criterion. For
instance, one can consider a gradient flow dynamics [128, 138], which writes, in its
simplest formulation,
dφ
dt
= −
∇E
µ
(φ).
In the long-time limit, the solution of the above dynamical system converges to a con-
figuration which is a local minimizer of the energy, and which is considered as the
reference solution of the atomistic model.
Yet another option is to study critical points of the energy rather than local mini-
mizers. See [129, 130] for such a choice in an atomistic to continuum setting (and also
[103] for a study in a two-dimensional setting, under some simplifying assumptions,
reminiscent of convexity assumptions). Note that critical points are also considered in
[12, 153], where the authors go beyond standard continuum mechanics models by con-
sidering elastic densities that depend on second derivatives of the deformation, hence
obtaining a continuum model with a small parameter (see Section 5.5, and energy
(5.11) in particular).
5.3 The QuasiContinuum method and the ghost forces
The method that we have analyzed in Section 4 is a toy example for more advan
ced
methods such as the QuasiContinuum method (QCM). In its initial version [150, 151],
this method starts from the continuum scale, with a standard continuum mechanics
model, discretized by a finite element method. The multiscale feature of the method
appears when the elastic energy of an element is computed. Depending on some crite-
ria, some elements are declared to be too heterogeneously strained for a macroscopic
description to hold, and they are considered as a set of discrete particles. The energy
of each element is computed according to the scale at which the element is described:
either the element is too heterogeneously strained, and its energy is computed on the
basis of an underlying atomistic model, or a standard continuum mechanics formula is
used.
In the second version of the method [146], that we describe below, the opposite
viewpoint is adopted. The starting point is a multibody atomistic energy, which is such
that it is possible to define the energy E
i
(φ) of the atom i when the current configura-
tion of the atomistic system is φ. We start from the energy
E
µ
(φ) =
N
X
i=1
E
i
(φ). (5.4)
