Duan C.G., Karelin V.Y. Abrasive Erosion and Corrosion of Hydraulic machinery

Подождите немного. Документ загружается.

94 Abrasive Erosion and Corrosion of Hydraulic Machinery

ASME Fluids Engineering Summer Meeting, June 21-25, 1998,

Washington, DC, FEDSM98-5229

2.13 Stieglmeier, M, Tropea, C, Weiser, N. and Nitsche, W., (1989).

'Experimental Investigation of Turbulent Flow through Axisymmetric

Expansions', ASME Journal of Fluids Engineering, Vol. Ill, pp.

464-471.

Chapter 3

Analysis and Numerical

Simulation of Liquid-Solid Two-

Phase Flow

Y. L. Wu

3.1 Basic Equations of Liquid-Solid Two-Phase Flow

through Hydraulic Machinery

3.1.1 Introduction

Several theoretical and experimental techniques for investigation of

multiphase flow are available. Basically, there are two fundamental theories

for two-phase flow, namely, the macroscopic continuum mechanics theory

and the microscopic kinetic theory. Marble (1963) [3.1] was one of pioneer

researchers to describe basic equations of two-phase flow. From the sixties to

seventies, general governing equations of two phase flow for the two fluid

model and the mixture model based on the continuum mechanics theory were

set up by Drew (1971)

[3.2],

Ishii (1975) [3.3] and others by using different

average methods. In the mixture model, it is assumed that there exists only

one type of mixture in fluid-dispersed flow, and that the whole flow space is

full of the mixture. The mixture flow is described by using a set of unique

characters. Roco & Reinhart (1980) [3.4] applied this model to calculate the

liquid-particle mixture flow through centrifugal pump impellers.

Abrasive Erosion and Corrosion of Hydraulic Machinery

In the two-fluid model, the dispersed phase is treated as a pseudo-fluid. In

the Eulerian approach, the flow of the dispersed phase is described by

conservation equations of mass, momentum and energy in continuum

mechanics. In this model, there exists the slip of parameters between the

carrier fluid and the dispersed phase. Based on the two-fluid model, increasing

interest in the prediction of turbulent multi-phase flow has been noticed during

the last twenty years, such as Danon et al (1974)

[3.5],

Al Taweel & Landau

(1977)

[3.6],

Genchiev & Karpuzov (1980)

[3.7],

Melville et al (1979)

[3.8],

Sharma & Crowe (1978)

[3.9],

Michaelides & Farmer (1984) [3.10] and

Shuen et al (1983)

[3.11].

Danon et al (1974) tried to develop the one-

equation turbulence model by adding a length scale determined experimentally

to the turbulent kinetic energy equation for calculating two-phase round jet-

turbulent-flow. Al Taweel & Landau (1977) deduced an energy spectrum

equation at the transient frequency band, adding a supplement dissipation

term to consider appearance of the dispersed phase. Calculated results show

that the increase of volumetric density of the dispersed phase causes the

turbulence intensity at the high frequency band decrease. Genchiev &

Karpuzov (1980) proposed that a sink term has to be added to the single

phase turbulent kinetic energy k equation to simulate the influence of the

disperse phase. These authors applied the two-equation turbulence model to

calculate two-phase turbulent flow. Two-equation turbulence models also

have been proposed for dilute fluid-particle flow by Elghobashi & Abou-Arab

(1983) [3.12] and Crowder et al (1984)

[3.13].

Algebraic and one equation

turbulence models have been suggested for the dense liquid-solid interaction

by Roco & Shook (1983)

[3.14].

Bertodano et al (1990) [3.15] applied the

two-fluid model combined with the Reynolds stress turbulence model to

analyze water-bubble flow. L.X. Zhou (1994) [3.16] suggested the k - s - k

gas-particulate two-phase turbulence model (and other models) to calculate

the gas-dispersed flow. Most of existing models are suitable only for

relatively dilute mixtures, in which the particle-particle collision effect and the

fluctuation energy interaction between the liquid and the particulate phases

are negligible. During the last decade, there has been an interesting

development in modeling dense solid-liquid flow, as shown, in references of

Ma & Roco (1988)

[3.17],

Ahmadi & Abu-Zaid (1990) [3.18] and Gidaspow

et al (1991)

[3.19].

As mentioned above, Roco & Shook (1983) applied the

macroscopic continuum theory and the one-equation turbulence model with

double averaging to calculate dense liquid-particle flow. A probabilistic

Analysis and Numerical Simulation of Liquid-Solid Two-Phase Flow 97

microscopic model for solving shear motion of spherical particles dominated

by friction and lubrication force was proposed by Ma & Roco (1988) to slow

granular flow and high dense coal slurry. Gidaspow et al (1991) have

developed a computer code that solves a generalization of N-S equations for

multiphase flow. Particulate phase viscosity and pressure were derived by

using the mathematical techniques of the dense gas kinetic theory.

3.1.2 General Concepts of Multiphase Flow

Basic Parameters of Multiphase Flow

The flow pattern of multiphase flow can be determined by the relations of

following characteristic times:

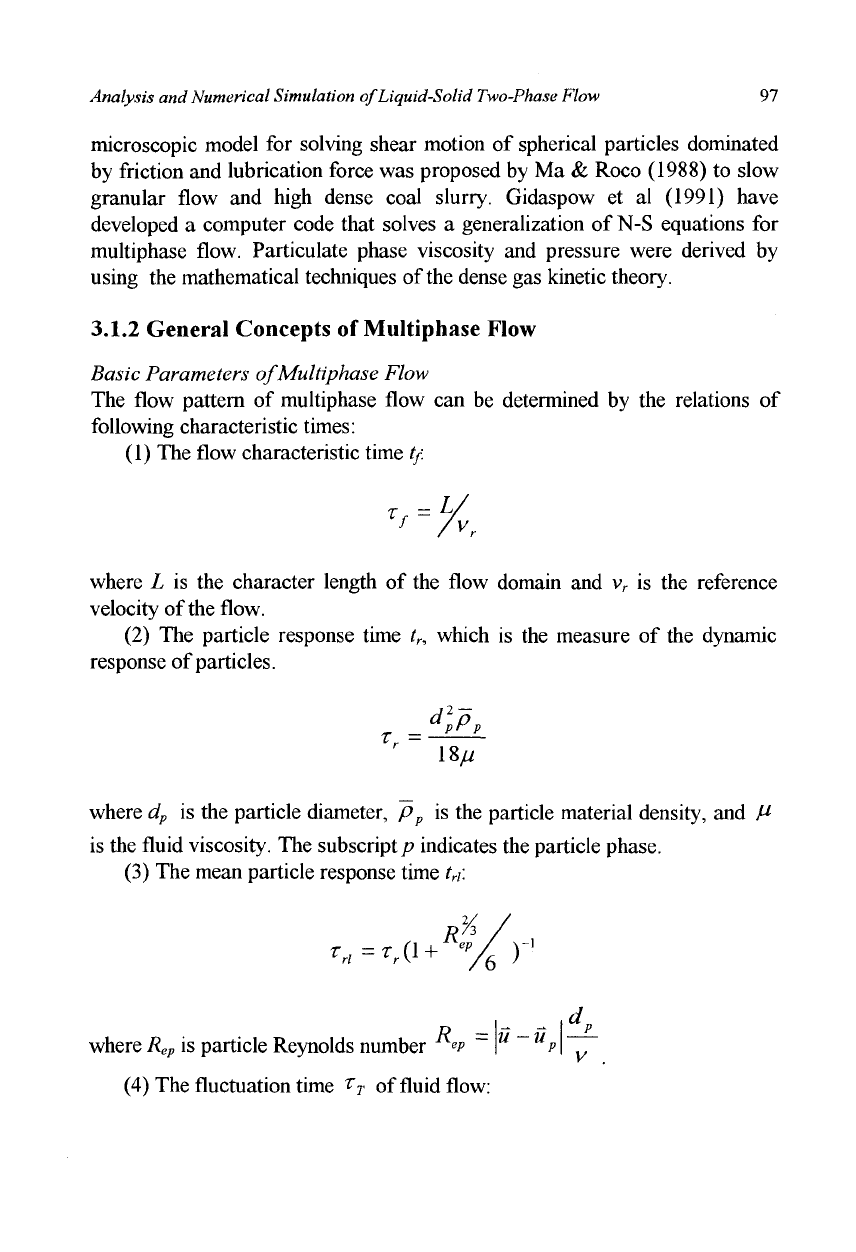
(1) The flow characteristic time t/.

r =V

J- /

J /V

/ r

where L is the character length of the flow domain and v

is the reference

velocity of the flow.

(2) The particle response time t

, which is the measure of the dynamic

response of particles.

18//

where d

is the particle diameter, p

is the particle material density, and H

is the fluid viscosity. The subscript/* indicates the particle phase.

(3) The mean particle response time t

where R

is particle Reynolds number

P \

u U

(4) The fluctuation time

T of fluid flow:

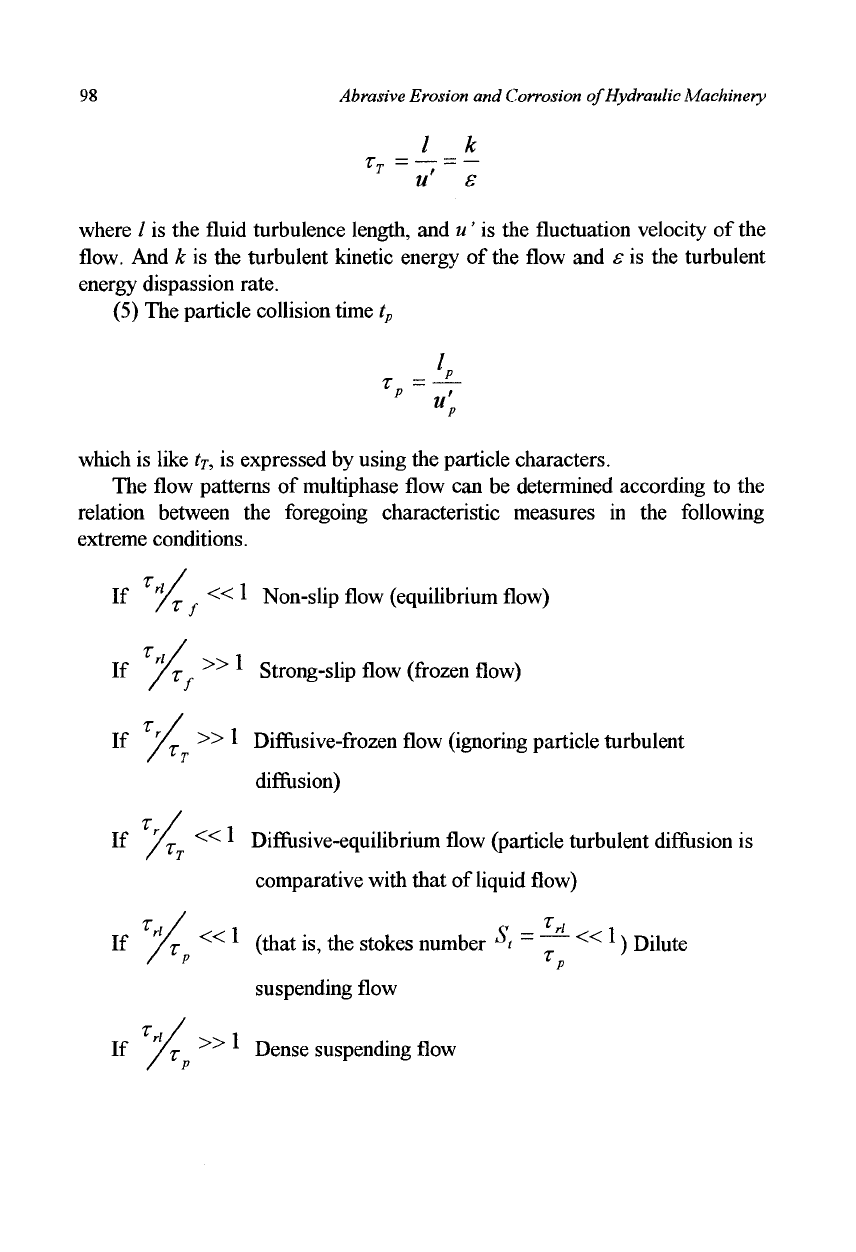
98 Abrasive Erosion and Corrosion of Hydraulic Machinery

I k

U £

where / is the fluid turbulence length, and u' is the fluctuation velocity of the

flow. And k is the turbulent kinetic energy of the flow and s is the turbulent

energy dispassion rate.

(5) The particle collision time t

-■•$

which is like t

, is expressed by using the particle characters.

The flow patterns of multiphase flow can be determined according to the

relation between the foregoing characteristic measures in the following

extreme conditions.

r,/

If /v , « I Non-slip flow (equilibrium flow)

If /x .

Strong-slip flow (frozen flow)

If y

Diffusive-frozen flow (ignoring particle turbulent

diffusion)

comparative with that of liquid flow)

If y

<<:

Diffusive-equilibrium flow (particle turbulent diffusion is

1rl/ ^^ 1 C rl ^^ i

If y-r (that is, the stokes number ' ~

) Dilute

suspending flow

If y

Dense suspending flow

Analysis and Numerical Simulation of Liquid-Solid Two-Phase Flow 99

And the stokes number

n '

is proportional to the (

p p

c)- C

the particle volume fraction.

Density and Volume Fraction

Relations between deferent density determinations of multiphase flow are as

follows:

+pp =p

+^pk =p

+Yu

k =pf

k-^-

where

P™

is the density of

mixture,

Pf is the fluid bulk density, Pk->P

are

the particle bulk densities, and Pp is the particle material density. And

u is

the particle number in the unit volume.

The volume fractions of the fluid phase Q- and the particle phase C

are

defined as:

For dilute two phase flow:

The mass loading is defined as

p(i

, where the subscript "0"

is the values at the entrance condition.

Volumetric Averaging of Multiphase Flow

A control volume has the surface dA, where there are several types (phases)

of particles. For each phase, the occupying volume is dvk with surface area

dAk. Then the local volumetric fraction of kih phase particle can be expressed

as:

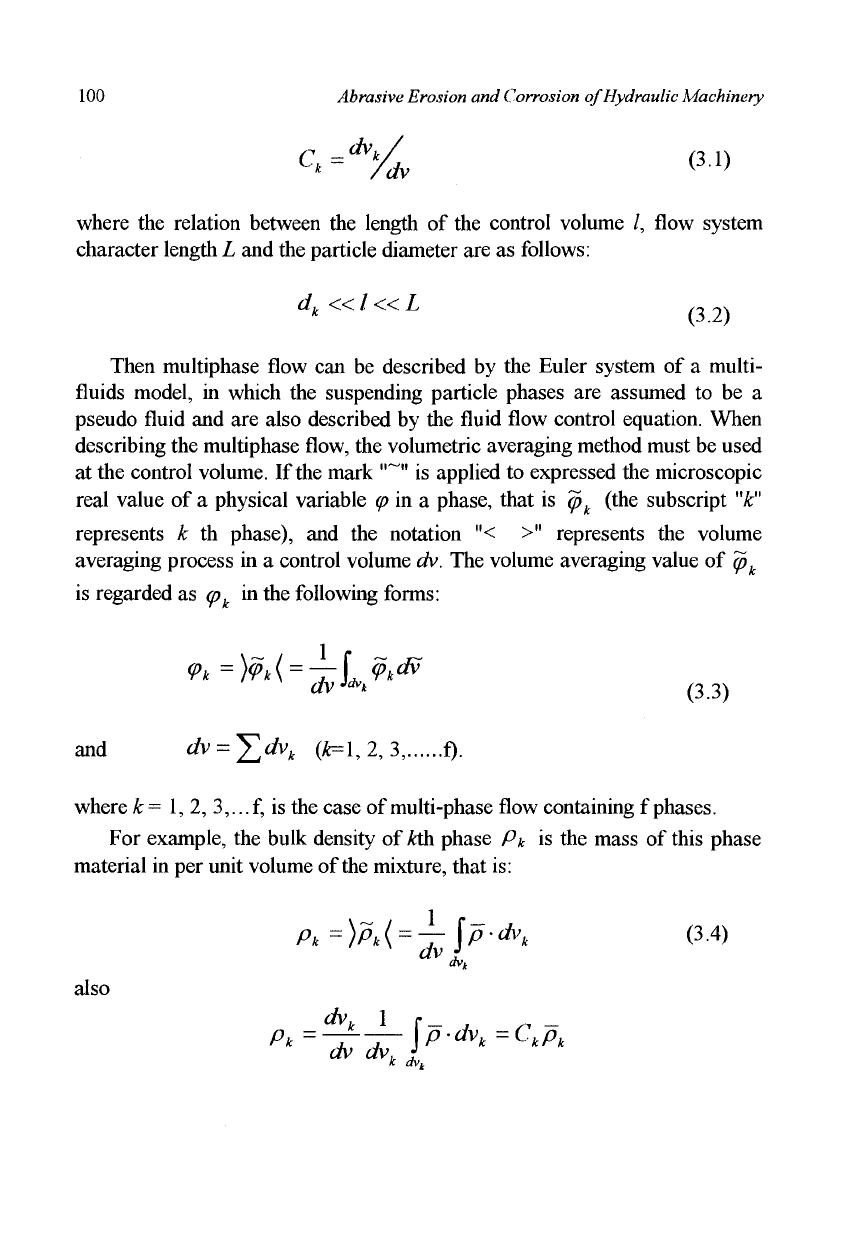
100 Abrasive Erosion and Corrosion of Hydraulic Machinery

where the relation between the length of the control volume

flow system

character length L and the particle diameter are as follows:

«l«L

(32)

Then multiphase flow can be described by the Euler system of a multi-

fluids model, in which the suspending particle phases are assumed to be

pseudo fluid and are also described by the fluid flow control equation. When

describing the multiphase flow, the volumetric averaging method must be used

at the control volume. If the mark "~" is applied to expressed the microscopic

real value of a physical variable

phase, that is

(the subscript "k"

represents

k th

phase), and the notation

"< >"

represents

the

volume

averaging process in a control volume dv. The volume averaging value of

is regarded as

in the following forms:

and dv =

Y,dv

(k=l, 2, 3,

f).

where k = 1, 2,

3,...

f, is the case of multi-phase flow containing f phases.

For example, the bulk density of Mi phase Pk is the mass of this phase

material in per unit volume of the mixture, that is:

Pk^)p

{

—\p-dv

(3.4)

also

1 I"- 7 n -

Analysis and Numerical Simulation of Liquid-Solid Two-Phase Flow

101

where Pk is the material density of this phase.

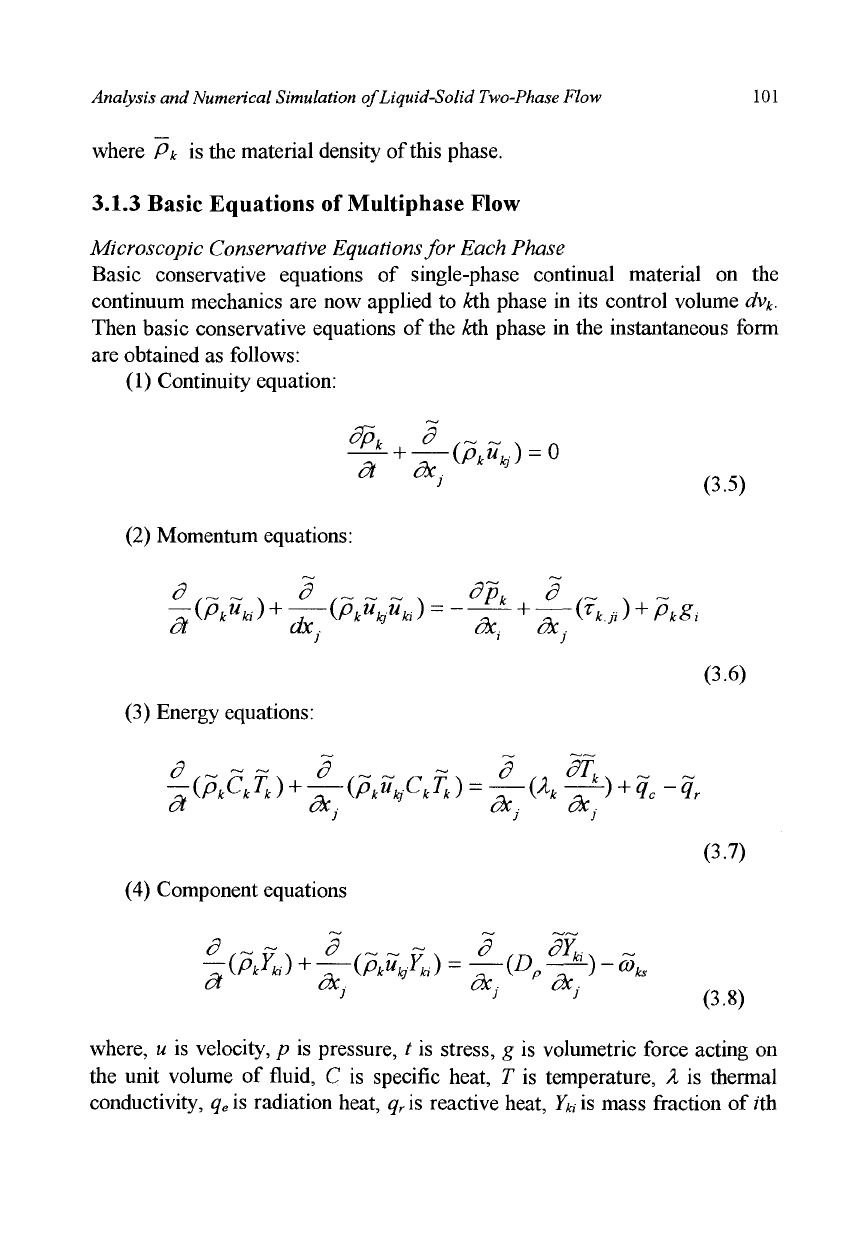
3.1.3 Basic Equations of Multiphase Flow

Microscopic Conservative Equations for Each Phase

Basic conservative equations of single-phase continual material on the

continuum mechanics are now applied to &th phase in its control volume dv

Then basic conservative equations of the kth phase in the instantaneous form

are obtained as follows:

(1) Continuity equation:

]

(35)

(2) Momentum equations:

d , . d , ~ . dp

d ,~ . ~

at ax

ax^

(3.6)

(3) Energy equations:

^(P

)

— (p

) = —{X

—-)

-q

at ax j ax

(3.7)

(4) Component equations

d ~ ~ d .— ~ d ,

. dYft. ~

—(A4-) + —(A"*4) = -z-(D

—*■)

at aXj. aXj. ax

(3.8)

where, u is velocity, p is pressure, / is stress, g is volumetric force acting on

the unit volume of fluid, C is specific heat, T is temperature, X is thermal

conductivity, q

is radiation heat, q,

reactive heat,

is mass fraction of /th

102 Abrasive Erosion and Corrosion of Hydraulic Machinery

component in kth phase,

a>k

is chemical reactive rate of kth phase, and D

the material conductivity of Mi phase.

Volumetrically Averaged Basic Equation of Two-Phase Laminar Flow

The volume averaging method, defined as foregoing, is applied to derive out

the volumetric averaged basic equation of two-phase laminar flow, which are

comparative to N-S equation in single-phase laminar flow.

The averaged value of differential of instantaneous variable (p is in the

following forms.

Tf 9k

* ' *. *"

.9)

ckj I ckj dv

JdA

where u

is the displacement velocity of interfaces; n

is the unit vector of

outward normal on interface of Mi phase.

By using equation (3.9) the volumetric averaged basic equation of Mi

phase laminar flow can be obtained.

(1) Continuity equations

£-(P>

* (k =

U-f)

(

3.io)

where pk is the bulk density of Mi phase, and S

is Mi phase mass source term

is as follows:

^=-^L

^(^-^K^ (3.ii)

For the dilute concentration multi-phase flow, the continuity equation for

liquid is as follows:

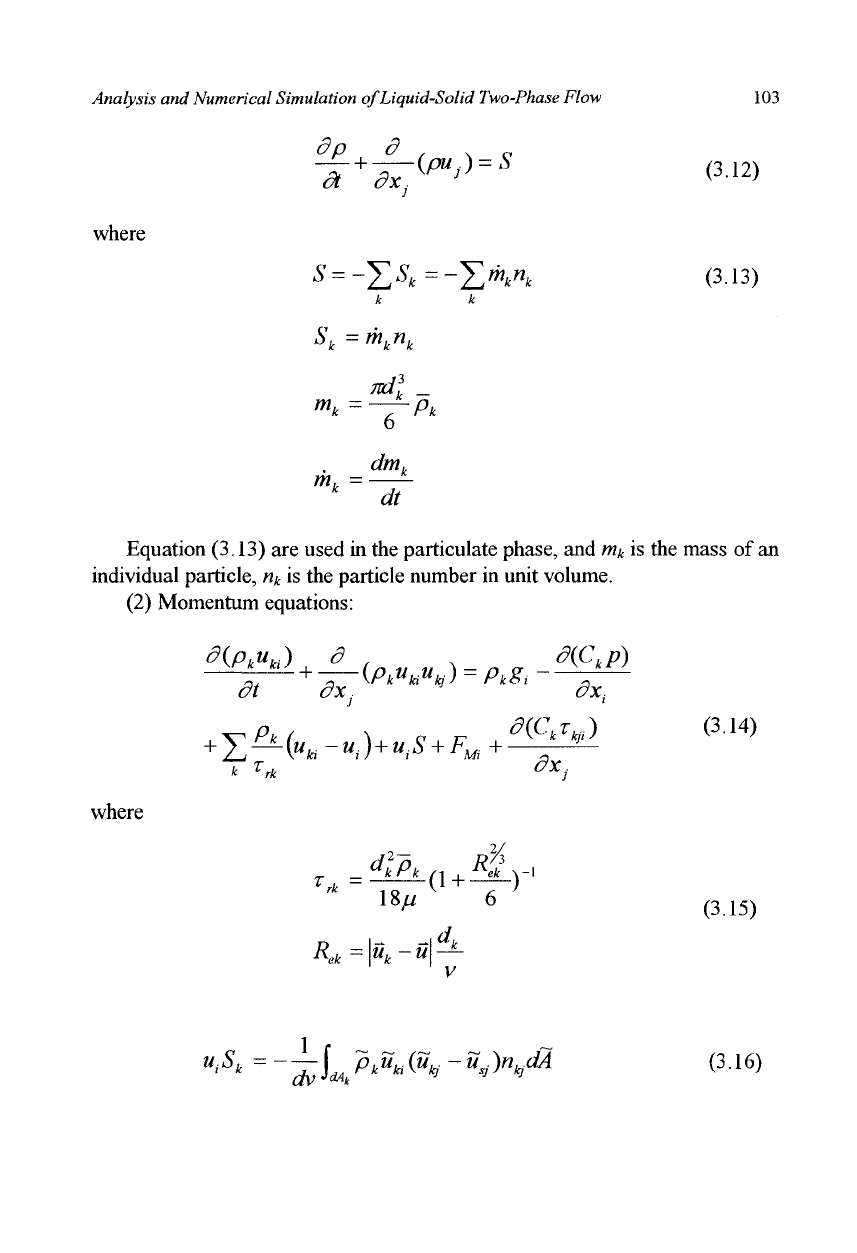
Analysis and Numerical Simulation of Liquid-Solid Two-Phase Flow

103

dp 3 , . „

) =

(3-12)

where

s=-2>,

-2>,«*

(

313

Tvdl

dm,,

Equation (3.13) are used in the particulate phase, and m

is the mass of an

individual particle, n

is the particle number in unit volume.

(2) Momentum equations:

ox, ox.

+ T — (

<ci-

S +

d(P

)

(3.14)

'/*

T„,

dx,.

where

18//

D I- H^t

(3.15)

^ L

"«

(«*

«*

>V^

(316)