Duan C.G., Karelin V.Y. Abrasive Erosion and Corrosion of Hydraulic machinery

Подождите немного. Документ загружается.

Abrasive Erosion and Corrosion of Hydraulic Machinery

by the normal distribution law and, consequently, the mathematical

expectation of energy W

imparted by the particles, with the loading

conditions being varied, can be described by using the Gaussian distribution.

2.2 Prediction Model of Hydraulic Abrasion

2.2.1 Prediction Erosion Model Proposed by Finnie and Bitter

Bitter (1963) classified the mechanisms of erosion wear into two categories,

i.e. those of cutting and deformation [2.4, 2.5]. When a particle hits the target

surface with relatively small angle, i.e. when the collision is more tangential,

the particle tends to scratch the surface and to remove a small amount of its

material. This process is called "cutting wear". On the other hand, when the

surface is exposed to repeated collisions with relatively large incident angle, it

undergoes the fatigue rupture through so-called cold work and a small amount

of the surface material is removed in the form of small fragments during a

subsequent impact. This process is known as "deformation wear". Several

proposals are found in the literature to express each of these processes. Of

these, the following equations by Finnie (1972) [2.6] and Bitter (1963) [2.4,

2.5] are adopted to calculate the cutting and the deformation wear,

respectively:

W„

■ o

sin 2a

' c

^9 2

—^-cos a

-sin

for

for tana < —

\a > —-

(2.36)

W„

(

s[na

(2.37)

where

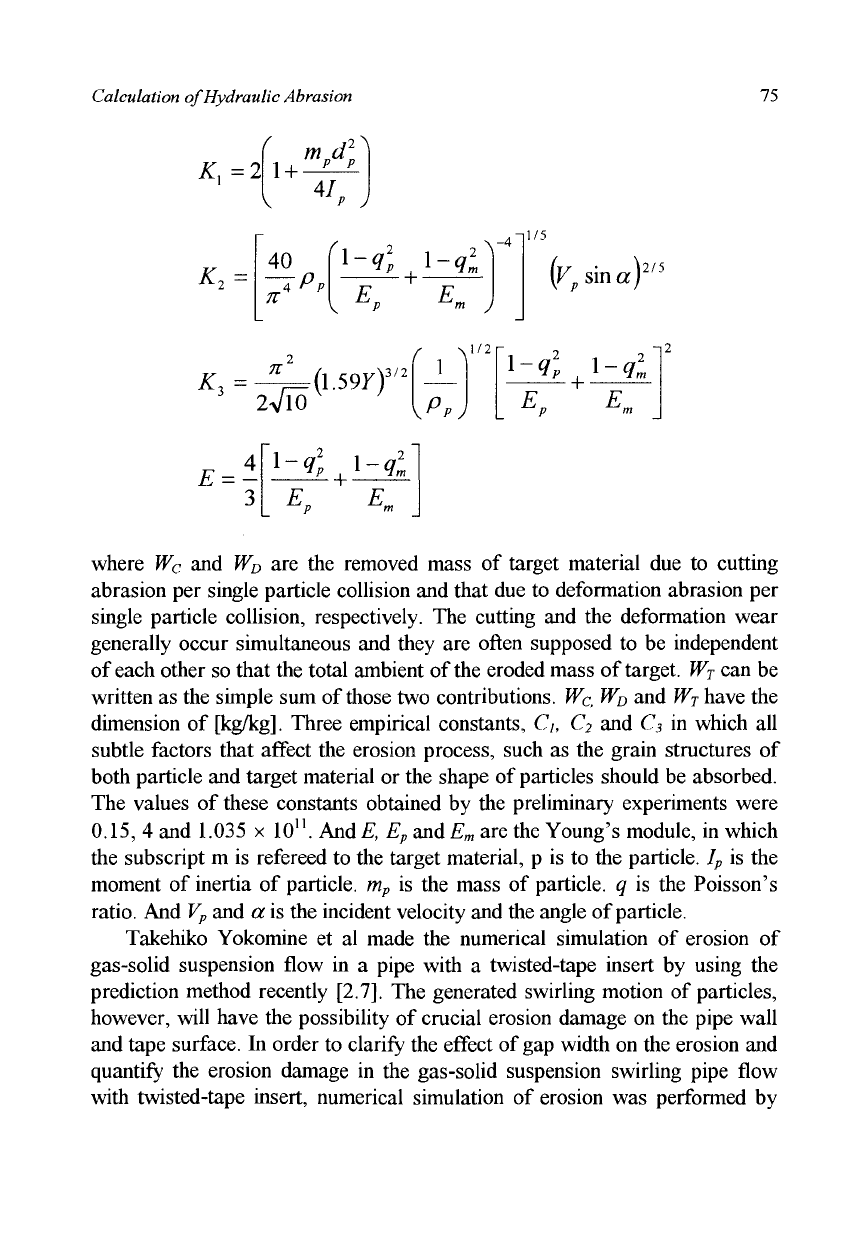
Calculation of Hydraulic Abrasion 75

K,=2

p p

p J

1/5

2V10

v^y

1/2

Ksina)-

!-^ , 1~<7

■

E E

p "

i-t^-d

EP Z~

where W

and JF

are the removed mass of target material due to cutting

abrasion per single particle collision and that due to deformation abrasion per

single particle collision, respectively. The cutting and the deformation wear

generally occur simultaneous and they are often supposed to be independent

of each other so that the total ambient of

the

eroded mass of

target.

can be

written as the simple sum of

those

two contributions. W

, W

and W

have the

dimension of [kg/kg]. Three empirical constants, C

and C

in which all

subtle factors that affect the erosion process, such as the grain structures of

both particle and target material or the shape of particles should be absorbed.

The values of these constants obtained by the preliminary experiments were

0.15,

4 and 1.035 x 10". And£, E

and£

are the Young's module, in which

the subscript m is refereed to the target material, p is to the particle. I

is the

moment of inertia of particle. m

is the mass of particle, q is the Poisson's

ratio.

And V

and a is the incident velocity and the angle of particle.

Takehiko Yokomine et al made the numerical simulation of erosion of

gas-solid suspension flow in a pipe with a twisted-tape insert by using the

prediction method recently

[2.7].

The generated swirling motion of particles,

however, will have the possibility of crucial erosion damage on the pipe wall

and tape surface. In order to clarify the effect of

gap

width on the erosion and

quantify the erosion damage in the gas-solid suspension swirling pipe flow

with twisted-tape insert, numerical simulation of erosion was performed by

Abrasive Erosion and Corrosion of Hydraulic Machinery

using Eulerian/Lagrangian approach and two-way coupling method. When the

gap is small, the belt-shaped highly concentrated region of particles is formed

near the pipe wall and causes the crucial erosion damage like ditch on the pipe

wall. If the gap is large, the erosion damage can be decreased and the uniform

distribution of particles near the pipe wall is realized. The latter effect is

advantageous to the use of increased heat capacity by adding particles.

Drtina and M. Krause (1994) [2.8] also applied the method developed

by Finnie (1972) for ductile materials to predict the abrasion of the guide

vanes of a hydraulic turbine. The abrasion rate, ER, is defined as the mass of

material removed to the mass of particle in the carrier fluid, that is,

ER=p„p

(2.38)

where S

is the weight of sands in a unit volume, p

is the material density of

metal, and

p„

is the penetration rate to be estimated. Basically the abrasion

rate,

ER, depends on the kinetic energy of impinging particle and its incidence

angle a:

"f{a) (2.39)

with

/(a)=-sin

a 0 < a < 0.4?r

f(a) - sin 2a -3 cos

a QAn<a<Q.5n

where n has been set to 2. It has to be kept in mind that for a given mass of

abrasion particles impinging on a surface the totally removed material

calculated via the foregoing equation will be independent of the particle size.

Finnie pointed out that this is true only for particles with diameters exceeding

100 um. Below this value abrasion becomes less effective with decreasing

particle size.

In order to verify the model and the computation method of silt abrasion

in hydraulic turbines, the test results of the hydraulic abrasion through guide

vanes in a model hydraulic turbine by P. Dritina and M. Krause (1994) [2.8]

Calculation of Hydraulic Abrasion

are selected to be compared with the present prediction by Yulin Wu. And the

silt condition and the flow condition through the vane are as follows:

Particle size d

= 0.060 mm

Vane orientation p = 59°

Inlet angle y = 29°

Turbulence intensity 5%

Inflow velocity 16.3 m/s

The three dimensional computation of silt-laden two-phase flow through

the guide vanes was carried out by using two-fluid model proposed in Chapter

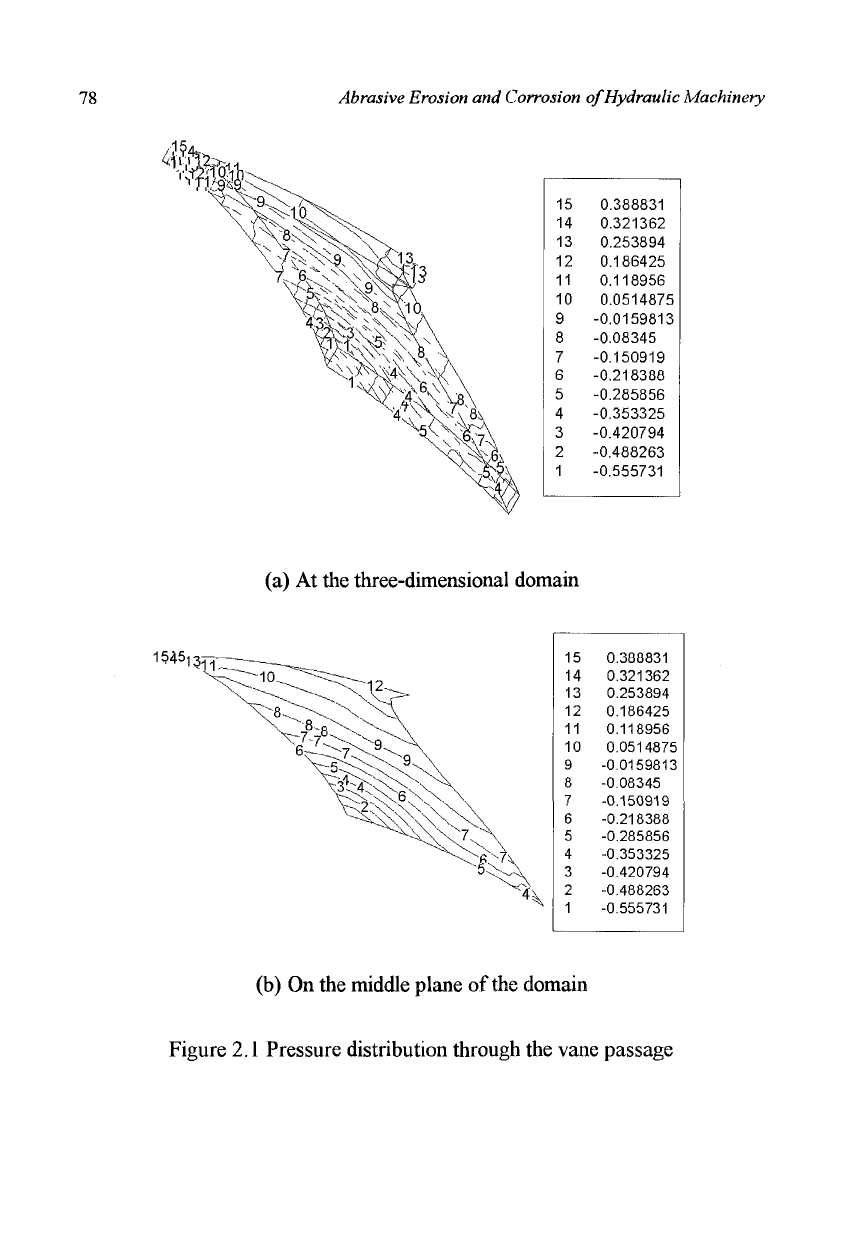
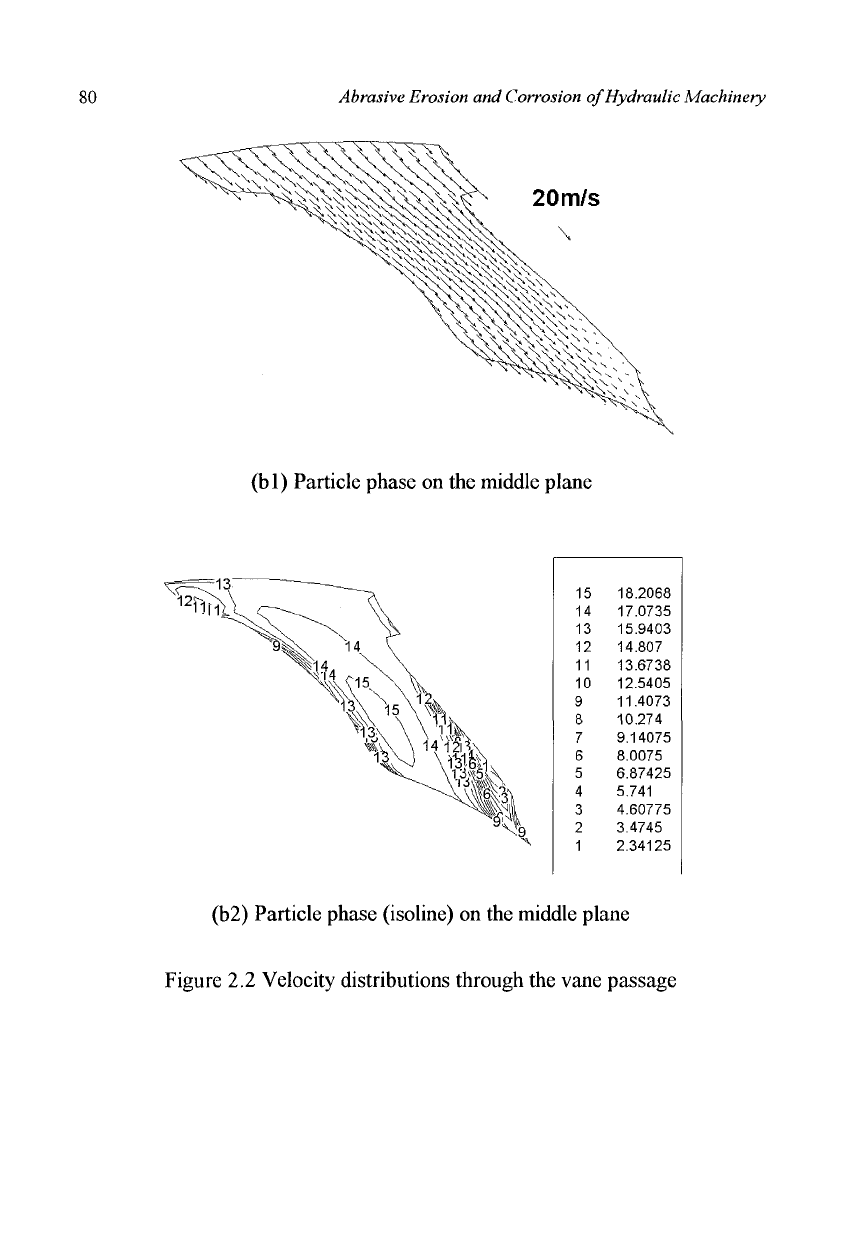
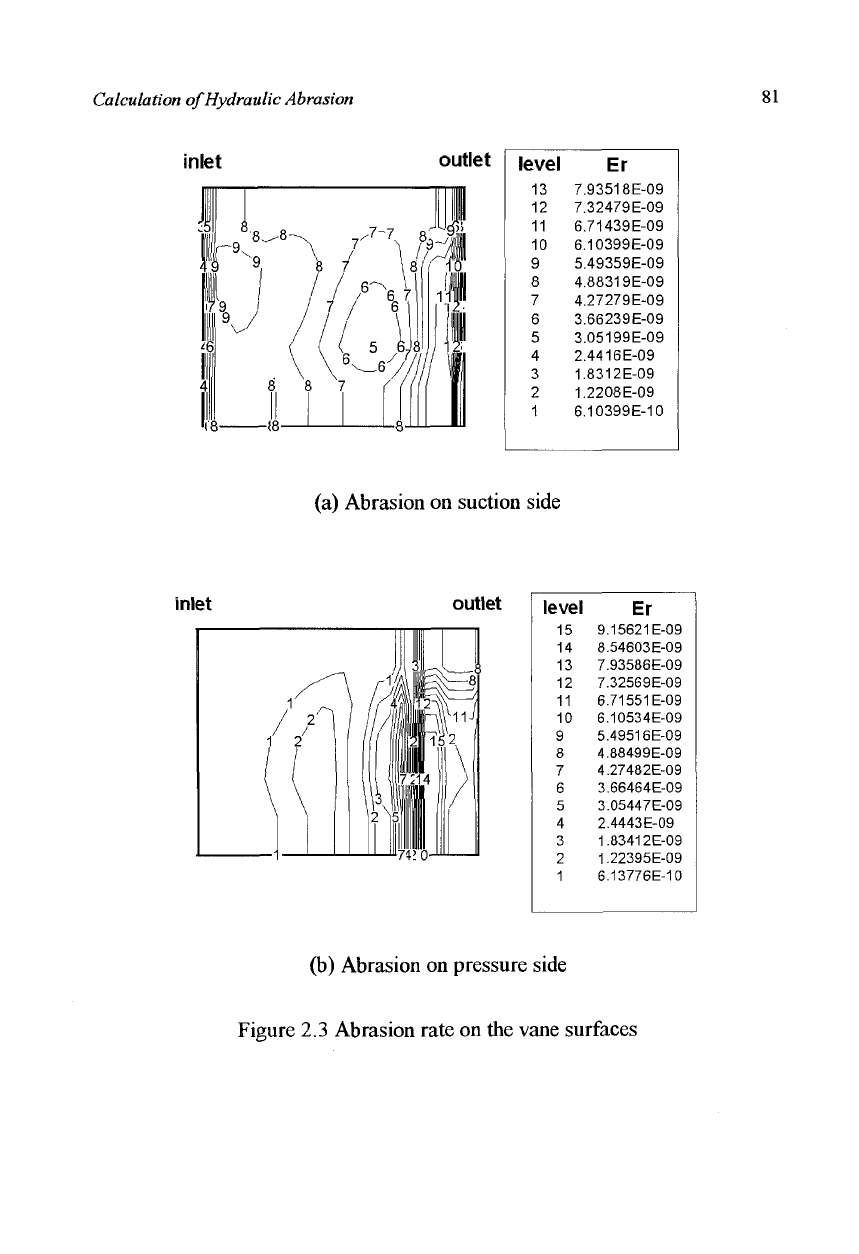
Figure 2.1 to Figure 2.3 show the flow calculation results and the silt

abrasion prediction results. Among them, Figure 2.1 (a) and (b) show the

pressure distribution through the vane passage at the three-dimensional

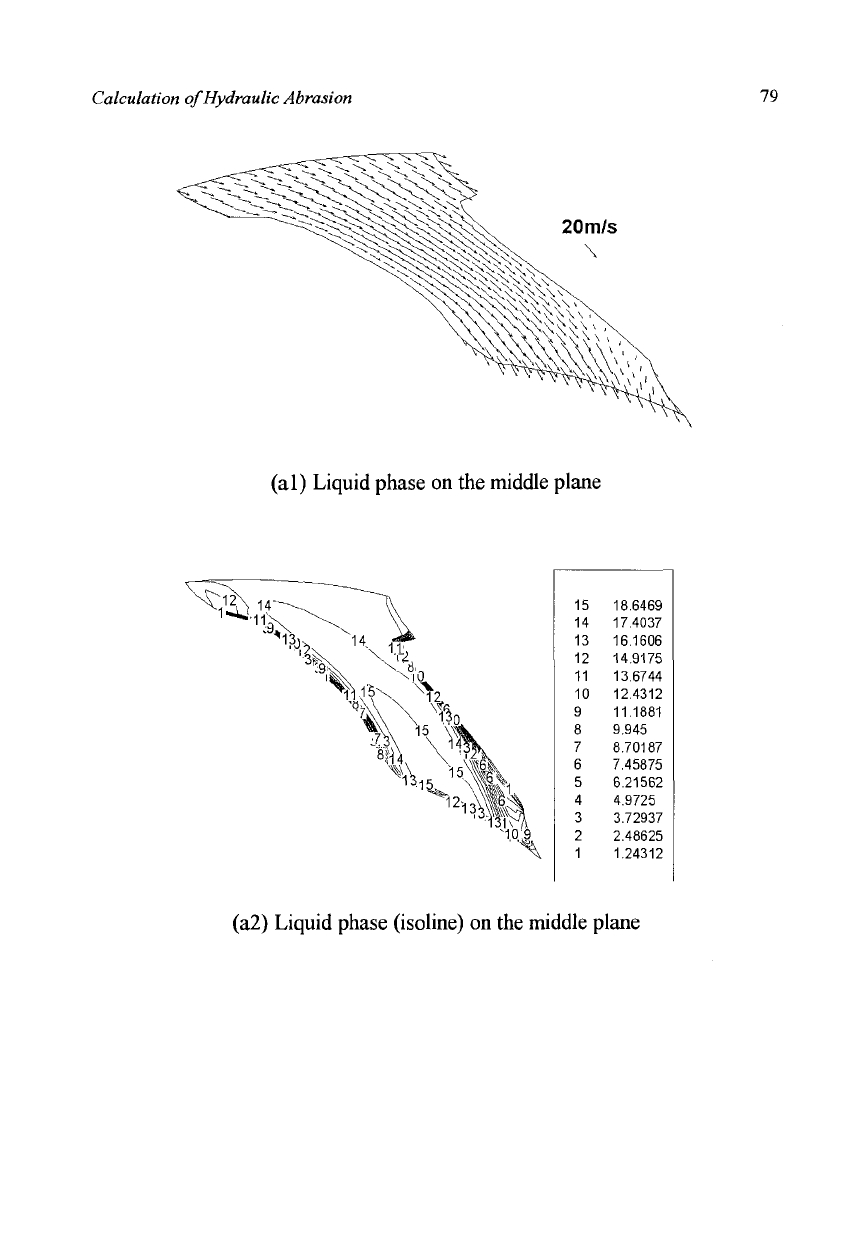
domain. Figure 2.2 (a) and (b) show the velocity distributions bother for the

liquid flow (Figure 2.2 (a)) and for the particle phase flow (Figure 2.2 (b)) on

the middle plane through the vane passage. Figure 2.3 is the silt abrasion rate

on the vane surfaces as the three-dimensional predicted results in the present

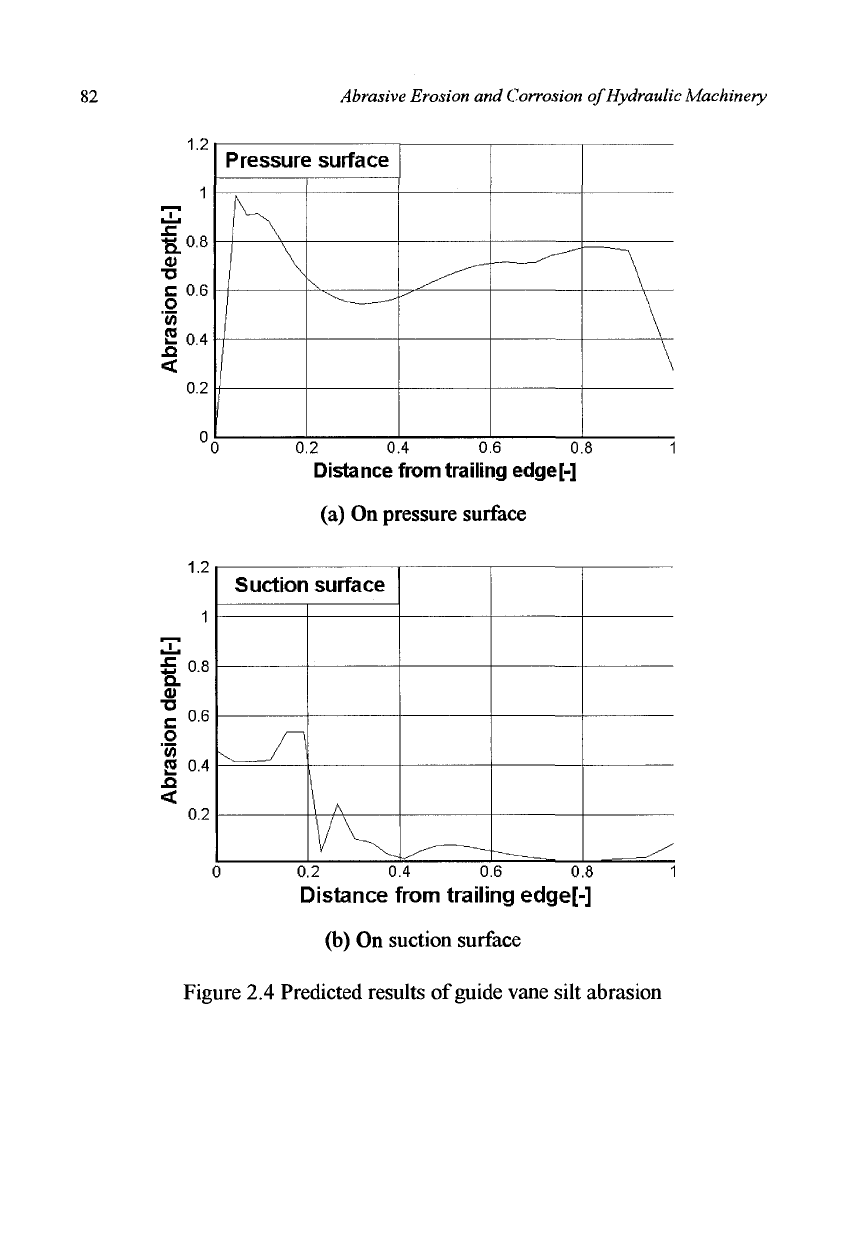
work. And Figure 2.4 (a) and (b) are the predicted silt abrasion depth on the

pressure and suction surfaces of

the

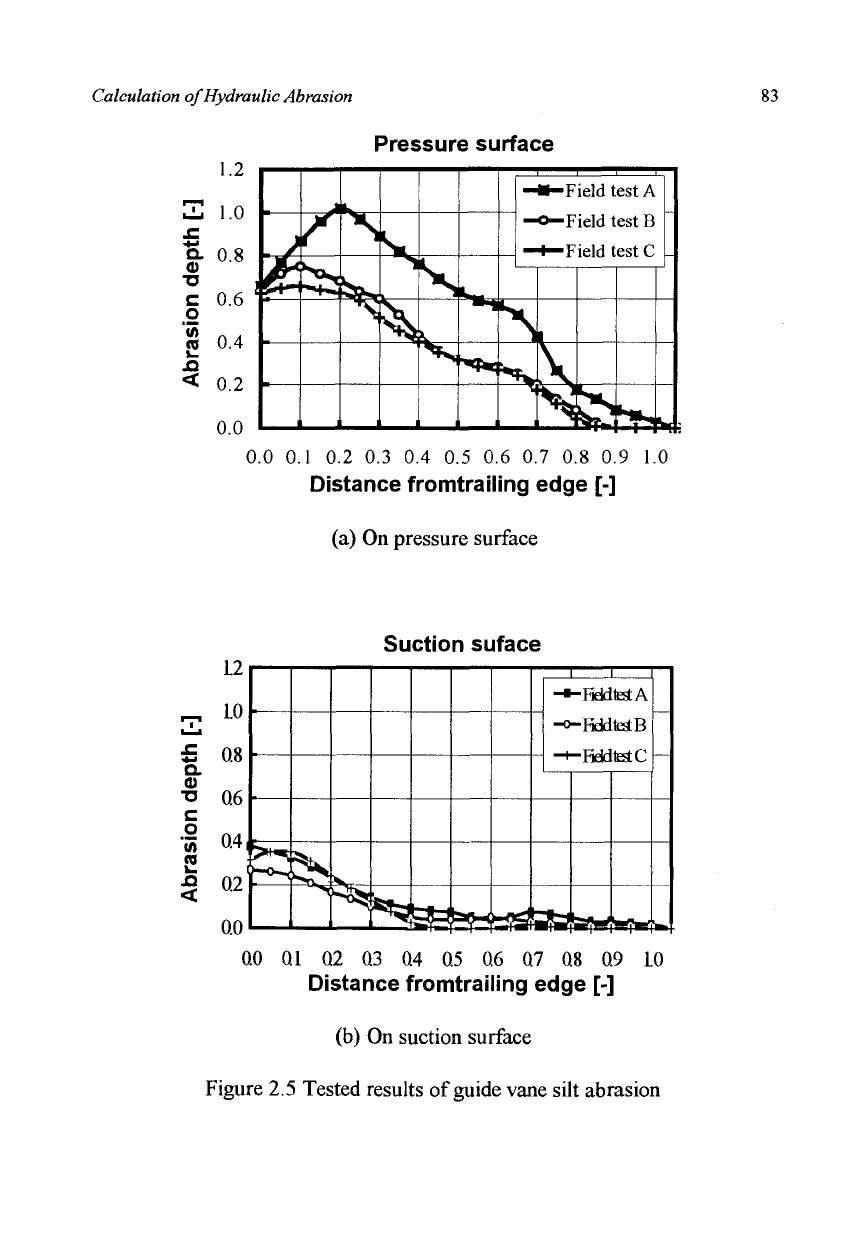
vane. The same depth and the field-tested

results by P. Dritina and M. Krause (1994) are shown in reference

[2.8].

The

same depth as those in Figure 2.4, as the field tested results by P. Dritina and

M. Krause (1994) are shown in Figure 2.5 (a) and (b). From the comparison

between the results, it is clear that the present computation for prediction of

silt abrasion is reasonable.

Abrasive Erosion and Corrosion of Hydraulic Machinery

0.388831

0.321362

0.253894

0.186425

0.118956

0.0514875

-0.0159813

-0.08345

-0.150919

-0.218388

-0.285856

-0.353325

-0.420794

-0.488263

-0.555731

(a) At the three-dimensional domain

1545,3

0.388831

0.321362

0.253894

0.186425

0.118956

0.0514875

-0.0159813

-0.08345

-0.150919

-0.218388

-0.285856

-0.353325

-0.420794

-0.488263

-0.555731

(b) On the middle plane of the domain

Figure 2.1 Pressure distribution through the vane passage

Calculation

Hydraulic Abrasion

20m/s

(al) Liquid phase on the middle plane

18.6469

17.4037

16.1606

14.9175

13.6744

12.4312

11.1881

9.945

8.70187

7.45875

6.21562

4.9725

3.72937

2.48625

1.24312

(a2) Liquid phase (isoline) on the middle plane

Abrasive Erosion and Corrosion of Hydraulic Machinery

20m/s

(bl) Particle phase on the middle plane

18.2068

17.0735

15.9403

14.807

13.6738

12.5405

11.4073

10.274

9.14075

8.0075

6.87425

5.741

4.60775

3.4745

2.34125

(b2) Particle phase (isoline) on the middle plane

Figure 2.2 Velocity distributions through the vane passage

Calculation of Hydraulic Abrasion

inlet

level

7.93518E-09

7.32479E-09

6.71439E-09

6.10399E-09

5.49359E-09

4.88319E-09

4.27279E-09

3.66239E-09

3.05199E-09

2.4416E-09

1.8312E-09

1.2208E-09

6.10399E-10

(a) Abrasion on suction side

inlet

outlet

level

9.15621

E-09

8.54603E-09

7.93586E-09

7.32569E-09

6.71551

E-09

6.10534E-09

5.49516E-09

4.88499E-09

4.27482E-09

3.66464E-09

3.05447E-09

2.4443E-09

1.83412E-09

1.22395E-09

6.13776E-10

(b) Abrasion on pressure side

Figure 2.3 Abrasion rate on the vane surfaces

Abrasive Erosion and Corrosion of Hydraulic Machinery

Pressure surface

0.2 0.4 0.6 0.:

Distance from trailing edge[-]

(a) On pressure surface

Suction surface

0.2 0.4 0.6 0.8

Distance from trailing edge[-]

(b) On suction surface

Figure 2.4 Predicted results of guide vane silt abrasion

Calculation

Hydraulic Abrasion

1.2

1.0

■D

0.6 '

A A

flj

0.4

0.2

0.0

Pressure surface

M Field test A

—o—

Field test B

—+—Field test C

Sf*.

-2t!fc

0.0

0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0

Distance fromtrailing edge

[-]

(a) On pressure surface

Suction suface

1—1

•a

'55

0.8

0.6

0.4

0.2

0.0

V-J

w£

-0

-FiddtetA

-BddtetB

-RddtestC

tew

Mto

—

0.0

Ql 02 03 04 0.5 0.6 07 08 0.9 LO

Distance fromtrailing edge

[-]

(b) On suction surface

Figure 2.5 Tested results of guide vane silt abrasion