Dionne G.F. Magnetic Oxides

Подождите немного. Документ загружается.

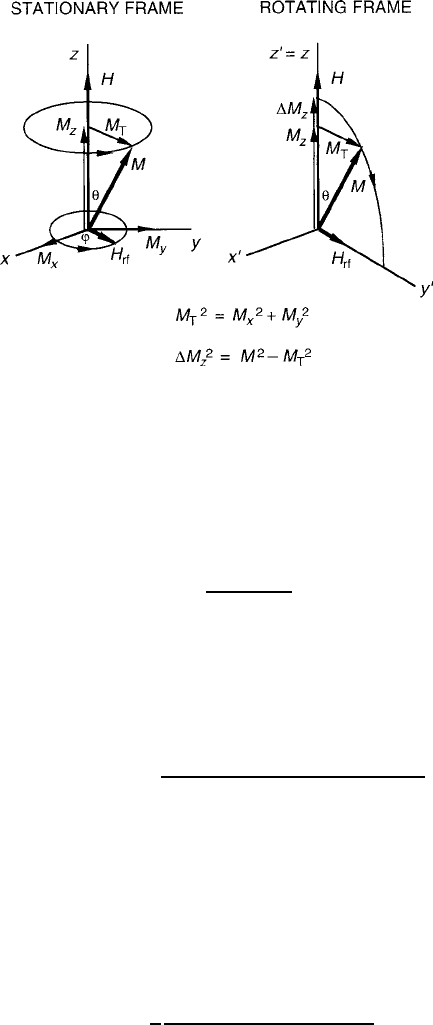
312 6 Electromagnetic Properties

Fig. 6.21 Reduction in M

under the inﬂuence of the torque created by a large H

,asviewedby

stationary and rotating frames of reference

To introduce analytically the nonlinear dependence of spin waves on larger rf

magnetic ﬁeld strengths, we begin by following the reasoning of Anderson and Suhl

[67] that the value of M

decreases when M is rotated from the z-axis by the torque

provided by the transverse H

ﬁeld, so that

 M

; (6.80)

where M

D M

C M

as seen from the Pythagorean vector triangle in Fig. 6.21.

In terms of the rf susceptibility for the circular polarization mode that produces

resonance, as deﬁned by (1.84)and(6.54), M

D 

C H

where



D 

C 



.M /

1 C 

.H  N

4M

 !/

; (6.81)

if we ignore the small 



term in the denominator. Note also that the reduc-

tion of the applied ﬁeld H from the z-axis demagnetizing ﬁeld N

4M

in a planar

disc magnetized normal to its surface (N

 1) is now included in the resonance

term. This geometry was chosen to emphasize the effect of H

on the rotation of

M from the z-axis.

From (6.138) in Appendix 6B, we repeat the relation that best illustrates the

instability:

4 M

4M .H

.N

4 M

C 

2

2k !0

: (6.82)

6.3 Exchange-Coupled Modes (Spin Waves) 313

The classical instability can be reasoned by envisioning perturbations of the M

component, deﬁned as M

. An increase in M

will reduce the right-hand side,

thereby producing a new value of M

. If the value is reduced, the system will relax

to equilibrium; if it increases, the value will diverge and the system will be unstable.

The solution of (6.82) produces the following relations at the critical condition:

N

4 M

crit

D 

1

2k!0

D H

k!0

(6.83)

and H

crit

D 

3=2

2k!0

.N

4M /

1=2

; (6.84)

or H

k!0



crit



2=3

4M /

1=3

: (6.85)

Note that the subscript k ! 0 has been added to 

and H (now without

subscript i) in these relations and in Appendix 6B to point out that the threshold

for spin-wave onset depends on the initial uniform precession values of the deco-

herence rate. It is these rates that must increase in order to restore equilibrium to the

precessing spin system.

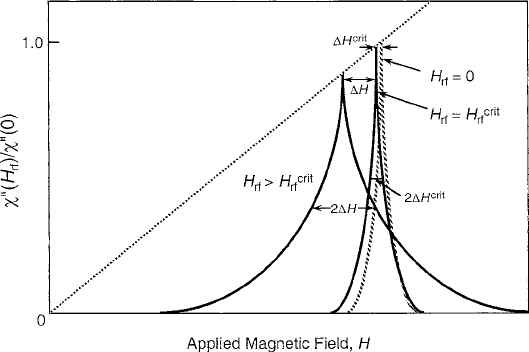
The above exercise reveals interesting effects where H

crit

: from (6.85)

H increases in direct proportion to H

2=3

, and from (6.22)and(6.24) the applied

magnetic ﬁeld H at the peak 

decreases by the amount H of the demagnetizing

ﬁeld, as sketched in Fig. 6.22. Because H is typically negligible compared to H or

4M , the shift in resonance is important only at or very near resonance. However,

the broadening of the line as it relates to increased rf loss away from resonance is of

great practical signiﬁcance, as discussed in Sect. 6.2. Because the increase in H

is reﬂected as a decrease in the peak value of 

for a Lorentzian line shape, we can

Fig. 6.22 Graphical sketch of the decline in 

, the growth of the linewidth H and its relation

to the downward shift in the resonance ﬁeld at a ﬁxed frequency, as the rf ﬁeld amplitude increases

above H

crit

314 6 Electromagnetic Properties

display the effect of the broadening on the value of 

/ normalized to 

.0/ by

merging (6.56) with (6.85), according to









.0/

peak

1 C



H

k!0



1

1 C



crit



2=3

.4M /

1=3

1

for H

 H

crit

(6.86)

1 C



4M



2=3

1



crit



2=3



crit



2=3

for H

 H

crit

;

for N

4M  H

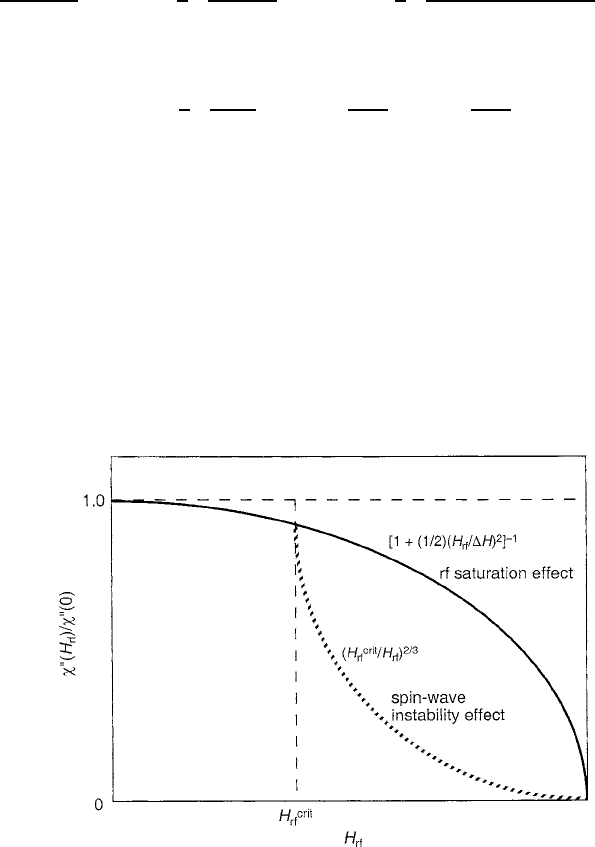
. From the sketch in Fig. 6.23, it can be seen how the generation

of spin waves inﬂuences the premature decline in the absorption peak and hence, the

attendant linewidth broadening.

Although the above model offers rationale for the spin-wave instability, the ac-

tual relations used in practical situations must be sensitive to the exchange coupling

among speciﬁc spin-wave modes. A more exact approach to the derivation of the

threshold conditions was developed by Suhl [68], in which the instability was found

to occur without a shift in the resonance ﬁeld. In this case, the restoration of equi-

librium is accounted for entirely by an increase in linewidth. As a consequence, the

Fig. 6.23 Graphical display of the effects on the peak 

below and above the critical ﬁeld H

crit

stated analytically in (6.91). The solid curve represents the saturation effect below H

crit

that is used

as a technique for measuring 

[4, 18]. The sharp decline of the dashed curve indicates how the

spin waves above H

crit

are broadening the linewidth

6.3 Exchange-Coupled Modes (Spin Waves) 315

relation of(6.20) between H and H

is linear, and the above-threshold part of

(6.25) would become



.0/

peak

crit

for H

crit

: (6.87)

6.3.3 Instability Threshold (Nonlinear Spin Waves)

The foregoingclassical model does not accurately represent the experimental reality.

It is included here to provide an introduction to the instability effect and the role

of demagnetizing ﬁelds that feedback any growth of the uniform precession angle,

thereby leading to a nonlinear increase in absorption. It cannot, for example, be

used to interpret the case of spherical specimens where the external demagnetizing

term is zero. This situation was addressed by Suhl [68] who reasoned that initial

demagnetizing effects of speciﬁc k>0spin waves themselves would destabilize

the uniform precession through variations in their exchange ﬁelds.

In contrast to the classical model in which the increased rf loss is viewed as

a transfer of energy to a collective spin-wave “bath,” the rigorous analysis treats

the spin waves or magnons as individuals of wave number k (see Fig. 6.20). The

computed results are in general accord with experiment. The uniform precession fre-

quency does not shift to lower values above the threshold as in the classical model,

but rather a second absorption peak [69] occurs as low as !

=2 .or H

=2/.This

effect is referred to as the subsidiary absorption, which is depicted schematically in

Fig. 6.24. The changes in  and M

as a function of H

were observed by Bloem-

bergen and Wang [70].

An important consideration is the threshold ﬁeld away from the resonance peak,

where many microwave devices are operated. As expected, the relation for the min-

imum threshold ﬁeld is frequency dependent. For a general shape

crit

.min/ D

2! .!  H C N

4M/

1

4M sin 

cos 

.!=2 C H  N

4M/

; (6.88)

where the angle 

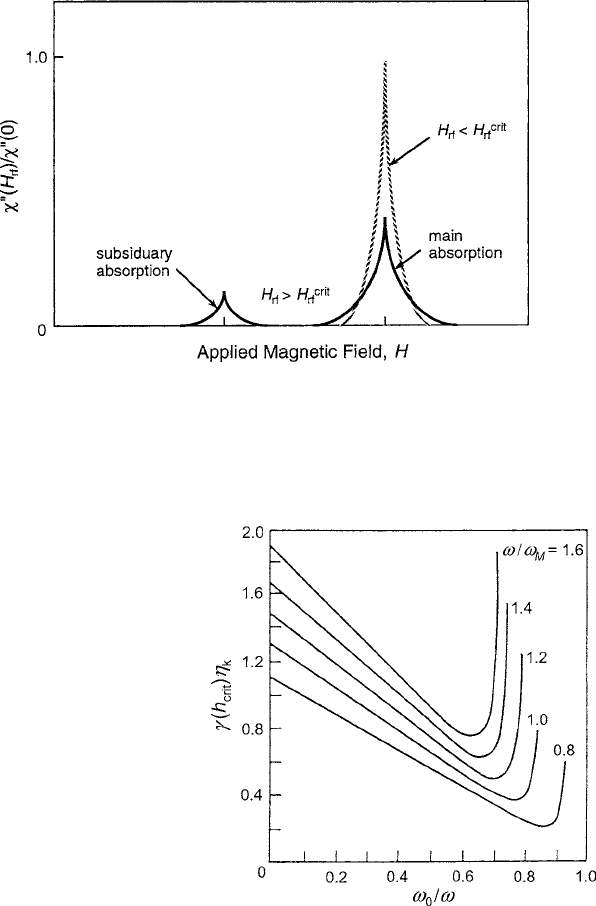
must fall somewhere between 0 and =2. From this relation,

the celebrated “butterﬂy” curves in Fig. 6.25 were constructed by Suhl for a spher-

ical geometry. Each point on any curve represents the critical ﬁeld for the onset of

exponential growth of an !

spin wave (magnon), with the minimum of the curve

occurring for the k ! 0 longest available wavelength, i.e., closest to uniform pre-

cession. This minimum condition is summarized by the relation

crit

.min/ D ˛H

k!0





; (6.89)

where ˛

1 is a proportionality parameter that is dependent on the particular con-

ditions chosen for the measurement, and H

k!0

is the spin-wave half-linewidth,

316 6 Electromagnetic Properties

Fig. 6.24 Graphical sketch of the appearance of the subsidiary absorption peak at a magnetic ﬁeld

below the main resonance ﬁeld, based on the theory of Suhl [69]. The subsidiary absorption is an

alternative loss mechanism to the classical broadening of the main resonance line and can appear

as low as one-half of the main resonance ﬁeld

Fig. 6.25 Butterﬂy curves

computed from (6.93)fora

specimen of spherical

geometry. Nomenclature

adjustments: h

crit

 H

crit

and 

 

1

. Minimum

values indicate the condition

for the onset of the subsidiary

absorption depicted in

Fig. 6.25, which appears in

the regime where H

i.e., the main resonance

frequency is less than the

signal frequency. Figure

reprinted from [68] with

permission.

 1956 by the

IEEE

which becomes the uniform-precession value in the limit of k D 0. A point worth

mentioning here is that H

crit

can be increased by raising the intrinsic linewidth

through the introduction of fast-relaxing impurities, e.g., rare-earth or Co

ions in

particular. In effect, the microwave energy accumulated in the uniform precession

6.3 Exchange-Coupled Modes (Spin Waves) 317

reservoir is being depleted, thereby delaying the onset of instabilities (see Figs. 6.13

and 6.20 and the discussion in Sect. 6.2.6). This trade-off between average power

loss and the more catastrophic effect of the nonlinear spin-wave generation is a tech-

nique used regularly in designing ferrite compounds for high-power applications.

Equation (6.89) is used to determine H

k!0

as a measure of the intrinsic spin–

lattice relaxation rate. With a polycrystalline specimen in which H is masked by

the inhomogeneous broadening discussed in Sect. 6.2.4, this technique has proven

to be of considerable value in estimating the peak-power capability of ceramic fer-

rites. Schloemann et al. [64] determined that the nonlinear threshold could also be

observed if the rf ﬁeld was applied parallel to H instead of transverse as Suhl con-

sidered. Subsequently, Patton examined the case of the general angle for linear and

circular (both Larmor and anti-Larmor) polarizations [71].

6.3.4 Magnetostatic Modes

In the foregoing discussion of spin waves, inﬁnite specimen dimensions were as-

sumed. From (6.76)thek

dependence on the spin-wave energy implies that long

wavelengths are not likely to be signiﬁcant. For typical values of !

in magnetic

oxides which reach toward 10

Hz, 

might be expected to be less that 100 lattice

parameter lengths. Therefore, the curves deﬁning the limits of the spin-wave mani-

fold in Fig. 6.19, include a cross-hatched region as k ! 0 for which spin waves are

inconsequential. For specimens of ﬁnite dimensions, however, additional “uniform”

precession modes can occur where (1) demagnetizing ﬁelds produce nonuniform

internal ﬁelds, particularly near surface boundaries and (2) where the rf signal mag-

netic ﬁeld is nonuniform within the specimen. The former case occurs when the

geometry is nonellipsoidal and is important in practical situations involving pla-

nar geometries. A classic example of this effect was reported by Dillon [72]. The

second instance is of concern in waveguides where rf modes provide variable ﬁeld

directions. The most striking example of this condition is the internal magnetostatic

(Walker) modes of a YIG sphere [73] that are described the general theory of mag-

netostatic modes in several texts [74–77].

To remain within the scope of this text, the topics of spin waves and magne-

tostatic waves will not be explored beyond these basic concepts. The interested

reader is encouraged to delve further into the subtleties of instability thresholds

and subsidiary absorption peaks in the various textbooks and reviews on the sub-

ject of microwave magnetism. Spin waves themselves are also vehicles for studying

fundamental physical phenomena of magnetic systems, including the generation and

propagationof microwave magnetic envelope(MME) solitons. The interested reader

is encouraged to consult the pioneering work of Chen et al. [78] and the results of

other investigators in this ﬁeld.

318 6 Electromagnetic Properties

6.4 Permeability and Propagation

Electromagnetic wave interactions with magnetic materials are important practi-

cal as well as fundamental areas of interest. In previous sections, nonresonance

(longitudinal H

 M

) and gyromagnetic resonance (transverse H

 M

) cases

were introduced in classical terms that involved paramagnetic or what amounts to

single-domain ferromagnetic systems. In many practical cases, the medium is a

polycrystalline ferrite that is operated below the longitudinal dispersion frequency

illustrated in Fig. 6.1 for maximum permeability, or above it for rf control applica-

tions such as Faraday rotation and phase shift near ferrimagnetic resonance. Despite

the fact that these two effects are independent and can occur in widely separated fre-

quency regions, any analytical simplicity that might be assumed disappears quickly

once the single-domain assumption is invalidated by the appearance of multiple do-

mains in partially magnetized materials [79,80].

6.4.1 Low-Frequency Longitudinal Permeability

When the medium is not fully magnetized, the magnetization can be divided into do-

mains in which the individual vectors are aligned according to local crystallographic

“easy” axes. In polycrystals, the distribution can occur as individual single-domain

grains if they are too small to support the formation of domain walls. As illustrated

in Fig. 6.26,theH

ﬁelds that ﬁx the directions of the M

vectors are set by the

orientation of the grains themselves, with or without domain walls. In either case,

propagating rf magnetic ﬁelds will be subject to longitudinal damping effects.

Since there is no resultant of the combined saturation M

vectors in an unsatu-

rated state, there is no reference direction by which longitudinal and transverse drive

ﬁelds can be distinguished. Where low anisotropy and ideal microstructure provide

negligible impediments to reversible wall movement, H

can exceed the coerciv-

ity ﬁeld H

thereby allowing domain wall movement to enhance the longitudinal

susceptibility. Furthermore, domain walls can resonate as damped forced harmonic

oscillators at a characteristic frequency related to the spin–lattice relaxation rate.

At higher frequencies approaching the microwave bands, gyromagnetism will occur

through transverse coupling about internal demagnetizing ﬁelds that originate in

part from poles on the surfaces of the walls. A result of this frequency dependence

is that absorption occurs beginning at about 1 MHz for longitudinal components of

rf drive ﬁelds and can reach into the GHz range for the transverse gyromagnetic

effects. These coexisting phenomena are contrasted in Fig. 6.1.

The basic susceptibility in the unmagnetized state is inﬂuenced by three princi-

pal factors. First, the magnitude of the initial susceptibility 

, which is determined

by the ease of magnetization rotation within the randomly oriented domains by

the H

 M

interaction in the absence of domain walls. Because the maximum

switching or hysteresis-loop coercive ﬁeld of a single-domain specimen is H



M

in simple linear terms. Second, when domain walls are present, an

6.4 Permeability and Propagation 319

Fig. 6.26 Two-dimensional sketches contrasting saturation magnetization vectors in a large mul-

tidomain single-crystal grain and an aggregate of small single-domain grains under the inﬂuence

of local H

ﬁelds. Figure reprinted from [80] with permission.

 2003 by the IEEE

enhanced susceptibility from reversible wall movement 

is obtained as the re-

duced coercive ﬁeld H

 H

allows 

M

. Third, the spectral character of

the resulting permeability is also determined by the lowest frequency !

at which

transverse H

 M

FMR interactions occur through the gyromagnetic conver-

sion constant . A further concern involves the highest frequency above which the

gyromagnetic effects inﬂuence permeability.

For a specimen of cubic lattice symmetry with K

<0the initial permeability

from rotation is expressed in the simplest terms by (5.50) as



D 1 C 4



2M

: (6.90)

For single-domain specimens, a dispersive decline of 

from its initial value can be

viewed as analogous to the paramagnetic system described in Sect. 6.1.1. The dis-

persion of the complex longitudinal  is determined by the nonresonant relaxation

rate !



D 1=,where is the effective spin–lattice relaxation time.

When unimpeded by microstructural defects and high anisotropy, the more dom-

inant mechanism for producing high 

in all but the infrequent occurrence of

single-domain particles is domain wall movement and resonance. In Appendix 6C,

the frequency dependence of susceptibility 

is derived for the harmonic motion

of domain walls according to

R







P

C !



.2M

cos .!t/ : (6.91)

320 6 Electromagnetic Properties

where the square of the wall resonance angular frequency !

D ˛

.8=3/ 

, the domain wall mass per unit area m

D .1=4/ 

2

=A, 

is the domain wall damping time constant, and &

is the total wall

surface area per unit volume for the case of K

<0. It should be noted that !

dependent jointly on the wall exchange energy A (hence the Curie temperature), the

anisotropy, and the disposition and density of the domain conﬁguration.

The susceptibility from reversible wall movement is deduced directly from the

solution of (6.86), according to [81]



.2M



 !



C .1=

cos .!t  /; (6.92)

where

tan  D



 !

It can be easily shown that the maximum frequency !

to avoid critical damping of

the resonance is then given by the minimum of the denominator of (6.92), which

becomes

D !



2

: (6.93)

Since !

already increases with

and 

, we now conclude that the thresh-

old for the collapse of 

is also dependent on 

, which requires large values to

allow the forced oscillation to remain below the critical damping limit of (6.93).

For the value of 

at ! D 0, other useful relations can be deduced from (6.92).

Since ˛

can also be expressed as 4M

=3

from Appendix 6C, we can introduce

the single-domain 

to obtain



.0/ D

D 3

 12

: (6.94)

As a consequence, an increase in 

of a factor between 30 and 40 over the mag-

netization rotation value could be expected from reversible domain wall movement.

Moreover, at ! D !

a peak in 

occurs, with an increase over the ! D 0 value as

estimated from the amplitude of (6.92), according to



.0/



 1=4

 !



.for !



 1/ : (6.95)

Note that the denominator of (6.95) is also the condition for the onset of critical

damping of the unforced oscillator .at H

D 0/. Above this frequency, the mate-

rial assumes single-domain character and the advantages to permeability from wall

movement are lost. Experimental evidence of this transition is already revealed in

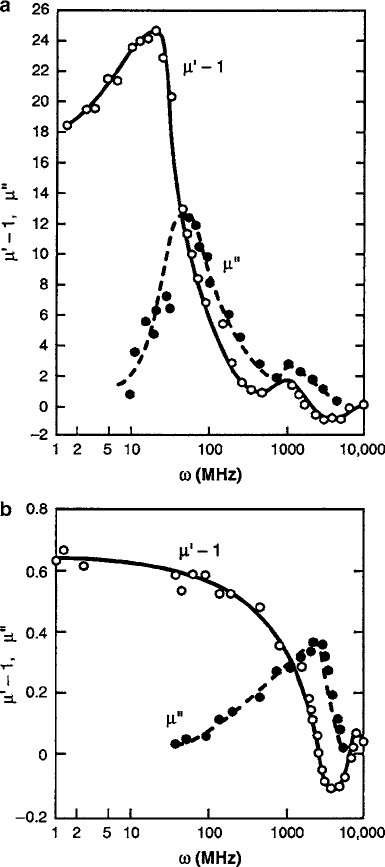
6.4 Permeability and Propagation 321

Fig. 6.27 Permeability

spectra contrasting (a) a solid

multidomain magnesium

ferrite-based ceramic with

(b) a 70% (by weight)

mixture of single-domain

particles of the same

compound dispersed in wax.

Original data are from Rado

et al. [82]. Figure reprinted

from [80] with permission.

 2003 by the IEEE

the early data of Rado et al. [82] shown in Fig. 6.27, where single- and multiple-

domain behavior are contrasted. In either case, resonance from transverse compo-

nents is reached at the microwave bands .>1 GHz/, which can sometimes overlap

the longitudinal dispersion region if !



is large enough.