Daniel W.W. Biostatistics: A Foundation for Analysis in the Health Sciences

Подождите немного. Документ загружается.

Can one conclude from these data that the sampled population is not normally distributed with a

mean of 1050 and a standard deviation of 50? Determine the p value for this test.

13.7.2 IQs of a sample of 30 adolescents arrested for drug abuse in a certain metropolitan jurisdiction

were as follows:

95 100 91 106 109 110

98 104 97 100 107 119

92 106 103 106 105 112

101 91 105 102 101 110

101 95 102 104 107 118

Do these data provide sufﬁcient evidence that the sampled population of IQ scores is not normally

distributed with a mean of 105 and a standard deviation of 10? Determine the p value.

13.7.3 For a sample of apparently normal subjects who served as controls in an experiment, the follow-

ing systolic blood pressure readings were recorded at the beginning of the experiment:

162 177 151 167

130 154 179 146

147 157 141 157

153 157 134 143

141 137 151 161

Can one conclude on the basis of these data that the population of blood pressures from which

the sample was drawn is not normally distributed with and Determine the p

value.

13.8 THE KRUSKAL–WALLIS ONE-WAY

ANALYSIS OF VARIANCE BY RANKS

In Chapter 8 we discuss how one-way analysis of variance may be used to test the null

hypothesis that several population means are equal. When the assumptions underlying

this technique are not met, that is, when the populations from which the samples are

drawn are not normally distributed with equal variances, or when the data for analysis

consist only of ranks, a nonparametric alternative to the one-way analysis of variance

may be used to test the hypothesis of equal location parameters. As was pointed out in

Section 13.5, the median test may be extended to accommodate the situation involving

more than two samples. A deﬁciency of this test, however, is the fact that it uses only a

small amount of the information available. The test uses only information as to whether

or not the observations are above or below a single number, the median of the combined

samples. The test does not directly use measurements of known quantity. Several

nonparametric analogs to analysis of variance are available that use more information by

s = 12?m = 150

13.8 THE KRUSKAL–WALLIS ONE-WAY ANALYSIS OF VARIANCE BY RANKS 717

taking into account the magnitude of each observation relative to the magnitude of every

other observation. Perhaps the best known of these procedures is the Kruskal–Wallis one-

way analysis of variance by ranks (8).

The Kruskal–Wallis Procedure The application of the test involves the

following steps.

1. The observations from the k samples are combined into a single

series of size n and arranged in order of magnitude from smallest to largest.

The observations are then replaced by ranks from 1, which is assigned to the small-

est observation, to n, which is assigned to the largest observation. When two or

more observations have the same value, each observation is given the mean of the

ranks for which it is tied.

2. The ranks assigned to observations in each of the k groups are added separately to

give k rank sums.

3. The test statistic

(13.8.1)

is computed. In Equation 13.8.1,

4. When there are three samples and ﬁve or fewer observations in each sample, the

signiﬁcance of the computed H is determined by consulting Appendix Table N.

When there are more than ﬁve observations in one or more of the samples, H is

compared with tabulated values of with degrees of freedom.

EXAMPLE 13.8.1

In a study of pulmonary effects on guinea pigs, Lacroix et al. (A-7) exposed ovalbu-

min (OA)-sensitized guinea pigs to regular air, benzaldehyde, or acetaldehyde. At the

end of exposure, the guinea pigs were anesthetized and allergic responses were

assessed in bronchoalveolar lavage (BAL). One of the outcome variables examined

was the count of eosinophil cells, a type of white blood cell that can increase with

allergies. Table 13.8.1 gives the eosinophil cell count for the three treatment

groups.

Can we conclude that the three populations represented by the three samples dif-

fer with respect to eosinophil cell count? We can so conclude if we can reject the null

hypothesis that the three populations do not differ in eosinophil cell count.

1*10

k - 1x

= the sum of the ranks in the jth sample

n = the number of observations in all samples combined

= the number of observations in the jth sample

k = the number of samples

H =

n1n + 12

j =1

- 31n + 12

, n

, . . . , n

718 CHAPTER 13 NONPARAMETRIC AND DISTRIBUTION-FREE STATISTICS

Solution:

1. Data. See Table 13.8.1.

2. Assumptions. The samples are independent random samples from

their respective populations. The measurement scale employed is at least

ordinal. The distributions of the values in the sampled populations are

identical except for the possibility that one or more of the populations

are composed of values that tend to be larger than those of the other

populations.

3. Hypotheses.

than at least one of the other populations.

Let

4. Test statistic. See Equation 13.8.1.

5. Distribution of test statistic. Critical values of H for various sample

sizes and levels are given in Appendix Table N.

6. Decision rule. The null hypothesis will be rejected if the computed

value of H is so large that the probability of obtaining a value that large

or larger when is true is equal to or less than the chosen signiﬁcance

level,

7. Calculation of test statistic. When the three samples are combined into

a single series and ranked, the table of ranks shown in Table 13.8.2 may

be constructed.

The null hypothesis implies that the observations in the three sam-

ples constitute a single sample of size 15 from a single population. If

this is true, we would expect the ranks to be well distributed among the

three groups. Consequently, we would expect the total sum of ranks to

be divided among the three groups in proportion to group size. Departures

a = .01.

: At least one of the populations tends to exhibit larger values

: The population centers are all equal.

13.8 THE KRUSKAL–WALLIS ONE-WAY ANALYSIS OF VARIANCE BY RANKS 719

TABLE 13.8.1 Eosinophil Count for

Ovalbumin-Sensitized Guinea Pigs

Eosinophil Cell Count

Air Benzaldehyde Acetaldehyde

12.22 3.68 54.36

28.44 4.05 27.87

28.13 6.47 66.81

38.69 21.12 46.27

54.91 3.33 30.19

Source: G. Lacroix. Used with permission.

(:10

)

from these conditions are reﬂected in the magnitude of the test statis-

tics H.

From the data in Table 13.8.2 and Equation 13.8.1, we obtain

8. Statistical decision. Table N shows that when the are 5, 5, and 5,

the probability of obtaining a value of is less than .009. The

null hypothesis can be rejected at the .01 level of signiﬁcance.

9. Conclusion. We conclude that there is a difference in the average

eosinophil cell count among the three populations.

10. p value. For this test, ■

Ties When ties occur among the observations, we may adjust the value of H by

dividing it by

(13.8.2)

where The letter t is used to designate the number of tied observations in a

group of tied values. In our example there are no groups of tied values but, in general,

there may be several groups of tied values resulting in several values of T.

The effect of the adjustment for ties is usually negligible. Note also that the effect

of the adjustment is to increase H, so that if the unadjusted H is signiﬁcant at the cho-

sen level, there is no need to apply the adjustment.

More than Three Samples/Large Samples Now let us illustrate the

procedure when there are more than three samples and at least one of the is greater

than 5.

T = t

- t.

1 -

- n

p 6 .009.

H = 9.14

H =

151162

1472

1162

1572

d- 3115 + 12= 9.14

720 CHAPTER 13 NONPARAMETRIC AND DISTRIBUTION-FREE STATISTICS

TABLE 13.8.2 The Data of Table 13.8.1 Replaced

by Ranks

Air Benzaldehyde Acetaldehyde

5213

93 7

8415

11 6 1 2

14 1 10

= 57R

= 16R

= 47

13.8 THE KRUSKAL–WALLIS ONE-WAY ANALYSIS OF VARIANCE BY RANKS 721

TABLE 13.8.3 Net Book Value of Equipment per Bed by Hospital Type

Type Hospital

ABCDE

$1735(11) $5260(35) $2790(20) $3475(26) $6090(40)

1520(2) 4455(28) 2400(12) 3115(22) 6000(38)

1476(1) 4480(29) 2655(16) 3050(21) 5894(37)

1688(7) 4325(27) 2500(13) 3125(23) 5705(36)

1702(10) 5075(32) 2755(19) 3275(24) 6050(39)

2667(17) 5225(34) 2592(14) 3300(25) 6150(41)

1575(4) 4613(30) 2601(15) 2730(18) 5110(33)

1602(5) 4887(31) 1648(6)

1530(3) 1700(9)

1698(8)

= 264R

= 159R

= 124R

= 246R

= 68

EXAMPLE 13.8.2

Table 13.8.3 shows the net book value of equipment capital per bed for a sample of

hospitals from each of ﬁve types of hospitals. We wish to determine, by means of the

Kruskal–Wallis test, if we can conclude that the average net book value of equipment

capital per bed differs among the ﬁve types of hospitals. The ranks of the 41 values,

along with the sum of ranks for each sample, are shown in the table.

Solution: From the sums of the ranks we compute

Reference to Appendix Table F with degrees of freedom indi-

cates that the probability of obtaining a value of H as large as or larger

than 36.39, due to chance alone, when there is no difference among the

populations, is less than .005. We conclude, then, that there is a difference

among the ﬁve populations with respect to the average value of the vari-

able of interest. ■

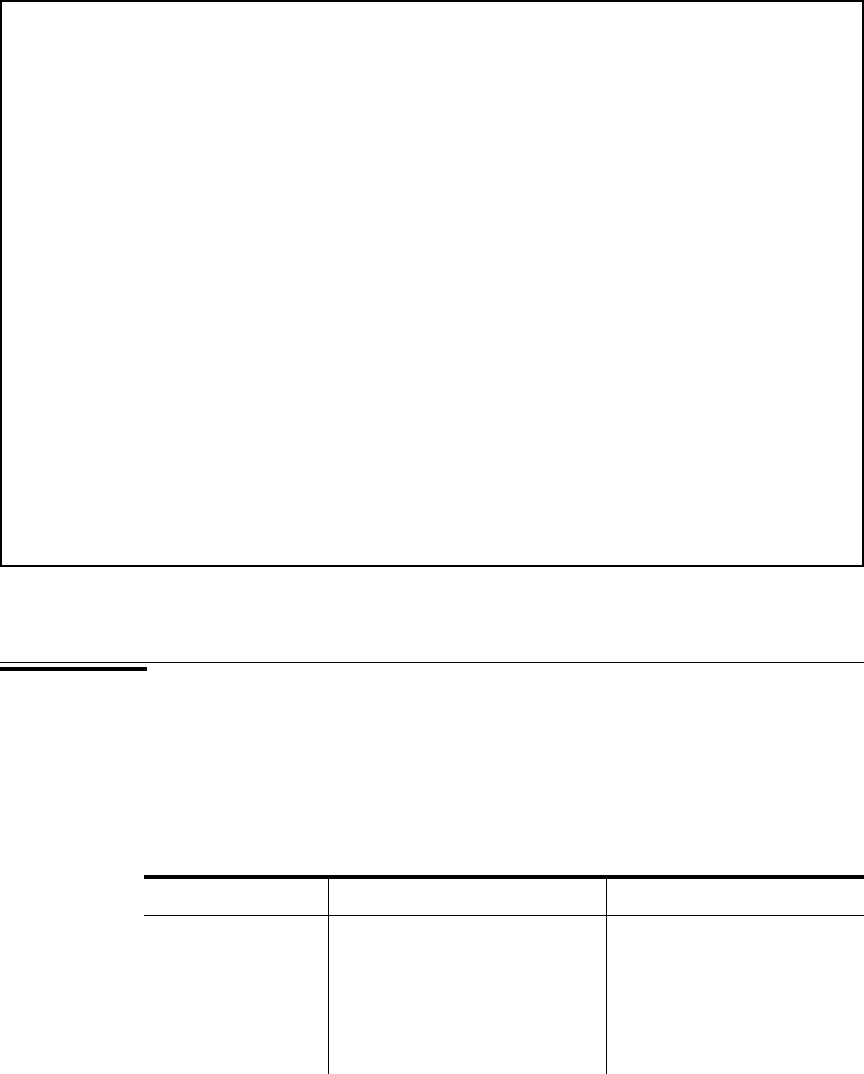
Computer Analysis The MINITAB software package computes the Kruskal–

Wallis test statistic and provides additional information. After we enter the eosinophil

counts in Table 13.8.1 into Column 1 and the group codes into Column 2, the MINITAB

procedure and output are as shown in Figure 13.8.1.

k - 1 = 4

= 36.39

H =

41141 + 12

1682

12462

11242

11592

12642

d - 3141 + 12

EXERCISES

For the following exercises, perform the test at the indicated level of signiﬁcance and determine

the p value.

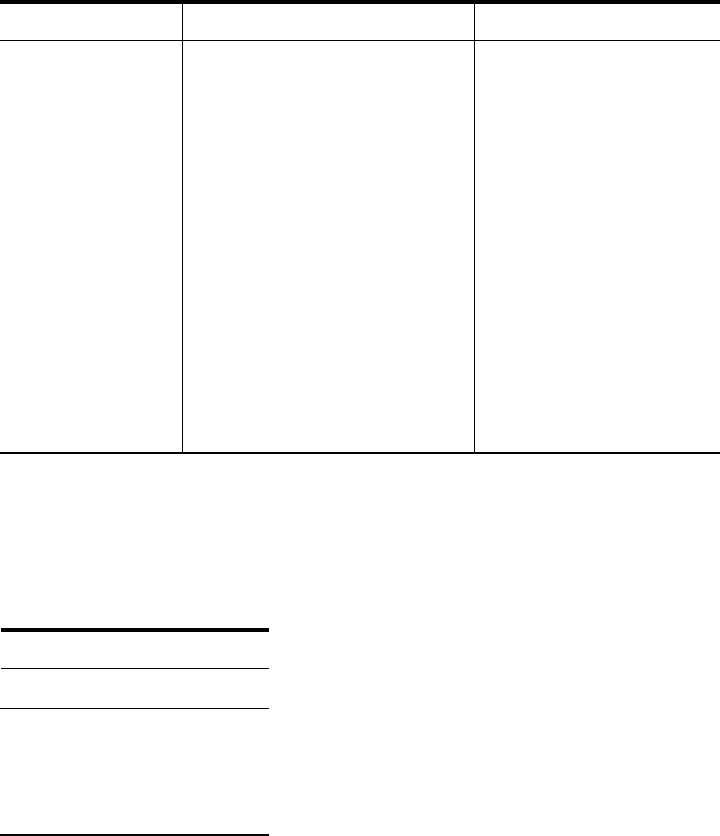
13.8.1 In a study of healthy subjects grouped by age (Younger: 19–50 years, Seniors: 65–75 years,

and Longeval: 85–102 years), Herrmann et al. (A-8) measured their vitamin B-12 levels (ng/L).

All elderly subjects were living at home and able to carry out normal day-to-day activities. The

following table shows vitamin B-12 levels for 50 subjects in the young group, 92 seniors, and

90 subjects in the longeval group.

Young (19–50 Years) Senior (65–75 Years) Longeval (85–102 Years)

230 241 319 371 566 170 148 149 631 198

477 442 190 460 290 542 1941 409 305 321

561 491 461 440 271 282 128 229 393 2772

347 279 163 520 308 194 145 183 282 428

566 334 377 256 440 445 174 193 273 259

260 247 190 335 238 921 495 161 157 111

300 314 375 137 525 1192 460 400 1270 262

722 CHAPTER 13 NONPARAMETRIC AND DISTRIBUTION-FREE STATISTICS

Data:

C1: 12.22 28.44 28.13 38.69 54.91 3.68 4.05 6.47 21.12 3.33 54.36 27.87 66.81 46.27 30.19

C2: 1 11112222233333

Dialog box: Session command:

Stat ➤ Nonparametrics ➤ Kruskal–Wallis MTB > Kruskal–Wallis C1 C2.

Type C1 in Response and C2 in Factor. Click OK.

Output:

Kruskal–Wallis Test: C1 versus C2

Kruskal–Wallis Test on C1

C2 N Median Ave Rank Z

1 5 28.440 9.4 0.86

2 5 4.050 3.2 -2.94

3 5 46.270 11.4 2.08

Overall 15 8.0

H = 9.14 DF = 2 P = 0.010

FIGURE 13.8.1 MINITAB procedure and output, Kruskal–Wallis test of eosinophil count data in Table 13.8.1.

(Continued)

Young (19–50 Years) Senior (65–75 Years) Longeval (85–102 Years)

230 254 229 452 298 748 548 348 252 161

215 419 193 437 153 187 198 175 262 1113

260 335 294 236 323 350 165 540 381 409

349 455 740 432 205 1365 226 293 162 378

315 297 194 411 248 232 557 196 340 203

257 456 780 268 371 509 166 632 370 221

536 668 245 703 668 357 218 438 483 917

582 240 258 282 197 201 186 368 222 244

293 320 419 290 260 177 346 262 277

569 562 372 286 198 872 239 190 226

325 360 413 143 336 240 241 203

275 357 685 310 421 136 195 369

172 609 136 352 712 359 220 162

2000 740 441 262 461 715 164 95

240 430 423 404 631 252 279 178

235 645 617 380 1247 414 297 530

284 395 985 322 1033 372 474 334

883 302 170 340 285 236 375 521

Source: W. Herrmann and H. Schorr. Used with permission.

May we conclude, on the basis of these data, that the populations represented by these samples

differ with respect to vitamin B-12 levels? Let

13.8.2 The following are outpatient charges made to patients for a certain surgical procedure

by samples of hospitals located in three different areas of the country:

Area

I II III

$80.75 $58.63 $84.21

78.15 72.70 101.76

85.40 64.20 107.74

71.94 62.50 115.30

82.05 63.24 126.15

Can we conclude at the .05 level of signiﬁcance that the three areas differ with respect to the charges?

13.8.3 A study of young children by Flexer et al. (A-9) published in the Hearing Journal examines the

effectiveness of an FM sound ﬁeld when teaching phonics to children. In the study, children in a

classroom with no phonological or phonemic awareness training (control) were compared to a class

with phonological and phonemic awareness (PPA) and to a class that utilized phonological and

phonemic awareness training and the FM sound ﬁeld (PPA/FM). A total of 53 students from three

separate preschool classrooms participated in this study. Students were given a measure of phone-

mic awareness in preschool and then at the end of the ﬁrst semester of kindergarten. The improve-

ment scores are listed in the following table as measured by the Yopp–Singer Test of Phonemic

Segmentation.

1- $1002

a = .01.

EXERCISES 723

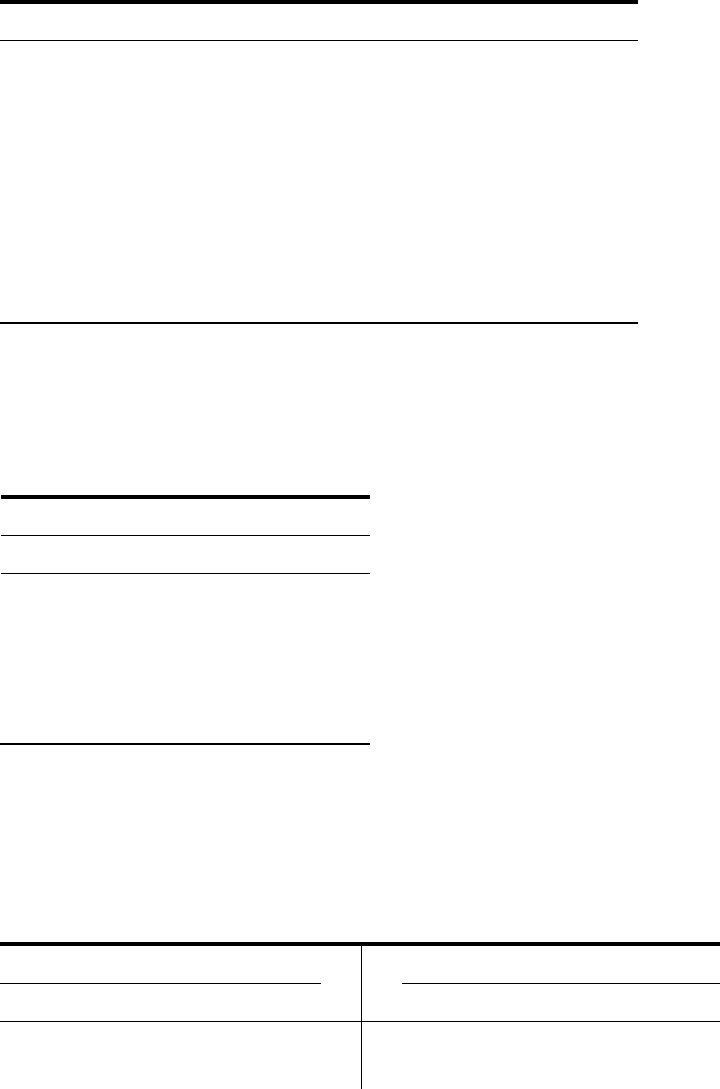
Improvement (Control) Improvement PPA Improvement PPA/FM

01 2 119

11 3 320

0 2 15 7 21

1 2 18 9 21

4 3 19 11 22

5 6 20 17 22

97 5 1715

9 8 17 17

13 9 18 17

18 18 18 19

020 1922

019

Source: John P. Holcomb, Jr., Ph.D. Used with permission.

Test for a signiﬁcant difference among the three groups. Let

13.8.4 Refer to Example 13.8.1. Another variable of interest to Lacroix et al. (A-7) was the number

of alveolar cells in three groups of subjects exposed to air, benzaldehyde, or acetaldehyde. The

following table gives the information for six guinea pigs in each of the three treatment groups.

Number of Alveolar Cells

Air Benzaldehyde Acetaldehyde

0.55 0.81 0.65

0.48 0.56 13.69

7.8 1.11 17.11

8.72 0.74 7.43

0.65 0.77 5.48

1.51 0.83 0.99

0.55 0.81 0.65

May we conclude, on the basis of these data, that the number of alveolar cells in ovalbumin-sensitized

guinea pigs differs with type of exposure? Let

13.8.5 The following table shows the pesticide residue levels (ppb) in blood samples from four popula-

tions of human subjects. Use the Kruskal–Wallis test to test at the .05 level of signiﬁcance the null

hypothesis that there is no difference among the populations with respect to average level of pes-

ticide residue.

Population Population

A BCDABCD

10 4 15 7 44 11 9 4

37 35 5 11 12 7 11 5

12 32 10 10 15 32 9 2

a = .05.

(:10

)

a = .05.

724 CHAPTER 13 NONPARAMETRIC AND DISTRIBUTION-FREE STATISTICS

Source: G. Lacroix. Used with permission.

(Continued)

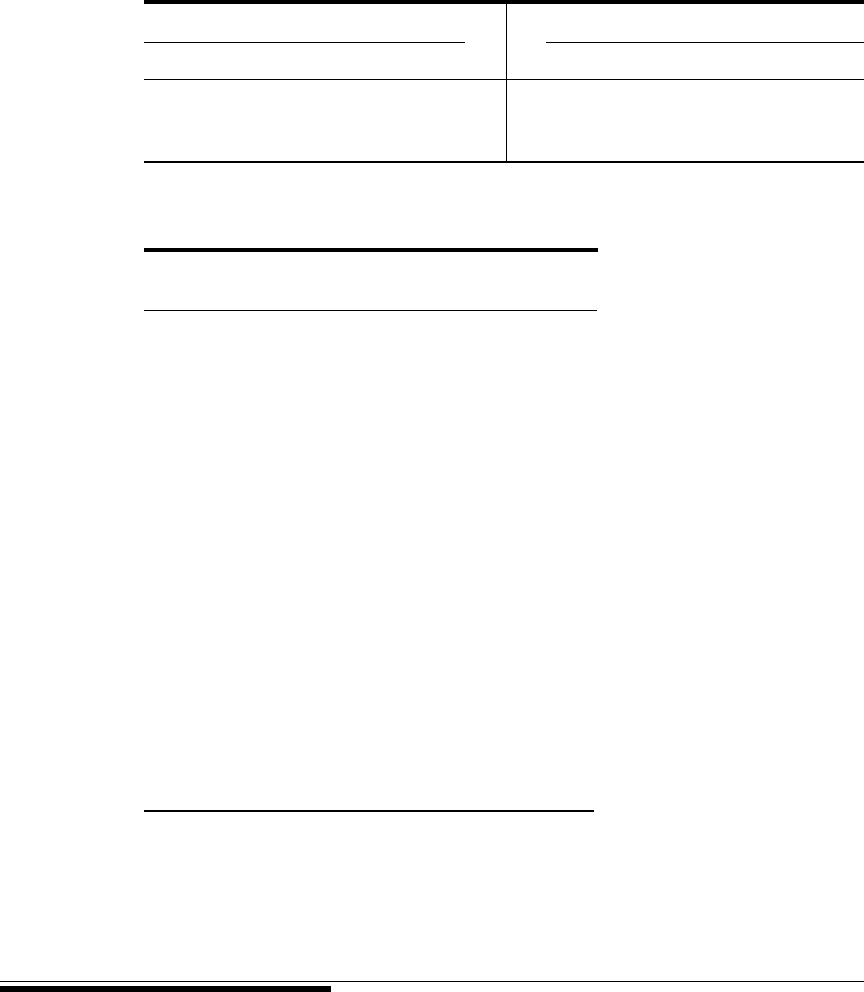
Population Population

A BCDABCD

31 19 12 8 42 17 14 6

11 33 6 2 23 8 15 3

91865

13.8.6 Hepatic -glutamyl transpeptidase (GGTP) activity was measured in 22 patients undergoing percu-

taneous liver biopsy. The results were as follows:

Hepatic

Subject Diagnosis GGTP Level

1 Normal liver 27.7

2 Primary biliary cirrhosis 45.9

3 Alcoholic liver disease 85.3

4 Primary biliary cirrhosis 39.0

5 Normal liver 25.8

6 Persistent hepatitis 39.6

7 Chronic active hepatitis 41.8

8 Alcoholic liver disease 64.1

9 Persistent hepatitis 41.1

10 Persistent hepatitis 35.3

11 Alcoholic liver disease 71.5

12 Primary biliary cirrhosis 40.9

13 Normal liver 38.1

14 Primary biliary cirrhosis 40.4

15 Primary biliary cirrhosis 34.0

16 Alcoholic liver disease 74.4

17 Alcoholic liver disease 78.2

18 Persistent hepatitis 32.6

19 Chronic active hepatitis 46.3

20 Normal liver 39.6

21 Chronic active hepatitis 52.7

22 Chronic active hepatitis 57.2

Can we conclude from these sample data that the average population GGTP level differs among

the ﬁve diagnostic groups? Let and ﬁnd the p value.

13.9 THE FRIEDMAN TWO-WAY ANALYSIS

OF VARIANCE BY RANKS

Just as we may on occasion have need of a nonparametric analog to the parametric one-

way analysis of variance, we may also ﬁnd it necessary to analyze the data in a two-way

classiﬁcation by nonparametric methods analogous to the two-way analysis of variance.

Such a need may arise because the assumptions necessary for parametric analysis of

variance are not met, because the measurement scale employed is weak, or because results

a = .05

13.9 THE FRIEDMAN TWO-WAY ANALYSIS OF VARIANCE BY RANKS 725

are needed in a hurry. A test frequently employed under these circumstances is the Fried-

man two-way analysis of variance by ranks (9, 10). This test is appropriate whenever the

data are measured on, at least, an ordinal scale and can be meaningfully arranged in a

two-way classiﬁcation as is given for the randomized block experiment discussed in Chap-

ter 8. The following example illustrates this procedure.

EXAMPLE 13.9.1

A physical therapist conducted a study to compare three models of low-volt electrical

stimulators. Nine other physical therapists were asked to rank the stimulators in order of

preference. A rank of 1 indicates ﬁrst preference. The results are shown in Table 13.9.1.

We wish to know if we can conclude that the models are not preferred equally.

Solution:

1. Data. See Table 13.9.1.

2. Assumptions. The observations appearing in a given block are inde-

pendent of the observations appearing in each of the other blocks, and

within each block measurement on at least an ordinal scale is achieved.

3. Hypothesis. In general, the hypotheses are:

The treatments all have identical effects.

At least one treatment tends to yield larger observations than

at least one of the other treatments.

For our present example we state the hypotheses as follows:

The three models are equally preferred.

The three models are not equally preferred.

Let a = .05.

726

CHAPTER 13 NONPARAMETRIC AND DISTRIBUTION-FREE STATISTICS

TABLE 13.9.1 Physical Therapists’ Rankings of

Three Models of Low-Volt Electrical Stimulators

Model

Therapist A B C

1231

2231

3231

4132

5321

6123

7231

8132

9132

15 25 14