Daniel W.W. Biostatistics: A Foundation for Analysis in the Health Sciences

Подождите немного. Документ загружается.

design than for a design in which different subjects are used for each occasion on which

measurements are made. Suppose, for example, that we have four treatments (in the usual

sense) or four points in time on each of which we would like to have 10 measurements.

If a different sample of subjects is used for each of the four treatments or points in time,

40 subjects would be required. If we are able to take measurements on the same sub-

ject for each treatment or point in time—that is, if we can use a repeated measures

design—only 10 subjects would be required. This can be a very attractive advantage if

subjects are scarce or expensive to recruit.

Disadvantages A major potential problem to be on the alert for is what is known

as the carry-over effect. When two or more treatments are being evaluated, the investi-

gator should make sure that a subject’s response to one treatment does not reﬂect a resid-

ual effect from previous treatments. This problem can frequently be solved by allowing

a sufﬁcient length of time between treatments.

Another possible problem is the position effect. A subject’s response to a treatment

experienced last in a sequence may be different from the response that would have

occurred if the treatment had been ﬁrst in the sequence. In certain studies, such as those

involving physical participation on the part of the subjects, enthusiasm that is high at the

beginning of the study may give way to boredom toward the end. A way around this

problem is to randomize the sequence of treatments independently for each subject.

Single-Factor Repeated Measures Design The simplest repeated

measures design is the one in which, in addition to the treatment variable, one additional

variable is considered. The reason for introducing this additional variable is to measure

and isolate its contribution to the total variability among the observations. We refer to

this additional variable as a factor.

DEFINITION

The repeated measures design in which one additional factor

is introduced into the experiment is called a single-factor repeated

measures design.

We refer to the additional factor as subjects. In the single-factor repeated measures

design, each subject receives each of the treatments. The order in which the subjects are

exposed to the treatments, when possible, is random, and the randomization is carried

out independently for each subject.

Assumptions The following are the assumptions of the single-factor repeated

measures design that we consider in this text. A design in which these assumptions are

met is called a ﬁxed-effects additive design.

1. The subjects under study constitute a simple random sample from a population of

similar subjects.

2. Each observation is an independent simple random sample of size 1 from each of

kn populations, where n is the number of subjects and k is the number of treat-

ments to which each subject is exposed.

8.4 THE REPEATED MEASURES DESIGN 347

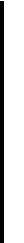
3. The kn populations have potentially different means, but they all have the same

variance.

4. The k treatments are ﬁxed; that is, they are the only treatments about which we

have an interest in the current situation. We do not wish to make inferences to

some larger collection of treatments.

5. There is no interaction between treatments and subjects; that is, the treatment and

subject effects are additive.

Experimenters may ﬁnd frequently that their data do not conform to the assumptions of

ﬁxed treatments and/or additive treatment and subject effects. For such cases the refer-

ences at the end of this chapter may be consulted for guidance.

In addition to the assumptions just listed, it should be noted that in a repeated-

measures experiment there is a presumption that correlations should exist among the

repeated measures. That is, measurements at time 1 and 2 are likely correlated, as are

measurements at time 1 and 3, 2 and 3, and so on. This is expected because the meas-

urements are taken on the same individuals through time.

An underlying assumption of the repeated-measures ANOVA design is that all of

these correlations are the same, a condition referred to as compound symmetry. This

assumption, coupled with assumption 3 concerning equal variances, is referred to as

sphericity. Violations of the sphericity assumption can result in an inﬂated type I error.

Most computer programs provide a formal test for the sphericity assumption along with

alternative estimation methods if the sphericity assumption is violated.

The Model The model for the ﬁxed-effects additive single-factor repeated meas-

ures design is

(8.4.1)

The reader will recognize this model as the model for the randomized complete block

design discussed in Section 8.3. The subjects are the blocks. Consequently, the notation,

data display, and hypothesis testing procedure are the same as for the randomized com-

plete block design as presented earlier. The following is an example of a repeated meas-

ures design.

EXAMPLE 8.4.1

Licciardone et al. (A-15) examined subjects with chronic, nonspecific low back pain.

In this study, 18 of the subjects completed a survey questionnaire assessing physi-

cal functioning at baseline, and after 1, 3, and 6 months. Table 8.4.1 shows the data

for these subjects who received a sham treatment that appeared to be genuine osteo-

pathic manipulation. Higher values indicate better physical functioning. The goal of

the experiment was to determine if subjects would report improvement over time

even though the treatment they received would provide minimal improvement. We

wish to know if there is a difference in the mean survey values among the four points

in time.

j = 1, 2, Á , ki = 1, 2, Á , n;

= m + b

+ t

348 CHAPTER 8 ANALYSIS OF VARIANCE

Solution:

1. Data. See Table 8.4.1.

2. Assumptions. We assume that the assumptions for the fixed-effects,

additive single-factor repeated measures design are met.

3. Hypotheses.

not all are equal

4. Test statistic.

5. Distribution of test statistic. F with numerator degrees of

freedom and denominator degrees of freedom.

6. Decision rule. Let . The critical value of F is 2.80 (obtained

by interpolation). Reject if computed V.R. is equal to or greater

than 2.80.

7. Calculation of test statistic. We use MINITAB to perform the calcula-

tions. We ﬁrst enter the measurements in Column 1, the row (subject)

codes in Column 2, the treatment (time period) codes in Column 3, and

proceed as shown in Figure 8.4.1.

a = .05

71 - 3 - 17 = 51

4 - 1 = 3

V.R. = treatment MS>error MS.

m’sH

: m

= m

8.4 THE REPEATED MEASURES DESIGN

349

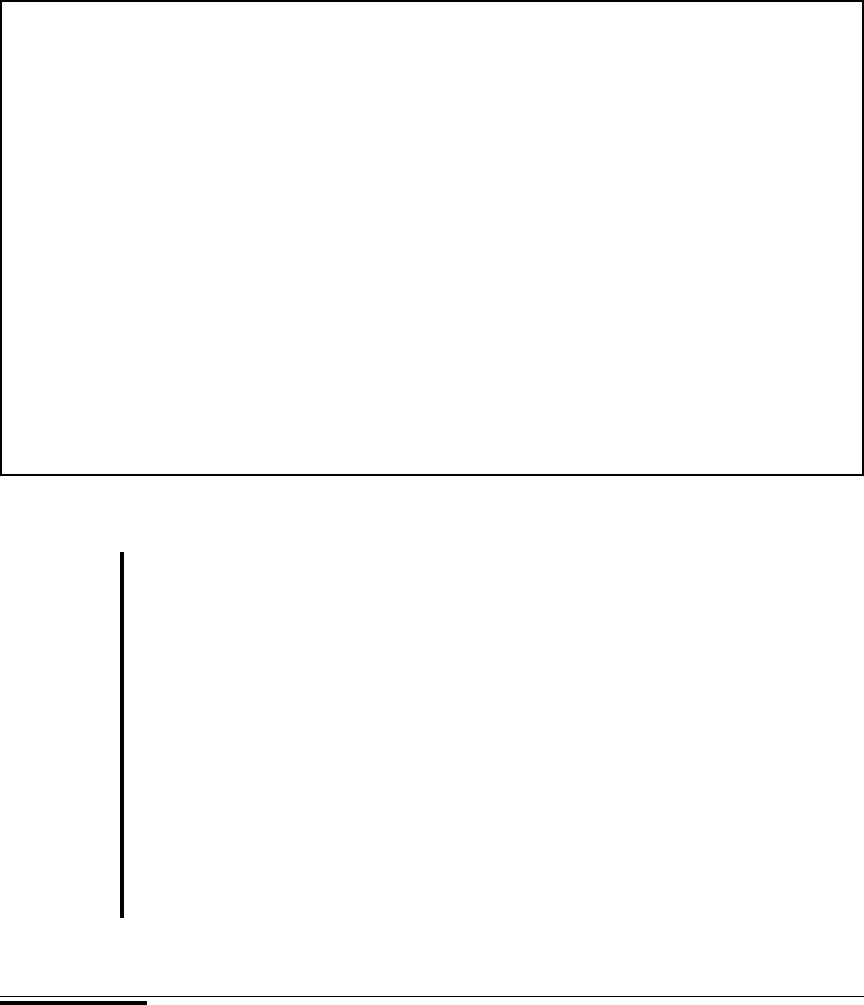
TABLE 8.4.1 SF-36 Health Scores at Four Different

Points in Time

Subject Baseline Month 1 Month 3 Month 6

1806095100

295909595

365555045

450457070

560758085

670707570

780808580

870607565

980807065

10 65 30 45 60

11 60 70 95 80

12 50 50 70 60

13 50 65 80 65

14 85 45 85 80

15 50 65 90 70

16 15 30 20 25

17 10 15 55 75

18 80 85 90 70

Source: John C. Licciardone. Used with permission.

8. Statistical decision. Since is greater than 2.80, we are able

to reject the null hypothesis.

9. Conclusion. We conclude that there is a difference in the four popula-

tion means.

10. p value. Since 5.50 is greater than 4.98, the F value for and

the p value is less than .005.

Figure 8.4.2. shows the SAS

output for the analysis of Example 8.4.1 and Figure 8.4.3

shows the SPSS output for the same example. Note that SPSS provides four potential

tests. The ﬁrst test is used under an assumption of sphericity and matches the outputs in

Figures 8.4.1 and 8.4.2. The next three tests are modifications if the assumption of

sphericity is violated. Note that SPSS modiﬁes the degrees of freedom for these three

tests, which changes the mean squares and the p values, but not the V. R. Note that the

assumption of sphericity was violated for these data, but that the decision rule did not

change, since all of the p values were less than

EXERCISES

For Exercises 8.4.1 to 8.4.3 perform the ten-step hypothesis testing procedure. Let

8.4.1 One of the purposes of a study by Liu et al. (A-16) was to determine the effects of MRZ 2兾579

on neurological deficit in Sprague-Dawley rats. In this study, 10 rats were measured at four time

a = .05.

df = 40,

a = .005

V.R. = 5.50

350

CHAPTER 8 ANALYSIS OF VARIANCE

Dialog box: Session command:

Stat ➤ ANOVA ➤ Twoway MTB > TWOWAY C1 C2 C3;

SUBC> MEANS C2 C3.

Type C1 in Response. Type C2 in Row factor and

Check Display means. Type C3 in Column factor and

Check Display means. Click OK.

Output:

Two-way ANOVA: C1 versus C2, C3

Analysis of Variance for C1

Source DF SS MS F P

C2 17 20238 1190 8.20 0.000

C3 3 2396 799 5.50 0.002

Error 51 7404 145

Total 71 30038

FIGURE 8.4.1 MINITAB procedure and output (ANOVA table) for Example 8.4.1.

■

EXERCISES 351

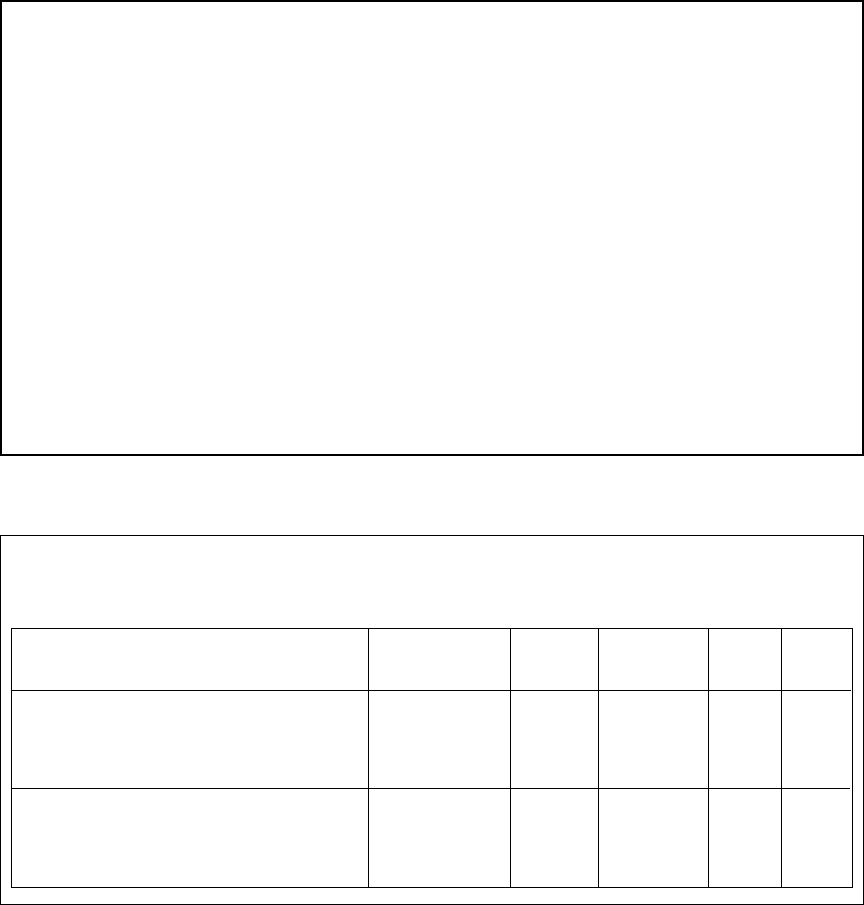
The ANOVA Procedure

Dependent Variable: sf36

Source DF Sum of Squares Mean Square F Value Pr > F

Model 20 22633.33333 1131.66667 7.79 <.0001

Error 51 7404.16667 145.17974

Corrected Total 71 30037.50000

R-Square Coeff Var Root MSE sf36 Mean

0.753503 18.18725 12.04906 66.25000

Source DF Anova SS Mean Square F Value Pr > F

subj 17 20237.50000 1190.44118 8.20 <.0001

time 3 2395.83333 798.61111 5.50 0.0024

FIGURE 8.4.2 SAS

output for analysis of Example 8.4.1.

FIGURE 8.4.3 SPSS output for the analysis of Example 8.4.1.

Tests of Within-Subjects Effects

Measure: MEASURE_1

Type III Sum Mean

Source of Squares df Square F Sig.

factor 1 Sphericity Assumed 2395.833 3 798.611 5.501 .002

Greenhouse-Geisser 2395.833 2.216 1080.998 5.501 .006

Huynh-Feldt 2395.833 2.563 934.701 5.501 .004

Lower-bound 2395.833 1.000 2395.833 5.501 .031

Error (factor 1) Sphericity Assumed 7404.167 51 145.180

Greenhouse-Geisser 7404.167 37.677 196.515

Huynh-Feldt 7404.167 43.575 169.919

Lower-bound 7404.167 17.000 435.539

periods following occlusion of the middle carotid artery and subsequent treatment with the

uncompetitive N-methly-D-aspartate antagonist MRZ 2兾579, which previous studies had sug-

gested provides neuroprotective activity. The outcome variable was a neurological function vari-

able measured on a scale of 0–12. A higher number indicates a higher degree of neurological

impairment.

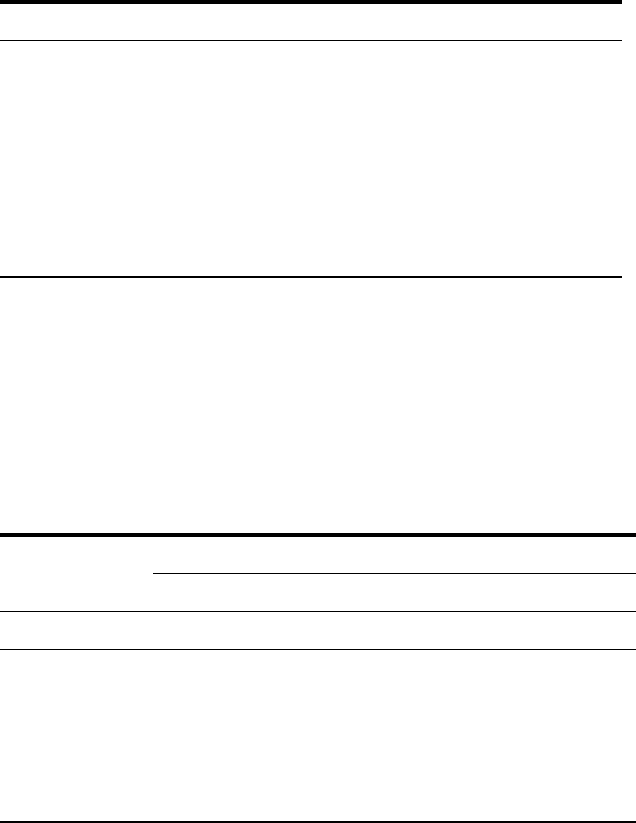
Rat 60 Minutes 24 Hours 48 Hours 72 Hours

111 9 8 4

211 7 5 3

311 10 8 6

411 4 3 2

511 10 9 9

611 6 5 5

711 6 6 6

811 7 6 5

911 7 5 5

10 11 9 7 7

Source: Ludmila Belayev, M.D. Used with permission.

8.4.2 Starch et al. (A-17) wanted to show the effectiveness of a central four-quadrant sleeve and screw

in anterior cruciate ligament reconstruction. The researchers performed a series of reconstructions

on eight cadaveric knees. The following table shows the loads (in newtons) required to achieve

different graft laxities (mm) for seven specimens (data not available for one specimen) using ﬁve

different load weights. Graft laxity is the separation (in mm) of the femur and the tibia at the

points of graft ﬁxation. Is there sufﬁcient evidence to conclude that different loads are required to

produce different levels of graft laxity? Let

Graft Laxity (mm)

Loads

Specimen 1 2 3 4 5

1 297.1 297.1 297.1 297.1 297.1

2 264.4 304.6 336.4 358.2 379.3

3 188.8 188.8 188.8 188.8 188.8

4 159.3 194.7 211.4 222.4 228.1

5 228.2 282.1 282.1 334.8 334.8

6 100.3 105.0 106.3 107.7 108.7

7 116.9 140.6 182.4 209.7 215.4

Source: David W. Starch, Jerry W. Alexander, Philip C. Noble, Suraj Reddy, and David M.

Lintner, “Multistranded Hamstring Tendon Graft Fixation with a Central Four-Quadrant or a

Standard Tibial Interference Screw for Anterior Cruciate Ligament Reconstruction,” American

Journal of Sports Medicine, 31 (2003), 338–344.

8.4.3 Holben et al. (A-18) designed a study to evaluate selenium intake in young women in the years

of puberty. The researchers studied a cohort of 16 women for three consecutive summers. One of

the outcome variables was the selenium intake per day. The researchers examined dietary journals

of the subjects over the course of 2 weeks and then computed the average daily selenium intake.

The following table shows the average daily selenium intake values for the 16 women

in years 1, 2, and 3 of the study.

1in mg>d2

a = .05.

352 CHAPTER 8 ANALYSIS OF VARIANCE

8.5 THE FACTORIAL EXPERIMENT 353

Subject Year 1 Year 2 Year 3 Subject Year 1 Year 2 Year 3

1 112.51 121.28 94.99 9 95.05 93.89 73.26

2 106.20 121.14 145.69 10 112.65 100.47 145.69

3 102.00 121.14 130.37 11 103.74 121.14 123.97

4 103.74 90.21 135.91 12 103.74 121.14 135.91

5 103.17 121.14 145.69 13 112.67 104.66 136.87

6 112.65 98.11 145.69 14 106.20 121.14 126.42

7 106.20 121.14 136.43 15 103.74 121.14 136.43

8 83.57 102.87 144.35 16 106.20 100.47 135.91

Source: David H. Holben, Ph.D. and John P. Holcomb, Ph.D. Used with permission.

8.4.4 Linke et al. (A -19) studied seven male mongrel dogs. They induced diabetes by injecting the ani-

mals with alloxan monohydrate. The researchers measured the arterial glucose (mg/gl), arterial

lactate (mmol/L), arterial free fatty acid concentration, and arterial -hydroxybutyric acid concen-

tration prior to the alloxan injection, and again in weeks 1, 2, 3, and 4 post-injection. What is the

response variable(s)? Comment on carryover effect and position effect as they may or may not be

of concern in this study. Construct an ANOVA table for this study in which you identify the sources

of variability and specify the degrees of freedom for each.

8.4.5 Werther et al. (A-20) examined the vascular endothelial growth factor (VEGF) concentration in

blood from colon cancer patients. Research suggests that inhibiting VEGF may disrupt tumor

growth. The researchers measured VEGF concentration (ng/L) for 10 subjects and found an

upward trend in VEGF concentrations during the clotting time measured at baseline, and hours

1 and 2. What is the response variable? What is the treatment variable? Construct an ANOVA

table for this study in which you identify the sources of variability and specify the degrees of

freedom for each.

8.5 THE FACTORIAL EXPERIMENT

In the experimental designs that we have considered up to this point, we have been

interested in the effects of only one variable—the treatments. Frequently, however, we

may be interested in studying, simultaneously, the effects of two or more variables.

We refer to the variables in which we are interested as factors. The experiment

in which two or more factors are investigated simultaneously is called a factorial

experiment.

The different designated categories of the factors are called levels. Suppose, for

example, that we are studying the effect on reaction time of three dosages of some drug.

The drug factor, then, is said to occur at three levels. Suppose the second factor of inter-

est in the study is age, and it is thought that two age groups, under 65 years and 65 years

and older, should be included. We then have two levels of the age factor. In general, we

say that factor A occurs at a levels and factor B occurs at b levels.

In a factorial experiment we may study not only the effects of individual factors

but also, if the experiment is properly conducted, the interaction between factors. To

illustrate the concept of interaction let us consider the following example.

EXAMPLE 8.5.1

Suppose, in terms of effect on reaction time, that the true relationship between three dosage

levels of some drug and the age of human subjects taking the drug is known. Suppose fur-

ther that age occurs at two levels—“young” (under 65) and “old” (65 and older). If the

true relationship between the two factors is known, we will know, for the three dosage lev-

els, the mean effect on reaction time of subjects in the two age groups. Let us assume that

effect is measured in terms of reduction in reaction time to some stimulus. Suppose these

means are as shown in Table 8.5.1.

The following important features of the data in Table 8.5.1 should be noted.

1. For both levels of factor A the difference between the means for any two levels of

factor B is the same. That is, for both levels of factor A, the difference between means

for levels 1 and 2 is 5, for levels 2 and 3 the difference is 10, and for levels 1 and 3

the difference is 15.

2. For all levels of factor B the difference between means for the two levels of factor A

is the same. In the present case the difference is 5 at all three levels of factor B.

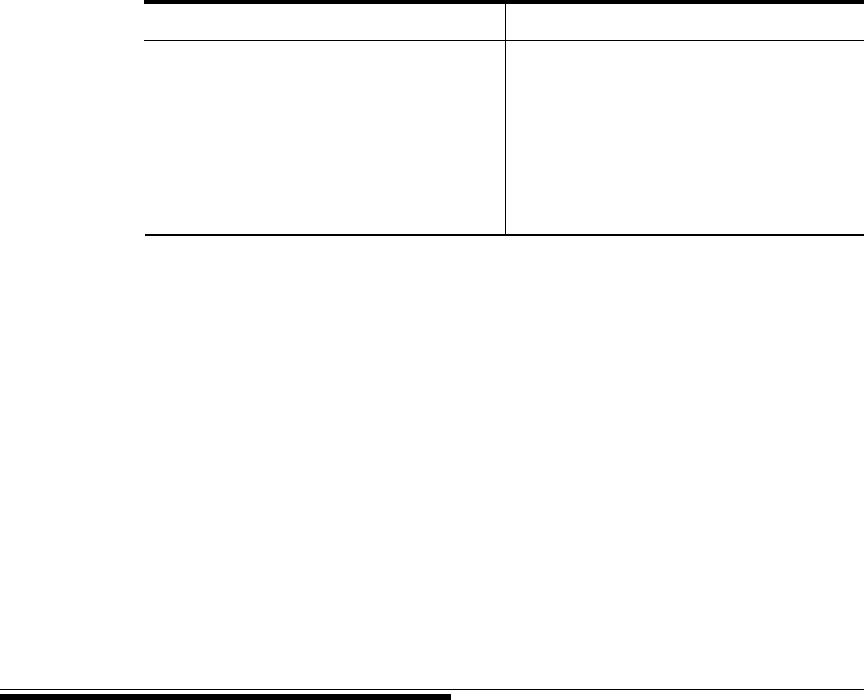
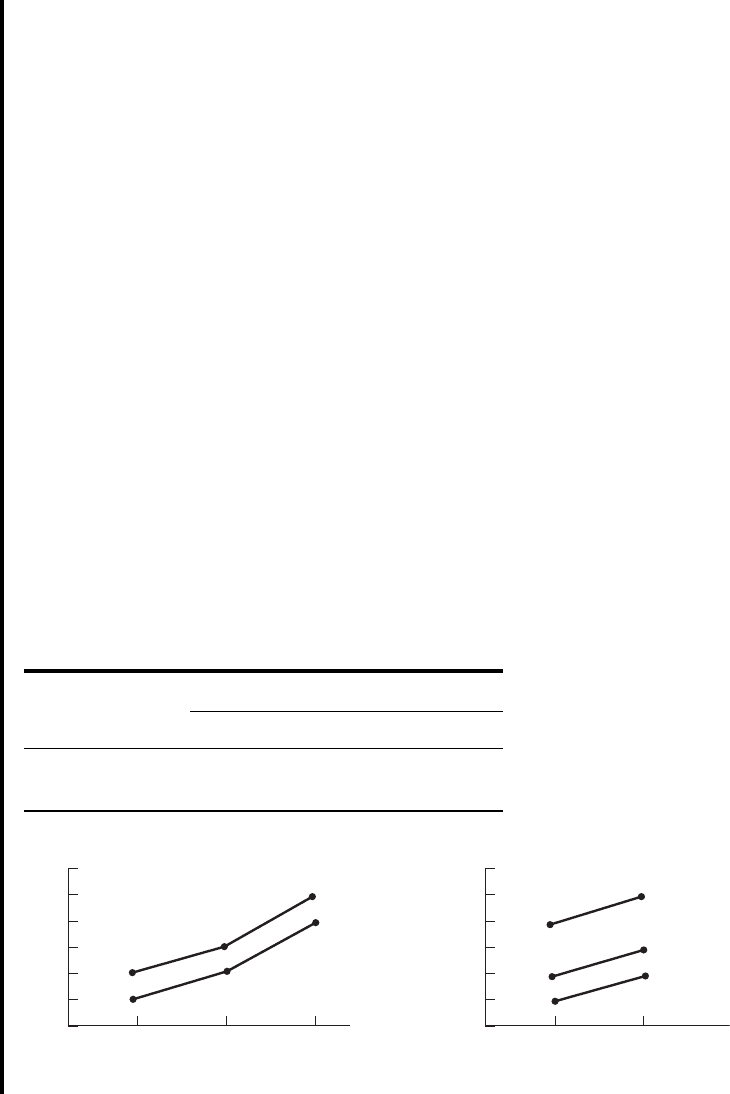
3. A third characteristic is revealed when the data are plotted as in Figure 8.5.1. We note

that the curves corresponding to the different levels of a factor are all parallel.

When population data possess the three characteristics listed above, we say that there is

no interaction present.

The presence of interaction between two factors can affect the characteristics of

the data in a variety of ways depending on the nature of the interaction. We illustrate

354 CHAPTER 8 ANALYSIS OF VARIANCE

TABLE 8.5.1 Mean Reduction in Reaction Time

(milliseconds) of Subjects in Two Age Groups at Three

Drug Dosage Levels

Factor

—Drug Dosage

Factor

—Age

Young

Old m

= 25m

= 15m

= 101i = 22

= 20m

= 10m

= 51i = 12

j  3j  2j  1

Reduction in reaction time

Drug dosage

Age

Drug dosage

FIGURE 8.5.1 Age and drug effects, no interaction present.

the effect of one type of interaction by altering the data of Table 8.5.1 as shown in

Table 8.5.2.

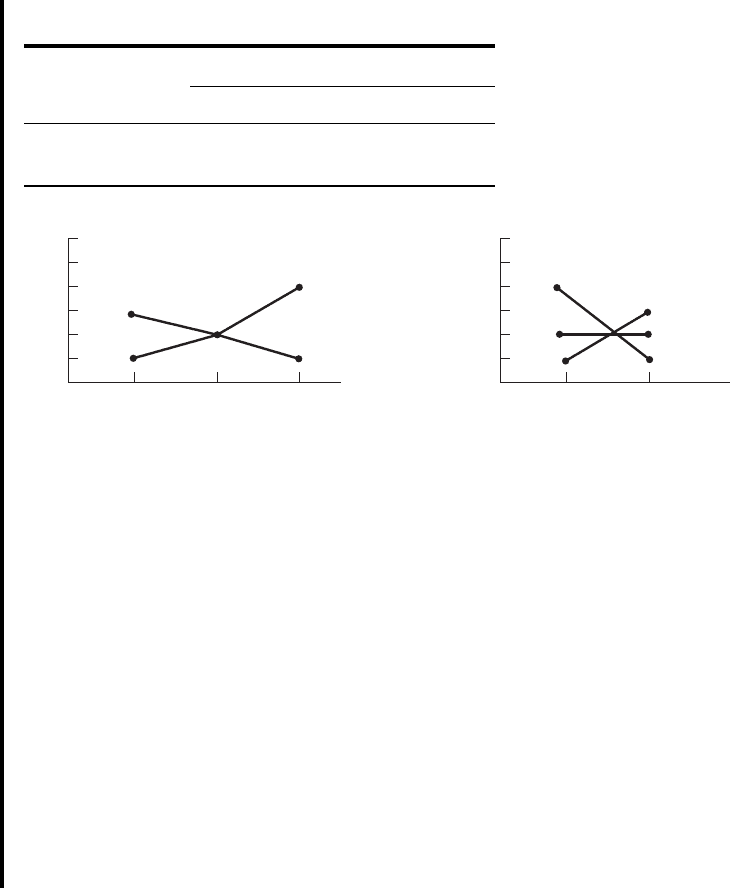
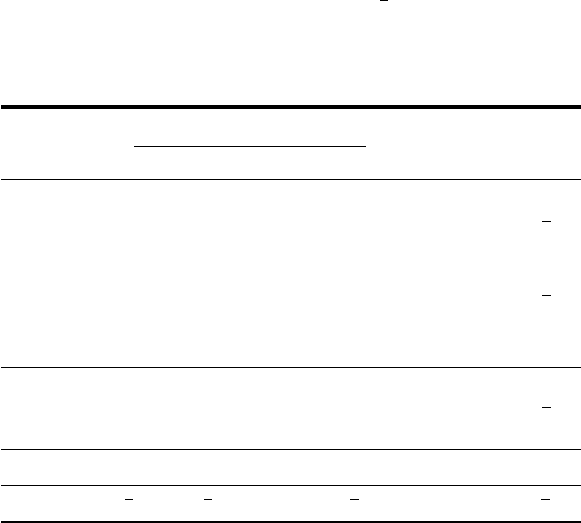
The important characteristics of the data in Table 8.5.2 are as follows.

1. The difference between means for any two levels of factor B is not the same for

both levels of factor A. We note in Table 8.5.2, for example, that the difference

between levels 1 and 2 of factor B is for the young age group and for the

old age group.

2. The difference between means for both levels of factor A is not the same at all levels

of factor B. The differences between factor A means are 0, and 15 for levels 1,

2, and 3, respectively, of factor B.

3. The factor level curves are not parallel, as shown in Figure 8.5.2.

When population data exhibit the characteristics illustrated in Table 8.5.2 and Fig-

ure 8.5.2, we say that there is interaction between the two factors. We emphasize that

the kind of interaction illustrated by the present example is only one of many types of

interaction that may occur between two factors. ■

In summary, then, we can say that there is interaction between two factors if a

change in one of the factors produces a change in response at one level of the other fac-

tor different from that produced at other levels of this factor.

Advantages The advantages of the factorial experiment include the following.

1. The interaction of the factors may be studied.

2. There is a saving of time and effort.

-10,

+5-5

8.5 THE FACTORIAL EXPERIMENT 355

TABLE 8.5.2 Data of Table 8.5.1 Altered to Show the

Effect of One Type of Interaction

Factor

—Drug Dosage

Factor

—Age

Young

Old m

= 5m

= 10m

= 151i = 22

= 20m

= 10m

= 51i = 12

j  3j  2j  1

Reduction in reaction time

Drug dosage

Age

Drug dosage

FIGURE 8.5.2 Age and drug effects, interaction present.

In the factorial experiment all the observations may be used to study the effects of each

of the factors under investigation. The alternative, when two factors are being investigated,

would be to conduct two different experiments, one to study each of the two factors. If this

were done, some of the observations would yield information only on one of the factors, and

the remainder would yield information only on the other factor. To achieve the level of accu-

racy of the factorial experiment, more experimental units would be needed if the factors were

studied through two experiments. It is seen, then, that 1 two-factor experiment is more eco-

nomical than 2 one-factor experiments.

3. Because the various factors are combined in one experiment, the results have a

wider range of application.

The Two-Factor Completely Randomized Design A factorial

arrangement may be studied with either of the designs that have been discussed. We illus-

trate the analysis of a factorial experiment by means of a two-factor completely random-

ized design.

1. Data. The results from a two-factor completely randomized design may be pre-

sented in tabular form as shown in Table 8.5.3.

Here we have a levels of factor A, b levels of factor B, and n observations for

each combination of levels. Each of the ab combinations of levels of factor A with

levels of factor B is a treatment. In addition to the totals and means shown in Table

8.5.3, we note that the total and mean of the ij th cell are

and x

ij#

= T

ij#>n

k =1

ijk

356 CHAPTER 8 ANALYSIS OF VARIANCE

TABLE 8.5.3 Table of Sample Data from a Two-Factor Completely

Randomized Experiment

Factor

Totals Means

Totals

Means x

. . .

.b.

.2.

.1.

. . .

.b.

.2.

.1.

abn

a2n

a1n

a. .

oooo

ab1

a21

a11

ooooooo

2bn

22n

21n

2. .

oooo

2b1

221

211

1bn

12n

11n

1. .

oooo

1b1

121

111