Daniel W.W. Biostatistics: A Foundation for Analysis in the Health Sciences

Подождите немного. Документ загружается.

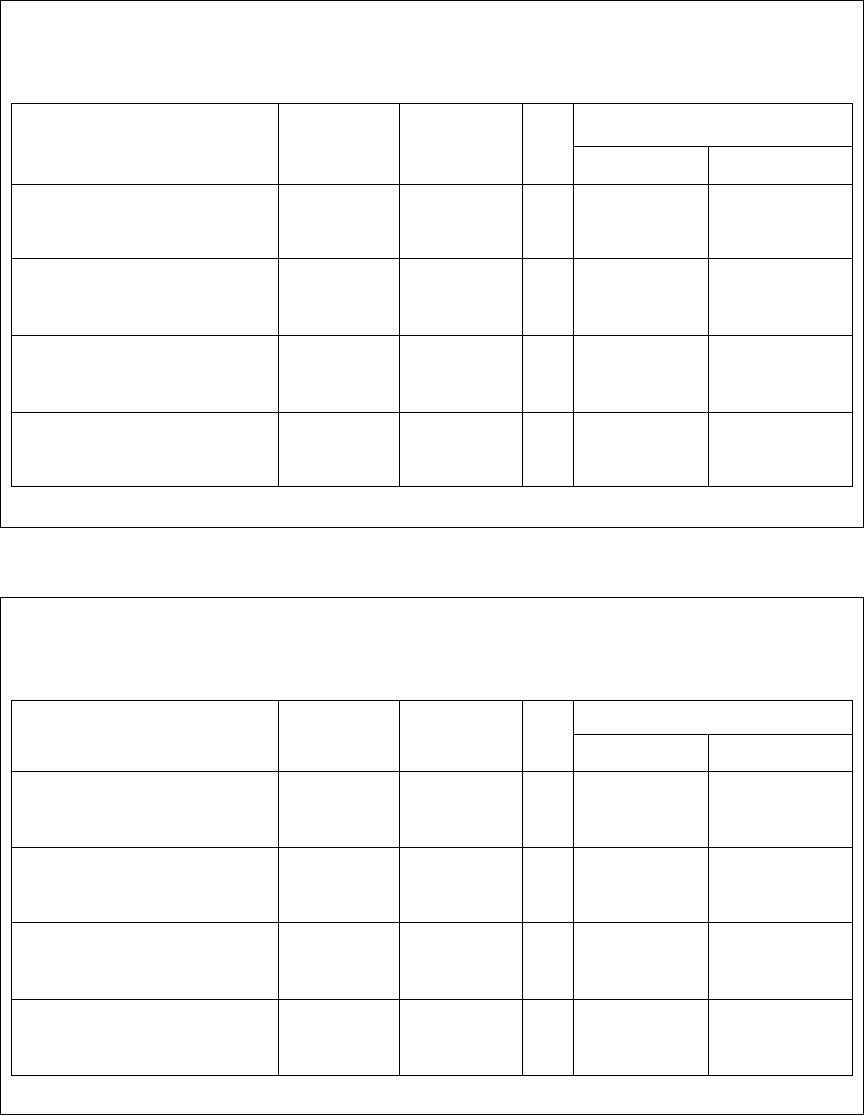
327

FIGURE 8.2.9 SPSS output for Tukey’s HSD using data from Example 8.2.1.

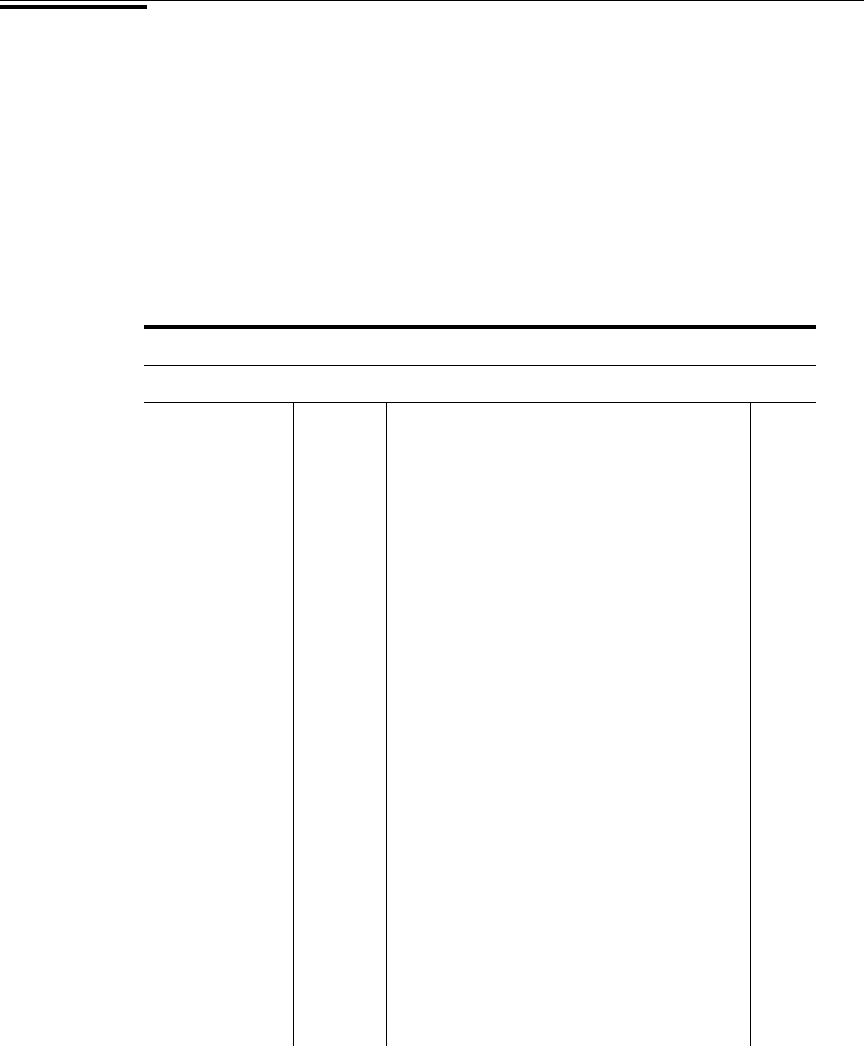
FIGURE 8.2.10 SPSS output for Bonferroni’s method using data from Example 8.2.1.

Multiple Comparisons

Dependent Variable: Selenium

Tukey HSD

Mean 95% Conﬁdence Interval

Difference

(I) Meat_type (J) Meat_type (I–J) Std. Error Sig. Lower Bound Upper Bound

VEN SQU 17.370190* 3.872837210 .000 27.44017302 7.30020793

RRB 3.2075427 3.346936628 .773 11.91010145 5.49501609

NRB 36.170840* 4.479316382 .000 47.81776286 24.52391634

SQU VEN 17.370190* 3.872837210 .000 7.30020793 27.44017302

RRB 14.162648* 3.701593729 .001 4.53792509 23.78737051

NRB 18.800649* 4.750167007 .001 31.15182638 6.44947187

RRB VEN 3.2075427 3.346936628 .773 5.49501609 11.91010145

SQU 14.162648* 3.701593729 .001 23.78737051 4.53792509

NRB 32.963297* 4.332113033 .000 44.22746845 21.69912540

NRB VEN 36.170840* 4.479316382 .000 24.52391634 47.81776286

SQU 18.800649* 4.750167007 .001 6.44947187 31.15182638

RRB 32.963297* 4.332113033 .000 21.69912540 44.22746845

* The mean difference is signiﬁcant at the .05 level.

Multiple Comparisons

Dependent Variable: Selenium

Bonferroni

Mean 95% Conﬁdence Interval

Difference

(I) Meat_type (J) Meat_type (I–J) Std. Error Sig. Lower Bound Upper Bound

VEN RRB 3.20754 3.34694 1.000 12.1648 5.7497

SQU 17.37019* 3.87284 .000 27.7349 7.0055

NRB 36.17084* 4.47932 .000 48.1587 24.1830

RRB VEN 3.20754 3.34694 1.000 5.7497 12.1648

SQU 14.16265* 3.70159 .001 24.0691 4.2562

NRB 32.96330* 4.33211 .000 44.5572 21.3694

SQU VEN 17.37019* 3.87284 .000 7.0055 27.7349

RRB 14.16265* 3.70159 .001 4.2562 24.0691

NRB 18.80065* 4.75017 .001 31.5134 6.0879

NRB VEN 36.17084* 4.47932 .000 24.1830 48.1587

RRB 32.96330* 4.33211 .000 21.3694 44.5572

SQU 18.80065* 4.75017 .001 6.0879 31.5134

* The mean difference is signiﬁcant at the .05 level.

EXERCISES

In Exercises 8.2.1 to 8.2.7, go through the ten steps of analysis of variance hypothesis testing to

see if you can conclude that there is a difference among population means. Let for each

test. Use Tukey’s HSD procedure to test for signiﬁcant differences among individual pairs of means

(if appropriate). Use the same value for the F test. Construct a dot plot and side-by-side box-

plots of the data.

8.2.1 Researchers at Case Western Reserve University (A-2) wanted to develop and implement a trans-

ducer, manageable in a clinical setting, for quantifying isometric moments produced at the elbow

joint by individuals with tetraplegia (paralysis or paresis of all four limbs). The apparatus, called

an elbow moment transducer (EMT), measures the force the elbow can exert when ﬂexing. The

output variable is voltage. The machine was tested at four different elbow extension angles, 30,

60, 90, and 120 degrees, on a mock elbow consisting of two hinged aluminum beams. The data

are shown in the following table.

Elbow Angle (Degrees)

30 60 90 120

1.094 0.000 0.000 0.558 0.003

0.050 1.061 0.053 0.010 0.006 0.012 0.529 0.062

0.272 1.040 0.269 0.028 0.026 0.524 0.287

0.552 1.097 0.555 0.055 0.053 0.555 0.555

1.116 1.080 1.103 0.105 0.108 0.539 1.118

2.733 1.051 2.727 0.272 0.278 0.536 2.763

0.000 1.094 0.553 0.555 0.557 0.006

0.056 1.075 0.052 0.840 0.834 0.544 0.050

0.275 1.035 0.271 1.100 1.106 0.539 0.277

0.556 1.096 0.550 1.647 1.650 1.109 0.557

1.100 1.100 1.097 2.728 2.729 0.003 1.085 1.113

2.723 1.096 2.725 0.005 0.003 1.070 2.759

1.108 0.003 0.014 1.110 0.010

0.055 1.099 0.052 0.027 1.069 0.060

0.273 1.089 0.270 0.057 1.045 0.286

0.553 1.107 0.553 0.111 1.110 0.564

1.100 1.094 1.100 0.276 1.066 1.104

2.713 1.092 2.727 0.555 1.037 2.760

0.007 1.092 0.022 0.832 2.728

1.104 1.099 2.694

1.121 1.651 0.004 2.663

1.106 2.736 0.566 2.724

1.135 0.564 0.000 1.116 2.693

0.008 1.143 0.017 0.556 0.245 2.762 2.670 0.000

1.106 0.555 0.497 0.563 2.720

1.135 0.567 0.001 0.551 2.688

1.156 0.559 0.248 0.551 2.660

1.112 0.551 0.498 0.561 0.556

1.104 0.019 1.107 0.001 0.555 0.560 -0.019-0.004

-1.165-1.187-1.143

-0.579-0.548-0.604

-0.295-0.258-0.274

-0.034-0.052-0.045

-1.180-1.168-1.162

-0.585-2.862-0.585-0.581

-0.289-1.755-0.298-0.258

-0.060-1.760-1.157-0.075-0.066

-0.003-1.168-0.876

-0.840-0.589

-0.593-0.297

-0.320-0.134

-0.097-0.046

-0.060-0.037

-0.011-0.023-0.003

0.001

-1.725

-1.176

-0.884

-0.602-0.002

-0.291

-0.118

-0.080

-0.039

-0.007-0.001-0.003

a = .05

328 CHAPTER 8 ANALYSIS OF VARIANCE

(Continued)

Elbow Angle (Degrees)

30 60 90 120

1.107 1.104 0.246 0.558 0.557

1.107 1.102 0.491 0.551 0.551

1.104 1.112 0.001 0.566 0.564

1.117 1.103 0.262 0.560 0.555

1.105 1.101 1.104 0.527 1.107 0.551

1.103 1.114 0.001 1.104 0.563

1.095 0.260 1.109 0.559

1.100 0.523 1.108 1.113

2.739 1.106 1.114

2.721 0.261 1.102 1.101

2.687 0.523 1.111 1.113

2.732 2.696 1.102 1.113

2.702 2.664 1.107 1.097

2.660 2.722 2.735 1.116

2.743 2.686 2.733 1.112

2.687 2.661 2.659 1.098

2.656 0.548 2.727 2.732

2.733 2.739 0.542 2.722

2.731 2.742 0.556 2.734

2.728 2.747

Source: S. A. Snyder, M.S. Used with permission.

8.2.2 Patients suffering from rheumatic diseases or osteoporosis often suffer critical losses in bone min-

eral density (BMD). Alendronate is one medication prescribed to build or prevent further loss of

BMD. Holcomb and Rothenberg (A-3) looked at 96 women taking alendronate to determine if a

difference existed in the mean percent change in BMD among ﬁve different primary diagnosis

classiﬁcations. Group 1 patients were diagnosed with rheumatoid arthritis (RA). Group 2 patients

were a mixed collection of patients with diseases including lupus, Wegener’s granulomatosis and

polyarteritis, and other vasculitic diseases (LUPUS). Group 3 patients had polymyalgia rheumat-

ica or temporal arthritis (PMRTA). Group 4 patients had osteoarthritis (OA) and group 5 patients

had osteoporosis (O) with no other rheumatic diseases identiﬁed in the medical record. Changes

in BMD are shown in the following table.

Diagnosis

RA LUPUS PMRTA OA O

11.091 7.412 2.961 11.146 2.937

24.414 5.559 0.293 15.968

10.025 4.761 8.394 4.563 4.082 5.349

2.832 6.645 1.719

6.835 4.839 4.329 6.445

3.321 1.850 11.288 1.302 1.234 20.243

1.493 3.997 5.299 3.290-2.817-3.933

-0.185-1.369

-0.093-3.527-3.156

-0.838-7.816

-3.669

-0.005

-1.162-1.189-1.164

-0.579-0.542-0.607

-0.270-0.292-0.290

-0.056-0.044-0.050

EXERCISES 329

(Continued)

Age Groups (Years) Age Groups (Years)

ABCD ABC D

1820 191 724 1652 1020 775

2588 1098 613 1309 805 1393

2670 644 918 1002 631 533

1022 136 949 966 641 734

1555 1605 877 788 760 485

222 1247 1368 472 449

1197 1529 1692 471 236

1249 1422 697 771 831

1520 445 849 869 698

489 990 1199 513 167

2575 489 429 731 824

1426 2408 798 1130 448

1846 1064 631 1034 991

1088 629 1016 1261 590

912 1025 42 994

1383 948 767 1781

Diagnosis

RA LUPUS PMRTA OA O

9.669 7.260 10.734 3.544 8.992

5.386 4.659 5.546 1.399 4.160 6.120

3.868 1.137 0.497 1.160 25.655

6.209 7.521 0.592

0.073 3.950 5.372

3.514 0.674 6.721

9.354 9.950

15.981 21.311 2.610 10.820

10.831 5.682 7.280

5.188 3.351 6.605

9.557 7.507

16.553 5.075

0.163

12.767

3.481

0.917

15.853

Source: John P. Holcomb, Ph.D. and Ralph J. Rothenberg, M.D. Used with permission.

8.2.3 Ilich-Ernst et al. (A-4) investigated dietary intake of calcium among a cross section of 113 healthy

women ages 20–88. The researchers formed four age groupings as follows: Group A, 20.0–45.9

years; group B, 46.0–55.9 years; group C, 56.0–65.9 years; and group D, over 66 years. Calcium

from food intake was measured in mg/day. The data below are consistent with summary statistics

given in the paper.

-1.892

-9.646

-0.372-2.308

-8.684

-5.640

-0.247

-1.864

330 CHAPTER 8 ANALYSIS OF VARIANCE

(Continued)

EXERCISES 331

Age Groups (Years) Age Groups (Years)

ABCD ABC D

1483 1085 752 937

1723 775 804 1022

727 1307 1182 1073

1463 344 1243 948

1777 961 985 222

1129 239 1295 721

944 1676 375

1096 754 1187

8.2.4 Gold et al. (A-5) investigated the effectiveness on smoking cessation of a nicotine patch, bupro-

pion SR, or both, when co-administered with cognitive-behavioral therapy. Consecutive consent-

ing patients assigned themselves to one of three treatments according to personal

preference: nicotine patch bupropion SR and bupropion SR plus

nicotine patch At their ﬁrst smoking cessation class, patients estimated the num-

ber of packs of cigarettes they currently smoked per day and the numbers of years they smoked.

The “pack years” is the average number of packs the subject smoked per day multiplied by the

number of years the subject had smoked. The results are shown in the following table.

1BNTP, n = 592.

1B;

n = 922,1NTP, n = 132,

1n = 1642

Pack Years

NTP B BNTP

1586090880

17 10 60 90 15 80

18 15 60 90 25 82

20 20 60 95 25 86

20 22 60 96 25 87

20 24 60 98 26 90

30 25 60 98 30 90

37 26 66 99 34 90

43 27 66 100 35 90

48 29 67 100 36 90

60 30 68 100 40 95

100 30 68 100 45 99

100 35 70 100 45 100

35 70 100 45 102

39 70 105 45 105

40 75 110 48 105

40 75 120 49 111

40 75 120 52 113

40 76 123 60 120

40 80 125 60 120

45 80 125 60 125

(Continued)

Pack Years

NTP B BNTP

45 80 126 64 125

45 80 130 64 129

50 80 130 70 130

51 80 132 70 133

52 80 132 70 135

55 84 142 75 140

58 84 157 75 154

60 84 180 76

60 90

Source: Paul B. Gold, Ph.D. Used with Permission.

332 CHAPTER 8 ANALYSIS OF VARIANCE

8.2.5 In a study by Wang et al. (A-6), researchers examined bone strength. They collected 10 cadaveric

femurs from subjects in three age groups: young (19–49 years), middle-aged (50–69 years), and

elderly (70 years or older) [Note: one value was missing in the middle-aged group]. One of the

outcome measures (W ) was the force in newtons required to fracture the bone. The following table

shows the data for the three age groups.

Young (Y) Middle-aged (MA) Elderly (E)

193.6 125.4 59.0

137.5 126.5 87.2

122.0 115.9 84.4

145.4 98.8 78.1

117.0 94.3 51.9

105.4 99.9 57.1

99.9 83.3 54.7

74.0 72.8 78.6

74.4 83.5 53.7

112.8 96.0

Source: Xiaodu Wang, Ph.D. Used with permission.

8.2.6 In a study of 90 patients on renal dialysis, Farhad Atassi (A-7) assessed oral home care practices.

He collected data from 30 subjects who were in (1) dialysis for less than 1 year, (2) dialysis for

1 to 3 years, and (3) dialysis for more than 3 years. The following table shows plaque index scores

for these subjects. A higher score indicates a greater amount of plaque.

Group 1 Group 2 Group 3

2.00 2.67 2.83 2.83 1.83 1.83

1.00 2.17 2.00 1.83 2.00 2.67

2.00 1.00 2.67 2.00 1.83 1.33

1.50 2.00 2.00 1.83 1.83 2.17

2.00 2.00 2.83 2.00 2.83 3.00

(Continued)

Group 1 Group 2 Group 3

1.00 2.00 2.17 2.17 2.17 2.33

1.00 2.33 2.17 1.67 2.83 2.50

1.00 1.50 2.00 2.33 2.50 2.83

1.00 1.00 2.00 2.00 2.17 2.83

1.67 2.00 1.67 2.00 1.67 2.33

1.83 .83 2.33 2.17 2.17 2.33

2.17 .50 2.00 3.00 1.83 2.67

1.00 2.17 1.83 2.50 2.83 2.00

2.17 2.33 1.67 2.17 2.33 2.00

2.83 2.83 2.17 2.00 2.00 2.00

Source: Farhad Atassi, DDS, MSC, FICOI. Used with permission.

EXERCISES 333

8.2.7 Thrombocytopaenia is a condition of abnormally low platelets that often occurs during necrotizing

enterocolitis (NEC)—a serious illness in infants that can cause tissue damage to the intestines.

Ragazzi et al. (A-8) investigated differences in the of platelet counts in 178 infants with NEC.

Patients were grouped into four categories of NEC status. Group 0 referred to infants with no gan-

grene, group 1 referred to subjects in whom gangrene was limited to a single intestinal segment,

group 2 referred to patients with two or more intestinal segments of gangrene, and group 3 referred

to patients with the majority of small and large bowel involved. The following table gives the

platelet counts for these subjects.

log

Gangrene Grouping

0123

1.97 2.33 2.48 1.38 2.45 1.87 2.37 1.77

0.85 2.60 2.23 1.86 2.60 1.90 1.75 1.68

1.79 1.88 2.51 2.26 1.83 2.43 2.57 1.46

2.30 2.33 2.38 1.99 2.47 1.32 1.51 1.53

1.71 2.48 2.31 1.32 1.92 2.06 1.08 1.36

2.66 2.15 2.08 2.11 2.51 1.04 2.36 1.65

2.49 1.41 2.49 2.54 1.79 1.99 1.58 2.12

2.37 2.03 2.21 2.06 2.17 1.52 1.83 1.73

1.81 2.59 2.45 2.41 2.18 1.99 2.55 1.91

2.51 2.23 1.96 2.23 2.53 2.52 1.80 1.57

2.38 1.61 2.29 2.00 1.98 1.93 2.44 2.27

2.58 1.86 2.54 2.74 1.93 2.29 2.81 1.00

2.58 2.33 2.23 2.00 2.42 1.75 2.17 1.81

2.84 2.34 2.78 2.51 0.79 2.16 2.72 2.27

2.55 1.38 2.36 2.08 1.38 1.81 2.44 2.43

1.90 2.52 1.89 2.46 1.98 1.74

2.28 2.35 2.26 1.66 1.57 1.60

2.33 2.63 1.79 2.51 2.05 2.08

1.77 2.03 1.87 1.76 2.30 2.34

1.83 1.08 2.51 1.72 1.36 1.89

1.67 2.40 2.29 2.57 2.48 1.75

(Continued)

334 CHAPTER 8 ANALYSIS OF VARIANCE

Gangrene Grouping

0123

2.67 1.77 2.38 2.30 1.40 1.69

1.80 0.70 1.75 2.49

2.16 2.67 1.75

2.17 2.37 1.86

2.12 1.46 1.26

2.27 1.91 2.36

Source: Simon Eaton, M.D. Used with permission.

8.2.8 The objective of a study by Romito et al. (A-9) was to determine whether there is a different

response to different calcium channel blockers. Two hundred and fifty patients with mild-to-

moderate hypertension were randomly assigned to 4 weeks of treatment with once-daily doses of

(1) lercanidipine, (2) felodipine, or (3) nifedipine. Prior to treatment and at the end of 4 weeks,

each of the subjects had his or her systolic blood pressure measured. Researchers then calculated

the change in systolic blood pressure. What is the treatment variable in this study? The response

variable? What extraneous variables can you think of whose effects would be included in the error

term? What are the “values” of the treatment variable? Construct an analysis of variance table in

which you specify for this study the sources of variation and the degrees of freedom.

8.2.9 Kosmiski et al. (A-10) conducted a study to examine body fat distributions of men infected and not

infected with HIV, taking and not taking protease inhibitors (PI), and having been diagnosed and not

diagnosed with lipodystrophy. Lipodystrophy is a syndrome associated with HIV/PI treatment that

remains controversial. Generally, it refers to fat accumulation in the abdomen or viscera accompanied

by insulin resistance, glucose intolerance, and dyslipidemia. In the study, 14 subjects were taking pro-

tease inhibitors and were diagnosed with lipodystrophy, 12 were taking protease inhibitors, but were

not diagnosed with lipodystrophy, ﬁve were HIV positive, not taking protease inhibitors, nor had

diagnosed lypodystrophy, and 43 subjects were HIV negative and not diagnosed with lipodystrophy.

Each of the subjects underwent body composition and fat distribution analyses by dual-energy X-ray

absorptiometry and computed tomography. Researchers were able to then examine the percent of body

fat in the trunk. What is the treatment variable? The response variable? What are the “values” of the

treatment variable? Who are the subjects? What extraneous variables can you think of whose effects

would be included in the error term? What was the purpose of including HIV-negative men in the

study? Construct an ANOVA table in which you specify the sources of variation and the degrees of

freedom for each. The authors reported a computed V.R. of 11.79. What is the p value for the test?

8.3 THE RANDOMIZED COMPLETE

BLOCK DESIGN

The randomized complete block design was developed about 1925 by R. A. Fisher, who

was seeking methods of improving agricultural ﬁeld experiments. The randomized com-

plete block design is a design in which the units (called experimental units) to which

the treatments are applied are subdivided into homogeneous groups called blocks, so

that the number of experimental units in a block is equal to the number (or some mul-

tiple of the number) of treatments being studied. The treatments are then assigned at

random to the experimental units within each block. It should be emphasized that each

treatment appears in every block, and each block receives every treatment.

8.3 THE RANDOMIZED COMPLETE BLOCK DESIGN 335

Objective The objective in using the randomized complete block design is to iso-

late and remove from the error term the variation attributable to the blocks, while assur-

ing that treatment means will be free of block effects. The effectiveness of the design

depends on the ability to achieve homogeneous blocks of experimental units. The abil-

ity to form homogeneous blocks depends on the researcher’s knowledge of the experi-

mental material. When blocking is used effectively, the error mean square in the ANOVA

table will be reduced, the V.R. will be increased, and the chance of rejecting the null

hypothesis will be improved.

In animal experiments, the breed of animal may be used as a blocking factor. Lit-

ters may also be used as blocks, in which case an animal from each litter receives a

treatment. In experiments involving human beings, if it is desired that differences result-

ing from age be eliminated, then subjects may be grouped according to age so that one

person of each age receives each treatment. The randomized complete block design also

may be employed effectively when an experiment must be carried out in more than one

laboratory (block) or when several days (blocks) are required for completion.

The random allocation of treatments to subjects is restricted in the randomized

complete block design. That is, each treatment must be represented an equal number of

times (one or more times) within each blocking unit. In practice this is generally accom-

plished by assigning a random permutation of the order of treatments to subjects within

each block. For example, if there are four treatments representing three drugs and a

placebo (drug A, drug B, drug C, and placebo [p]), then there are 4!  24 possible per-

mutations of the four treatments: (A, B, C, P) or (A, C, B, P) or (C, A, P, B), and so

on. One permutation is then randomly assigned to each block.

Advantages One of the advantages of the randomized complete block design is

that it is easily understood. Furthermore, certain complications that may arise in the

course of an experiment are easily handled when this design is employed.

It is instructive here to point out that the paired comparisons analysis presented in

Chapter 7 is a special case of the randomized complete block design. Example 7.4.1, for

example, may be treated as a randomized complete block design in which the two points

in time (Pre-op and Post-op) are the treatments and the individuals on whom the meas-

urements were taken are the blocks.

Data Display In general, the data from an experiment utilizing the randomized

complete block design may be displayed in a table such as Table 8.3.1. The following

new notation in this table should be observed:

grand total = T

j =1

i =1

mean of the ith block = x

j =1

total of the ith block = T

j =1

indicating that the grand total may be obtained either by adding row totals or by adding

column totals.

Two-Way ANOVA The technique for analyzing the data from a randomized com-

plete block design is called two-way analysis of variance since an observation is

categorized on the basis of two criteria—the block to which it belongs as well as the treat-

ment group to which it belongs.

The steps for hypothesis testing when the randomized complete block design is

used are as follows:

1. Data. After identifying the treatments, the blocks, and the experimental units, the

data, for convenience, may be displayed as in Table 8.3.1.

2. Assumptions. The model for the randomized complete block design and its under-

lying assumptions are as follows:

The Model

(8.3.1)

In this model

is a typical value from the overall population.

is an unknown constant.

represents a block effect reﬂecting the fact that the experimental unit fell

in the ith block.

represents a treatment effect, reﬂecting the fact that the experimental unit

received the jth treatment.

is a residual component representing all sources of variation other than

treatments and blocks.

j = 1, 2, Á , ki = 1, 2, Á , n;

= m + b

+ t

336 CHAPTER 8 ANALYSIS OF VARIANCE

TABLE 8.3.1 Table of Sample Values for the Randomized

Complete Block Design

Treatments

Blocks 1 2 3

...

Total Mean

Total

Mean x

...

oooooooo

...