Coburn J.W., Herdlick J.D. College Algebra: Graphs and Models
Подождите немного. Документ загружается.

140 CHAPTER 1 Relations, Functions, and Graphs 1–56
College Algebra G&M—
EXAMPLE 10
䊳
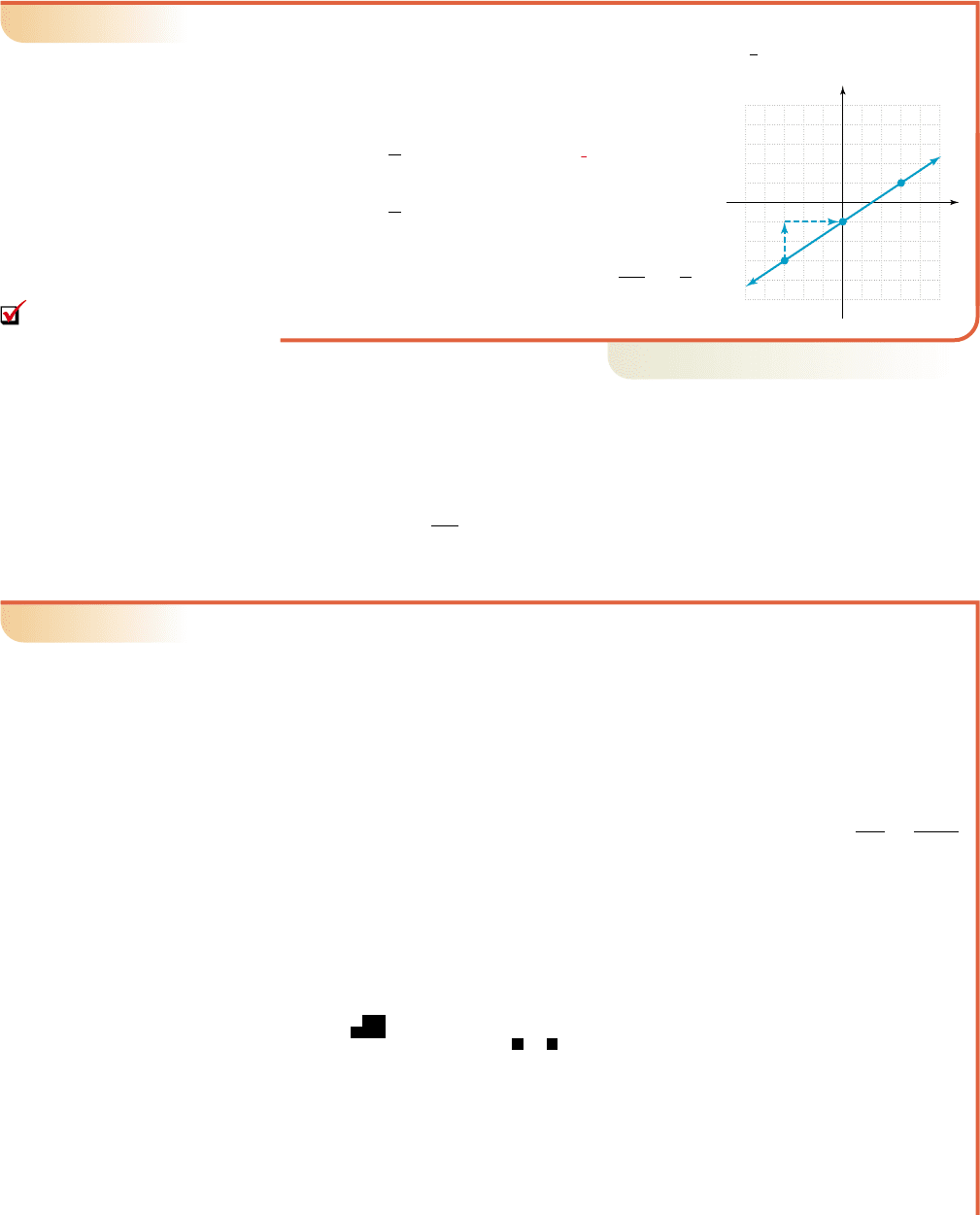
Using y y
1
m(x x
1
) as a Formula
Find the equation of the line in point-slope form, if and ( ) is on the
line. Then graph the line.
Solution
䊳
point-slope form
substitute for
m
; ( )
for (
x
1
,
y
1
)
simplify, point-slope form
To graph the line, plot ( ) and use
to find additional points on the line.
Now try Exercises 83 through 94
䊳
D. Applications of Linear Equations
As a mathematical tool, linear equations rank among the most common, powerful, and
versatile. In all cases, it’s important to remember that slope represents a rate of change.
The notation literally means the quantity measured along the y-axis, is chang-
ing with respect to changes in the quantity measured along the x-axis.
EXAMPLE 11
䊳
Relating Temperature to Altitude
In meteorological studies, atmospheric temperature depends on the altitude
according to the formula where T(h) represents the
approximate Fahrenheit temperature at height h (in thousands of feet, ).
a. Interpret the meaning of the slope in this context.
b. Determine the temperature at an altitude of 12,000 ft.
c. If the temperature is what is the approximate altitude?
Algebraic Solution
䊳
a. Notice that h is the input variable and T is the output. This shows
meaning the temperature drops for every 1000-ft increase in altitude.
b. Since height is in thousands, use .
original formula
substitute 12 for
h
result
Technology Solution
䊳
At a height of 12,000 ft, the temperature is about .16.5°
16.5
T11223.51122 58.5
T1h23.5h 58.5
h 12
3.5°F
¢T
¢h
3.5
1
,
8°F
0 h 36
T1h23.5h 58.5,
m
¢y
¢x
¢y
¢x
2
3
3, 3
y 3
2
3
1x 32
3, 3
2
3
y 132
2
3
3x 1324
y y
1
m1x x
1
2
3, 3m
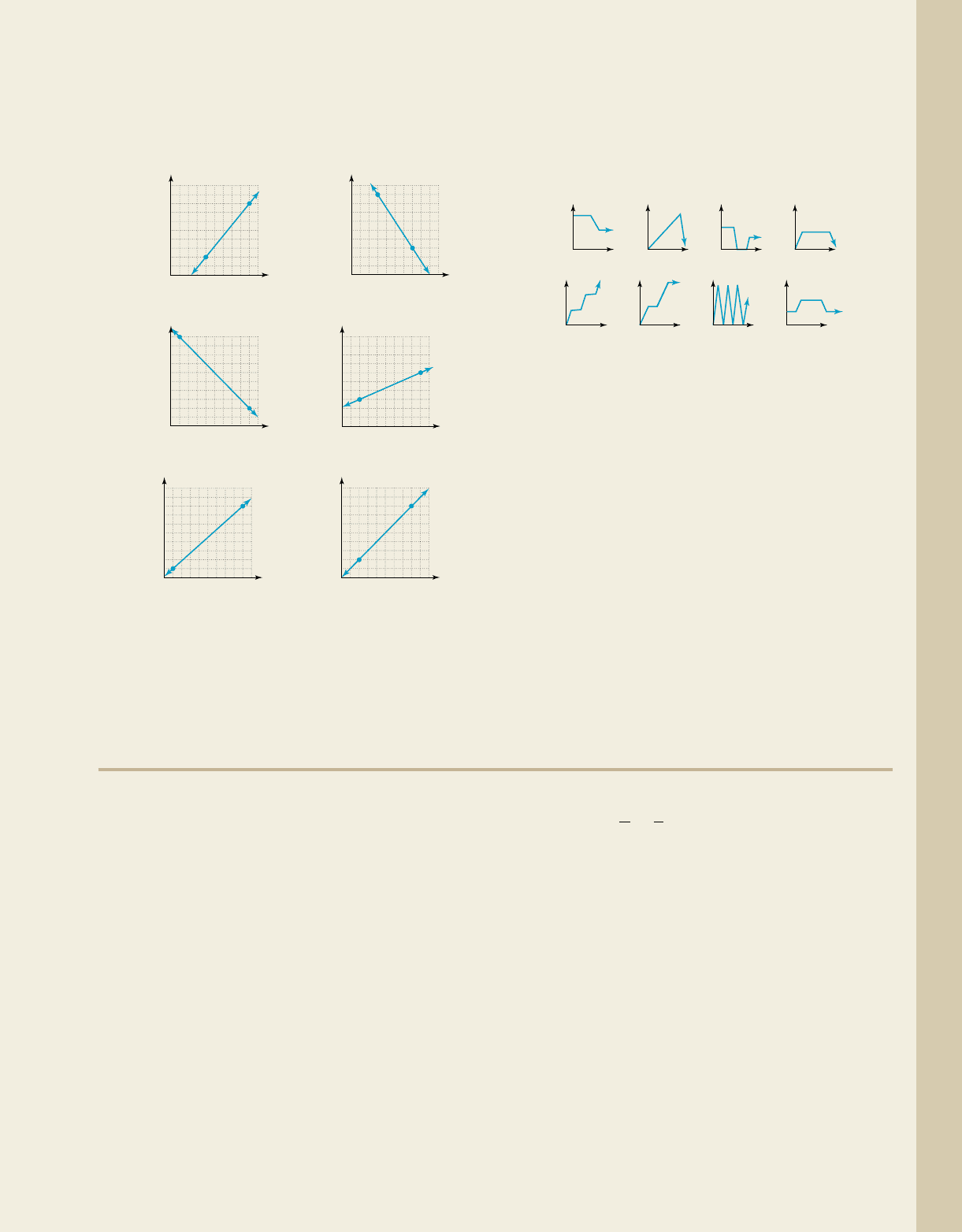
2
3
y2
x3
(3, 3)
y 3 s (x 3)
y
5
5
x
55
C. You’ve just seen how
we can write a linear equation
in point-slope form
cob19545_ch01_134-147.qxd 11/22/10 1:53 PM Page 140

c. Replacing T(h) with 8 and solving gives
Algebraic Solution
䊳
original formula
substitute 8 for
T
(
h
)
subtract 58.5
divide by
The temperature is about at a height of .
Graphical Solution
䊳
Since we’re given , we can set and . At
ground level , the formula gives a temperature of , while at ,
we have . This shows appropriate settings for the range would be
and (see figure). After setting ,
we press and move the cursor until we find an output value near , which
occurs when X is near 19. To check, we input 19 for x and the calculator displays
an output of , which corresponds with the algebraic result (at 19,000 ft, the
temperature is ).8°F
8
8
TRACE
Y
1
3.5X 58.5Ymax 50Ymin 50
T136267.5
h 3658.5°1x 02
Xmax 40Xmin 00 h 36
19 1000 19,000 ft8°F
3.5 19 h
66.5 3.5h
8 3.5h 58.5
T1h23.5h 58.5
1–57 Section 1.4 Linear Functions, Special Forms, and More on Rates of Change 141
College Algebra G&M—
40
50
50
0
Now try Exercises 105 and 106
䊳
In many applications, outputs that are integer or rational values are rare, making it
difficult to use the feature alone to find an exact solution. In the Section 1.5, we’ll
develop additional ways that graphs and technology can be used to solve equations.
In some applications, the relationship is known to be linear but only a few points on
the line are given. In this case, we can use two of the known data points to calculate the
slope, then the point-slope form to find an equation model. One such application is lin-
ear depreciation, as when a government allows businesses to depreciate vehicles and
equipment over time (the less a piece of equipment is worth, the less you pay in taxes).
EXAMPLE 12A
䊳
Using Point-Slope Form to Find a Function Model
Five years after purchase, the auditor of a newspaper company estimates the value
of their printing press is $60,000. Eight years after its purchase, the value of the
press had depreciated to $42,000. Find a linear equation that models this
depreciation and discuss the slope and y-intercept in context.
Solution
䊳
Since the value of the press depends on time, the ordered pairs have the form (time,
value) or (t, v) where time is the input, and value is the output. This means the
ordered pairs are (5, 60,000) and (8, 42,000).
slope formula
simplify and reduce
18,000
3
6000
1
1t
2
, v
2
2 18, 42,00021t
1
, v
1
2 15, 60,0002;
42,000 60,000
8 5
m
v
2
v
1
t
2
t
1
TRACE
cob19545_ch01_134-147.qxd 11/1/10 7:20 AM Page 141

142 CHAPTER 1 Relations, Functions, and Graphs 1–58
WORTHY OF NOTE
Actually, it doesn’t matter which of
the two points are used in Example
12A. Once the point (5, 60,000) is
plotted, a constant slope of
will “drive” the line
through (8, 42,000). If we first graph
(8, 42,000), the same slope would
“drive” the line through (5, 60,000).
Convince yourself by reworking the
problem using the other point.
m 6000
The slope of the line is , indicating the printing press loses
$6000 in value with each passing year.
point-slope form
substitute for
m
; (5, 60,000) for (
t
1
,
v
1
)
simplify
solve for
v
The depreciation equation is . The v-intercept (0, 90,000)
indicates the original value (cost) of the equipment was $90,000.
Once the depreciation equation is found, it represents the (time, value) relationship
for all future (and intermediate) ages of the press. In other words, we can now predict
the value of the press for any given year. However, note that some equation models are
valid for only a set period of time, and each model should be used with care.
EXAMPLE 12B
䊳
Using a Function Model to Gather Information
From Example 12A,
a. How much will the press be worth after 11 yr?
b. How many years until the value of the equipment is $9000?
c. Is this function model valid for (why or why not)?
Solution
䊳
a. Find the value v when :
equation model
substitute 11 for
t
result (11, 24,000)
After 11 yr, the printing press will only be worth $24,000.
b. “...value is $9000” means :
value at time
t
substitute for
v
(
t
)
subtract 90,000
divide by
After 13.5 yr, the printing press will be worth $9000.
c. Since substituting 18 for t gives a negative quantity, the function model is not
valid for . In the current context, the model is only valid while
and solving shows the domain of the function in this
context is .
Now try Exercises 107 through 112
䊳
t 30, 154
6000t 90,000 0
v 0t 18
6000 t 13.5
6000t 81,000
6000t 90,000 6000t 90,000 9000
v1t2 9000
v1t2 9000
24,000
v111260001112 90,000
v1t26000t 90,000
t 11
t 18 yr
v1t26000t 90,000
v 6000t 90,000
v 60,000 6000t 30,000
6000 v 60,000 60001t 52
v v
1
m1t t
1
2
¢value
¢time
6000
1
College Algebra G&M—
D. You’ve just seen how
we can apply the slope-
intercept form and point-slope
form in context
cob19545_ch01_134-147.qxd 11/22/10 1:54 PM Page 142
1–59 Section 1.4 Linear Functions, Special Forms, and More on Rates of Change 143
College Algebra G&M—
2. The notation indicates the is
changing in response to changes in .
¢cost
¢time
䊳
CONCEPTS AND VOCABULARY
Fill in each blank with the appropriate word or phrase. Carefully reread the section if needed.
1. For the equation the slope is
and the y-intercept is .
y
7
4
x 3,
3. Line 1 has a slope of The slope of any line
perpendicular to line 1 is .
0.4.
4. The equation is called the
form of a line.
y y
1
m1x x
1
2
5. Discuss/Explain how to graph a line using only the
slope and a point on the line (no equations).
6. Given and is on the line. Compare
and contrast finding the equation of the line using
versus y y
1
m1x x
1
2.y mx b
15, 62m
3
5
䊳
DEVELOPING YOUR SKILLS
Solve each equation for y and evaluate the result using
7. 8.
9. 10.
11. 12.
For each equation, solve for y and identify the new
coefficient of x and new constant term.
13. 14.
15. 16.
17. 18.
Evaluate each equation by selecting three inputs that
will result in integer values. Then graph each line.
19. 20.
21. 22.
23. 24.
Find the x- and y-intercepts for each line, then
(a) use these two points to calculate the slope of the
line, (b) write the equation with y in terms of x (solve
for y) and (c) compare the calculated slope and
y-intercept to the equation from part (b). Comment
on what you notice.
25. 26.
27. 28.
29. 30. 5y 6x 254x 5y 15
2x 3y 92x 5y 10
3y 2x 63x 4y 12
y
1
3
x 3y
1
6
x 4
y
2
5
x 3y
3
2
x 2
y
5
4
x 1y
4
3
x 5
7
12
y
4
15
x
7
6
5
6
x
1
7
y
4
7
0.7x 0.6y 2.40.5x 0.3y 2.1
9y 4x 186x 3y 9
1
7
y
1
3
x 2
1
3
x
1
5
y 1
0.2x 0.7y 2.10.4x 0.2y 1.4
3y 2x 94x 5y 10
x 5, x 2, x 0, x 1, and x 3.
Write each equation in slope-intercept form (solve for y)
and function form, then identify the slope and y-intercept.
31. 32.
33. 34.
35. 36.
37. 38.
For Exercises 39 to 50, use the slope-intercept form to
state the equation of each line. Verify your solutions to
Exercises 45 to 47 using a graphing calculator.
39. 40.
41.
42. y-intercept
43. y-intercept (0, 2)
44. y-intercept
45. is on the line13, 22m 4;
10, 42m
3
2
;
m 3;
10, 32m 2;
(1, 0)
(2, 3)
(0, 3)
54321
54321
1
2
3
4
5
2
3
4
5
1
y
x
x
y
54321
54321
1
2
3
4
5
2
3
4
5
1
(5, 5)
(5, 1)
(0, 3)
x
y
54321
54321
1
2
3
4
5
2
3
4
5
1
(3, 1)
(0, 1)
(3, 3)
5y 3x 20 03x 4y 12 0
2x 5yx 3y
y 2x 45x 4y 20
4y 3x 122x 3y 6
1.4 EXERCISES
cob19545_ch01_134-147.qxd 11/22/10 1:55 PM Page 143
46. is on the line
47. is on the line
48. 49.
50.
Write each equation in slope-intercept form, then use the
rate of change (slope) and y-intercept to graph the line.
51. 52.
53. 54.
Graph each linear equation using the y-intercept and
rate of change (slope) determined from each equation.
55. 56.
57. 58.
59. 60.
61. 62.
Find the equation of the line using the information
given. Write answers in slope-intercept form.
63. parallel to through the point
64. parallel to through the point
65. perpendicular to through the point
66. perpendicular to through the point
67. parallel to through the point
68. parallel to through the point
69. parallel to , through the point (2, 5)
70. perpendicular to through the point (2, 5)y 3
y 3
13, 42
15y 8x 50,
12, 12
12x 5y 65,
15, 32
x 4y 7,
16, 32
5y 3x 9,
13, 52
6x 9y 27,
15, 22
2x 5y 10,
y
3
2
x 2y
1
2
x 3
y 3x 4y 2x 5
y
4
5
x 2y
1
3
x 2
y
5
2
x 1y
2
3
x 3
3x 2y 42x 3y 15
2y x 43x 5y 20
161412810
800
400
1600
2000
1200
x
y
34323026 28
600
300
1200
1500
900
x
y
20181612 14
4000
2000
8000
10,000
6000
x
y
14, 72m
3
2
;
15, 32m 2;
144 CHAPTER 1 Relations, Functions, and Graphs 1–60
College Algebra G&M—
Write the equations in slope-intercept form and state
whether the lines are parallel, perpendicular, or neither.
71. 72.
73. 74.
75. 76.
A secant line is one that intersects a graph at two or
more points. For each graph given, find an equation of
the line (a) parallel and (b) perpendicular to the secant
line, through the point indicated.
77. 78.
79. 80.
81. 82.
Find the equation of the line in point-slope form, then
graph the line.
83.
84.
85.
86.
87.
88. m 1.5; P
1
10.75, 0.1252
m 0.5; P
1
11.8, 3.12
P
1
11, 62, P
2
15, 12
P
1
13, 42, P
2
111, 12
m 1; P
1
12, 32
m 2; P
1
12, 52
(1, 3)
x
y
55
5
5
(0, 2)
x
y
55
5
5
(1, 2.5)
x
y
55
5
5
(1, 3)
x
y
55
5
5
(1, 3)
x
y
55
5
5
(2, 4)
x
y
5
5
5
5
11y 5x 776x 8y 2
5y 11x 1353x 4y 12
2x 3y 64x 3y 18
4x 6y 122x 5y 20
2x 3y 65y 4x 15
3y 2x 64y 5x 8
cob19545_ch01_134-147.qxd 11/1/10 7:21 AM Page 144
Find the equation of the line in point-slope form, and
state the meaning of the slope in context—what
information is the slope giving us?
89. 90.
91. 92.
93. 94.
Eggs per hen per week
Temperature in °F
8075706560
4
2
0
8
10
6
x
y
Cattle raised per acre
Rainfall per month
(in inches)
54321
40
20
0
80
100
60
x
y
Online brokerage houses
Independent investors (1000s)
108645 7 9231
4
2
3
5
7
9
1
0
8
10
6
x
y
Student’s final grade (%)
(includes extra credit)
Hours of television per da
y
5432 2.5 3.5 4.51 1.50.50
40
20
30
50
70
90
10
80
100
60
x
y
Typewriters in service
(in ten thousands)
Year (1990 → 0)
8645 7 92310
4
2
3
5
7
9
1
8
10
6
x
y
Income
(in thousands)
Sales (in thousands)
x
y
8645 7 92310
4
2
3
5
7
9
1
8
10
6
1–61 Section 1.4 Linear Functions, Special Forms, and More on Rates of Change 145
College Algebra G&M—
Using the concept of slope, match each description with
the graph that best illustrates it. Assume time is scaled
on the horizontal axes, and height, speed, or distance
from the origin (as the case may be) is scaled on the
vertical axis.
95. While driving today, I got stopped by a state
trooper. After she warned me to slow down, I
continued on my way.
96. After hitting the ball, I began trotting around the
bases shouting, “Ooh, ooh, ooh!” When I saw it
wasn’t a home run, I began sprinting.
97. At first I ran at a steady pace, then I got tired and
walked the rest of the way.
98. While on my daily walk, I had to run for a while
when I was chased by a stray dog.
99. I climbed up a tree, then I jumped out.
100. I steadily swam laps at the pool yesterday.
101. I walked toward the candy machine, stared at it for
a while then changed my mind and walked back.
102. For practice, the girls’ track team did a series of
25-m sprints, with a brief rest in between.
x
y H
x
y G
x
y F
x
y
E
x
y
A
x
y D
x
y C
x
y B
䊳
WORKING WITH FORMULAS
103. General linear equation:
The general equation of a line is shown here, where
a, b, and c are real numbers, with a and b not
simultaneously zero. Solve the equation for y and
note the slope (coefficient of x) and y-intercept
(constant term). Use these to find the slope and
y-intercept of the following lines, without solving
for y or computing points.
a. b.
c. d. 3y 5x 95x 6y 12
2x 5y 153x 4y 8
ax by c
104. Intercept-Intercept form of a linear
equation:
The x- and y-intercepts of a line can also be found
by writing the equation in the form shown (with
the equation set equal to 1). The x-intercept will be
(h, 0) and the y-intercept will be (0, k). Find the
x- and y-intercepts of the following lines using this
method. How is the slope of each line related to the
values of h and k?
a. b.
c. 5x 4y 8
3x 4y 122x 5y 10
x
h
y
k
1
cob19545_ch01_134-147.qxd 11/22/10 1:56 PM Page 145
146 CHAPTER 1 Relations, Functions, and Graphs 1–62
College Algebra G&M—
䊳
APPLICATIONS
105. Speed of sound: The speed of sound as it travels
through the air depends on the temperature of the
air according to the function where
V represents the velocity of the sound waves in
meters per second (m/s), at a temperature of
Celsius. (a) Interpret the meaning of the slope
and y-intercept in this context. (b) Determine the
speed of sound at a temperature of . (c) If the
speed of sound is measured at 361 m/s, what is the
temperature of the air?
106. Acceleration:A driver going down a straight
highway is traveling 60 ft/sec (about 41 mph) on
cruise control, when he begins accelerating at a rate
of 5.2 ft/sec
2
. The final velocity of the car is given
by where V is the velocity at time t.
(a) Interpret the meaning of the slope and y-intercept
in this context. (b) Determine the velocity of the
car after 9.4 seconds. (c) If the car is traveling at
100 ft/sec, for how long did it accelerate?
107. Investing in coins: The purchase of a “collector’s
item” is often made in hopes the item will increase
in value. In 1998, Mark purchased a 1909-S VDB
Lincoln Cent (in fair condition) for $150. By the
year 2004, its value had grown to $190. (a) Use the
relation (time since purchase, value) with
corresponding to 1998 to find a linear equation
modeling the value of the coin. (b) Discuss what
the slope and y-intercept indicate in this context.
(c) How much was the penny worth in 2009?
(d) How many years after purchase will the penny’s
value exceed $250? (e) If the penny is now worth
$170, how many years has Mark owned the penny?
108. Depreciation: Once a piece of equipment is put
into service, its value begins to depreciate. A
business purchases some computer equipment for
$18,500. At the end of a 2-yr period, the value of the
equipment has decreased to $11,500. (a) Use the
relation (time since purchase, value) to find a linear
equation modeling the value of the equipment.
(b) Discuss what the slope and y-intercept indicate in
this context. (c) What is the equipment’s value after
4 yr? (d) How many years after purchase will the
value decrease to $6000? (e) Generally, companies
will sell used equipment while it still has value
and use the funds to purchase new equipment.
According to the function, how many years will
it take this equipment to depreciate in value to
$1000?
t 0
V
26
5
t 60,
20°C
T°
V
3
5
T 331,
109. Internet connections: The number of households
that are hooked up to the Internet (homes that are
online) has been increasing steadily in recent years.
In 1995, approximately 9 million homes were
online. By 2001 this figure had climbed to about
51 million. (a) Use the relation (year, homes online)
with corresponding to 1995 to find an
equation model for the number of homes online.
(b) Discuss what the slope indicates in this context.
(c) According to this model, in what year did the
first homes begin to come online? (d) If the rate of
change stays constant, how many households were
on the Internet in 2006? (e) How many years
after 1995 will there be over 100 million
households connected? (f) If there are 115 million
households connected, what year is it?
Source: 2004 Statistical Abstract of the United States,
Table 965
110. Prescription drugs: Retail sales of prescription
drugs have been increasing steadily in recent years.
In 1995, retail sales hit $72 billion. By the year
2000, sales had grown to about $146 billion.
(a) Use the relation (year, retail sales of prescription
drugs) with corresponding to 1995 to find a
linear equation modeling the growth of retail sales.
(b) Discuss what the slope indicates in this context.
(c) According to this model, in what year will sales
reach $250 billion? (d) According to the model,
what was the value of retail prescription drug sales
in 2005? (e) How many years after 1995 will retail
sales exceed $279 billion? (f) If yearly sales totaled
$294 billion, what year is it?
Source: 2004 Statistical Abstract of the United States,
Table 122
111. Prison population: In 1990, the number of persons
sentenced and serving time in state and federal
institutions was approximately 740,000. By the year
2000, this figure had grown to nearly 1,320,000.
(a) Find a linear function with corresponding
to 1990 that models this data, (b) discuss the slope
ratio in context, and (c) use the equation to estimate
the prison population in 2010 if this trend continues.
Source: Bureau of Justice Statistics at www.ojp.usdoj.gov/bjs
112. Eating out: In 1990, Americans bought an average of
143 meals per year at restaurants. This phenomenon
continued to grow in popularity and in the year 2000,
the average reached 170 meals per year. (a) Find a
linear function with corresponding to 1990 that
models this growth, (b) discuss the slope ratio in
context, and (c) use the equation to estimate the
average number of times an American will eat at a
restaurant in 2010 if the trend continues.
Source: The NPD Group, Inc., National Eating Trends, 2002
t 0
t 0
t 0
t 0
cob19545_ch01_134-147.qxd 11/1/10 7:21 AM Page 146
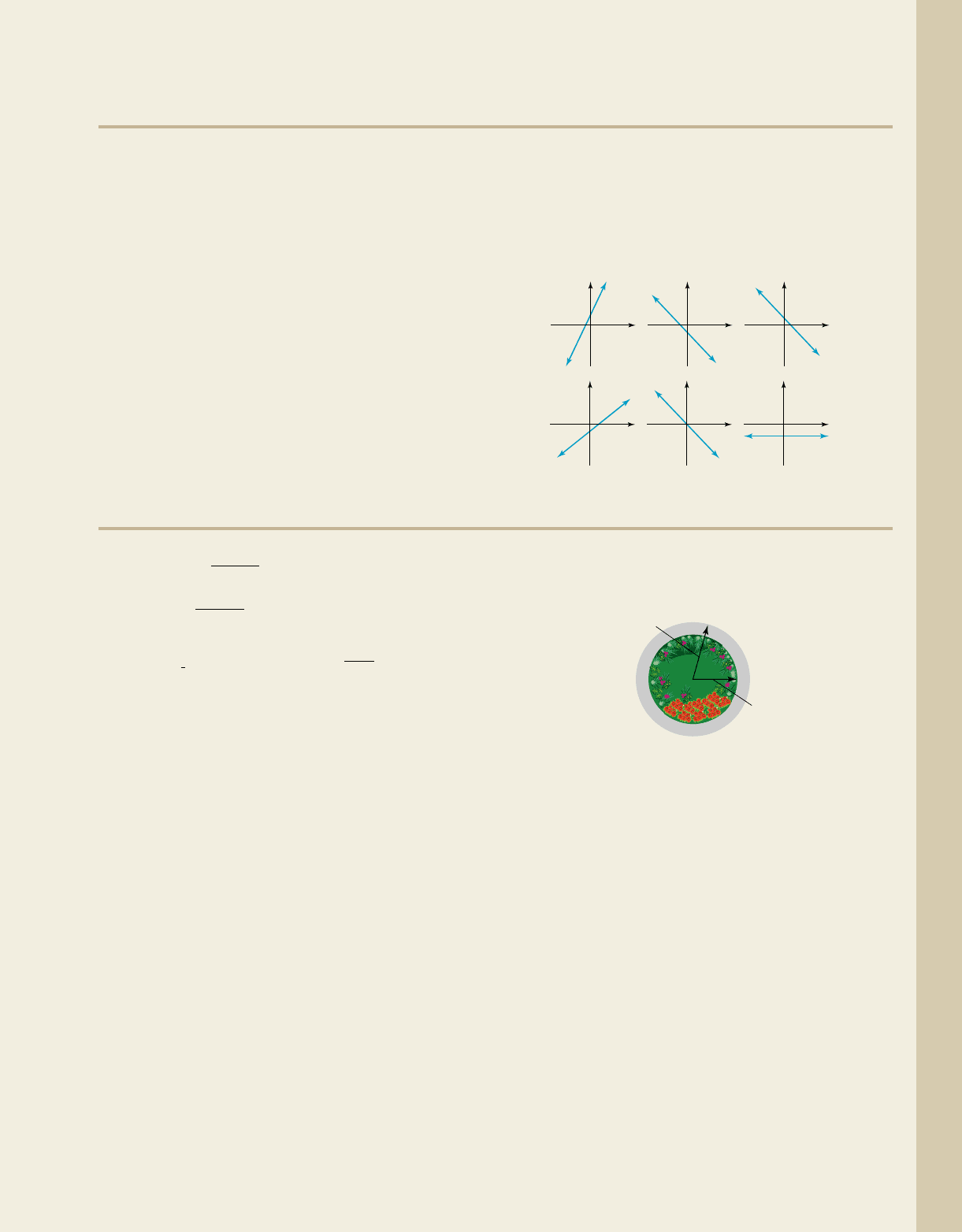
1–63 Section 1.4 Linear Functions, Special Forms, and More on Rates of Change 147
College Algebra G&M—
䊳
EXTENDING THE CONCEPT
113. Locate and read the following article. Then turn in
a one-page summary. “Linear Function Saves
Carpenter’s Time,” Richard Crouse, Mathematics
Teacher, Volume 83, Number 5, May 1990:
pp. 400–401.
114. The general form of a linear equation is
, where a and b are not simultaneously
zero. (a) Find the x- and y-intercepts using the
general form (substitute 0 for x, then 0 for y).
Based on what you see, when does the intercept
method work most efficiently? (b) Find the slope
and y-intercept using the general form (solve for y).
Based on what you see, when does the slope-
intercept method work most efficiently?
ax by c
115. Match the correct graph to the conditions stated for
m and b. There are more choices than graphs.
a. b.
c. d.
e. f.
g. h.
x
y
x
y
x
y
(4) (5) (6)
x
y
x
y
x
y
(1) (2) (3)
m 0, b 6 0m 7 0, b 0
m 6 0, b 0m 0, b 7 0
m 7 0, b 7 0m 6 0, b 7 0
m 7 0, b 6 0m 6 0, b 6 0
䊳
MAINTAINING YOUR SKILLS
116. (1.3) Determine the domain:
a.
b.
117. (R.6) Simply without the use of a calculator.
a. b.
118. (R.3) Three equations follow. One is an identity,
another is a contradiction, and a third has a
solution. State which is which.
21x 52 13 1 9 7 2x
21x 42 13 1 9 7 2x
21x 52 13 1 9 7 2x
281x
2
27
2
3
y
5
2x 5
y 12x 5
119. (R.2) Compute the area of the circular sidewalk
shown here . Use your calculator’s value
of and round the answer (only) to hundredths.
1A r
2
2
8 yd
10 yd
cob19545_ch01_134-147.qxd 11/1/10 7:21 AM Page 147

Note the two expressions are equal (the equation is true) only when the input is .
Solving equations graphically is a simple extension of this observation. By treating
the expression on the left as the independent function Y
1
, we have and
the related linear graph will contain all ordered pairs shown in Table 1.4 (see Fig-
ure 1.67). Doing the same for the right-hand expression yields
and its related graph will likewise contain all ordered pairs shown in the Table 1.5 (see
Figure 1.68).
Y
1
Y
2
The solution is then found where , or in other words, at the point where these
two lines intersect (if it exists). See Figure 1.69.
Most graphing calculators have an intersect feature that can quickly find the
point(s) where two graphs intersect. On many calculators, we access this ability using
the sequence (CALC) and selecting option 5:intersect (Figure 1.70).
TRACE
2nd
Y
1
Y
2
2x 9 31x 12 2
Y
2
31X 12 2,
Y
1
2X 9
x 2
College Algebra G&M—
1.5 Solving Equations and Inequalities Graphically;
Formulas and Problem Solving
In this section, we’ll build on many of the ideas developed in Section R.3 (Solving
Linear Equations and Inequalities), as we learn to manipulate formulas and employ
certain problem-solving strategies. We will also extend our understanding of graphical
solutions to a point where they can be applied to virtually any family of functions.
A. Solving Equations Graphically Using the
Intersect Method
For some background on why a graphical solution is effective, consider the equation
. By definition, an equation is a statement that two expres-
sions are equal for some value of the variable (Section R.3). To highlight this fact, the
expressions and are evaluated independently for selected inte-
gers in Tables 1.4 and 1.5.
31x 12 22x 9
2x 9 31x 12 2
LEARNING OBJECTIVES
In Section 1.5 you will see
how we can:
A. Solve equations
graphically using the
intersection-of-graphs
method
B. Solve equations
graphically using the
x-intercept/zeroes
method
C. Solve linear inequalities
graphically
D. Solve for a specified
variable in a formula or
literal equation
E. Use a problem-solving
guide to solve various
problem types
x 2x ⫺ 9
3 15
2 13
1 11
0 9
1 7
2 5
3 3
Table 1.4
x ⫺3(x ⫺ 1) ⫺ 2
310
27
14
01
1 2
2 5
3 8
Table 1.5
∂
f
10
10
10
10
Figure 1.67
Figure 1.69
Figure 1.68
10
10
10
10
10
10
10
10
148 1–64
cob19545_ch01_148-162.qxd 11/1/10 7:22 AM Page 148

Because the calculator can work with up to 10
expressions at once, it will ask you to identify
each graph you want to work with—even when
there are only two. A marker is displayed on
each graph in turn, and named in the upper left
corner of the window (Figure 1.71). You can
select a graph by pressing , or bypass a graph
by pressing one of the arrow keys. For situa-
tions involving multiple graphs or multiple
solutions, the calculator offers a “GUESS?”
option that enables you to specify the approxi-
mate location of the solution you’re interested in (Figure 1.72). For now, we’ll simply
press two times in succession to identify each graph, and a third time to bypass the
“GUESS?” option. The calculator then finds and displays the point of intersection
(Figure 1.73). Be sure to check the settings on your viewing window before you begin,
and if the point of intersection is not visible, try 3:Zoom Out or other window-
resizing features to help locate it.
EXAMPLE 1A
䊳
Solving an Equation Graphically
Solve the equation using
a graphing calculator.
Solution
䊳
Begin by entering the left-hand expression as Y
1
and the right-hand expression as Y
2
(Figure 1.74).
To find points of intersection, press
(CALC) and select option 5:intersect, which
automatically places you on the graphing
window, and asks you to identify the
“First curve?.” As discussed, pressing
three times in succession will identify each
graph, bypass the “Guess?” option, then
find and display the point of intersection
(Figure 1.75). Here the point of intersection
is ( ), showing the solution to this
equation is (for which both
expressions equal 3). This can be verified
by direct substitution or by using the
TABLE feature.
This method of solving equations is called the Intersection-of-Graphs method,
and can be applied to many different equation types.
x 2
2, 3
ENTER
TRACE
2nd
21x 32 7
1
2
x 2
ZOOM
ENTER
ENTER
1–65 Section 1.5 Solving Equations and Inequalities Graphically; Formulas and Problem Solving 149
College Algebra G&M—
10
10
10
10
10
10
10
10
10
10
10
10
Figure 1.71
Figure 1.70
Figure 1.72
Figure 1.73
Figure 1.74
Figure 1.75
10
10
10
10
cob19545_ch01_148-162.qxd 11/1/10 7:22 AM Page 149
