CIMA - C3 Fundamentals Of Business Mathematics

Подождите немного. Документ загружается.

100 3: Data presentation ⏐ Part B Summarising and analysing data

Class interval Tally marks Total

$25 and less than $30 /// 3

$30 and less than $35 //// 4

$35 and less than $40 ////

//// 10

$40 and less than $45 ////

//// //// 15

$45 and less than $50 ////

//// //// /// 18

$50 and less than $55 ////

//// //// //// 20

$55 and less than $60 ////

//// /// 13

$60 and less than $65 ////

/// 8

$65 and less than $70 ////

/ 6

$70 and less than $75 ///

Total

100

3.7 Cumulative frequency distributions

A cumulative frequency distribution (or cumulative frequency table) can be used to show the total number of times

that a value above or below a certain amount occurs.

There are two possible cumulative frequency distributions for the grouped frequency distribution in Paragraph 3.4.

Cumulative Cumulative

frequency frequency

≥ 700

20 < 800 1

≥ 800

19 < 900 2

≥ 900

18 <1,000 4

≥1,000

16 <1,100 9

≥1,100

11 <1,200 16

≥1,200

4 <1,300 20

(a) The symbol > means 'greater than' and ≥ means 'greater than or equal to'. The symbol < means

'less than' and ≤ means 'less than or equal to'. These symbols provide a convenient method of

stating classes.

(b) The first cumulative frequency distribution shows that of the total of 20 employees, 19 produced 800

units or more, 18 produced 900 units or more, 16 produced 1,000 units or more and so on.

produced under 800 units, two produced under 900 units, four produced under 1,000 units and so

on.

3.8 Frequency distributions – a summary

Students often find frequency distributions tricky. The following summary might help to clarify the different types

of frequency distribution we have covered in this section.

(a) Frequency distribution. Individual data items are arranged in a table showing the frequency each

individual data item occurs.

(b) Grouped frequency distribution – discrete variables. Data items which are discrete variables, (eg

the number of marks obtained in an examination) are divided into classes of say 10 marks. The

numbers of students (frequencies) scoring marks within each band are then grouped into a single

frequency.

Part B Summarising and analysing data ⏐ 3: Data presentation 101

distributions for discrete variables (above.) However, as they are concerned with continuous

variables note the following points.

(i) There is an open-ended class at the end of the range.

(ii)

Class intervals must be carefully considered so that they capture all of the data once (and

only once!).

(d) Cumulative frequency distribution. These distributions are used to show the number of times that a

value above or below a certain amount occurs. Cumulative frequencies are obtained by adding the

individual frequencies together.

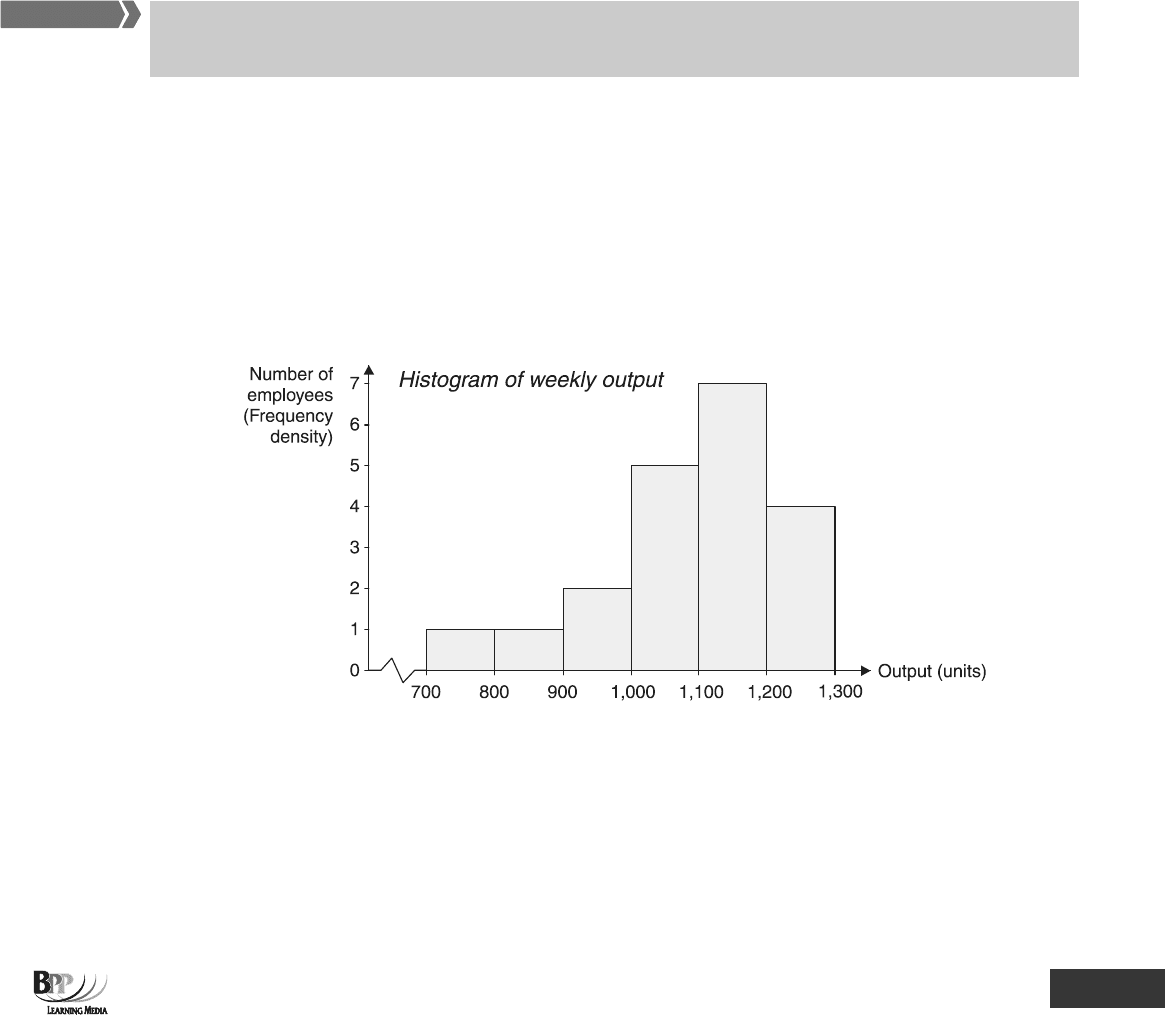
4 Histograms

A frequency distribution can be represented pictorially by means of a histogram. The number of observations in a

class is represented by the area covered by the bar, rather than by its height.

4.1 Histograms of frequency distributions with equal class intervals

If all the class intervals are the same, as in the frequency distribution in Paragraph 3.4, the bars of the histogram

all have the same width and the heights will be proportional to the frequencies. The histogram looks almost

identical to a bar chart except that the bars are joined together. Because the bars are joined together, when

presenting discrete data the data must be treated as continuous so that there are no gaps between class intervals.

For example, for a cricketer's scores in various games the classes would have to be ≥ 0 but < 10, ≥ 10 but < 20

and so on, instead of 0–9, 10–19 and so on.

A histogram of the distribution in Paragraph 3.4 would be drawn as follows.

Note that the discrete data have been treated as continuous, the intervals being changed to >700 but ≤ 800, >800

but ≤ 900 and so on.

4.2 Histograms of frequency distributions with unequal class intervals

If a distribution has unequal class intervals, the heights of the bars have to be adjusted for the fact that the bars

do not have the same width.

T F

RWAR

102 3: Data presentation ⏐ Part B Summarising and analysing data

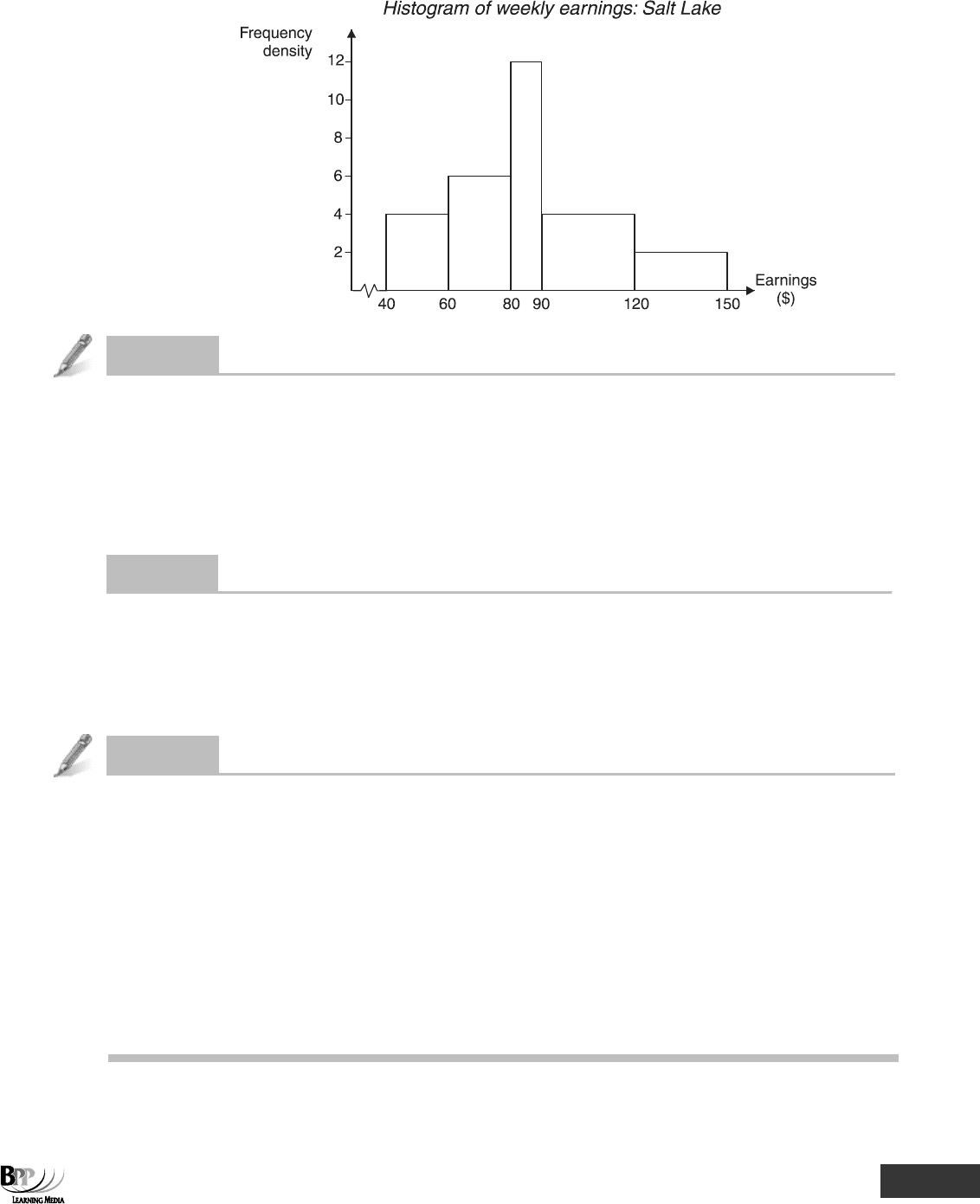
4.2.1 Example: A histogram with unequal class intervals

The weekly wages of employees of Salt Lake Company are as follows.

Wages per employee Number of employees

Up to and including $60 4

> $60 ≤ $80

> $80 ≤ $90

> $90 ≤ $120

More than $120 3

The class intervals for wages per employee are not all the same, and range from $10 to $30.

Solution

A histogram is drawn as follows.

(a) The width of each bar on the chart must be proportionate to the corresponding class interval. In

other words, the bar representing wages of > $60 ≤ $80, a range of $20, will be twice as wide as the

bar representing wages of > $80 ≤ $90, a range of only $10.

(b) A standard width of bar must be selected. This should be the size of class interval which occurs

most frequently. In our example, class intervals $10, $20 and $30 each occur once. An interval of

$20 will be selected as the standard width.

that of the adjoining class. In this example, the class 'up to and including $60' will become >$40 ≤

$60 and the class 'more than $120' will become >$120 ≤ $150.

(d) Each frequency is then multiplied by (standard class width

÷ actual class width) to obtain the

height of the bar in the histogram.

Adjustment factor =

Standard class width

Current class width

(e) The height of bars no longer corresponds to frequency but rather to frequency density and hence

the vertical axis should be labelled

frequency density.

(f) Note that the data is considered to be

continuous since the gap between, for example, $79.99 and

$80.00 is very, very small.

Class interval Size of interval Frequency Adjustment Height of bar

> $40 ≤ $60

20 4

× 20/20

> $60

≤ $80

20 6

× 20/20

> $80

≤ $90

10 6

× 20/10

> $90

≤ $120

30 6

× 20/30

> $120

≤ $150

30 3

× 20/30

(a) The first two bars will be of normal height.

(b) The third bar will be twice as high as the class frequency (6) would suggest, to compensate for the

fact that the class interval, $10, is only half the standard size.

to compensate for the fact that the class interval, $30, is 150% of the standard size.

Formula to

learn

Part B Summarising and analysing data ⏐ 3: Data presentation 103

Question

Histogram (1)

In a histogram in which one class interval is one and a half times as wide as the remaining classes, the height to be

plotted in relation to the frequency for that class is

× 1.5

× 1.00

× 0.75

× 0.67

Answe

If a distribution has unequal class intervals, the heights of the bars have to be adjusted for the fact that the bars do

not have the same width. If the width of one bar is one and a half times the standard width, we must divide the

frequency by one and a half, ie multiply by 0.67 (1/1.5 = 2/3 = 0.67).

The correct answer is D.

Question

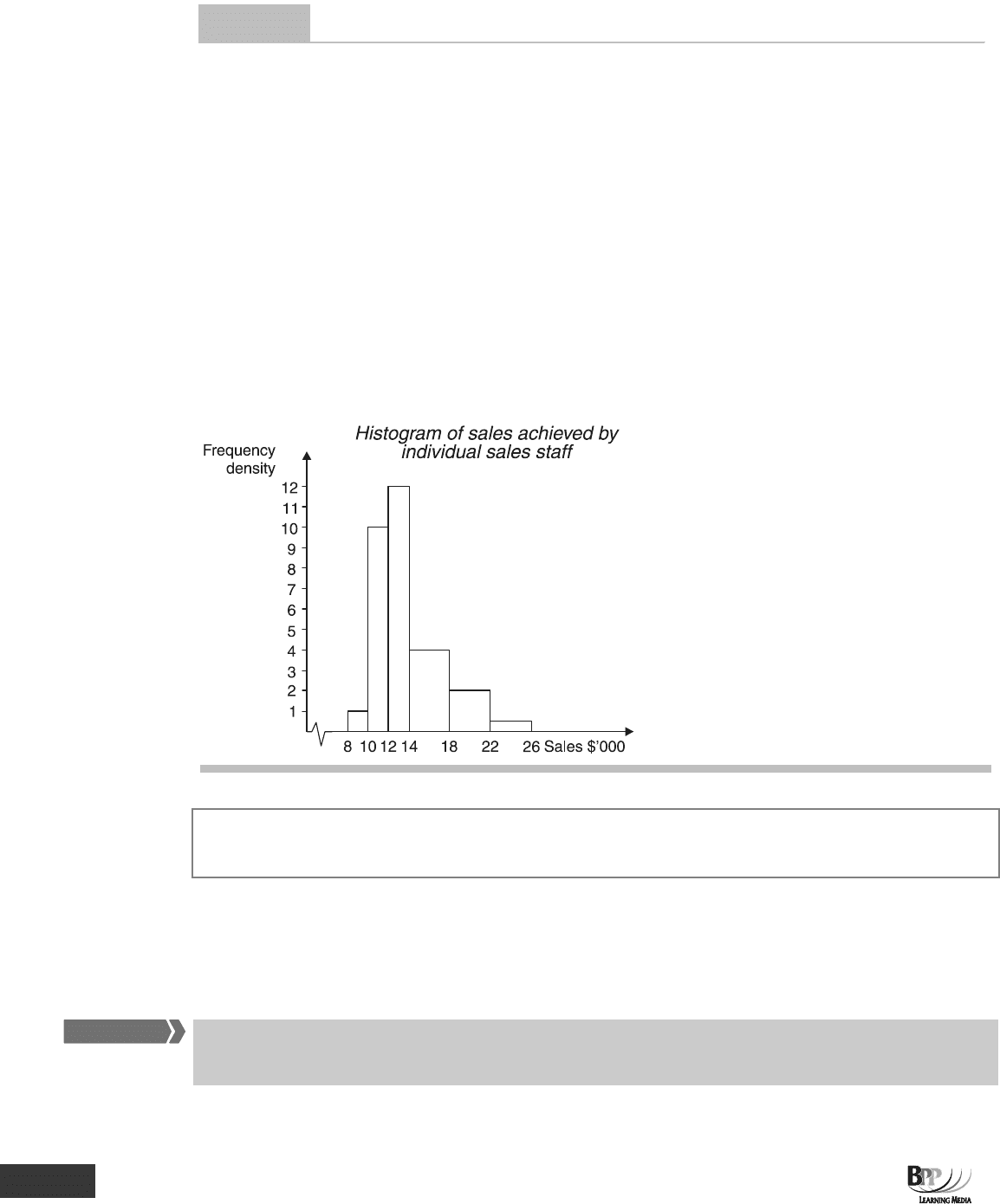
Histogram (2)

The following grouped frequency distribution shows the performances of individual sales staff in one month.

Sales Number of sales staff

Up to $10,000 1

> $10,000

≤ $12,000

> $12,000

≤ $14,000

> $14,000

≤ $18,000

> $18,000

≤ $22,000

> $22,000 1

Required

Draw a histogram from this information

104 3: Data presentation ⏐ Part B Summarising and analysing data

Answe

This is a grouped frequency distribution for continuous variables.

Before drawing the histogram, we must decide on the following.

(a) A

standard class width: $2,000 will be chosen.

(b) An

open-ended class width. In this example, the open-ended class width will therefore be $2,000 for class

'up to $10,000' and $4,000 for the class '> $22,000'.

Size of Height of

Class interval interval Frequency Adjustment bar

Up to $10,000 2,000 1

× 2/2

> $10,000

≤ $12,000

2,000 10

× 2/2

> $12,000

≤ $14,000

2,000 12

× 2/2

> $14,000

≤ $18,000

4,000 8

× 2/4

> $18,000

≤ $22,000

4,000 4

× 2/4

> $22,000 4,000 1

× 2/4

A very common assessment question requires you to work out the heights of bars where there are unequal class

intervals.

5 Ogives

Just as a grouped frequency distribution can be graphed as a histogram, a cumulative frequency distribution can

be graphed as an ogive.

An ogive shows the cumulative number of items with a value less than or equal to, or alternatively greater than or

equal to, a certain amount.

T F

RWAR

Assessment

focus point

Part B Summarising and analysing data ⏐ 3: Data presentation 105

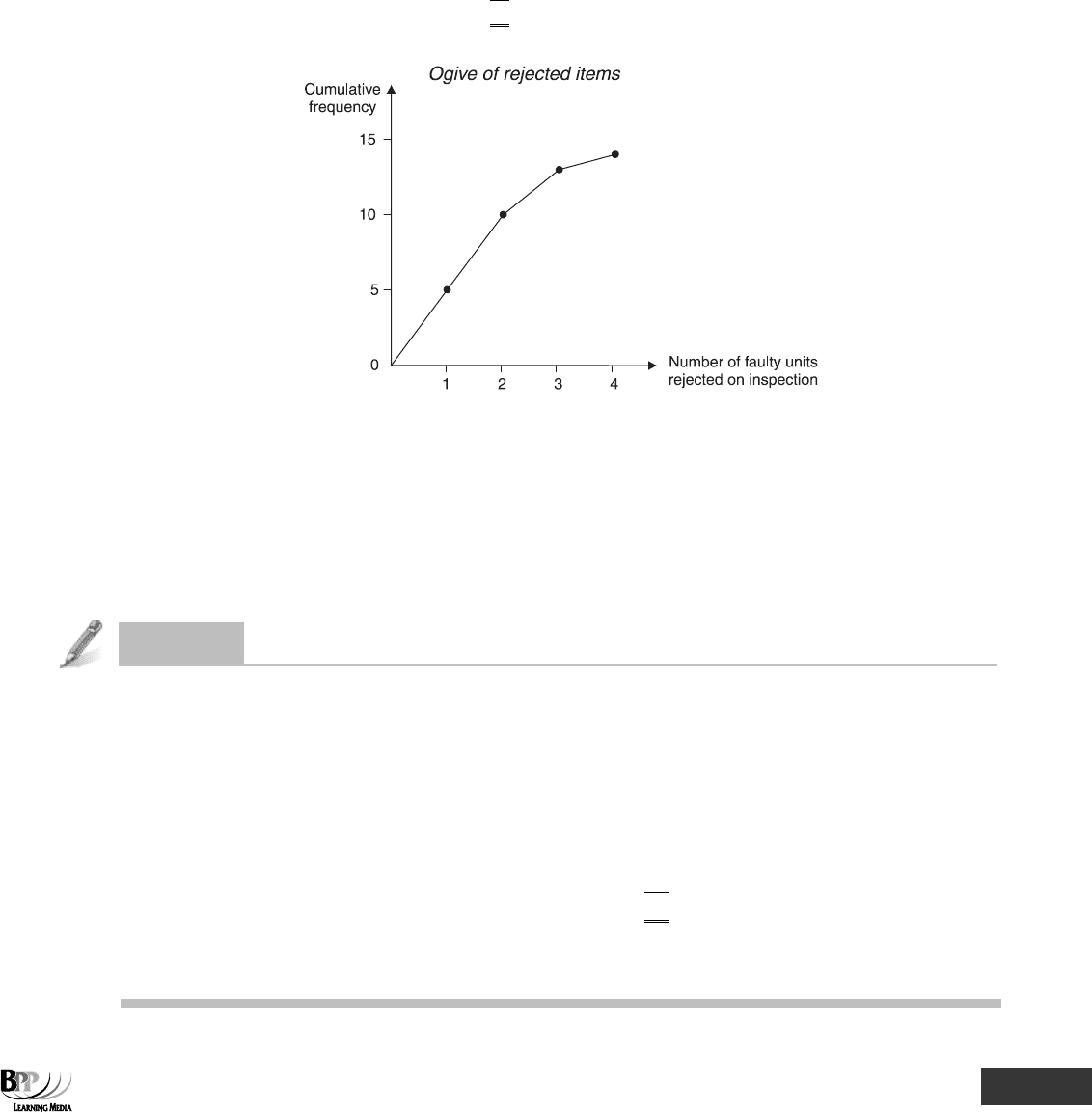
5.1 Example: Ogives

Consider the following frequency distribution.

Number of faulty

Cumulative

units rejected on inspection

Frequency

frequency

> 0,

≤ 1

> 1,

≤ 2

> 2,

≤ 3

> 3,

≤ 4

An ogive would be drawn as follows.

The ogive is drawn by plotting the cumulative frequencies on the graph, and joining them with straight lines.

Although many ogives are more accurately curved lines, you can use straight lines to make them easier to draw. An

ogive drawn with straight lines may be referred to as a cumulative frequency polygon

(or cumulative frequency

diagram) whereas one drawn as a curve may be referred to as a cumulative frequency curve.

For grouped frequency distributions, where we work up through values of the variable, the cumulative frequencies

are plotted against the

upper limits of the classes. For example, for the class 'over 2, up to and including 3', the

cumulative frequency should be plotted against 3.

Question

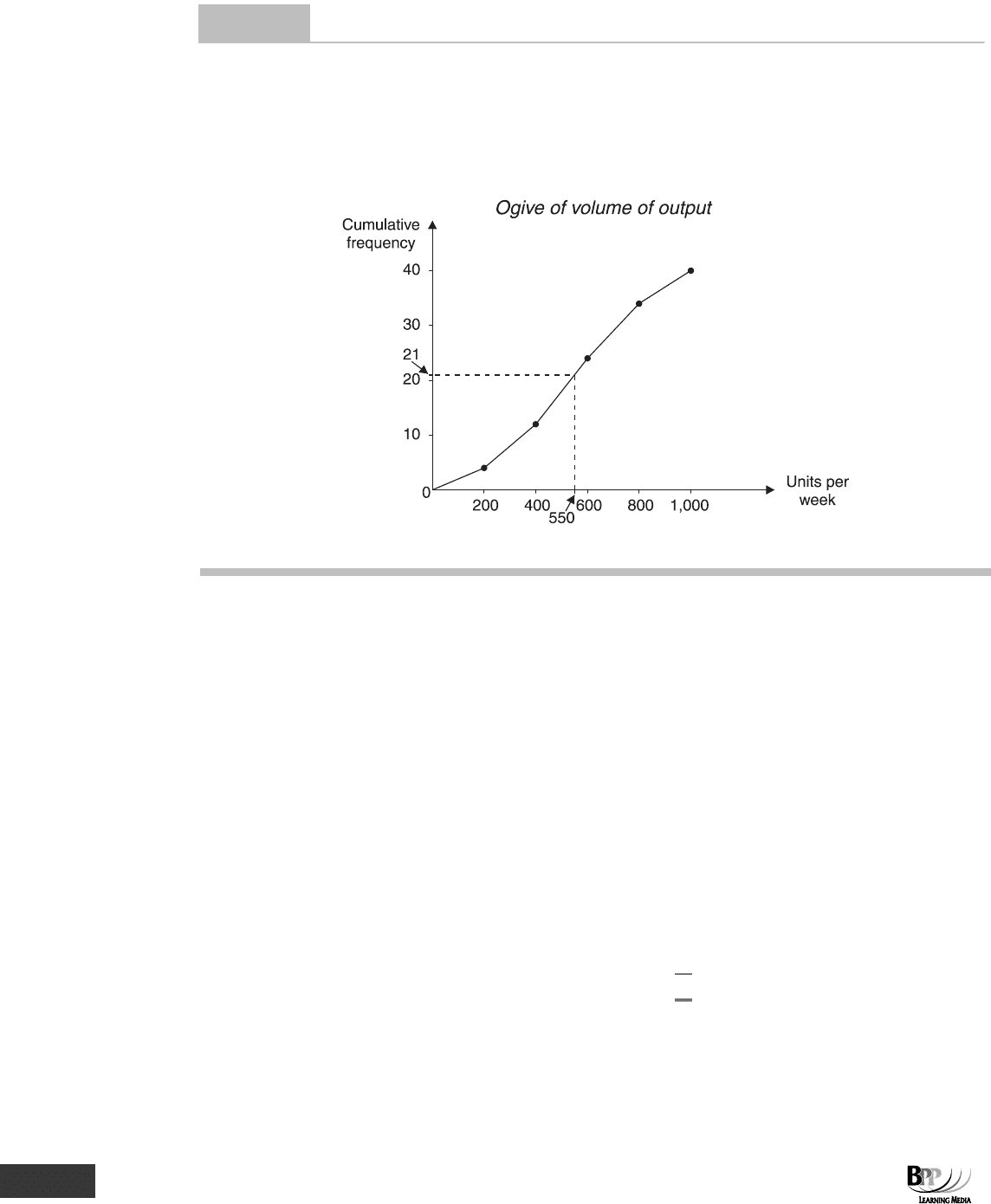
Ogives

A grouped frequency distribution for the volume of output produced at a factory over a period of 40 weeks is as

follows.

Output (units)

Number of times output achieved

> 0

≤ 200

> 200

≤ 400

> 400

≤ 600

> 600

≤ 800

> 800

≤ 1,000

Required

Draw an appropriate ogive, and estimate the number of weeks in which output was 550 units or less.

106 3: Data presentation ⏐ Part B Summarising and analysing data

Answe

Upper limit of interval Frequency Cumulative frequency

200 4 4

400 8 12

600 12 24

800 10 34

1,000 6 40

The dotted lines indicate that output of up to 550 units was achieved in 21 out of the 40 weeks.

5.2 Downward-sloping ogives

We can also draw ogives to show the cumulative number of items with values greater than or equal to some given

value.

5.3 Example: Downward-sloping ogives

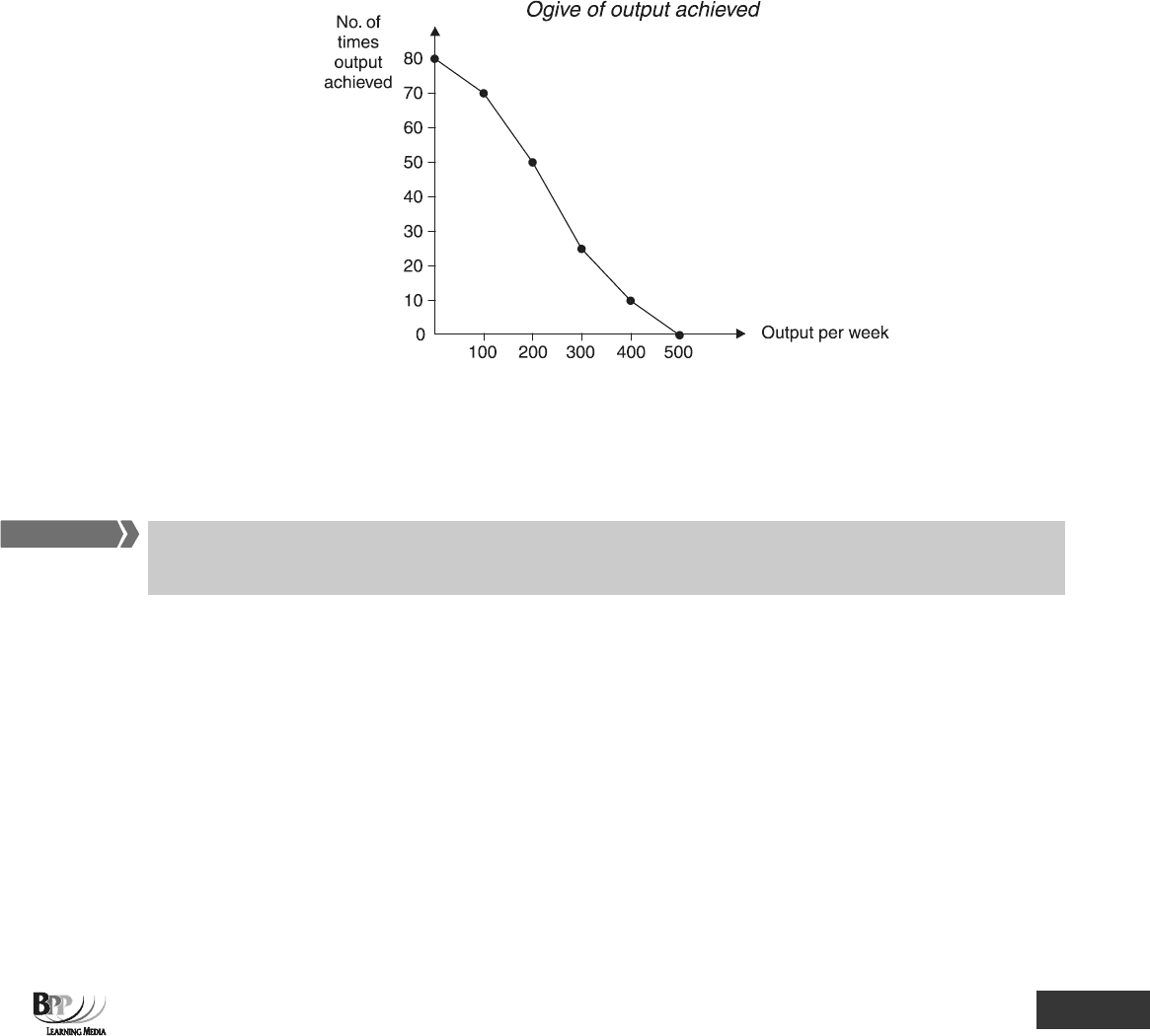
Output at a factory over a period of 80 weeks is shown by the following frequency distribution.

Output per week

Number of times output achieved

Units

> 0

≤ 100

> 100

≤ 200

> 200

≤ 300

> 300

≤ 400

> 400

≤ 500

Required

Present this information in the form of a downward-sloping ogive.

Part B Summarising and analysing data ⏐ 3: Data presentation 107

Solution

If we want to draw an ogive to show the number of weeks in which output exceeded a certain value, the

cumulative total should begin at 80 and drop to 0. In drawing an ogive when we work down through values of the

variable, the

descending cumulative frequency should be plotted against the lower limit of each class interval.

Lower limit of interval Frequency Cumulative ('more than') frequency

0 10 80

100 20 70

200 25 50

300 15 25

400 10 10

500 0 0

Make sure that you understand what this curve shows. For example, 350 on the x axis corresponds with about 18

on the y axis. This means that output of 350 units or more was achieved 18 times out of the 80 weeks.

6 Scatter diagrams

Scatter diagrams are graphs which are used to exhibit data, (rather than equations) in order to compare the way in

which two variables vary with each other.

6.1 Constructing a scatter diagram

The x axis of a scatter diagram is used to represent the independent variable and the y axis represents the

dependent variable.

To construct a scatter diagram or scattergraph, we must have several pairs of data, with each pair showing the

value of one variable and the corresponding value of the other variable. Each pair is plotted on a graph. The

resulting graph will show a number of pairs, scattered over the graph. The scattered points might or might not

appear to follow a trend.

T F

RWAR

108 3: Data presentation ⏐ Part B Summarising and analysing data

6.2 Example: Scatter diagram

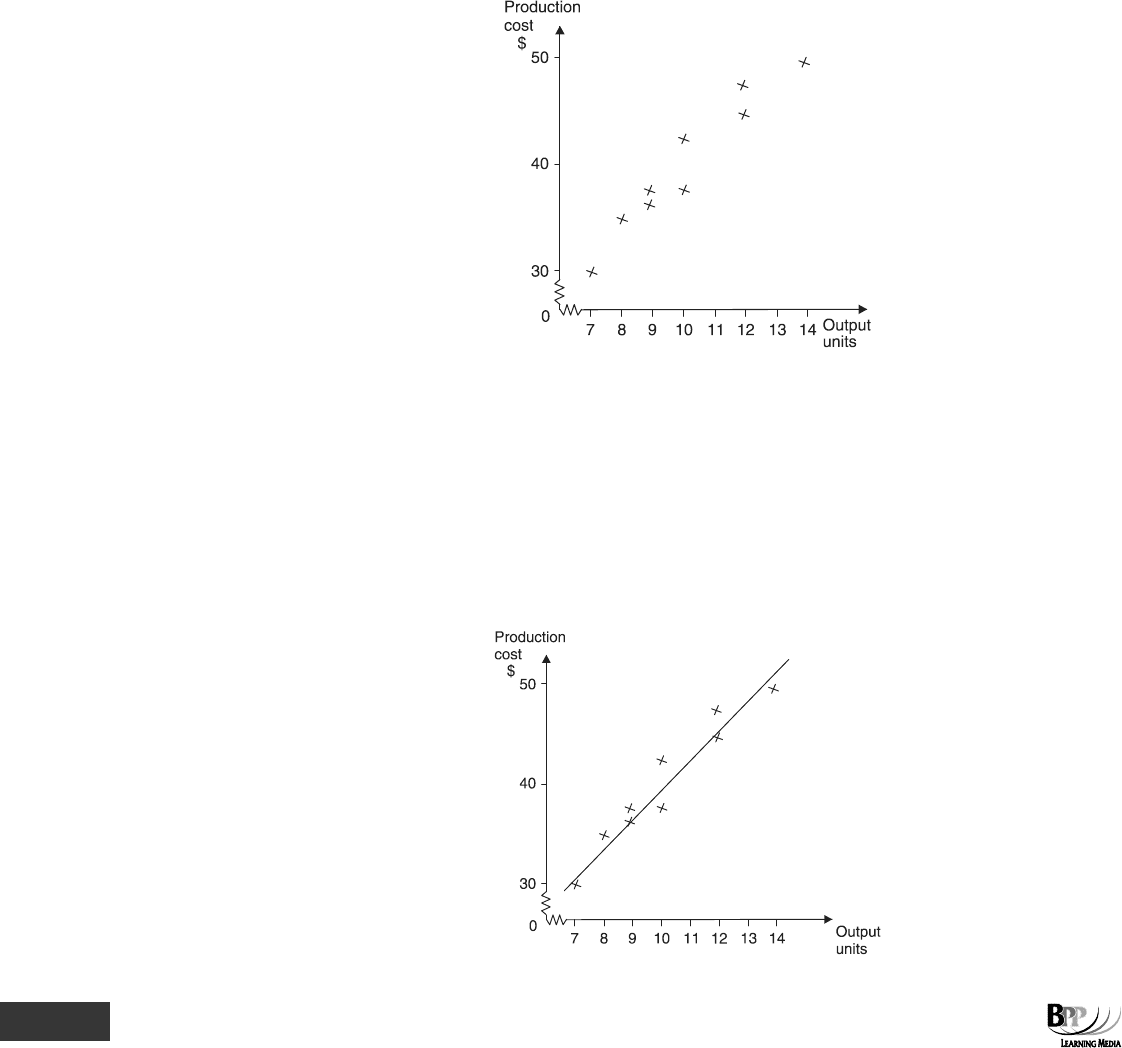
The output at a factory each week for the last ten weeks, and the cost of that output, were as follows.

Week 1 2 3 4 5 6 7 8 9 10

Output (units) 10 12 10 8 9 11 7 12 9 14

Cost ($) 42 44 38 34 38 43 30 47 37 50

Required

Plot the data given on a scatter diagram.

Solution

The data could be shown on a scatter diagram as follows.

(a) The cost depends on the volume of output: volume is the independent variable and is shown on the x axis.

(b) You will notice from the graph that the plotted data, although scattered, lie approximately on a rising trend

line, with higher total costs at higher output volumes. (The lower part of the axes have been omitted, so as

not to waste space. The break in the axes is indicated by the jagged lines.)

6.3 The trend line

For the most part, scatter diagrams are used to try to identify trend lines.

If a trend can be seen in a scatter diagram, the next step is to try to draw a trend line.

Part B Summarising and analysing data ⏐ 3: Data presentation 109

6.3.1 Using trend lines to make predictions

(a) In the previous example, we have drawn a trend line from the scatter diagram of output units and

production cost. This trend line might turn out to be, say, y = 10 + 3x. We could then use this trend

line to establish what we think costs ought to be, approximately, if output were, say, 10 units or 15

units in any week. (These 'expected' costs could subsequently be compared with the actual costs, so

that managers could judge whether actual costs were higher or lower than they ought to be.)

(b) If a scatter diagram is used to record sales over time, we could draw a trend line, and use this to

forecast sales for next year.

6.3.2 Adding trend lines to scatter diagrams

The trend line could be a straight line, or a curved line. The simplest technique for drawing a trend line is to make a

visual judgement about what the closest-fitting trend line seems to be, the 'line of best fit'.

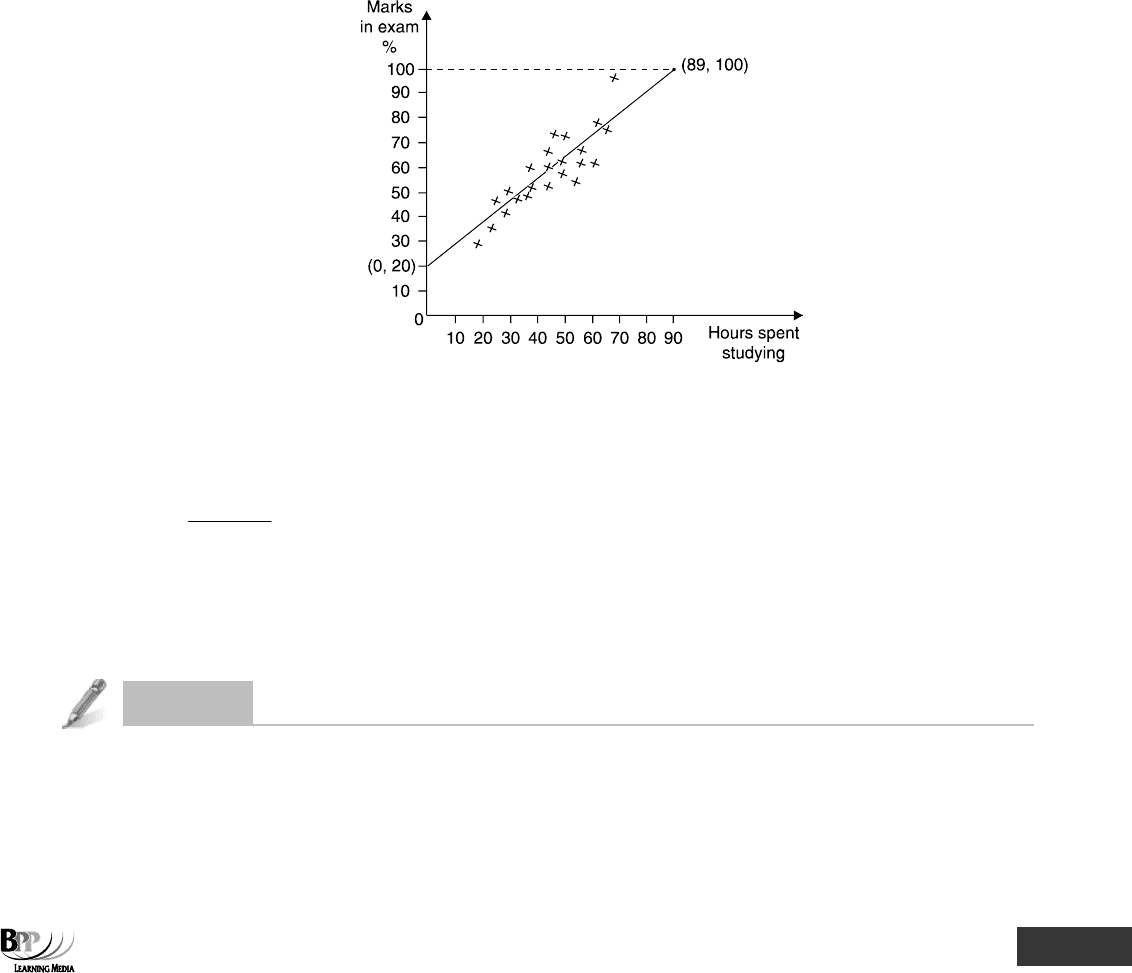
Here is another example of a scatter diagram with a trend line added.

The equation of a straight line is given by

y = a + bx, where a is the intercept on the y axis and b is the gradient.

The line passes through the point x = 0, y = 20, so a = 20. The line also passes through x = 89, y = 100, so:

100 = 20 + (b

× 89)

b =

)20100( −

= 0.9

The line is y = 20 + 0.9x

We will look at this in more detail in Chapter 11 on forecasting.

Question

Definite variables

The quantities of widgets produced by WDG Co during the year ended 31 October 20X9 and the related costs were

as follows.