Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon
Подождите немного. Документ загружается.


64
Chapter 4: Transference Couples and Weighted Maximal Estimates
have (in the notation of (4.27))
{Wkj ,y}j>1 C Mp,_,(R), and
'P,",
({WkJ,,y}j>1) < Kp,w
IIWIIMp.,(R) NP
({kj}j>1)
.
Theorem 4.20 can be extended so as to transfer maximal multiplier esti-
mates from LP(T) to L7(w). Let Mp(Z) be the space of Fourier multipliers for
17(T). Given a sequence of Fourier multipliers {Oj}j>1 C Mp(Z), we denote
by 91p ({lbj}j>1) the strong type (p, p) norm of the maximal multiplier trans-
form on LP(T) corresponding to {l6j}j>1. In particular, for {kj}j>1 C L'(T),
we have Np
({k3}3>1)
= 'tp
({}). The extended version of Theorem
4.20 takes the following form.
Theorem 4.21. Suppose that w E Wp, %Y E Mp,,,,(]R), and the support of WY
is contained in [-1/2,1/2]. Then for each sequence {0j}j>1 C Mp(Z), we
have {Wjj,v}j>1 C MP,u,(R), and
9lp,.
({Wws,',}j>1) C Kp,w II41IIMp,u(R)% ({(bj}j>1)
. (4.28)
Proof. It is enough to establish (4.28) in the case of a finite sequence
N
C
Mp(Z). Thus we wish to show that
91p'.
1) :5 Kp,, IIWIIMp,,(R)`1p ({Oj} 1)
. (4.29)
In the special case where each (bj, 1 < j < N, is finitely supported, there is
a trigonometric polynomial kj such that kj = Oj, and it follows by Theorem
4.20 that (4.29) holds in the case where each cj has finite support.
In order to treat the general case of a sequence {Oj}N j=1 C Mp(Z), let
{jctt}'
0
denote the Fejer kernel for T, and for n > 0, 1 < j < N, put
latt j = l 5,.. Since O,,,j is finitely supported, we have for each n > 0:
,-
1)
Kp,.
I%
and consequently
91".
({Won
}j_1) < Kp pp IIMp.W(R) °.p ({4 } 1) (4.30)
Temporarily fix j in the range 1 < j < N.
It is easy to see that
the sequence IWOn,j,*}0
o
is uniformly bounded on R and tends point-
wise on R to WO" 'F as n -* oo.
It follows that for f E S(R), we have
II
f) - ,p
f)vIIL2(R)
-+ 0, as n -4 oo. Hence as n -+ oo,
II1
sup
I (Wmn.j,k
1)V
1sup
N
I (Wm,, f JV III
y
-3 0.
L (R)

Berkson, Paluszyziski, and Weiss
65
This furnishes a subsequence {sups<j<N
I) vI}
which conver-
ges
v_1
a.e. on R to supl<j<N
I(Wij,. f )vl. Using this together with (4.30),
we
can apply Fatou's Lemma to deduce that for each f E S(R),
sup
(W0j,,p
I<j<N
Kp,w
II LQ(w)
LP(w)
(4.31)
It follows, in particular, that { Wo 4, } j
1
C Mr,. (R). Since S(R) is dense
in L1(w), we can now use (4.31) to obtain (4.29), and thereby complete the
proof of Theorem 4.21.
A straightforward "surgical" procedure will enable us to pass from Theorem
4.21 to the case where %F is assumed to be an arbitrary compactly supported
element of Mp,w(R). The specific outcome is formulated as follows.
Theorem 4.22. Suppose that w E Wp, P E Mp,w(R), and T has compact
support. Let N be a nonnegative integer such that the support of ' is con-
tained in [-N - (1/2), N+ (1/2)]. Then for each sequence
C Mp(Z),
we have
}j>1 S M,,,w(R), and
9tp ,
({Wwj,'p}j>1 ) (2N + 1)Kpw N'IIM,,.(R) "p ({oj}j>1)
.
Proof. We can assume without loss of generality that T vanishes outside
[-N - (1/2), N + (1/2)). For -N < k < N, let Wk = X[k-(1/2),k+(1/2))T,
and let Tk be the translate of 'Pk by k. Thus, 'P = Lk
_N
T k, and, for
-N < k < N, Tk has support contained in [-1/2,1/2]. Given a sequence
{¢j}j>1 C Mp(Z), we apply Theorem 4.21 to Tk. This gives us:
` tp,w (IWo"Tk }j>1) < Kp,w II'`IIM,,W(R) `nP ({(pj}j>1) ,
for - N < k < N.
Since 91p,w ({WOj,rk}j>1) = 9Zp,w ({w,,,vk}j>1), for -N < k < N, we
have
`npw
,yk}j>1)
< Kp,w (I1`IIM,,,(R) 97p
for - N < k < N.
(4.32)
The remainder of the proof is evident from (4.32) and the identity
N
W4,,,y=
for all j> 1.
k=-N

66
Chapter 4: Transference Couples and Weighted Maximal Estimates
We close the discussion with some observations about the relationship
between the existing literature and our results in this section. Theorem 4.22
constitutes a weighted generalization of the unweighted result in [2, Theorem
(1.1)]; that is, Theorem 4.22 essentially reduces to [2, Theorem (1.1)] when
w E Wp is specialized to be the function identically one on R. In the special
case of Theorem 4.20 where w = 1, %F = X(_1/2,1/2), and {k3},>1 is the
Dirichlet kernel for T, we have the unweighted Carleson-Hunt Theorem for
LI(R), 1 < p < oo, which was shown by other methods in [14]. The situation
regarding the weighted Carleson-Hunt Theorem in the setting of R is more
complicated. It is known in the literature that the demonstration in [12]
of the weighted Carleson-Hunt Theorem for T can be adapted to provide a
weighted Carleson-Hunt Theorem for L1(w), w E Ap(R), 1 < p < oo (see the
comments in [10, p. 466]). Theorem 4.20 falls short of this much generality,
since it only provides the weighted Carleson-Hunt Theorem for L1(w) when
wEWW.
References
[1] N. Asmar, E. Berkson, and T. A. Gillespie, Transferred bounds for square
functions, Houston J. Math. (memorial issue dedicated to Domingo Her-
rero), 17 (1991), 525-550.
[2] ,
On Jodeit's multiplier extension theorems, Journal d'Analyse
Math. 64 (1994), 337-345.
[3] P. Auscher and M. J. Carro, On relations between operators on RN, TN
and ZN, Studia Math. 101 (1992), 165-182.
[4] E. Berkson, J. Bourgain, and T. A. Gillespie, On the almost everywhere
convergence of ergodic averages for power-bounded operators on LP-sub-
spaces, Integral Equations and Operator Theory 14 (1991), 678-715.
[5] E. Berkson and T. A. Gillespie, Spectral decompositions and vector-valued
transference, in Analysis at Urbana II, Proceedings of Special Year in
Modern Analysis at the Univ. of Ill., 1986-87, London Math. Soc. Lecture
Note Series 138, Cambridge Univ. Press, Cambridge (1989), 22--51.
[6]
, Mean-boundedness and Littlewood-Paley for separation-preserving
operators, Trans. Amer. Math. Soc. 349 (1997), 1169-1189.
[7]
, Multipliers for weighted D' -spaces, transference, and the q-variation
of functions, Bull. des Sciences Math 122 (1998), 427-454.
Berkson, Paluszyriski, and Weiss
67
[8] E. Berkson, M. Paluszynski, and G. Weiss, Transference couples and their
applications to convolution operators and maximal operators in "Interac-
tion between Functional Analysis, Harmonic Analysis, and Probability,"
Proceedings of Conference at Univ. of Missouri-Columbia (May 29-June
3, 1994), Lecture Notes in Pure and Applied Mathematics, vol. 175, Mar-
cel Dekker, Inc., New York (1996), 69-84.
[9] K. de Leeuw, On Lp multipliers, Annals of Math. 81 (1965), 364-379.
[10] J. Garcia-Cuerva and J. L. Rubio de Francia, Weighted Norm Inequalities
and Related Topics, North-Holland Mathematics Studies 116 = Notas de
Matematica (104), Elsevier Science Publ., New York (1985).
[11] R. Hunt, B. Muckenhoupt, and R. Wheeden, Weighted norm inequalities
for the conjugate function and Hilbert transform, Trans. Amer. Math.
Soc. 176 (1973), 227-251.
[12] R. Hunt and W.-S. Young, A weighted norm inequality for Fourier series,
Bull. Amer. Math. Soc. 80 (1974), 274-277.
[13] M. Jodeit, Restrictions and extensions of Fourier multipliers, Studia
Math. 34 (1970), 215-226.
[14] C. Kenig and P. Tomas, Maximal operators defined by Fourier multipliers,
Studia Math. 68 (1980), 79-83.

Chapter 5
Periodic Solutions of Nonlinear
Wave Equations
Jean Bourgain
We are investigating here the construction by the [5] method of space and
time periodic solutions of one-dimensional nonlinear wave equations of the
following form
Dy + y3 + F(x, y) = 0, F(x, y) = 0(y4).
This more resonant case, due to the absence of a linear term p y, was left aside
in [5]. Second, we consider periodic solutions of certain non-Hamiltonian
NLW, with nonlinearities involving derivatives (of first order). The model
case discussed here is the equation
Ely + py + (yt)2 = 0,
p#o,
but the method is by no means restricted to that example. It mainly demon-
strates the applicability of the Lyapounov-Schmidt decomposition method to
construct periodic (or quasiperiodic) solutions independently of Hamiltonian
structure. In the last section, we construct smooth families in a of periodic
solutions of NLW
Dy+py+y3+ef(x,y) =0
with p = p(E) a parameter depending smoothly in e. This result is in the
spirit of [12, 13]. We also comment on issues concerning admissible frequen-
cies for the perturbed invariant tori.
The author is grateful to C. Wayne for many comments and improvements
of earlier versions of the paper.
69
70
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
5.1
Periodic Solutions of Nonlinear Wave
Equations of the form y + y3 + 0(y4) = 0.
Consider the 1D NLW equation
ytt - Yxx + y3 + F(x, y) = 0,
(5.1)
wherel
F(x, y) = 0(y4).
(5.2)
Thus we have no linear term my, m # 0, at our disposal as in an equation
of the form
ytt - yzx + my + 0(y
3)
= 0 (5.3)
as considered in [5]. We assume F(x, y) is an even periodic function in x and
in fact take for simplicity F of the form
F(x,y) =
aj(x)y',
(5.4)
4<j<d
where the aj are trigonometric cosine polynomials in x (see comments later
on). Our aim is to construct periodic solutions of (5.1) with periods in a set of
positive measure, using the method from [5] combined with some ingredients
from [11]. In [5], equation (5.3) with m 54 0 was considered and the more
resonant case (5.1) left open. In [11], periodic solutions of the equation
ytt-yxx+y3=0
(5.5)
are produced for periods of bounded type (this avoids the small divisor prob-
lems). As in [11], our starting point is the explicit solution by the 4K-periodic
elliptic function
cn ',
00
2 2a '
ex n-t s +e-x n-t z
COS 2K (2n - 1)t;
K
7 n=1
of the differential equation
Cu+C3=0.
'It should be mentioned that, if F(x, y) is x-independent, one may easily construct
periodic solutions of the form y(x, t) = y, (z + At) since the corresponding ODE satisfied
by yl is Hamiltonian. This observation is due to C. Wayne.
Bourgain
71
(See [7] pp. 343, 345).
Rescaling (5.1), letting
y -4 by,
(5.8)
we get the equation
lift - yzz +
62y3 + 63F, (X, y) = 0
(5.9)
(d will be restricted to a neighborhood of 0).
Defining
yo(x,t) = 4K c(4K(x + At)) = c(x + At),
(5.10)
where
A =
l + b2, (5.11)
we get thus a solution of the equation
°yo + o2yo = 0, (5.12)
which is 1-periodic in x and T = A-' periodic in t.
The main idea is to
perform a perturbative procedure starting from yo in order to obtain a so-
lution of (5.9). The perturbation scheme is based on the Newton iteration
procedure as in [5], yielding a quadratic error at each step, hence doubly
exponentially fast convergent. It is therefore less sensitive to small divisor
phenomena than if one would perform an expansion in a 8-series (as in [11]).
During this process, a sequence of restrictions will appear that essentially
will restrict 8 (and hence A) to a Cantor-like set of positive measure (as in
[5]).
The solution y of (5.9) will be given by a Fourier series of the form
y(x,t) _ E &, k) cos 27r(nx + kAt). (5.13)
n,kEZ,n>O
Observe that by (5.6) and (5.10)
yo(x, t) = 8,/2- a E
e7r(n-1/2) + e-a(n-1/2)
cos 21r(2n - 1) (x + At). (5.14)
Thus the assumption on F to be an even and periodic function in x is
consistent with a representation of y in the form (5.13), in the sense that
F(x, y(x, t)) remains a Fourier series of the form (5.13). As will be apparent
later on, treating the problem in generality would lead to certain resonance
problems we do not intend to analyze here.
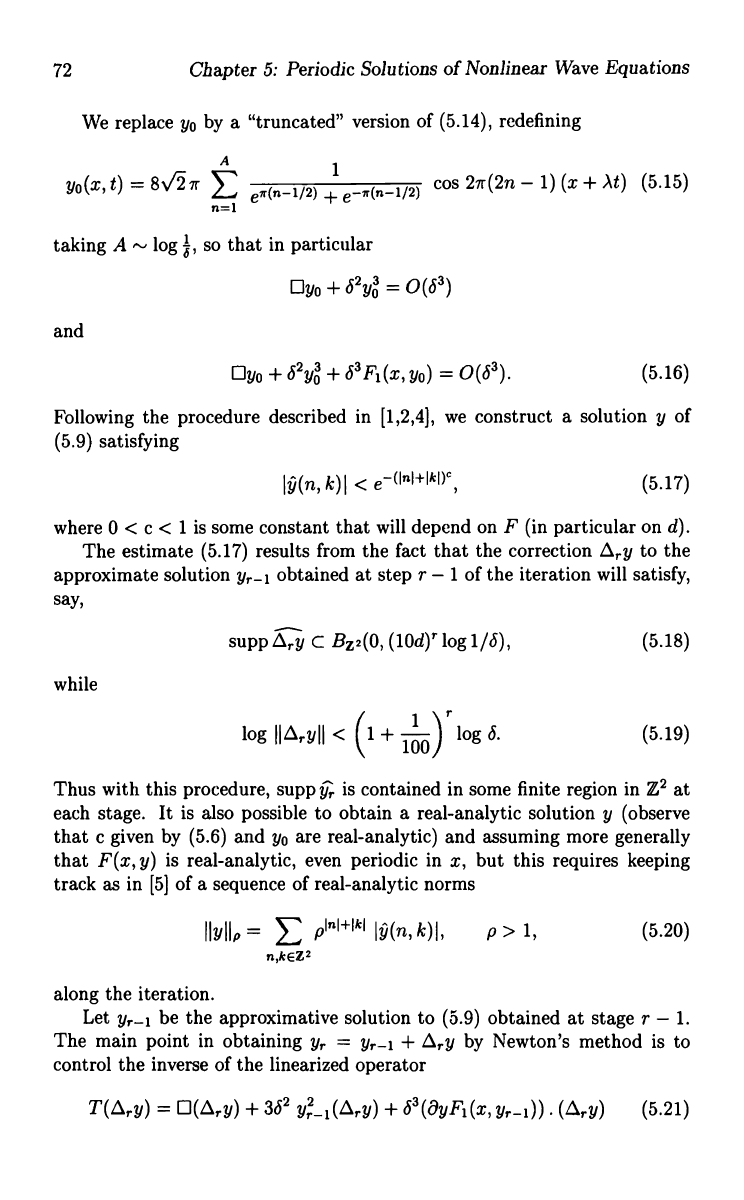
72
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
We replace yo by a "truncated" version of (5.14), redefining
A
yo(x, t) = 8v'2-7r
n=1
1
cos 27r(2n - 1) (x + At)
(5.15)
eir(n-1/2) + e-,r(n-1/2)
taking A .' log
so that in particular
Dyo+62yo =O(63)
and
Dyo + 62yo + 63F1(x,Yo) = O(63).
(5.16)
Following the procedure described in [1,2,4], we construct a solution y of
(5.9) satisfying
Iy(n, k) I < e-(InI+IkU`
(5.17)
where 0 < c < 1 is some constant that will depend on F (in particular on d).
The estimate (5.17) results from the fact that the correction try to the
approximate solution yr_1 obtained at step r - 1 of the iteration will satisfy,
say,
supp try C BZ2(0, (10d)r log 1/6), (5.18)
while
109 Iltryll <
(1
+
100)rlog 5.
(5.19)
Thus with this procedure, supp yr is contained in some finite region in Z2 at
each stage. It is also possible to obtain a real-analytic solution y (observe
that c given by (5.6) and yo are real-analytic) and assuming more generally
that F(x, y) is real-analytic, even periodic in x, but this requires keeping
track as in [5] of a sequence of real-analytic norms
HOP = E PlnI+IkI
I y(n, k)I, p > 1,
n,kEZ2
(5.20)
along the iteration.
Let yr_1 be the approximative solution to (5.9) obtained at stage r - 1.
The main point in obtaining yr = yr_1 + try by Newton's method is to
control the inverse of the linearized operator
T(try) = O(try) + 352 yr_1(try) + b3(8yFi(x, yr-1)) (try)
(5.21)

Bourgain
73
with Z2-lattice representation (expressing T passing to Fourier transform)
T == D + 362 Sy2_1 + 63
where D is the diagonal operator
Dn,k = 41r
2 (-k2 A2
+ n2)
(5.22)
(5.23)
and S, denotes the (Toeplitz) operator with matrix elements
SO (x, x') = (x - x') (5.24)
expressing 4-multiplication in Fourier space.
In order to obtain A ,y with the desired properties, we ensure bounds and
off-diagonal decay estimates on the inverse of restrictions
TM = TI
lnl<M, Ikl<M
(5.25)
of the form
IITMifI < 6-2M° (for some constant C)
(5.26)
and
ITMi(x,x')I < b-2e-zlx-a'I`
for say
Ix - 4 > Ma
(5.27)
(cf. [1]).
One then defines try satisfying TM(try)+PM[b2yr_1+63F1(x, yr_1)] = 0,
where PM refers to the projection on Fourier modes InI < M, IkI < M.
At the first step, we consider for T the operator
D + 362 Syo
(5.28)
to obtain thus
IIAIyII = O(51-)
(5.29)
and
El yl + 52yi + 63 F1 (x, yi) = 0(64-).
(5.30)
The main point is the discussion of T-i. The structure of "singular sites"
when InI+1kI -+ oc has here a simple pattern of lacunarily separated elements
as in [5]. However, there is also the behavior of T,yo (Mo a sufficiently large
constant) that has to be taken into account, depending on specific properties
of yo and thus (5.6).
