Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon

Подождите немного. Документ загружается.

Chapter 5: Periodic Solutions of Nonlinear Wave Equations

In view of (5.26) and (5.27), it will be convenient to renormalize T defining

with, say,

T = 60T'

(5.31)

60 = 10662.

(5.32)

Thus by (5.22), (5.23), and (5.11)

5.33T' = 47r26o 2[n2 - k2(1 + 62)] 1 +3

Sy' +

SaF,(x,y._1)

( )

bo 80

Hence, the off-diagonal part of T' is O(10-10), and we may define the set of

singular sites (depending on 6) as

I, _ {(n, k) E Z+ x Z : In2 -k 2(1 + 82) I < 802}. (5.34)

The inverse of the restriction may then clearly be analyzed with a

Neumann series expansion.

Deleting in [0, do] a set of small relative measure, one may ensure 6 satis-

fying for any fixed y > 1

1+62+nI?IIk 1+6211>c1Ik17 (k,nEZ,k

0). (5.35)

Here we use the notation I

II for the distance to the nearest integer. Let

(n1, k1) E I (n2, k2) E I In, I < Ind. It follows from (5.34) and (5.35) that

62 62

clIn'

- n211

< 1n11, (5.36)

and thus one has the separation property

in, - n2I >

10-12

In1I1/'r.

(5.37)

It is clear from this separation of singular sites that we may choose a

sufficiently large constant MO such that

I, C IZ° U {xa}, xa E I, (5.38)

with

Qo = [0, Mo] x [-Mo, Mo] C Z2,

(5.39)

dist (xa, Ho) > Ixa 10,

(5.40)

Ixa - X#I >

Ixa11/2

for a 54 Q.

(5.41)

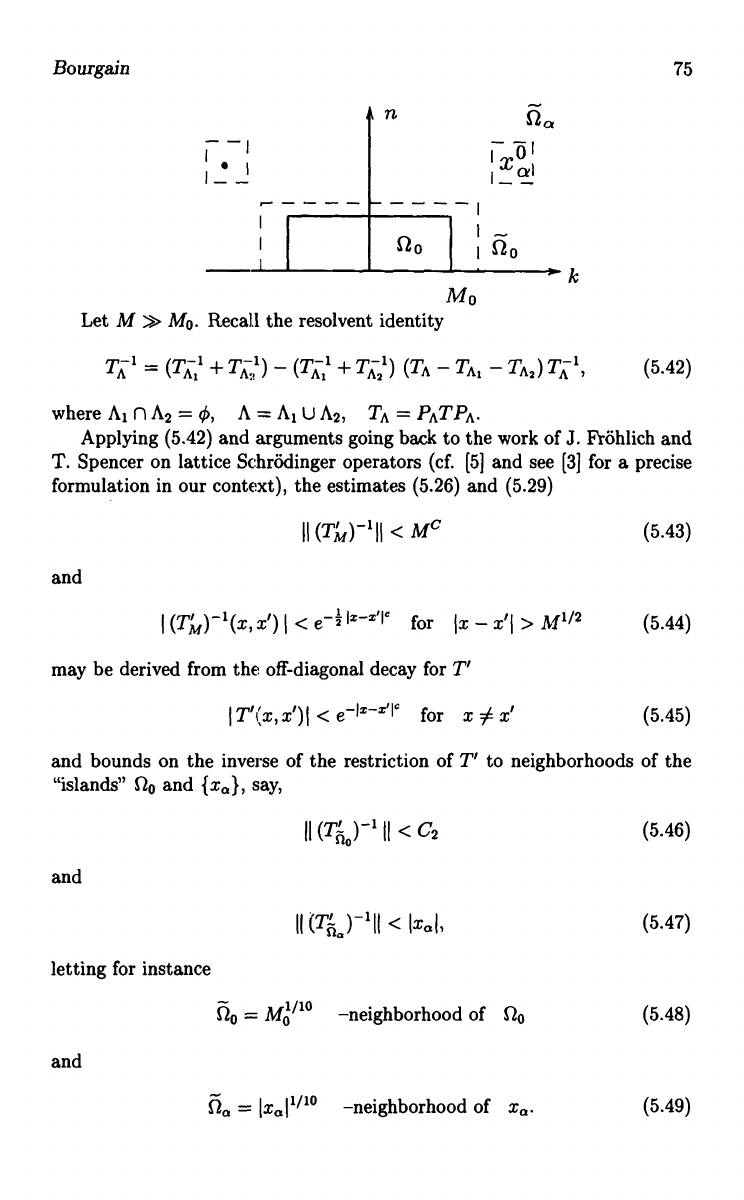
Bourgain

Let M >> M°. Recall the resolvent identity

xcrl

Tn' = (T,,' + T ') - (Tall + Tn2') (TA

- TA, - TA,) Tn',

(5.42)

where A,flA2=0, A=A, UA2i TA=PATPA.

Applying (5.42) and arguments going back to the work of J. Frohlich and

T. Spencer on lattice Schrodinger operators (cf. [5] and see [3] for a precise

formulation in our context), the estimates (5.26) and (5.29)

II (TM)-111 < M°

and

(5.43)

I (TM)-'(x, x') I <

e-2 Ix-x'I`

for Ix - x'I > M112 (5.44)

may be derived from the off diagonal decay for '

for x # x'

(5.45)

and bounds on the inverse of the restriction of T' to neighborhoods of the

"islands" S20 and {xa}, say,

II (T0)-' II < C2 (5.46)

and

(TU-'Ii < Ixal,

(5.47)

tti f i t

ng ns ance

ore

S20 = M0I/10

-neighborhood of

00 (5.48)

and

a =

IxaI'/10

-neighborhood of

xa. (5.49)

Chapter 5: Periodic Solutions of Nonlinear Wave Equations

To obtain (5.47), we proceed simply by first-order eigenvalue variation,

using 62 as parameter. Observe that T' and its restrictions are self-adjoint.

Thus if Tnav -= pv, IvI2 = 1, (5.33) yields

a2)

2} v, v) N -41r2k'oo 2 +

0(1) J02

-Ixal2bo 2,

(5.50)

where xa = (na, ka) E I. Remark that

ey,)

W-11.

(5.51)

The main contribution is given by the first increment A I y = 63Tno F1 (x, yo).

From (5.50) and appropriate restrictions of 6, one may then fulfill (5.47).

Considering consecutive size regions Inl + IkI - 26, we first restrict 6 to

small intervals on which the singular sites set {xa} and hence S2a, S2a may

be fixed. A further restriction of 6 using (5.50) then allows us also to ensure

the properties (5.47). This process is similar to the one in [5].

Next, we turn our attention to condition (5.46), which is the core of the

matter. Thus we need to ensure that

(Tl?jo)

Recall (5.39). We assume

Decompose first

where

-1

< C2 b-2. (5.52)

Mo S2 < 10-6.

(5.53)

no = 9' U S2", (5.54)

Q'= {(n, k) E S2o I n2 $ k2}. (5.55)

Since, for (n, k) E S2', by (5.53)

In2-k2(1+b2)I> In 2-k21-M062>

(5.56)

it follows from (5.33) that

IIT51III <1.

(5.57)

Bourgain 77

Writing Tno as block matrix

Too =

To,

Tn.

where U = O(82), (5.52) reduces thus to

(Tn,,

- U* Tst 1U)-1

C6-2

(5.58)

or, since yr-1 = Yo + 0(8) and (5.33), to

11 (-47r2k2lln,, + 3 Pn,,SSoPn,')-' [[ < C.

(5.59)

Write further

Sl" = Q11 U lift

", (5.60)

where

Sl+={(k,k)I0<k<Mo} and Sl"={(k,-k)I 0<k<Mo}. (5.61)

Since

supp yo C {(n, k) E Z2 I n = k},

(5.62)

it follows from (5.61) and (5.62) that

Pn+SvoPoll = 0 = Pn'l SSgPn+ and

P,,- yo(0, 0) lic, . (5.63)

Therefore, (5.59) clearly reduces to the following two statements

II [PM0 (-47r2k21 + 3 S,,) PMo ]'' 11 < C

(5.64)

-47r2k2 + 3

where c is given by (5.6) and (5.10), i.e.,

for all

k E Z, (5.65)

14vF2 ir E 1 cos 27r (2n - 1)

n=1

ch(n - 2)9r

(5.66)

and PMo denotes the projection on the linear span of even functions

[cos 27rkx 10:5 k < Mo]. (5.67)

Chapter 5: Periodic Solutions of Nonlinear Wave Equations

Verification of (5.64).

Assuming

f = f (k) cos 27rke,

(5.68)

k=0

we need to show that

11PMo(f"+362f)112>

111112.

(5.69)

Consider the Sturm-Liouville operator

Q =2 + 3(c)2

(5.70)

with 1-periodic potential 3(c)2, acting on 1-periodic functions. Then L2(T)

is the direct sum of invariant spaces of even and odd functions. We are here

only interested in the behavior on the space of even functions. It follows from

(5.7) and (5.10) that

(c)" + (e)3 = 0 (5.71)

and hence (e)' satisfies

Q(c)' = 0. (5.72)

It follows that 0 is in the periodic (in fact in the Dirichlet) spectrum of Q.

Since c is even, (e)' is odd. Assume 0 is also in the spectrum of Qiepr, where

epf denotes even periodic functions. Thus

Qcp = 0,

cp 96 0

even periodic.

(5.73)

Since (e)', cc are linearly independent, the Wronskian

= (O)'cp' - P" W

(5.74)

does not vanish identically. On the other hand, (5.72) and (5.73) imply that

= (e)'cP" -

(e)"c

= 0,

(5.75)

so that W has to be a nonzero constant. Thus

(5.76)

Bourgain

Now, by (5.74) and (5.71)

f()3cp

W= -2 = 2 (5.77)

while by (5.74) and (5.73) also

W=-2

E<p"=6

(5.78)

and (5.76), (5.77) and (5.78) are clearly contradictory. Thus 0 is not in the

spectrum of Q I (where of denotes even functions) and there is some constant

a > 0 so that

IlQ

fl12 > a II f 112 for f even. (5.79)

Coming back to (5.68) and (5.69) write

11 PMo (f" + 3(E)2f) P2 Ilf"+3PMo(E2f)112

IIQf112-311(E)2f-PMo(2f)112

112 -

11((Z)2f )" 112

II Qf 112-M (Ilf"112+IIf 112)

M) I1Qf112-M Ilfll2

t t l

)

o] 11 f 112

(by (5.80)

11 f 112

if we let MO be large enough and for some constants A and B.

Verification of (5.65).

From (5.66), we need to verify that

12 1

0 {k21 k E Z},

n=1

[ch (n - 2) 7r]

and this expression equals 1.9.. .

(5.80)

Hence (5.64) and (5.65) and thus (5.59), (5.58), (5.52) and (5.46) hold,

required to establish (5.43), (5.44) and (5.26), (5.27). This completes the

discussion of the linearized operator in the Newton scheme. Thus, following

[2], [3], and [5], we obtain the following.

Chapter 5: Periodic Solutions of Nonlinear Wave Equations

Theorem 5.1. Consider the equation

Oy + y3 + F(x, y) = 0

(5.81)

with

F(x, y) =

aj(x) y3

(5.82)

j=4

and aj trigonometric cosine polynomials. Then (5.81) has periodic solutions

(1-periodic in space and A-1-periodic in time) of the form

y(x, t) = 4 K 6 en (4K(x + At)) + O(52-) (5.83)

with

A= 1+52

(5.84)

for 5 taken in a set of positive measure (the relative measure --+ 1 when

5->0).

5.2

Periodic Solutions of Certain Non-

Hamiltonian Nonlinear Wave Equations

As pointed out in [1] and [5], the construction of time periodic and quasiperi-

odic solutions using the Lyapounov-Schmidt decomposition and the Newton

iteration method is a priori not restricted to Hamiltonian problems. Our pur-

pose here is to treat a model example, suggested to the author by C. Wayne

(private communication), a NLW of the form

ytt - y:: + py + (yt)2 = 0,

p ¢ 0 (5.85)

(for p = 0, there are only constant solutions).

Recall that our method consists of dividing the problem into an infinite-

dimensional piece (the P-equations) containing small divisor difficulties and

a remaining finite set of equations (the Q-equations) used to determine the

parameters. Essentially speaking, the main ingredient of relevance in solving

the P-equations is the geometric structure of "singular sites" of the linearized

equation, which technically often constitutes the hardest part of the problem.

The methods of [5], as sketched in the example of the previous section and [l]

appear as rather general implicit function type results, largely independent of

Hamiltonian structure. Most of the structure appears in the context of the Q-

equations, deduced in the Hamiltonian case from an appropriate normal form.

Bourgain

They determine the free parameters and are solved by the ordinary implicit

function theorem. In example (5.85), the following difficulties appear.

(i)

The appearance of derivatives in the nonlinearity. As pointed out

in [1], the arguments used there to construct time-periodic or quasiperiodic

solutions permit us in the NLW-case to consider also first-order derivatives.

The type of nonlinearity considered in [1] was of the form

& 1/2

(

dx2

F(x, y)

(5.86)

corresponding to a Hamiltonian problem

yt=Bv

vt = -By + F(x, y),

where

1/2

dx2)

(5.87)

As far as solving the P-equations, one may consider more generally a

nonlinear term of the form

F(x, y, axy, aty)

(5.88)

and in particular equation (5.85). One observation here is that in the pres-

ence of derivatives in the nonlinear perturbation, already in the construction

of time-periodic solutions, the structure of the singular sites of the linearized

operators presents some of the difficulties of the quasiperiodic case. In par-

ticular, one does not have the separation properties at infinity appearing in

the [5] work. Indeed, in the case (5.87), the linearized operator T expressed

on the

Z1+1-lattice has the form

(-(k, A) 2 + n2) Il + inl SooF(x,y) (5.89)

-(k, A)2 + n2

Il+S

aF(x

)

(5.90)

Ini

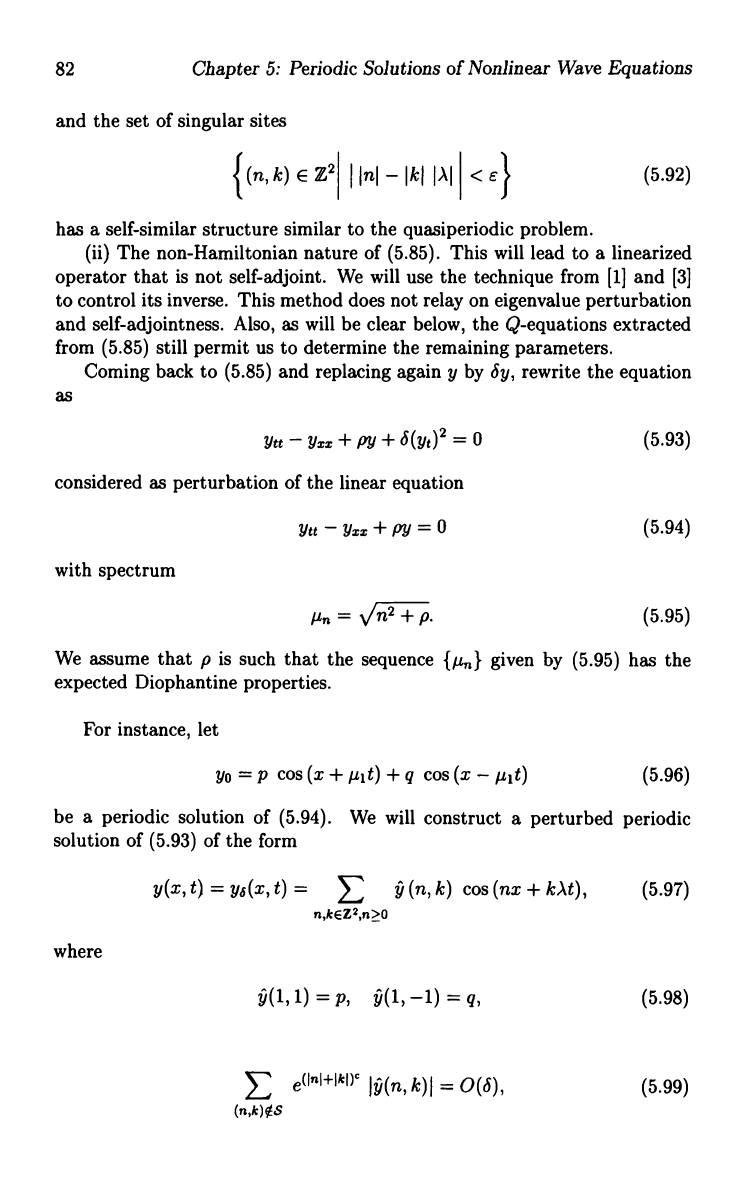
In the periodic case, the diagonal part is thus

k2,\2 + n2

0 91)(5,

InI

n .

Chapter 5: Periodic Solutions of Nonlinear Wave Equations

and the set of singular sites

I (n, k) E Z2

Inj - IkI JAI

< e1 (5.92)

has a self-similar structure similar to the quasiperiodic problem.

(ii) The non-Hamiltonian nature of (5.85). This will lead to a linearized

operator that is not self-adjoint. We will use the technique from [1] and [3]

to control its inverse. This method does not relay on eigenvalue perturbation

and self-adjointness. Also, as will be clear below, the Q-equations extracted

from (5.85) still permit us to determine the remaining parameters.

Coming back to (5.85) and replacing again y by 8y, rewrite the equation

ytt - yxx + py + 5(yt)2 = 0

(5.93)

considered as perturbation of the linear equation

Ytt-yxx+py=0

(5.94)

with spectrum

An =

n2 + p. (5.95)

We assume that p is such that the sequence {µn} given by (5.95) has the

expected Diophantine properties.

For instance, let

yo = p cos (x + µ1t) + q cos (x - pit)

(5.96)

be a periodic solution of (5.94). We will construct a perturbed periodic

solution of (5.93) of the form

y(x, t) = yo(x, t) = E y (n, k) cos (nx + kAt),

(5.97)

n,kEZ2,n>O

where

y(1,1)

= p,

y(1, -1) = q,

(5.98)

E e(Inl+IkD` fy(n,

k) I = 0(6),

(5.99)

(n,k)fS

Bourgain

with

the resonant set and where

is the perturbed frequency.

S = 1(11 1), (1, -1)}

(5.100)

A2 = 1+p+0(62)

(5.101)

Remark: One may construct space and time-periodic solutions of (5.85) of

the form y(x, t) = c(x + At), where c satisfies the ODE

c'+A2 1(c)2+A2

1c=0

(observation due to C. Wayne). This equation has indeed small amplitude

periodic solutions which will in turn give space-time periodic solutions of

equation (5.85).

This is the reason why we have chosen an unperturbed

solution of the form (5.96); cf. [11].

Expressing (5.93) in Fourier yields

(-k2A2 + n2 + P) y(n, k) + 6 (yt)2 (n, k) = 0.

(5.102)

The P-equations (resp.

Q-equations) are obtained restricting (k, n) V S

(resp. (k, n) E S). Starting from yo given by (5.96), we get

(yo)t =° -A,(p sin(x + Alt) - q sin(x - pit))

(5.103)

and thus from (5.102)

= p cosy (x + Alt) + q cpos (x - tilt)

+ 2 1

+ 4(1 A )-Fp cos (2x +

2plt)

1_a )+p cos (2x -

2plt) - 2M cos 2x -

4-+

2pg

cos Aplt}

+ 0(62).

Hence

Yt = -Pi ({p sin (x + Alt) - q sin

(x - Alt) }

- dpi { 4(1_A2)}p sin (2x + 21Alt)

4(1_A2))-FP

sin (2x - 2pit) - A% sin 2plt } + 0(52).