Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon
Подождите немного. Документ загружается.


84
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
Specifying (n, k) = (1, 1) and (n, k) = (1, -1), substitution of the previous
equation in (5.102) yields for the Q-equations
2 2
+ 0(5) = 0
11 - \2 + p +
4(1-,\2)+ p - q
1 - A2 + p + 62,14
9(1-- P - _
+ 0(53) = 0.
4A -p
(5.104)
Assume p > 0, p # 4/3 fixed. For A2 = 1 + p + O(52), one may then solve
(5.104) for p = p(A), q = q(A). In order to be able to solve the P-equations
by Newton's method, a sequence of conditions on p, q, A are imposed that
eventually will restrict A down to some Cantor-like set C of positive measure,
such that for A E C one gets a solution of (5.93) of the form (5.97) with
p = p(A), q = q(A) in (5.98).
We now turn our attention to the P-equation. The linearization of (5.102)
at a given approximative solution y yields the linearized operator
T = (-k2A2 + n2 + p)Il + 25[S1
, o
(kA)1].
(5.105)
What follows is a bit easier in the present context of time-periodic solu-
tions than in the general quasiperiodic case, due to the fact that here, for
singular sites, necessarily ski - InI.
Use (5.101) to write
-k 2,\2 + n2 + p = (1 - k2)p + n2 - k2 + O(k262).
(5.106)
Since we excluded the resonant cases (n, k) = (1, 1) and (n, k) = (1, -1)
(n > 0) and p is assumed to fulfill typical Diophantine conditions, no singular
sites appear in the region Int + Iki < Mo = Mo(5) = 6-c'. Applying the
resolvent identity, the control of T-1 may then be achieved from that of Ti',
where A is a box of the form
A+= 1L<n< 11 L]
x
[
10AL<k<
12 L]
(5.107)
or
1110LIX[
L<-k<1012A]
(5.108)
with L > io MO (5), say. Assume (n, k) restricted to a box A+, say. Instead
of considering T, one may invert
T,_ -k2A2+n2+p
Il + 2SS
(5 109)
k

Bourgain
85
restricted to A+ (this operator is not self-adjoint since it is the sum of a
diagonal and an antisymmetric matrix). Defining the set of singular sites I,
of T' as the pairs (n, k) E A+ such that (c2 < cl sufficiently small)
I-k2 A2+n2+pl
<E",
kA
we get, since k > M,
(5.110)
Ika - nI < 5". (5.111)
This permits us to conclude a separation of the singular sites by &-e3, for
some c3 > 0.
Indeed, if (nl, kl) E I (nzi kz) c I and Ikl - k2I < b, it
follows from (5.101) and (5.111) that
II (kl - k2) 1 + plI < 36". (5.112)
Hence, since p is assumed Diophantine,
Ik1 - kzI `° < II(ki - k2)2p1I < 10b°zIkl - k21,
(5.113)
Ik1 -
k2$
>
10
-c2/I+C > 8-C3
implying the previous statement about separation.
Our aim is to get for suitable restrictions of the parameters p, q, A again
good (polynomial) bounds on the inverse (TT+)-1 and off-diagonal decay.
This is achieved by an inductive process considering restrictions of the oper-
ator to boxes Q C A+ of increasing size scale, much in the spirit of [1], [3],
and [8]. These boxes will be obtained by restriction of the k-index only, thus
Q
IL<n< 10L]
xJ,
(5.114)
where J is an interval. in [-!-L L < k <
-L] of a certain size o(L). The
multiscale analysis of the inverse of
TQ
=
-k2,\2kan2
+
p
II + SSIQ
(5.115)
will relate to size scales of J. Observe that in (5.115) k, n - L.
Restricting T' to the complement of I,, the inverse is controlled by a
Neumann series.
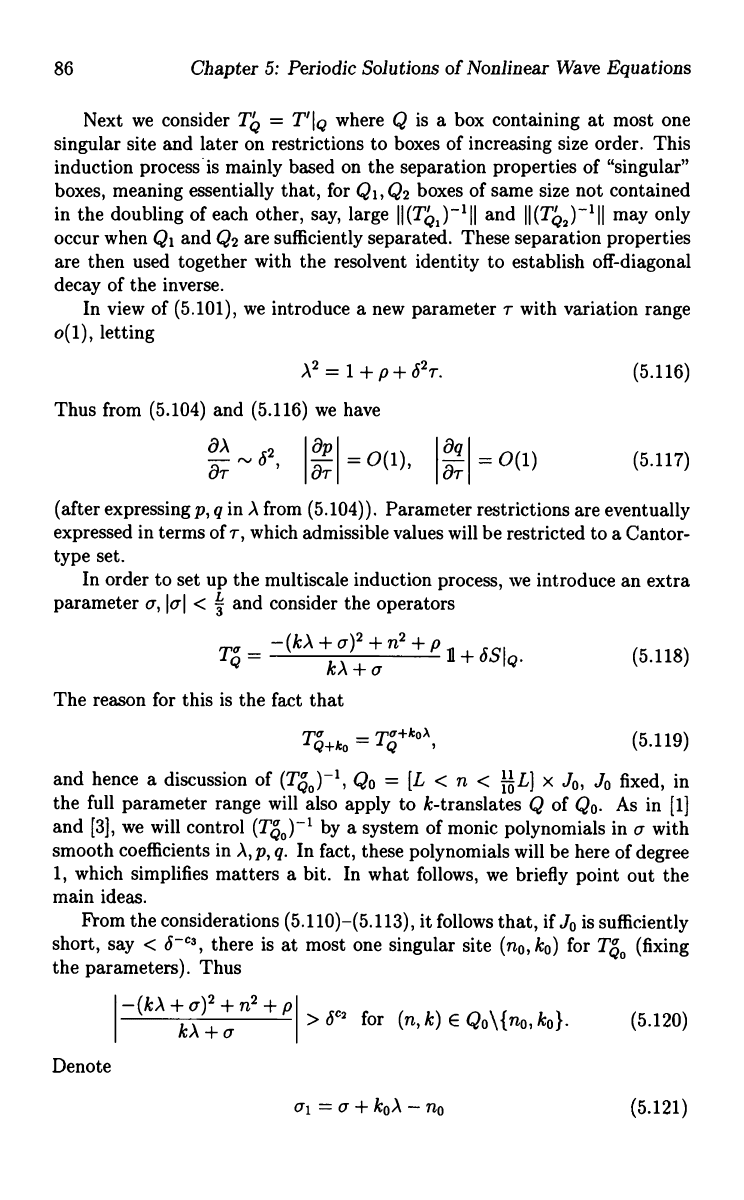
86
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
Next we consider TT = T`IQ where Q is a box containing at most one
singular site and later on restrictions to boxes of increasing size order. This
induction process is mainly based on the separation properties of "singular"
boxes, meaning essentially that, for Q1, Q2 boxes of same size not contained
in the doubling of each other, say, large
II(T4,)-1II and
II(T4s)-1II
may only
occur when Ql and Q2 are sufficiently separated. These separation properties
are then used together with the resolvent identity to establish off-diagonal
decay of the inverse.
In view of (5.101), we introduce a new parameter r with variation range
0(1), letting
A2=1+p+b2r. (5.116)
Thus from (5.104) and (5.116) we have
ar N
b2, ap
= 0(1),
Ilq
I
= 0(1) (5.117)
OIT
(after expressing p, q in A from (5.104)). Parameter restrictions are eventually
expressed in terms of r, which admissible values will be restricted to a Cantor-
type set.
In order to set up the multiscale induction process, we introduce an extra
parameter a, Jul < a and consider the operators
TZ -(kA+o)2+n2+pI+bS(Q
(5.118)
Q
kA+Q
The reason for this is the fact that
T°+koa
T°
o - - Q
(5.119)
and hence a discussion of (TQo)-1, Qo = [L < n < 1L] x Jo, Jo fixed, in
10
the full parameter range will also apply to k-translates Q of Qo. As in [1]
and [3], we will control
(TQo)-1 by
a system of monic polynomials in or with
smooth coefficients in A, p, q. In fact, these polynomials will be here of degree
1, which simplifies matters a bit. In what follows, we briefly point out the
main ideas.
From the considerations (5.110)-(5.113), it follows that, if Jo is sufficiently
short, say < b-`3, there is at most one singular site (no, ko) for TQo (fixing
the parameters). Thus
(kA+Q)2+n2+p
CZ
b f
k
k
or (n,
o}.
) E Qo\{no,
kA + o
I
>
(5.120)
Denote
Ql = Q + koa - no
(5.121)
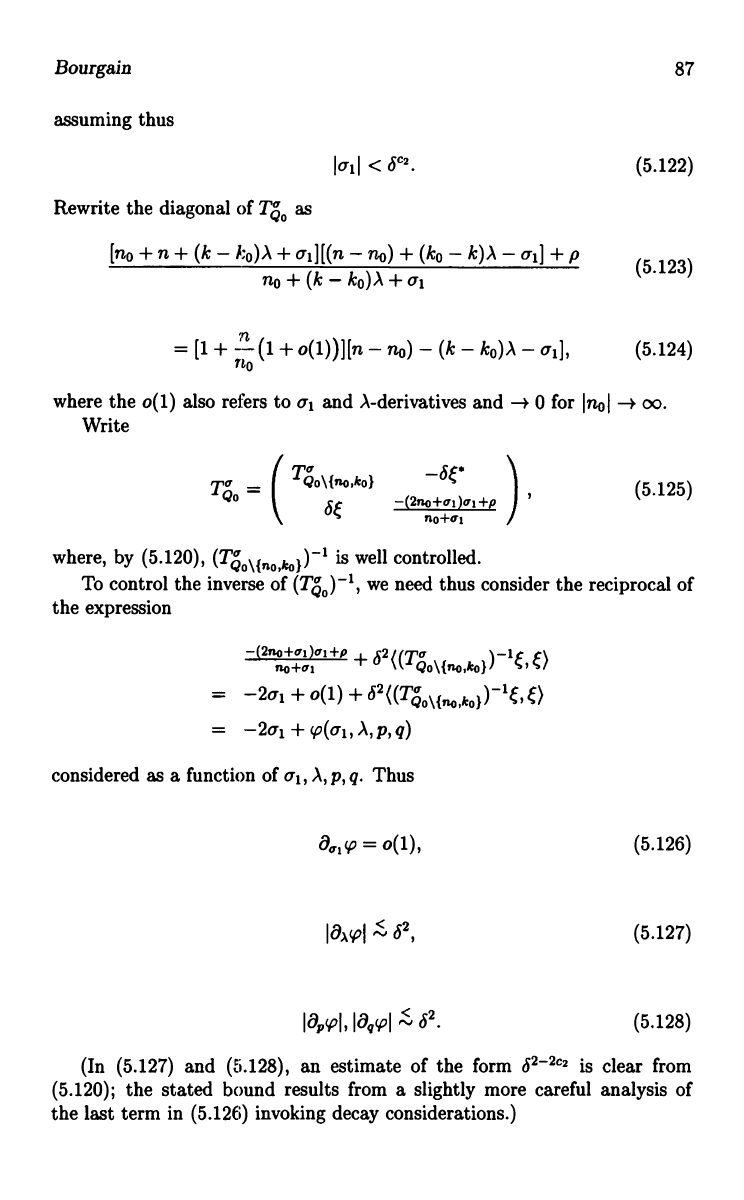
Bourgain
87
assuming thus
lo1I < 812
(5.122)
Rewrite the diagonal of TQo as
[no+n+(k-ko)A+al][(n-no)+(ko-k)A-o1]+p
no +(k-ko)A+o1
(5.123)
_ [1 +
n
(1 + o(1))][n - no) - (k - ko)A - 01], (5.124)
710
where the o(1) also refers to o1 and A-derivatives and - 0 for Inol -i oo.
Write
T_ Tea\{no,ko}
-g-
-
St -(2*b+oj)
Z.
no+al
(5.125)
where, by (5.120), (TQo\{no,ko})-1 is well controlled.
To control the inverse of (TQo)-1, we need thus consider the reciprocal of
the expression
e
-(2no+oi)o1+P
2 0 1
n0+01
+(1 ((TQ0\{n0,ko})-CCS,ttS)
-201 +o(j)+S2 ((To\{no,ko})-1S, t)
-201 + W(o1 i A, R q)
considered as a function of o1i A, p, q. Thus
o1+P
i%1V = 0(1),
(5.126)
aavl N 82,
(5.127)
1,9,Vl, lagwvl N 62.
(5.128)
(In (5.127) and (5.128), an estimate of the form 62-2c2 is clear from
(5.120); the stated bound results from a slightly more careful analysis of
the last term in (5.126) invoking decay considerations.)

88
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
From the implicit function theorem, one may then replace (5.126) by
al - 'b(A, p, q), (5.129)
where z/, still satisfies (5.127) and (5.128).
Observe that, from (5.121), if we let a = 0,
aa,
8a
Nko - L>Mo. (5.130)
Hence, considering A, p, q as a function of T, weI have
[koa - no - ii(A,p, q)] > M082
(5.131)
by invoking (5.117).
In order to obtain separation of "singular" matrices TT, and TQ2 with
Qi = Qo + ki
(i=1,2)
(5.132)
and
Ik, - k21 >> IQoI,
(5.133)
one has to avoid simultaneous almost vanishing of two expressions
ai -
V51 (A, p, q)
0, (5.134)
a2 - 02(A, p, q)
0, (5.135)
where
ai = a + ko,iA - no,,,
Iki - ko,il < IQoI
(5.136)
If (5.134), (5.135), and (5.136) hold, we have (eliminating a)
(ko,l - ko,2)A - no,l + no,2 - zlii (A, p, q) + 02(A, p, q)
0. (5.137)
Hence
II(ko,i - k0,2)A-Vi(A,p,q)+V,2(A,p,q)II
0, (5.138)
where
Iko,i - ko,21 >> IQoI
(5.139)

Bourgain 89
To avoid this, we use the fact that
as
[(ko,l - ko,2).\ -'1(A,p, q) + 2(',p, q)] '
ko,1 - ko,2. (5.140)
Hence
19
[(ko,i - ko,2)A - 01 (A, p, q) + 2(A, p, q)]
> IQoI52
(5.141)
The preceding is the first step in the multiscale analysis. For the continu-
ation of the process, we refer the reader to [1] and [3]. This permits us for
the restrictions TQ, Q c A±, to get estimates on the inverse that are pow-
erlike in IQI with exponential off-diagonal decay; similarly for the inverse of
restrictions of T as needed in the Newton scheme. For details, the reader is
referred to [1] and [3].
The result of the preceding is the following.
Theorem 5.2. Consider a NLW equation
yet - yxx + py + (yt)2 = 0
(5.142)
with periodic boundary conditions, where p > 0 is a typical number. Then
for b sufficiently small taken in a Cantor set of positive measure, (5.139) has
a time-periodic solution of the form (5.97)-(5.99)
y(x, t)* = p cos(x + at) + q cos(x - .\t)
+ En>o,(n,k)#(1,1),(1,-1)
y(n, k)
cos(nx + At),
where
(5.143)
p = p(5), q = q(5) = O(5) and A2 = 1 + p + 0(52)
(5.144)
and the last term in (5.140) is 0(52).
5.3
Some Remarks on Frequencies and
Parameters
Consider a perturbed Hamiltonian system
8Ho
+ 6
OH1
(5.145)
iq=
84
ag,
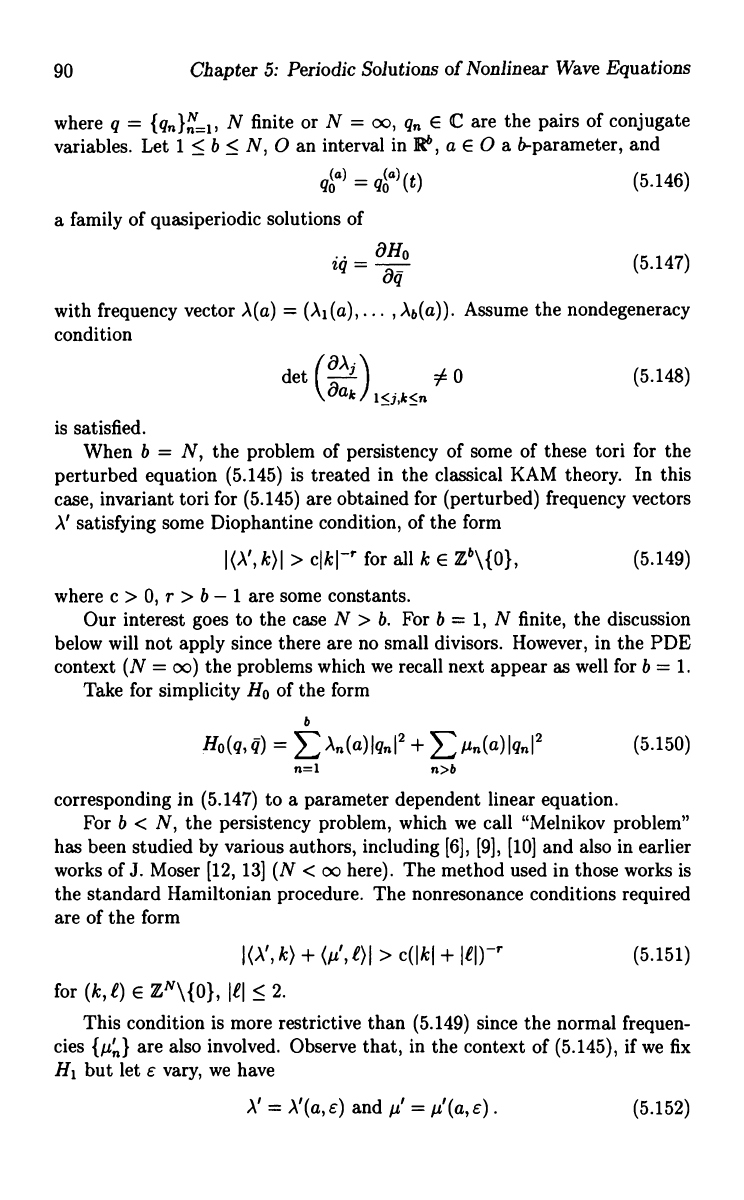
90
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
where q = {qn}n 1, N finite or N = oo, q,, c C are the pairs of conjugate
variables. Let 1 < b < N, 0 an interval in Rb, a E 0 a b-parameter, and
qoa)
=
qo 1(t)
(5.146)
a family of quasiperiodic solutions of
=
&
qoi
147)
(54
.
with frequency vector .A (a) _ (AI(a), ... , J1b(a)). Assume the nondegeneracy
condition
det I a2 I # 0
\ 8ak / 1< j,k <n
(5.148)
is satisfied.
When b = N, the problem of persistency of some of these tori for the
perturbed equation (5.145) is treated in the classical KAM theory. In this
case, invariant tori for (5.145) are obtained for (perturbed) frequency vectors
A' satisfying some Diophantine condition, of the form
I(A',k)I > clkl-r for all k E Zb\{0},
(5.149)
where c > 0, r > b - 1 are some constants.
Our interest goes to the case N > b. For b = 1, N finite, the discussion
below will not apply since there are no small divisors. However, in the PDE
context (N = oo) the problems which we recall next appear as well for b = 1.
Take for simplicity Ho of the form
b
Ho(q,4) _ Ean(a)Ignl2+E{ln(a)Jq.J'
(5.150)
n=1
n>b
corresponding in (5.147) to a parameter dependent linear equation.
For b < N, the persistency problem, which we call "Melnikov problem"
has been studied by various authors, including [6], [9], [10] and also in earlier
works of J. Moser [12, 13] (N < oo here). The method used in those works is
the standard Hamiltonian procedure. The nonresonance conditions required
are of the form
J(A', k) + V, t) I > c(JkJ + IiI)`
(5.151)
for (k, t) E Z "\{0}, It < 2.
This condition is more restrictive than (5.149) since the normal frequen-
cies {µ;a} are also involved. Observe that, in the context of (5.145), if we fix
H1 but let a vary, we have
A' = A'(a, e) and p' = µ'(a, e)
. (5.152)
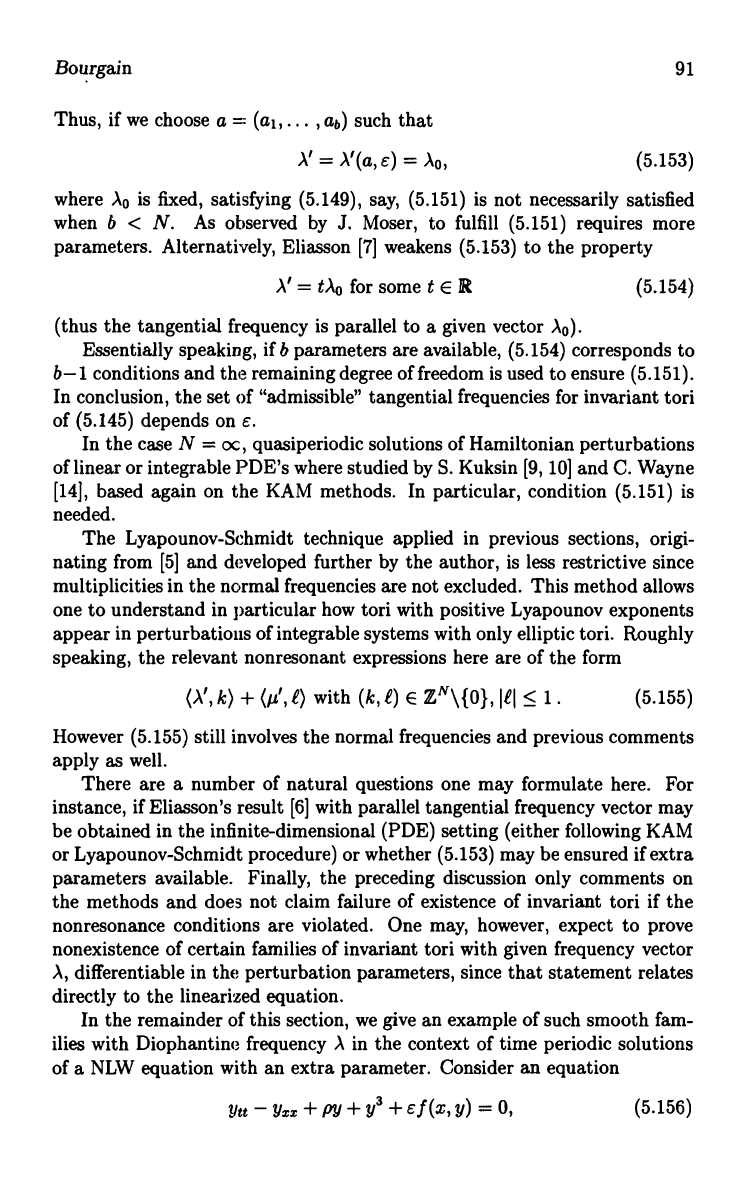
Bourgain
91
Thus, if we choose a =: (al,
... ,
ab) such that
A' = A'(a, e) = Ao,
(5.153)
where A0 is fixed, satisfying (5.149), say, (5.151) is not necessarily satisfied
when b < N. As observed by J. Moser, to fulfill (5.151) requires more
parameters. Alternatively, Eliasson [7] weakens (5.153) to the property
A' = tAo for some t E R
(5.154)
(thus the tangential frequency is parallel to a given vector Ao).
Essentially speaking, if b parameters are available, (5.154) corresponds to
b-1 conditions and the remaining degree of freedom is used to ensure (5.151).
In conclusion, the set of "admissible" tangential frequencies for invariant tori
of (5.145) depends on e.
In the case N = oc, quasiperiodic solutions of Hamiltonian perturbations
of linear or integrable PDE's where studied by S. Kuksin [9, 10] and C. Wayne
[14], based again on the KAM methods. In particular, condition (5.151) is
needed.
The Lyapounov-Schmidt technique applied in previous sections, origi-
nating from [5] and developed further by the author, is less restrictive since
multiplicities in the normal frequencies are not excluded. This method allows
one to understand in particular how tori with positive Lyapounov exponents
appear in perturbations of integrable systems with only elliptic tori. Roughly
speaking, the relevant nonresonant expressions here are of the form
(A', k) + (p', f) with (k, B) E ZN\{O}, jti < 1. (5.155)
However (5.155) still involves the normal frequencies and previous comments
apply as well.
There are a number of natural questions one may formulate here. For
instance, if Eliasson's result [6] with parallel tangential frequency vector may
be obtained in the infinite-dimensional (PDE) setting (either following KAM
or Lyapounov-Schmidt procedure) or whether (5.153) may be ensured if extra
parameters available. Finally, the preceding discussion only comments on
the methods and does not claim failure of existence of invariant tori if the
nonresonance conditions are violated. One may, however, expect to prove
nonexistence of certain families of invariant tori with given frequency vector
A, differentiable in the perturbation parameters, since that statement relates
directly to the linearized equation.
In the remainder of this section, we give an example of such smooth fam-
ilies with Diophantine frequency A in the context of time periodic solutions
of a NLW equation with an extra parameter. Consider an equation
ytt - yaz + py + y3 + e f (X, y) = 0,
(5.156)
92
Chapter 5: Periodic Solutions of Nonlinear Wave Equations
where p will be a parameter. Assume for simplicity that f is a sum of the
form
f (x, y) = bi(x)yj (5.157)
f>1
with (bb} even trigonometric polynomials in x (real-analytic assumptions
should work as well). Thus according to (5.145)
Ho(y, v) =
f [(Y)2
+ ZY' +
4y4 +
2v2] , (5.158)
Hl (y, v) = f
F(x, y)
(8OF = f), (5.159)
with (y, v = y) as canonical variables.
Our parameters a will be extracted from the nonlinearity y3 by amplitude-
frequency modulation, and we let p in (5.156) be a second parameter.
For c > 0, 6 > 0, denote by A,,6 the set of frequencies A satisfying
following Diophantine conditions
IIkAII = min Ik) - nj >
clkl-'-6 for all k
E Z\{0}
(5.160)
and
I -(k2-1)A2+n2-11>Clkl-6 for allkEZ\{1,-1}.
(5.161)
Cl
l
(CA )
0 f
ll d
0
ear
y mes
,,6
or a >
.
We prove the following
Theorem 5.3. Let A E A,,6 (6 small enough). For sufficiently small e, there
are smooth functions of e
p = p(e) , a = a(e)
(5.162)
such that (5.156) has a A-periodic solution in time, of the form
y,(x, t) = a cos x cos At +
E
yE(n, k) cos nx cos kAt
(5.163)
n,k>O,(n,k)$(1,1)
depending smoothly on e and such that
r
e-(InI+IkU`I1/e(n,
k)I = 0(e + 1a13)
(5.164)
(n,k)#(1,1)
(a is small).
Bourgain
93
Note that the representation (5.163) is compatible with the assumption
that (5.157) is even in x. This result is in the spirit of some results from [12]
and [13].
We construct (5.163) following the Lyapounov-Schmidt scheme from [5],
perturbing off from the linear equation
ytt-yza+Py=0
(5.165)
with periodic solution
yo (x, t) = a cos x cos Aot
(5.166)
and
-
Ao = \o(P) =
1
+P .
(5.167)
The Q-equation is obtained by substituting (5.163) in (5.156) and projecting
on the (1, 1) mode. Thus we get
(-A2+1+p)a+y3(1,1)+ef(x,y)(1,1) = 0,
(5.168)
hence of the form
-A2+1+p+ 9 a2+O(a3)+O(e) = 0.
(5.169)
16
The system of P-equations
(-A2k2 + n2 + p)y(n, k) + y3(n, k) + of (x, y)(n, k) = 0,
(5.170)
(n, k) E Z+ x Z+\{1,1 }, is solved by the Newton iteration procedure.
At stage r, an approximate solution y,.
is obtained and the linearized
equation at yT is given by
T=D+S, (5.171)
where D is diagonal with diagonal elements
Dn k = -A2k2 + n2 + p + Q(0, 0) + e8y f (0, 0)
(5.172)
and S with 0-diagonal, given by the Toeplitz operator
S = Sm-m(oo)
(5.173)
and
0 = 3yf + e8y f (x, y,.)
(5.174)
