Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon

Подождите немного. Документ загружается.

304

Chapter 19: Developments in Inverse Problems

19.4

The Inverse Conductivity Problem in

n>3

The identifiability question was resolved in [61] for smooth enough conduc-

tivities. The result is

Theorem 19.6. Let ryi E C2(S2), yi strictly positive, i = 1, 2. If A7, = A72

then 7i = rye in S2.

In dimension n > 3 this result is a consequence of a more general result.

Let q E LO°(Sl). We define the Cauchy data as the set

Cq - { (Ulan, avIon)

is a solution of

(A - q)u = 0 in Q. (19.32)

We have that C. C HZ (8Sl) x 11-1 2(090). If 0 is not a Dirichlet eigenvalue of

A - q, then in fact Cq is a graph, namely

Cq = {(f,Aq(f)) E Hl(8SZ) x H-2(Ocl)},

where Aq(f) = a Ian with u E Hi (1) the solution of

(A - q)u = 0 in 0,

Ulan = f

Aq is the Dirichlet to Neumann map in this case.

Theorem 19.7. Let qi E L°°(SZ), i = 1, 2. Assume Cq, = Cq2, then qi = q2.

We now show that Theorem 19.7 implies Theorem'19.6.

Using (19.21) we have that

7i 2

Cq. _

{(f,

try' '

Ian

av l an) f + y°

lanA

(ryt = l

f))

f e II Z (acl)

}

Then we conclude Cq, = CQ2 since we have the following result due to Kohn

and Vogelius [29].

Theorem 19.8. Let ryi E C'(12) and strictly positive. Assume A7, = A72.

Then

'Milan

80ry21 jal < 1.

0 9,

Uhlmann 305

Remark. In fact, Kohn and Vogelius proved that if yi E C°°(5), ryi strictly

positive, then A7, = A.,., implies that

8ayt

y21

d a.

This settled the identifiability question in the real-analytic category. They

extended the identifiability result to piecewise real-analytic conductivities in

[30]. For related results see [18].

Proof of 19.7. Let ui E H'(Q) be a solution of

(i -gi)ui=0in0, i=1,2.

Then using the divergence theorem we have that

u2 - ul

0uav 2

dS.

(qi - g2)ulu2dx =

fan

Now it is easy to prove that if Cot = C., then the LHS of (19.33) is zero.

Now we extend qi = 0 in SZ`. We take solutions of (z - gi)ui = 0 in R"

of the form

ui = ex"(1 + Q; (x, pi)),

i = 1,2

(19.34)

with (piI large, i = 1, 2, with

Pi =

+ i

f k+1

(19.35)

p2=-i+i (k

and rI, k, I E R" such that

17712 =

Ik(2

+ (112.

(19.36)

Condition (19.36) guarantees that pi

pi = 0, i = 2. Replacing (19.34) into

(qi

- g2)ulu2dx = 0

(19.37)

we conclude

dx.

(qi - q2)(-k)

(qi -

(19.38)

306

Chapter 19: Developments in Inverse Problems

Now II0Q;IIL2lnl < j. Therefore by taking 111 -4 oo we get that

41 42(k) = 0,

V k E 1R",

concluding the proof.

We now discuss Theorem 19.8.

Sketch of proof of Theorem 19.8. We outline an alternative proof to the one

given by Kohn and Vogelius of Theorem 19.8. In the case 'y E Coo (S2) we

know, by another result of Calderon [14], that Ay is a classical pseudodiffe-

rential operator of order 1. Let (x', x") be coordinates near a point xo E Oil

so that the boundary is given by x" = 0. If A.y(x', ') denotes the full symbol

of A.y in these coordinates. It was proved in [63] that

A ,(X', f') = 'y(x', 0)

ao(x', ') + r(x', c'), (19.39)

where ao(x', C') is homogeneous of degree 0 in C' and is determined by the

normal derivative of y at the boundary and tangential derivatives of y at the

boundary. The term r(x', 6') is a classical symbol of order -1. Then -y ion is

determined by the principal symbol of Ay and

ion is determined by the

principal symbol and the term homogeneous of degree 0 in the expansion of

the full symbol of A.. More generally, the higher order normal derivatives of

the conductivity at the boundary can be determined recursively. In [34] one

can find a more general approach to the calculation of the full symbol of the

Dirichlet to Neumann map.

The case y E C' (S2) of Theorem 19.8 follows using an approximation

argument [63]. For other results and approaches to boundary determination

of the conductivity, see [4], [11], [35].

It is not clear at present what is the optimal regularity on the conductivity

for Theorem 19.6 to hold. Chanillo proves in [15] that Theorem 19.6 is valid

under the assumption that Ay E FP, p > 21, where FP is the Fefferman-

Phong class and it is also small in this class. He also presents an argument of

Jerison and Kenig that shows that if one assumes yi E W2'P(S2) with p > a

then Theorem 19.6 holds. An identifiability result was proven by Isakov [26]

for conductivities having jump-type singularities across a submanifold.

R. Brown [10] has shown that Theorem 19.6 is valid if one assumes yi E

C1+`(12) by using the arguments in [61] combined with Theorem 19.5.

The arguments used in the proofs of Theorems 19.6, 19.7, 19.8 can be

pushed further to prove the following stability estimates. For stability esti-

mates for the inverse scattering problem at a fixed energy see [52].

Theorem 19.9 ([3]). Suppose that s > i and that yl and y2 are CO0 con-

ductivities on St C R" satisfying

Uhimann

307

(i)0<<-y,<E,

(ii)

Then there exists C = C(Q, E, n, s) and 0 < o, < 1 (o- = a(n, s)) such that

117' - 721IL-(n) <- C{, log IIA71- An 11

,-=1-° + IIA7, - Aw 1I;,- }

(19.40)

where II

III,Z denotes the operator norm as operators from Hf'(8Q) to

H 2 (8D).

This result is a consequence of the next two results.

Theorem 19.10 ([3]). Assume 0 is not a Dirichlet eigenvalue of A - qt,

i=1,2. Lets > 2, n > 3 and

Then there exists C = C(SZ, M, n, s) and 0 < or < 1 (a = a(n, s)) such that

Ilgt - g211x-1(n) <- C(I log IIAgi - AQ2II;,-, I-° + IIAqi - Ag2II z,-1.

(19.41)

The stability estimate at the boundary is of Holder type.

Theorem 19.11 ([63]). Suppose that yt and rye are COD functions on St C

R' satisfying

(_)0<E-<'y <E,

(ii) IIy'IIc2(n) < E.

Given any 0 < or < nit, there exists C = C(Q, E, n, a) such that

Ilyi -

y2IIL0(8n)

<- CIIA71- A.NII;,-

(19.42)

and

19-h 0572

8v av

L°O(8ft)

(19.43)

The complex geometrical optics solution of Theorems 19.6 and 19.7 were

also used by A. Nachman [35] and R. Novikov [45] to give a reconstruction

procedure of the conductivity from A7.

We first can reconstruct y at the boundary since yl

(l;'I is the principal

symbol of A7 (see (19.39)). In other words, in coordinates (x', x") so that

O 'D is locally given by x" = 0 we have

y(x'' 0) = lim e-""'4">

a->oo

308

Chapter 19: Developments in Inverse Problems

with w' E lRi-1 and Iw'J = 1.

In a similar fashion, using (19.39), one can find e I

by computing the

principal symbol of (A.y-ylanAj) where Al denotes the Dirichlet to Neumann

map associated to the conductivity 1.

Therefore if we know A., we can determine Aq. We will then show how to

reconstruct q from Aq. Once this is done, to find f, we solve the problem

L.u-qu=0inIl,

(19.44)

Ulan = vr--YI,,.

Let ql = q, q2 = 0 ink formula (19.33). Then we have

quv =

- A0) (vI

) uI oS, (19.45)

n n

where u, v E H1(St) solve Du - qu = 0, Av = 0 in Q. Here A0 denotes the

Dirichlet to Neumann map associated to the potential q = 0. We choose

p=, i = 1, 2 as in (19.35) and (19.36).

Take v = ex'PI, u := up = ex'P2(1 + 1/'q(x, p2)) as in Theorem 19.1. By

taking lim in (19.45) we conclude

IlHoo

) tL I dS.

q"1'-k) = lim f (Aq - Ao) (ex-P' 1,

III-+00

n n an

So the problem is then to recover the boundary values of the solutions up

from Aq.

The idea is to find up

by looking at the exterior problem. Namely by

extending q = 0 outside 0, up solves

Aup=0inRn-S2,

v A (

)

(19.46)

Also note that

= q up

e-x'PUP - 1 E La(r). (19.47)

Let P E '- 0 with p- p = 0. Let Gp(x, y) E D'(Rn x Rn) denote the Schwartz

kernel of the operator Ap 1. Then we have that

gp(x) = ex*PGP(x) (19.48)

is a Green's kernel for A, namely

Ogp = ba. (19.49)

Uhlmann

309

We shall write the solution of (19.46) and (19.47) in terms of single- and

double-layer potentials using this Green's kernel (also called Faddeev's Green's

kernel (20].

For applications to inverse scattering at fixed energy of this

Green's kernel see [19], [35], [45], [46], [47].)

We define the single- and double-layer potentials

1S°f (x) =

Jan

g,(x - y).f (y)dSy,

x E R" - 51, (19.50)

D f( °( - )f( )dS lR" f1)=J 19

° x x y y y, xE -

(

)

B° f (x) = p.v. Jn g0

(x - y) f (y)dSy, x E 852. (19.52)

Nachman showed that f,, = u°Ian is a solution of the integral equation

fp=ex'°-

(SPAq-B°-

2I) fp (19.53)

Moreover, (19.53) is an inhomogeneous integral equation of Fredholm type

for f°, and it has a unique solution in H12(090). The uniqueness of the ho-

mogeneous equation follows from the uniqueness of the solutions in Theorem

19.7.

We end this section by considering an extension of Theorem 19.6 to quasi-

linear conductivities.

Let y(x, t) be a function with domain S2 x ][t. Let a be such that 0 < a < 1.

We assume

y E

C1"'(?i x [-T, T])

V T, (19.54)

y(x,t) > O V (x,t) E 52 x R.

(19.55)

If f E C2'°(8 l), there exists a unique solution of the Dirichlet problem (see

e.g., [19])

J div(y(x, u)Du) = 0 in 52,

_ .f

asl

Then the Dirichlet to Neumann map is defined by

(19.56)

A,(f) = ,Y(X,f)l en8-Jast

(19.57)

where u is a solution to (19.56). Sun [53] proved the following result.

310

Chapter 19: Developments in Inverse Problems

Theorem 19.12 ([53]). Let n > 3. Assume y= E C',' (Si x [-T, T]) V T > 0

and A7i = A72. Then yl(x, t) = y2(x, t) on S1 x R.

The main idea is to linearize the Dirichlet to Neumann map at constant

boundary data equal to t (then the solution of (19.56) is equal to t). Isakov

[27] was the first to use a linearization technique to study an inverse parabolic

problems associated to nonlinear equations. The case of the Dirichlet to Neu-

mann map associated to the Schrodinger equation with a nonlinear potential

was considered in [29], [30] under some assumptions on the potential. We

note that, in contrast to the linear case, one cannot reduce the study of

the inverse problem of the conductivity equation (19.56) to the Schrodinger

equation with a nonlinear potential since a reduction similar to (19.21) is

not possible in this case. The main technical lemma in the proof of Theorem

19.12 is

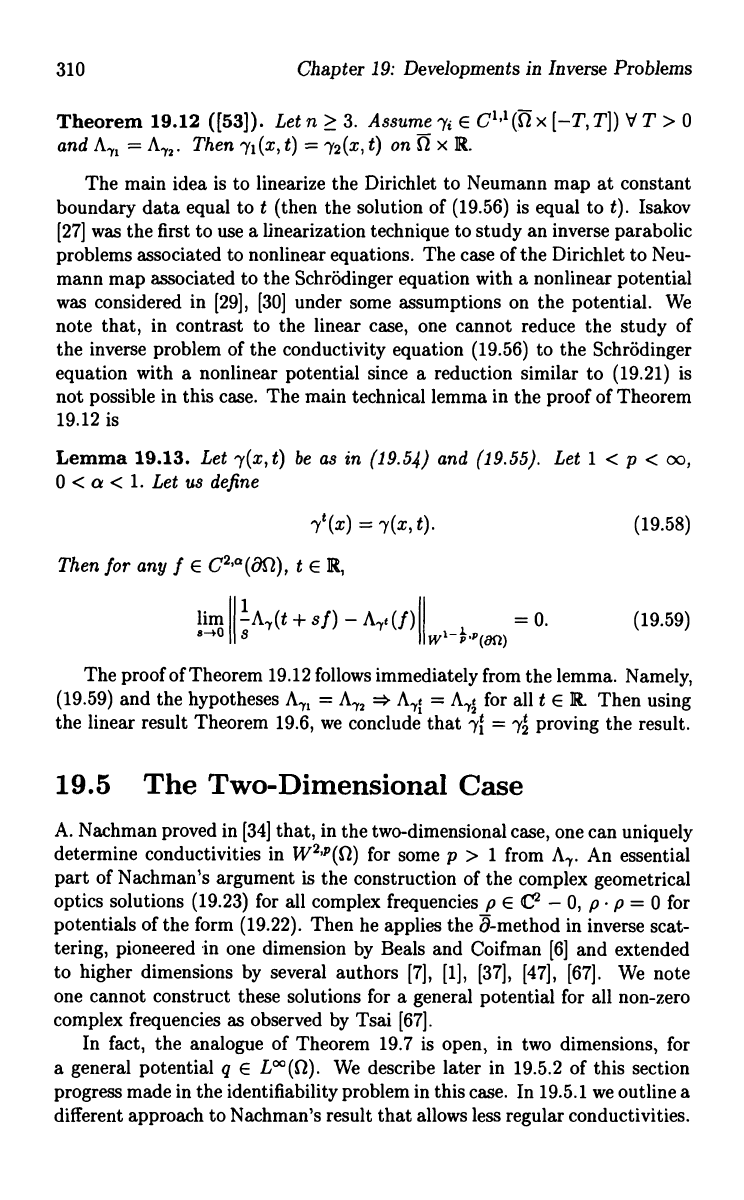
Lemma 19.13. Let y(x, t) be as in (19.54) and (19.55). Let 1 < p < oo,

0 < a < 1. Let us define

'Y`(x) = y(x,t) (19.58)

Then for any f

E C2,a(Ocl),

E Il8,

°II1A7(t+sf)-A7°(f)II

=0.

(19.59)

w P"iacz)

The proof of Theorem 19.12 follows immediately from the lemma. Namely,

(19.59) and the hypotheses A7, = A72

A71 = A72t for all t E lit Then using

the linear result Theorem 19.6, we conclude that yl = y2 proving the result.

19.5 The Two-Dimensional Case

A. Nachman proved in [34] that, in the two-dimensional case, one can uniquely

determine conductivities in W2'p(Il) for some p > 1 from A.Y. An essential

part of Nachman's argument is the construction of the complex geometrical

optics solutions (19.23) for all complex frequencies p E C2 - 0, p p = 0 for

potentials of the form (19.22). Then he applies the 8-method in inverse scat-

tering, pioneered in one dimension by Beals and Coifman [6] and extended

to higher dimensions by several authors [7], [1],

[37], [47], [67]. We note

one cannot construct these solutions for a general potential for all non-zero

complex frequencies as observed by Tsai [67].

In fact, the analogue of Theorem 19.7 is open, in two dimensions, for

a general potential q E L°°(I)). We describe later in 19.5.2 of this section

progress made in the identifiability problem in this case. In 19.5.1 we outline a

different approach to Nachman's result that allows less regular conductivities.

Uhlmann

311

19.5.1 The Inverse Conductivity Problem

In this section we describe an extension of Nachman's result to W'P(S2), p >

2, conductivities proved by Brown and the author [12]. We follow an earlier

approach of Beals and Coifman [8] and L. Sung [59], who studied scattering

for a first-order system whose principal part is

Theorem 19.14. Let n = 2.

Let y E W''p(S2), p > 2, ry strictly positive.

Assume A.,, = A7,. Then

'yi = 'Yz in N.

We. first reduce the conductivity equation to a first-order system. We

define

q =

-2alog'y

(19.60)

and define a matrix potential Q by

0 q

(19.61)

q 0

We let D be the operator

(19.62)

An easy calculation will show that, if u satisfies the conductivity equation

div(yVu) = 0, then

v ,

(au)

solves the system

(19.63)

- Q

= 0.

(19.64)

In [12] are constructed matrix solutions of (19.64) of the form

esZk

= rn(z, k) (19.65)

0 e-`Yk

312

Chapter 19: Developments in Inverse Problems

where z = x1+ix2i k E C, with m -+ 1 as jzl - no in a sense to be described

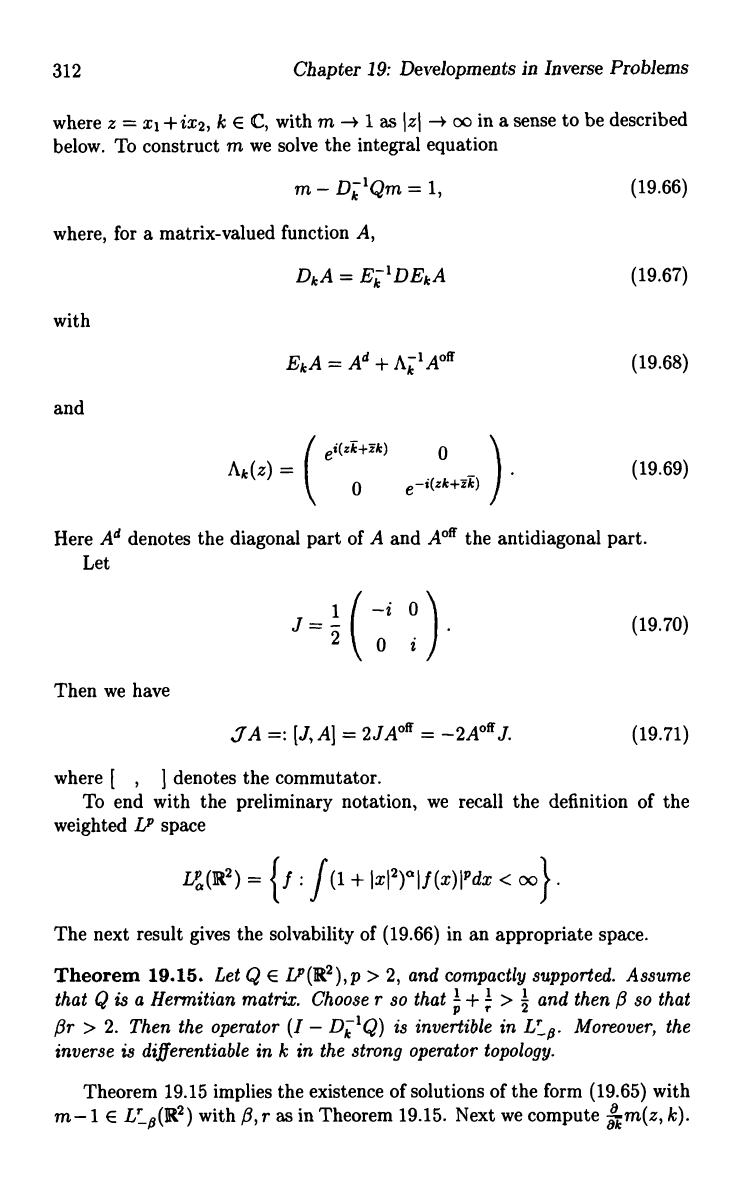
below. To construct m we solve the integral equation

m - Dk'Qm = 1,

(19.66)

where, for a matrix-valued function A,

DkA = Ek'D EkA

(19.67)

with

EkA = Ad + A

k' A°

(19.68)

and

ei(J+Ak)

Ak(z) _

(19.69)

i(k

+A)

Here Ad denotes the diagonal part of A and A°ff the antidiagonal part.

Let

J = 2

-i 0

(19.70)

0 i

Then we have

JA =: [J, Al = 2JA°ff = -2A°ff J. (19.71)

where [

J denotes the commutator.

To end with the preliminary notation, we recall the definition of the

weighted LP space

La(R2) = I f :

f(i + Ix)2)0If(x)j dx < oo } .

The next result gives the solvability of (19.66) in an

appro)))priate space.

Theorem 19.15. Let Q E LP (R), p > 2, and compactly supported. Assume

that Q is a Hermitian matrix. Choose r so that 1 + 1 > 2 and then ,l3 so that

Or > 2. Then the operator (I - D-1Q) is invertible in L',6. Moreover, the

inverse is differentiable in k in the strong operator topology.

Theorem 19.15 implies the existence of solutions of the form (19.65) with

m-1 E

L_,6 (lR2) with /3, r as in Theorem 19.15. Next we compute ekm(z, k).

Uhlmann

313

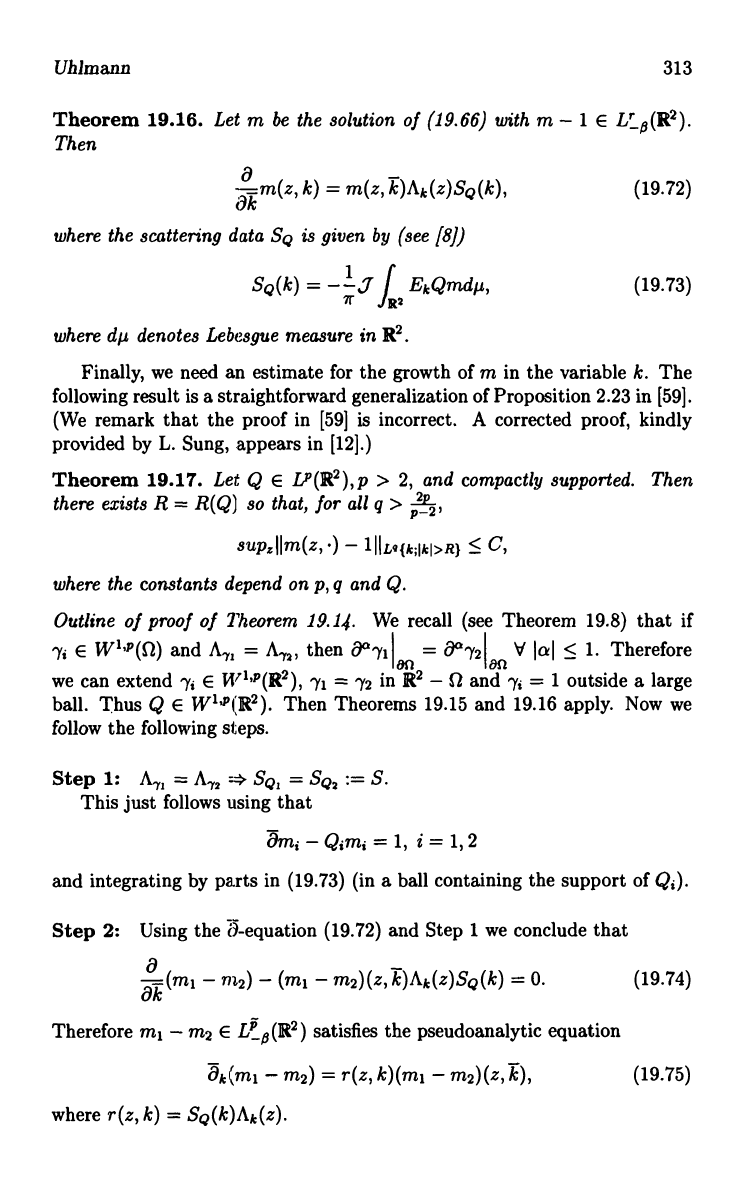
Theorem 19.16. Let m be the solution of (19.66) with m - 1 E Lr,6(R2).

Then

m(z, k) = m(z, k)Ak(z)SQ(k), (19.72)

where the scattering data SQ is given by (see [8])

SQ(k) = ..!

EkQmdp, (19.73)

where d1c denotes Lebesgue measure in R2.

Finally, we need an estimate for the growth of m in the variable k. The

following result is a straightforward generalization of Proposition 2.23 in [59].

(We remark that the proof in [59] is incorrect. A corrected proof, kindly

provided by L. Sung, appears in [12].)

Theorem 19.17. Let Q E Lp(R2),p > 2, and compactly supported. Then

there exists R = R(Q) so that, for all q IP-

p-21

supzII m(z, .)

- 1IIL9{k;IkI>R} < C,

where the constants depend on p, q and Q.

Outline of proof of Theorem 19.14. We recall (see Theorem 19.8) that if

y: E Wl'P(Q) and A7i = A7 then a0

yiI

&-Y2 180 V Ial < 1. Therefore

an =

we can extend y; E W"(R2), yi = y2 in R2 - 1 and yi = 1 outside a large

ball. Thus Q E Wl4'(R2). Then Theorems 19.15 and 19.16 apply. Now we

follow the following steps.

Step 1: A71 = A72 SQ, = SQ2 := S.

This just follows using that

ami - Q;mi = 1, i = 1, 2

and integrating by parts in (19.73) (in a ball containing the support of Q;).

Step 2:

Using the iequation (19.72) and Step 1 we conclude that

ak (ml - m2) - (ml - m2)(z, k)Ak(z)SQ(k) = 0.

(19.74)

Therefore ml - m2 E L? #(R2) satisfies the pseudoanalytic equation

ak(MI - m2) = r(z, k)(ml - m2)(z, k),

(19.75)

where r(z, k) = SQ(k)Ak(z).