Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon
Подождите немного. Документ загружается.


244
Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations
is defined by
00
E2'°`IJoj(f)II1 < oo
(1/q+1/p= 1).
-00
. -0,oo
If S(t) = exp(t0) is the heat semigroup, then Bq
is characterized by
sup t
" / 2 I S(t) f I I
q= C < oo.
(15.4)
t>o
Definition 15.2. We will denote by Bq the homogeneous Besov space Bq
Q'00
when a=l - q, 3 <q<oo.
It is easily checked that
L3(IIt3) C
L3,-(W)
C Bq C Bq C Boo's,
(15.5)
where 3:5 q:5 q' < +oo.
The Banach space B',-,,,"00 consists of f = Og, where g belongs to the
Zygmund class defined by
Ig(x + y) + g(x - y) - 2g(x)I <- Clyl (15.6)
forxEIV,yER".
In the next section, we will show that IIuoI Is, < i7(q) for some q E (3,9)
suffices for the existence of a global solution.
Here g(q) is a continuous
function of q, which tends to 0 as q tends to 9. It means that we are far from
obtaining an optimal sufficient condition for global solutions. For the sake
of simplicity we will concentrate on the special case q = 6.
15.3 A Sufficient Condition for Global
Solutions
The following theorem is implicit in some joint work between M. Cannone,
F. Planchon, and the author of these notes:
Theorem 15.3. There exists a positive constant 77 > 0 such that for any
uo E CO '(R) and
Iluo11s8 < 77,
(15.7)
there exists a global solution u(x, t) to (15.1) such that u(x, 0) = uo(x).

Meyer
245
Let us stress that Theorem 15.3 cannot be valid without (15.7). Such a
claim would contradict Theorem 15.1. A second observation is given by the
following Gagliardo-Nirenberg-type inequality.
Lemma 15.4. There exists a constant C such that for any f in Sobolev
space H' (R3 )
fjfj! S CIIVf!I2lIfIIam
(15.8)
A fortiori (15.7) implies IIuo114 < C,iIIVuo112, which is a strong form of
the negation of (15.2).
We should also observe that uo(x) and .uo(Ax), A > 0, have the same
B6 norm. Therefore Theorem 15.3 is in full agreement with the rescaling of
(15.1).
Theorem 15.3 explains some observations we made after stating Theorem
15.1. If cp E Co (R3) and e > 0 tends to 0, then the norm in B6 of cc(ex)
is c
and tends to infinity. Therefore (15.7) is violated, and we know that
the solution to (15.1) blows up whenever a is small enough. In contrast, if g
is fixed in the Schwartz class S(R3) we consider uo(x) = ew-g(x). Then
1IuoUUBe =
+0(1w!"2)
as
awl -3 +oo
(15.9)
and (15.7) is satisfied for jwj large enough.
15.4
Self-Similar Solutions to the Nonlinear
Heat Equation
The following theorem was found by A. Haraux and F. Weissler.
Theorem 15.5. There exists a (nontrivial) function w(x) in the Schwartz
class S(R3) such that
/
u(x,t)=wl),
t>0, xE&3.
(15.10)
is a global solution to (15.1).
This self-similar solution is invariant under the rescaling Au(Ax, Alt) _
u(x, t).
The corresponding initial value uo(x) = 0 identically.
It means that
in Theorem 15.3, u E C([0, oo), L3(R3)) cannot be replaced by the weaker
condition
t) 113 < oo
(15.11)
t>o
246 Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations
without losing uniqueness. But the stronger condition does not imply unique-
ness either [19].
In the seminal paper [9] by A. Haraux and F. Weissler, w(x) was a radial
function. A more systematic treatment can be found in a remarkable paper
by M. Escobedo and 0. Kavian [7].
15.5
Multilinear Operators Arising in the Proof
of Theorem 15.3
The following treatment of (15.1) is due to T. Kato, who converted (15.1) into
an integral equation. Instead of writing at = Au + u3 with u(x, 0) = uo(x),
he wrote
u(t) = S(t)uo +
J
S(t - s)u3(s) ds (15.12)
e
0
where S(t) = et° is the heat semigroup and u(x, t) is viewed as a vector-
valued function of the time variable t. Kato's program consisted in looking
for solutions u(t) belonging to C([0, oo); E), where E is a suitable Banach
space of functions of x. The norm of u(t) in C([0, oo); E) is supt>o Ilu(-, t)II E.
The collection of Banach spaces which are being used splits into two classes.
If E belongs to the first class, E will be a separable Banach space and the
condition u E C([0, oo); E) means that u, as a function of t, is continuous
when E is equipped with the topology defined by the norm. When E belongs
to the second class, E will always be the dual F' of a separable Banach space
F, and the continuity requirement will concern the a(E, F) topology.
Examples of this situation are given by E = L3'°° (the weak L3-space),
which is the dual of F = L3/2,1 (the corresponding Lorentz space), or by
E = Bq a' (the homogeneous Besov space), which is the dual of F = Bq
I
(when 1/p + 1/q = 1).
T. Kato's program consists in solving (15.12) through Picard's fixed-point
theorem. The norm of u in the Banach space X = C([0, oo); E) is defined as
I IUIIX = sup Ilu(', t)IIE
(15.13)
t>o
Then the main issue is to prove the estimate
Ilr(u1,U2,U3)Ilx 5 CIIUIIIXIIu2IIxIIu3IIx
for the trilinear operator IF defined by
(15.14)
tr(u1, u2, u3) =
jS(i - s)ul (s)u2(s)u3(s) ds.
(15.15)
Meyer
247
We wrote u(s) =
s) and so on for keeping the notation as simple as
possible.
A solution u E X of (15.12) is called a "mild solution" of (15.1). When-
ever (15.14) holds, Picard's fixed-point scheme can be applied and yields a
mild solution as long as the norm of uo in E is small enough. More precisely
C-1/2 where C is defined by (15.14).
we should have IIUOIIE < 3
73
T. Kato and F. Weissler applied this program to E = L3(R3), but the
fundamental trilinear estimate is definitely incorrect. T. Kato was able to
offer a substitute for the missing estimate. Indeed, he considered a subspace
T C X consisting of function u(x, t) which are continuous on [0, oo) with
values in L3(He) and such that t) is continuous on [0, oo) with values
in L6(R3 ). Moreover, one imposes that limt-+o t1/4I
t) 116
= 0 as well as
lime-.+. t1/4I1u(., t)116 = 0.
Finally one writes
IuII- =
t > 0}
(15.16)
and
Ilul IT = 11U1 1X + (lull..
Then the following estimates are easily verified
Ir(u1, u2, u3) I Ir <-
CIIu111.1Iu211.11u311,
and
(15.17)
(15.18)
IIS(t)uollT <-11u0113
(15.19)
Therefore Picard's scheme can be used to solve (15.12) inside the Banach
space T whenever Iluo113 is small enough. The drawback of this approach is
the lack of uniqueness inside the "natural space" X. Indeed we get uniqueness
inside the smaller space T.
In [19], E. Terraneo proved the lack of uniqueness inside the "natural
space" X.
Our Theorem 15.3 is a slight improvement on Kato's ideas. We con-
sider the Banach space Y of functions u(x, t) which are continuous on (0, oo)
with values in L6(R3) such that IIull. is finite and limt.+o
t)116 =
0. If no belongs to L3(R3), then S(t)uo belongs to
Y and we have IIS(t)uoll
=11u011B6 < r!
Then Picard's scheme can be applied inside Y and yields a mild solution
to (15.12). Then it is easy to check that
t
1 0
S(t - s)u3(s) ds 116 -+ 0 as t -+ 0, (15.20)
I
0
248
Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations
which will imply that the solution which is constructed belongs to X. If uo
belongs to CO -(R), ), we want to prove that the "mild solution" arising from
Kato's program is a classical solution to (15.1). The reader is referred to [7],
[9] where the issue is discussed.
Let us remark that Theorem 15.3 is not optimal and better sufficient
conditions can be found in [11]. However, Theorem 15.3 paves the way to
the general methodology that will be used later in these notes.
15.6
More Self-Similar Solutions to the
Nonlinear Heat Equation
We already observed that if u(x, t) is a solution to (15.1), so are ua(x, t) _
Au(Ax, alt) for A > 0. A solution u(x, t) is self-similar if u,, = u. In other
terms
(Vxlt-) -
(15.21)
In contrast with the approach indicated by A. Haraux and F. Weissler, we
want to construct our self-similar solutions by solving an initial-value prob-
lem. This approach will exclude the Haraux-Weissler's self-similar solution
for which the initial value is 0. For this trivial initial value, our approach will
uniquely yield the trivial solution to (15.12). If in the distributional sense,
limtlo 7V (7) = uo(x) exists, then this distribution uo(x) will be homoge-
neous of degree '1. This remark implies that the functional space E which
will be used in our methodology should contain homogeneous functions of
degree -1. This excludes the D' spaces but includes the Besov over spaces
. a,00
Bq
, when a = 1 - 3/q or the Lorentz space setting.
Theorem 15.6. There exist two positive constants Q > 0 and ry > 0 with
the following properties: if uo E L3'0O(R3) satisfies
6 and is
homogeneous of degree -1, there exists a unique solution u(x, t) of (15.12)
such that
u(x, t) = S(t)[uo](x} + W ( ) , (15.22)
VIFVXt_
where
W(x) E L3(R3) n L6(R3) and 11WI16 < ,Q.
(15.23)
Indeed, (15.22) implies that u(., t) is a continuous function of t with values
in L3'O° equipped with its a(L3,°°, L3/2,1) topology.
Meyer
249
Since uo(x) is homogeneous of degree -1,
5.24)
S(t)[uo](x)
=
7Vo (7xt)
(1
and u(x, t) is a self-similar solution to
(15.12).
If uo = 0, Theorem 15.5 yields u(x, t) = 0.
It means that the Haraux-
Weissler self-similar solution 1w
satisfies JJwJJ6 > $. This was observed
by M. Escobedo and 0. Kavian: all the self-similar solutions to the nonlinear
heat equation they constructed have "large" norms.
To illustrate Theorem 15.5, let us consider the trivial example where
uo(x) = 771x)-1, 77 > 0 being small. This example was treated in the Haraux-
Weissler seminal paper, and they found that the corresponding solution to
(15.12) was given by
u(x, t) =
f
u
(IxJ\
I
(15.25)
where 0 < u(r) < Co on [0, oo) and lim,.,+oo ru(r) = L > 0.
It means that u(x, t) ti Ixl at infinity (t being frozen). Therefore u(x, t)
belongs to L3,o° as expected from Theorem 15.5 and u(x,t) E L6(R3)
The Lorentz space L3°°°(R3) was one example of functional space which
is adapted to finding self-similar solutions to the nonlinear heat equation. In
. -a,eo
the next section the Besov space B.
, a = 1-
3/q, 3 < q < 9, will be used
for the same goal.
Theorem 15.5 was obtained in a joint work with 0. Barraza [2].
15.7 Another Approach to Self-Similar
Solutions
Instead of using the Lorentz space L3'°°(R3), we will be using the Besov space
. -a,oo
Bq
= Bq where 3 < q < 9. We first construct general solutions inside a
functional setting which is adapted to the existence of nontrivial self-similar
solutions. This approach will be used for Navier-Stokes equations.
Theorem 15.7. Let us assume that 3 < q < 9. Then there exist two positive
constants rl(q) and 13(q) with the following properties: if JJUOJJB, < rl(q),
then there exists a solution u(x, t) to (15.12) which satisfies the following
properties
u(x, 0 = S(t) [uo] (x) + w(x, t),
(15.26)

250
Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations
sup 00,
(15.27)
t>o
sup t°12l lw(-, t) l lq < /3(q)
t>o
(15.28)
Moreover such a solution to (15.12) is uniquely defined by (15.26), (15.27),
and (15.28).
In order to find self-similar solutions, it suffices to assume that uo(x) is
homogeneous of degree -1. Then the scaling invariance of the nonlinear heat
equation implies that whenever uo(x) is replaced by Auo(Ax), then u(x, t) is
replaced by Au(.x, A2t).
Finally, the uniqueness of the solution implies Au(Ax, alt) = u(x, t), and
our u(x, t) is a self-similar solution. This approach is due to M. Cannone.
Theorem 15.6 both generalized Theorem 15.3 and Theorem 15.5. Let us
explain why. Indeed, Theorem 15.3 is a special case of Theorem 15.6 (q = 6)
and for obtaining Theorem 15.5 it suffices to observe that the Lorentz space
L3,°°(R3) is contained in each Besov space Bq. Indeed L3'°°(R3) is contained
. 0,00
inside the smallest one, i.e., B3
.
The reason why Theorem 15.5 was stated before is the following. Any
analyst will tell you that (in three dimensions) Ixl-1 belongs to L3'°°(R3)
(i.e., any weak L3), and only a few would consider this function as belonging
to our fancy Besov spaces.
15.8
Convergence to a Self-Similar Solution
In this section, the exponent q will always belong to the open interval (3, 9).
If u(x,t) is a global solution to (15.12), so is
U,\ (X, t) =
au(,\x, Alt) for A
> 0.
In order to study the large-scale behavior of u(x, t), it becomes natural to
raise the following problem. Does there exist a limit function v(x, t) such
that
lim llua(., t) - v(., t)11L9pe3) = 0
At+oo
uniformly in t E [to, t1] whenever t1 > to > 0.
(15.29)
We then have v(x, t) = 7 V (
) and
Jim11fu(fx,t)-V(x)11q=0.
(15.30)

Meyer
251
Another way of raising the same problem is to ask for an asymptotic
expansion
x t)
(15.31)u(x,t) = 1 V (71x ) + 1 R 1
71
where V E LQ(iR3) and
li.m t)JJq = 0
(t -+ +oo). (15.32)
t 4+o0
The following theorem was proved in a joint work with F. Planchon ([17]).
We want to know whether (15.31) holds for a global solution to the nonlinear
heat equation. An obvious necessary condition is J J f u(f x, t) J Jq < C for
t > T. We will modify our problem by imposing the stronger condition
JJfu(fx, t)IIq < C,
0 < t < 00,
(15.33)
which is no longer necessary to the problem we have in mind. Then our
problem can be efficiently solved whenever 3 < q < 9.
Theorem 15.8. Let u,(x, t), x E R3, t > 0, be a global solution to (15.33)
and
u(x, t) -+ uo(x) as t tends to 0.
(15.34)
The limit in (15.34) is taken in the distributional sense.
Then uo(x) E
Bq
, a = 1 -
3/q, 3 < q < 9.
Moreover, if (15.31) and (15.32) hold,
then V (
) is
a self-similar solution to the nonlinear heat equation. Con-
7t 7-t
versely there exists a positive constant ,B (q) such that whenever J Juo J J
<
/3(q), the following properties (a) and (b) are equivalent ones:
a. (15.31) and (15.32) hold.
b.
a4
Auo(Ax) -4 vo(x) as A -1 +oo and
(15.35)
to/2JJS(t)[uo - vo]JJq
(t -+ +oo)
(15.36)
It is easily checked that the second requirement (15.36) implies the first
one. Both of them concern the large-scale behavior of the initial value uo(x)
and tell that the infrared limits of uo (x) and vo (x) are the same.
For stressing the difference between (15.35) and (15.36) let us consider a
wavelets expansion of f (x) = vo(x) - uo(x). One uses the wavelets which are
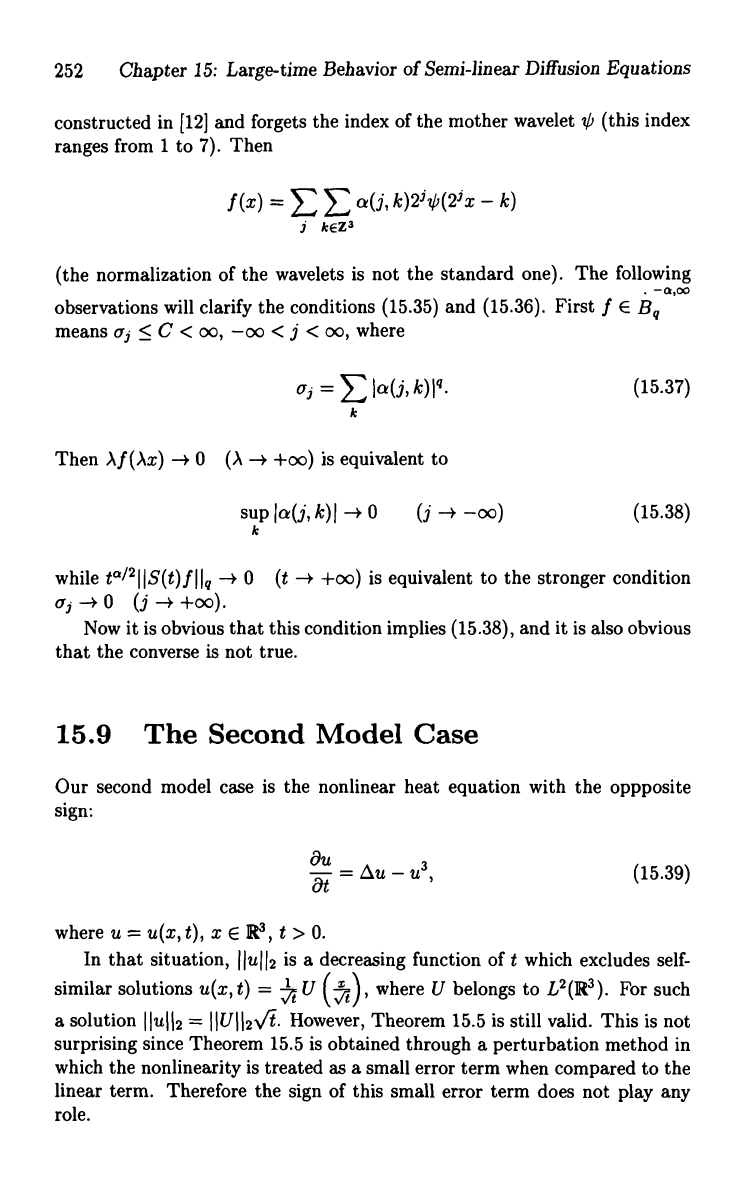
252
Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations
constructed in [12] and forgets the index of the mother wavelet ib (this index
ranges from 1 to 7). Then
f (x) _ 1: 1: a(j, k)2jV,(2jx - k)
j
kEZ3
(the normalization of the wavelets is not the standard one). The following
observations will clarify the conditions (15.35) and (15.36). First f E BQ
means aj < C < oo, -oo < j < oo, where
aj=EIa(j,k)I9.
k
Then Af (Ax) -a 0
(A -* +oo) is equivalent to
(15.37)
sup Ia(j, k) I -+ 0 (j --+ -oo)
(15.38)
k
while ta/21 I S(t) f I as - 0 (t --+ +oo) is equivalent to the stronger condition
aj -> 0 (j -* +oo).
Now it is obvious that this condition implies (15.38), and it is also obvious
that the converse is not true.
15.9
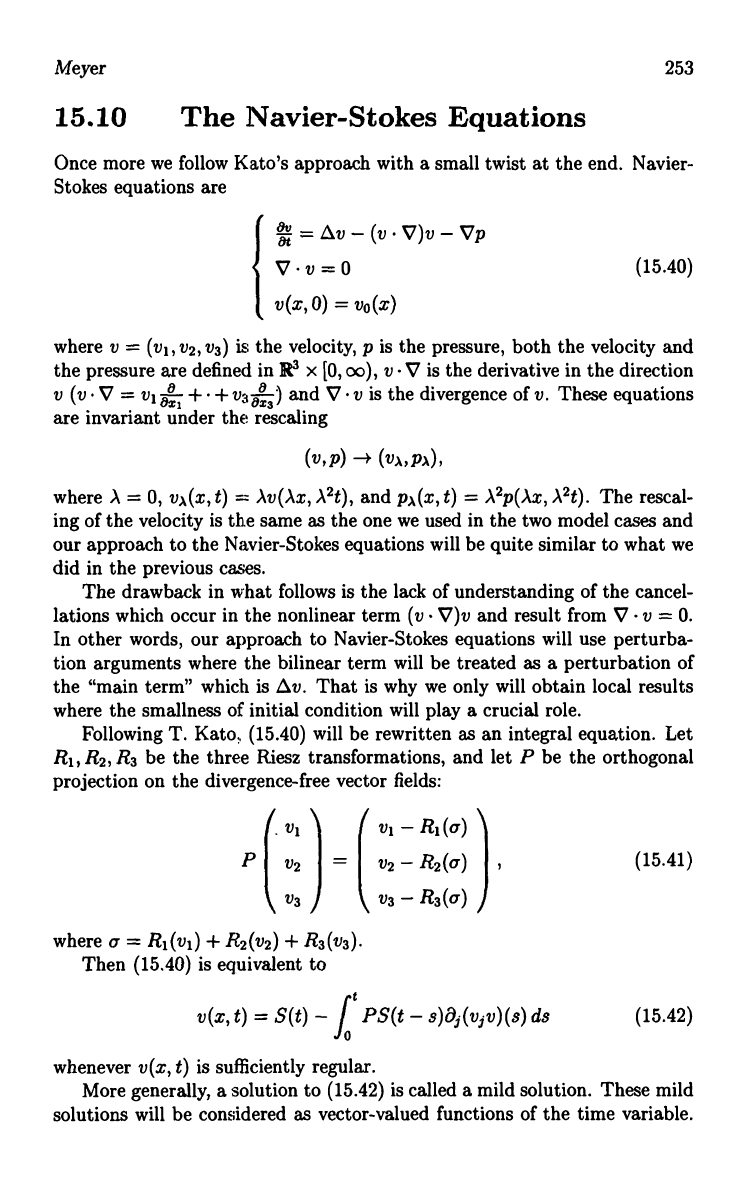
The Second Model Case
Our second model case is the nonlinear heat equation with the oppposite
sign:
8u
N
= Au - u3
(15.39)
where u=u(x,t),xElR,t>0.
In that situation, IIulI2 is a decreasing function of t which excludes self-
similar solutions u(x, t) _' U
where U belongs to L2(R3). For such
a solution IIu1I2 = IIUII2f However, Theorem 15.5 is still valid. This is not
surprising since Theorem 15.5 is obtained through a perturbation method in
which the nonlinearity is treated as a small error term when compared to the
linear term. Therefore the sign of this small error term does not play any
role.
Meyer
253
15.10
The Navier-Stokes Equations
Once more we follow Kato's approach with a small twist at the end. Navier-
Stokes equations are
V-v=0
(15.40)
v(x,0) = vo(x)
where v = (V1, v2, v3) is the velocity, p is the pressure, both the velocity and
the pressure are defined in R3 x [0, oo), v - V is the derivative in the direction
v (v . V = vl
1
+ - + v3 eXg) and
v is the divergence of v. These equations
09
are invariant under the resealing
(v,p) -+ (va,pa),
where A = 0, va(x, t) = \v(Ax, A2t), and pa(x, t) = A2p(Ax, A2t). The reseal-
ing of the velocity is the same as the one we used in the two model cases and
our approach to the Navier-Stokes equations will be quite similar to what we
did in the previous cases.
The drawback in what follows is the lack of understanding of the cancel-
lations which occur in the nonlinear term (v 0)v and result from V - v = 0.
In other words, our approach to Navier-Stokes equations will use perturba-
tion arguments where the bilinear term will be treated as a perturbation of
the "main term" which is Av. That is why we only will obtain local results
where the smallness of initial condition will play a crucial role.
Following T. Kato, (15.40) will be rewritten as an integral equation. Let
R1, R2, R3 be the three Riesz transformations, and let P be the orthogonal
projection on the divergence-free vector fields:
v1
vi - R, (a)
P
v2 = v2-R2(o)
,
(15.41)
v3 v3 - R3 (a)
where o = R1(vl) + R2(v2) + R3(v3).
Then (15.40) is equivalent to
tv(x, t) = S(t) -
J
PS(t - s)aj(v?v)(s) ds
(15.42)
0
whenever v(x, t) is sufficiently regular.
More generally, a solution to (15.42) is called a mild solution. These mild
solutions will be considered as vector-valued functions of the time variable.
