Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon
Подождите немного. Документ загружается.


224
Chapter 14: Nodal Sets of Sums of Eigenfunctions
are directly inspired by the Calderon program for singular integral operators
with minimal smoothness.
There are other new results that we will not
discuss.
In particular, we alert the reader to important works of Thomas
Wolff surveyed in [28, 29].
The approach here simplifies slightly the original proof of Donnelly and
Fefferman by eliminating the need for a technically demanding version of
Carleman inequalities. But we wish to emphasize that this paper does not
prove any new theorems. Rather it recovers results of Fang-Hua Lin [22].
Lin was led to similar questions through an attempt to extend results of
Donnelly and Fefferman to solutions of the heat equation. The details of the
present method are different only because Lin does not rely on Carleman
inequalities. There are also more recent and more general estimates due to
Igor Kukavica [18, 19, 20].
14.2
Statements of Results
Let M be a compact C°° manifold. Consider an eigenfunction cp satisfying
AV = -W2(Q.
If the boundary of M is empty, then there are no boundary conditions, but if
the boundary is nonempty, then we impose either Dirichlet boundary condi-
tions (cp = 0 on 8M) or Neumann boundary conditions (8V/8v = 0 on 8M).
The results of Donnelly and Fefferman can be stated as follows.
Theorem 14.1 (Doubling). There are constants C1 and C2 depending only
on M such that
i141+C2
IT'I,
max W - e
B(r)
where B(r) and B(2r) represent concentric balls of M. In particular,
vanishes at most at a rate rclW+c2
W
Theorem 14.2. If M is real-analytic, then there are constants C1 and C2
depending only on M such that the (n - 1) -dimensional Hausdorff measure
11n_1 of the zero set of cp satisfies
Wn_1({x E M : cc(x) = 0}) < C1w + C2.
These theorems describe the sense in which cp resembles a polynomial of
degree at most C1w + C2. As already observed in [6], one cannot hope for a
lower bound for the Hausdorff measure of the nodal set because in the case
of sums of eigenfunctions the nodal sets can be the empty set.
Let Wk be an eigenfunction with eigenvalue wk, that is, Ocpk = -Wkcp, with
Dirichlet or Neumann boundary conditions in the case that the boundary is
nonempty. We will prove

Jerison and Lebeau 225
Theorem 14.3. Theorems 14.1 and 14.2 are valid for any sum of the form
E aksak
Wk <W
How should one characterize a sum of eigenfunctions? Donnelly [6] uses
the fact that cp satisfies
But then he is forced to deal with a higher-order operator. He obtains the
same type of estimates as above for sums of eigenfunctions, but his constants
depend not only on w, but also on the number of terms in the sum. More
recently Kukavica [18, 19, 20] has proved estimates for Ak that are uniform
in k and he has deduced Theorem 14.3 .
We will follow an approach similar to Lin [22]. Namely, consider a func-
tion F defined on R x M by
(i
+ 0)F in. R x M; F(0, x) = 0; OtF(0, x) = o(x).
The solution to this problem is
F(t,x) =
raksinh(wkt)Vk(x)
[r
wk
Denote XT = [-T, T] x MLl.- Then the quantitative property that characterizes
the "degree" of our sum. of eigenfunctions is (for, say, T = 1, 2)
II FII xl(xT)
e'11W1I La(M).
(14.1)
(Here H1(XT) denotes the Sobolev space of functions with first derivatives
in L2(XT). This discussion is not very sensitive to the particular choice of
function spaces.)
The case of the constant function (with zero eigenvalue) has to be dis-
cussed separately:
sinh(wt)
lim = t.
W- +O
W
Therefore, in the case wk = 0, the expression wk 1 sinh(wkt) is replaced by the
function t. The constant function is always an eigenfunction in the Neumann
problem and in the case of empty boundary. This gives the extra dividend
that Theorem 14.3 applies not only to the zero set, but also any level set
{cp = a}. In fact, we can even add the constant function in the Dirichlet
case because the comparison inequality (14.1) is still valid. (This remark will
be proved in an appendix,) Thus in all cases the theorem applies to level
sets as well as the zero set.
226
Chapter 14: Nodal Sets of Sums of Eigenfunctions
14.3
Carleman Inequalities
Let L = 88 + A. The first Carleman-type inequality that we need is
J l p 1'Lgj2 > c J
[Ip-,IVgl2
+ lp-Ogl2] for all g E Co (B\{0}).
(14.2)
Here B is the ball of fixed small radius around the origin 0 and p is a
carefully chosen function comparable to the distance to the origin. The main
point is that the inequality is valid uniformly as 0 -+ oo. This inequality was
proved by Aronszajn (3] in 1957 in the C°° case. When the coefficients of
the operator L are of class C°,1, it was proved by Aronszajn, Krzywicki, and
Szarski in 1963 [4]. The case of nonsmooth coefficients is used in the proof in
the case in which the boundary is nonempty. (See [8] and the remarks later
on.)
The proofs in [4] are not easy to read. The best reference for a proof
of (14.2) is Proposition 2.10 of [7] (smooth case) and [8] (CO,' case). The
statement omits the gradient term on the right-hand side. But inequality
(2.9) of [8] and the subsequent inequality K > I leading to the proof of
Proposition 2.10 in that paper show that the gradient term can be included.
As mentioned before, there is the slight simplification that we need only the
cased=0.
It is not hard to deduce from (14.2) that the following inequality holds.
Lemma 14.4. For any nonempty open set V, V C X1, there exists a > 0
and C such that
IIFllH'(xt) <_
Rather than carry out the proof of Lemma 14.4, we refer to the analogous
proof of Lemma 14.5 below. An immediate consequence of Lemma 14.4 is
the unique continuation property for the operator L, namely, if F = 0 on V,
then F is identically zero on all of X1.
For the remainder of the paper, we use the shorthand M for the subset
{0} x M in XT. The next lemma makes the connection between F and its
values on M.
Lemma 14.5. Let B be a ball in X2 centered on M and disjoint from M.
Let B+ = { (t, x) E B : t > 01. Suppose that V C B+ and that F(0, x) = 0.
There exists a > 0 and C depending only on V, B and M such that
IIFJJH.(v) s
CliatFllL2(BnM)IIFIIHI(B+)
This lemma implies another unique continuation result; namely, if F and
8tF are zero on B f1 M, then F is identically zero in B. It can be deduced
Jerison and Lebeau
227
from a boundary Carleman-type inequality
f JB+ I (at + o)gI2e21'` dt dx + Q .fBnm(atg)2e2B10 dx
> c f JB+(I'IOt,agl2 + 133g2)e2B+G dt dx
for all g E Co (B) such that g = 0 on M. The hypothesis on 0 that is needed
will be. discussed later. This Carleman inequality was proved by Lebeau and
Robbiano [21] and used there for an application to the theory of optimal
control for solutions of the heat equation.' Closely related inequalities are
proved in [16]. We are indebted to Louis Nirenberg for pointing out what
may be the earliest instance of inequalities of the type in Lemma 14.5 (in
different function spaces and for equations with real analytic coefficients) in
the work of Flitz John [14,15]. John's main theme is that the extra hypothesis
of boundedness of the solution over the larger set X2 restores something
resembling well-posedness of what otherwise is an ill-posed Cauchy problem
in the sense of Hadamard.
14.4 Main Idea of the Carleman Method
The idea of the Carleman method is to prove inequalities with weights e00
with 0 largest on the set from which the uniqueness propagates. Thus we
want 0 to take its largest values on B n M.2 On the other hand, there is a
kind of convexity constraint on 0, to be specified later, that will be needed
in order for inequality (14.3) to be true.
Suppose, without loss of generality, that (0, 0) is the center of B. We let
O(t, x) = -t
+ t2/2 - Ix12/4.
(14.3)
This function was chosen so that (14.3) is valid, as we shall see below. Pick
three numbers 0 > '01 > 02 > 03. Define Si = {7, > z/,,} and S, = Si n it >
0}. Thus S, C S2 C S3. Let X E Co (S3) satisfy X = 1 on SS and define
g = XF. Assume for simplicity that 0 is the ordinary Laplace operator (for
'They show that given any nonempty open set U in (t, x)-space with 0 < t < T and
any initial condition u(0, x) = f (x) one can choose an inhomogeneous term Q supported
in U so that the solution to (8t + A)u = Q satisfies u(T, x) = 0. In other words, any
disturbance (initial condition) can be restored to zero using a "control" (inhomogeneous
term) supported in an arbitrarily small region of space-time.
2Similarly, in (14.2) the key feature for applications of the function p-6 is that it is
largest (+oo in fact) at the central point. Thus the Aronszajn inequality actually proves
what is called strong unique continuation; namely, a function satisfying an elliptic equation
that vanishes to infinite order at a point must vanish identically.

228
Chapter 14: Nodal Sets of Sums of Eigenfunctions
a general Laplace-Beltrami one has to use normal coordinates and make a
somewhat more detailed computation):
(J + A)g = 2Vt,xx Vt,.F + ((dt + 0)X)F = 0 on S2+.
Substituting into (14.3), we have
e2o+Gs
I IFII ;(Sg
I IatFIIL2(S3nM) >
Ce2ov1,
IIFIIH.(Si
)-
The term on the left is increased if one replaces H' (S3 \S2) with H' (S3 ).
(It may seem as if this gives away too much. But we are retaining the very
small factor e2o+G2.) Now by ordinary arithmetic, selecting the best choice
of a, one can find a > 0 depending on ipi > Y'2 for which the conclusion of
Lemma 14.5 holds.
Now we come to the proof of (14.3). Define
Lo f = eo'n' (fit + 0) (e-o* f).
This conjugated operator is the same as the one treated by Gunther Uhlmann
in chapter 19 and by coincidence we have used the same notation L,6 .3 We
change variables by replacing g by the function f = e-o0g. Then
f f [(O + 0)g]2e2o" = J
J(Lf).
Decompose Lp into its self-adjoint and skew-adjoining parts.
Lg=P+Q, P'=P, Q'=-Q.
Then
and
IILofIi2 = ((P+Q)f,(P+Q)f)
=
I IPf 112 + I IQf I12 + (P1, Qf) + (Qf, Pf )
(P f, Q f) = (f, PQ f) + boundary terms
= (f , [P, Q] f) + (f , Q P f) + boundaryterms
= (f, [P, Q] f) - (Q f, Pf) + boundaryterms.
3Paul Malliavin informed us in his lecture that in 1954 Alberto Calderon had already
essentially proved his result on the inverse problem discussed by Gunther Uhlmann, al-
though his paper on that subject appeared much later. Thus very early in his career
CalderGn was preoccupied with the analysis of operators conjugated by real as well as
imaginary exponentials, that is, Fourier analysis in the complex domain. The fact that he
was considering Carleman inequalities and this inverse problem at about the same time
makes the connections between the two problems seem far from coincidental.

Jerison and Lebeau 229
In all,
I I L9f I I2 = I I Pf I I2 + I IQf 112 + (f , [P, Q] f) + boundary terms.
Therefore, in order to find a lower bound for
II Laf 112 we will want some
condition on 0 that guarantees that
[P, Q] > > 0 modulo P and Q.
This is a convexity condition on V). The condition can be phrased in terms
of the symbol of the operators P and Q. It is important to require uniform
control with respect to the parameter 0, which is similar to an extra dual
variable.
Let us carry out the computations explicitly. Recall that z/' was given in
(14.3) and A is the ordinary Laplace operator. Then
P= O
-,t2
+L)O
Q
200t,xVJ V t,. = f (Ot + 0)O.
Note that z/i was chosen so that Vt,.V)(0, 0) = (-1, 0) and the Hessian of -0
at (0, 0) is the diagonal matrix with first entry 1 and -1/2 as the remaining
nonzero diagonal elements. Therefore
[P, Q] _ --208 + 00 + 403 + lower order terms.
The first and last terms on the right-hand side are positive as operators,
but the middle term 0,, is not. But we only need positivity modulo P and
Q. This "bad" term can be absorbed by adding and subtracting a suitable
multiple of P to obtain.
[P, Q] = 20P- 408? - 00 + 203 + lower order terms.
The term 403 was very helpful and the multiple of P that was subtracted
needed to be small enough.to cancel only part of the 403 term. Furthermore,
because f = 0 on M = {t = 01,
boundary term = -20
J
(at f )2 dx.
t-o
Combining the formulas above one obtains
IILaf112+20 ft=o(8tf)2dx
IIPf112+IIQfI12+/3311f112+(f,-4082f)+(f,-,BOf)+20(f,Pf)
6jjVt, f112 + 2/3311fII2
4
[f11(Vt,.g)e,6,11I2
+
i03I{9ef+L112].
230
Chapter 14: Nodal Sets of Sums of Eigenfunctions
This proves the Carleman-type inequality (14.3).
Arguments of the kind
just given, involving commutators and integration by parts, can be found in
Hormander's text [12, p.
182] where they are attributed to F. Treves [26].
They are closely related to the arguments of Calderon [5]. The same device
was used by J. J. Kohn to make subelliptic estimates of operators arising in
analysis in several complex variables [17].
14.5
Applications to Sums of Eigenfunctions
Lemma 14.4 says
IIFIIHI(xl) <_ CIIFIIHI(V)IIFIIXI=(x2)'
and Lemma 14.5 says
I IFI IHI(v) <_ CI I atFII L2(BnM) II FII
HI(B+)
Therefore,
2 1 -2
.IIFIIHI(x,) S CIIc9tFIIL2(BnM)IIFIIHI(x2)
Recall that cP = O F. Recall further that, for T = 1, 2,
I
I FII HI(xr)
eTWII (PII
L2(M).
Combining these inequalities, one obtains the following theorem.
Theorem 14.6. Let eo be a sum of eigenfunctions as in Theorem 14.3. For
any ball B there exist constants C1 and C2 depending only on M and the
radius of B such that
II'PIIL2(M) <_ eCIW+C2II'IIL2(BnM)
Theorem 14.6 is the crucial global estimate. The remainder of the proof
of Theorem 14.3 follows [7, 8].
Theorem 14.7 ([7J, Proposition 6.7). Let B, denote the ball of radius r
in C. Let R" be the real n-dimensional subspace of purely real vectors of
C". Let H be a holomorphic function on B2 satisfying
IIHIILo(B2) <
e"IIHIIL-(Blnlen).
Then there are dimensional constants C1 and C2 such that
Wn_1({H=0}nB112nIR") <C1p+C2.
Theorems 14.6 and 14.7 and real-analytic hypoellipticity for the operator
8i + A on IR x M ([24, 27]) give the following corollary.

Jerison and Lebeau
231
Corollary 14.8. If M and aM are real analytic, and cp is as in Theorem
14.3, then
9'ln_1{gyp = 0} < C1w + C2-
Next, we wish to deduce the doubling property for V. If one replaces a ball
with a slightly smaller ball of comparable radius, elliptic regularity implies
that the Sobolev norm of F controls the L°° norm of F and vice versa.
In particular, Theorem 14.6 and elliptic regularity imply that F satisfies
doubling at unit scale. Next, Corollary 3.5 of [7] (applied to L with A = 0)
says that if F satisfies doubling at unit scale then it satisfies doubling at all
smaller scales.
Finally, we need to pass from doubling for F to doubling for W. Lemma
14.5 says that
I IFI I xl(v) <_ CI IOOFI I L2(BnM) I I FII H1(B+)
One can rescale this estimate to sets of size r < 1. At the same time we wish
to use more appropriate function spaces for this scaling, which is possible
because elliptic regularity estimates are valid independent of scale. To state
a rescaled version of Lemma 14.5, consider a ball B,. or radius r centered on
M and a ball VV, contained in B,+ = Br fl {t > 0}. Then
IIFIILc(V,)
<CIIra:FlIi-(B,nM)IIFII
"Br)-
The doubling condition for F proved in the preceding paragraph implies
IIFIIL°°(Blor) <_ e1"+1IIFIIL-(V,).
Combined with the scale-invariant version of Lemma 14.5, this yields
I
I rcoI I L-(B,TnM) <_ CI IFI IL-(B,,) <_ e °"i+°' I I rWI I L-(B,.nM),
which is the desired doubling condition for W.
We need to make a few more comments to complete the proof in the case
in which the boundary of M is nonempty. Lemma 14.5 was proved only for
balls that are disjoint from the boundary of M. Nevertheless, we shall see
that the doubling property for
is still valid up to the boundary. This is
because, as described in [8], one can glue an isomorphic copy of M onto M
at the boundary and consider even functions on the "doubled" manifold in
the case of Neumann boundary conditions. In the case of Dirichlet boundary
conditions, one takes the odd extension of the sum of eigenfunctions plus
the constant (even) extension of the constant. Then the C°,1 coefficient case
estimates of [4] apply, and one can prove the doubling property for F for
232
Chapter 14: Nodal Sets of Sums of Eggenfunctions
balls that do not touch the boundary. In this way, the estimate up to the
boundary of the type in Lemma 14.5 is never needed. Instead it suffices
to have such an estimate for balls whose diameters are comparable to their
distances to the boundary.
The estimate of Theorem 14.6 is best possible in the following sense.
Proposition 14.9. For any Sl C M such that Si # M, and any w > 1,
there exists a sum of eigenfunctions p of eigenvalue at most w such that
1k0IIL2(M) > ea''IIcoIIL2(n)
Proof. Consider the heat kernel
p(t, x, y) = Ee-t'l Vk(x)Vk(y)'
k
where cok is a complete system of normalized eigenfunctions. Then choose
0
y EM \H such that co (y) # 0. It is not hard to show that
sup p(t, x, y) < Ae-alt for 0
< t < 1.
ZEN
Let t = 1/w and define
V(x) _
[et'kcck(y)]
cok(x)
WkGW
L
Then
Ilp(t,
y) -
L2(M) S
E e-2 "k (1 +
Wk),
< Ce-`/t.
Wk>l/t
Hence
On the other hand,
II(PIIL2(n) < Ce-°/t
II(aIIL2(M) >- cI(PI(y)I > 0,
independent of t. This proves the proposition. While Theorem 14.6 is best
possible for sums of eigenfunctions, it is not optimal for single eigenfunc-
tions. For example, the eigenfunctions sin(7rkx) on the unit interval are
equidistributed.
Finally, let us note that the estimates hold for infinite sums of eigenfunc-
tions with exponentially decreasing coefficients.
Jerison and Lebeau
233
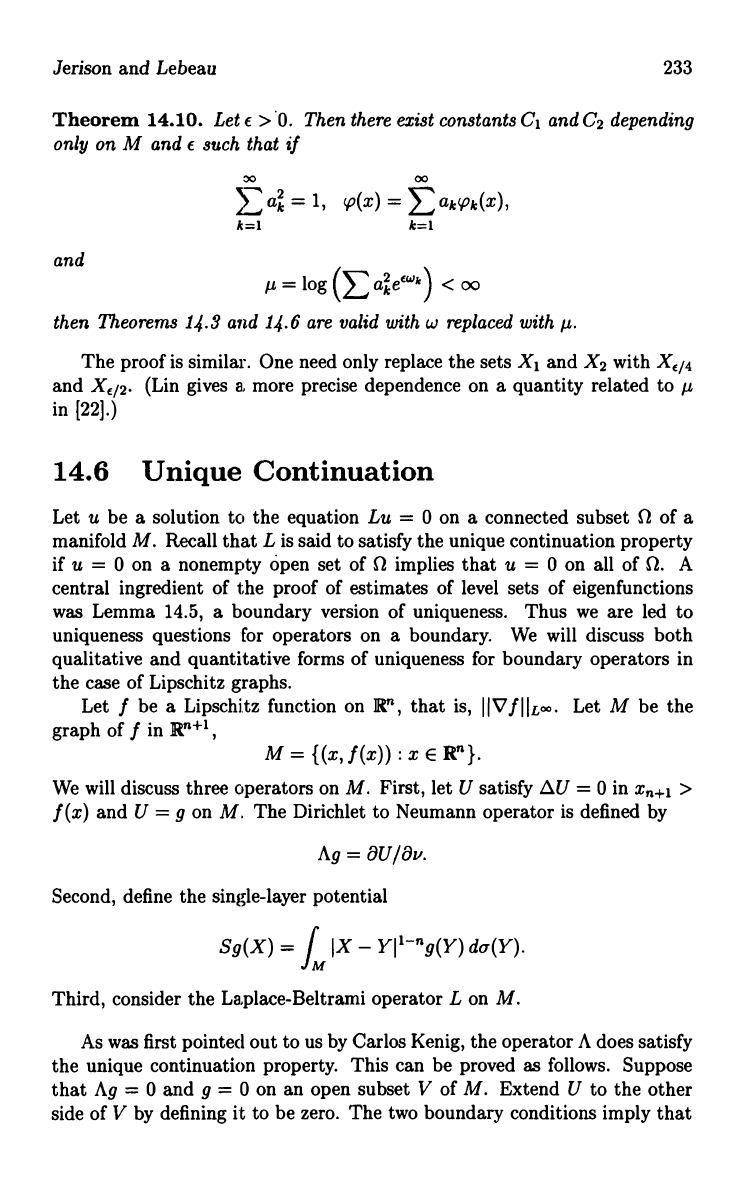
Theorem 14.10. Let e >'O. Then there exist constants C1 and C2 depending
only on M and a such that if
00 00
E akcok(x),
ak = 1,
'P(x) =
k=1 k=1
and
p = log M
akeE"k) < 00
then Theorems 14.3 and 14.6 are valid with w replaced with p,.
The proof is similar. One need only replace the sets X1 and X2 with XE14
and XE/2. (Lin gives a more precise dependence on a quantity related to p
in (22].)
14.6
Unique Continuation
Let u be a solution to the equation Lu = 0 on a connected subset S1 of a
manifold M. Recall that L is said to satisfy the unique continuation property
if u = 0 on a nonempty open set of St implies that u = 0 on all of Q. A
central ingredient of the proof of estimates of level sets of eigenfunctions
was Lemma 14.5, a boundary version of uniqueness. Thus we are led to
uniqueness questions for operators on a boundary. We will discuss both
qualitative and quantitative forms of uniqueness for boundary operators in
the case of Lipschitz graphs.
Let f be a Lipschitz function on R", that is, IIV f lILCC.
Let M be the
graph of f in R+1,
M={(x,f(x)):xER"}.
We will discuss three operators on M. First, let U satisfy DU = 0 in x"+1 >
f (x) and U = g on M. The Dirichlet to Neumann operator is defined by
Ag = 8U/8v.
Second, define the single-layer potential
- Yl"g(Y) da(Y).
Sg(X) = I
Third, consider the La.place-Beltrami operator L on M.
As was first pointed out to us by Carlos Kenig, the operator A does satisfy
the unique continuation property. This can be proved as follows. Suppose
that Ag = 0 and g = 0 on an open subset V of M. Extend U to the other
side of V by defining it to be zero. The two boundary conditions imply that
