Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon

Подождите немного. Документ загружается.

234

Chapter 14: Nodal Sets of Sums of Eigenfunctions

the equation AU = 0 is valid in a neighborhood in Rnt1 of any point of V.

Therefore, by unique continuation for the ordinary Laplace operator, U is

identically zero.

The qualitative uniqueness property for A led Kenig to conjecture quan-

titative forms of unique continuation such as Carleman inequalities.

It is

useful to formulate several quantitative properties that are closely related,

or perhaps equivalent. For example, one variant is the substitute for well-

posedness proved in Lemma 14.7. Another is the conjecture that if g = 0

on an open set, then IAgI satisfies a doubling condition. More precisely, the

doubling constant on a unit ball controls the doubling constant on all smaller

concentric balls. Furthermore, the density IAgJ should be an A.. weight. (For

a discussion of the weight condition for solutions of elliptic equations, see the

work of Garofalo and Lin [111.)

In particular, if JAgi were to satisfy the

weight property, then one could prove the following version of uniqueness: If

g = 0 on an open set V and Ag = 0 on a set of positive measure in V, then

U is identically zero. Results of this type have been proved by Adolfsson,

Escauriaza, Kenig, and Wang [1, 2, 16] for convex domains and for Cl," do-

mains for any a > 0. But as we have learned from the work of Calderdn, the

natural scale-invariant question is the one for Lipschitz graphs.

Let us turn to the second operator, the single-layer potential. This is an

integral operator with an explicit kernel that is smoothing of first order. (As

is well known, the operator is equal to A-1 in the case that M is a hyper-

plane and has the same principal part in general.) The analogous question

is whether solutions to Sg = 0 satisfy a doubling condition or if g is an A,,,,

weight on any bounded subset of M. As far as we know, this question has

not been raised before. Some evidence in favor of such bounds can be found

in [13], where certain Carleman-type estimates for fractional powers of the

Laplace operator are proved.

Finally, we consider the Laplace-Beltrami operator on M. If M were C1,1,

then the coefficients would be C°,1 and the theorem of [4] would imply unique

continuation for this operator. But since we are only assuming that M is

C°'1, the coefficients are merely bounded and measurable, an entire derivative

short of the hypothesis of [4]. The hypothesis that the coefficients are C0,1

is known to be best possible. Extending earlier examples of Plis [25], Miller

[23] gave an example in three variables of an elliptic second-order operator

with C° coefficients for any a < 1 having nonzero compactly supported

solutions. Thus there is evidence against any unique continuation result for

this operator. On the other hand, the examples of Plis and Miller are not

graphs and the assumption that M is a Lipschitz graph is known to suffice

for other estimates in harmonic analysis. (See [9, 10].) So we can still ask

the question whether unique continuation is valid for the Laplace-Beltrami

operator for M and whether solutions satisfy a doubling or A00 property.

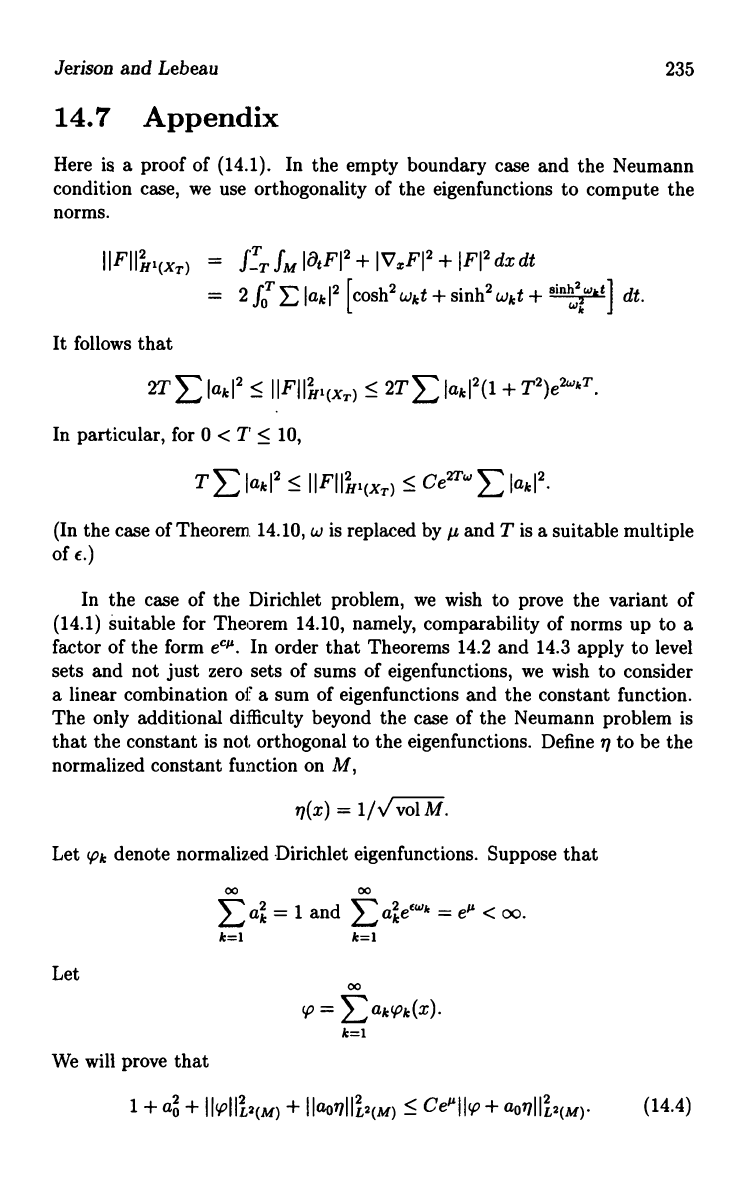
Jerison and Lebeau

235

14.7

Appendix

Here is a proof of (14.1).

In the empty boundary case and the Neumann

condition case, we use orthogonality of the eigenfunctions to compute the

norms.

IIFJ I Hl(xT)

= J T fM I

atFI2 + IV FI2 + IFI2 dx dt

= 2 f

E Iak I2 [cosh2 wkt + sinh2 Wkt + 81°n-2

`, dt.

It follows that

2TEIakI2 < IIFI12I(xT) <_ 2T>2Iakl2(1+T2)e2)tT.

In particular, for 0 < T< 10,

TEIakI2

< IIFI12 (xT)

<Ce2T"1: Iak12.

(In the case of Theorem. 14.10, w is replaced by it and T is a suitable multiple

of e.)

In the case of the Dirichlet problem, we wish to prove the variant of

(14.1) suitable for Theorem 14.10, namely, comparability of norms up to a

factor of the form e`µ. In order that Theorems 14.2 and 14.3 apply to level

sets and not just zero sets of sums of eigenfunctions, we wish to consider

a linear combination of a sum of eigenfunctions and the constant function.

The only additional difficulty beyond the case of the Neumann problem is

that the constant is not orthogonal to the eigenfunctions. Define 71 to be the

normalized constant function on M,

fi(x) = 1/

vol M.

Let Wk denote normalized Dirichlet eigenfunctions. Suppose that

00aa2

= land E ake"'k = eµ < 00.

k=1 k=1

Let

We will prove that

'F =

Eak'Pk(x)

k=1

1 + ao + I V II

L2(M) + I Iaorll I L2(M) <

Cep`) I' + a077112

2(M).

(14.4)

236

Chapter 14: Nodal Sets of Sums of Eigenfunctions

This gives the upper bound

II F + aot17I Ixl(x,,z) <_ a"III + ao771I L2(M).

The lower bound is similar, since a similar argument shows that any sum of

eigenfunctions with exponentially decreasing coefficients on sets t = to has

the same very weak orthogonality to the constant function.

We claim that there is a dimensional constant m and a constant C de-

pending on M such that

IWk(x)I < Cwk dist (x, W) (14.5)

for all x such that dist (x, 8M) < 1/wk. To prove (14.5), choose local coor-

dinates so that the boundary is a hyperplane and fix a cube with one face on

aM of side length 1/wk. Dilate by the factor wk to rescale the cube to a unit

cube. Denote by f the rescaled eigenfunction Wk. Then f satisfies an elliptic

equation of the form L f = cf, with a constant c comparable to 1 in a unit

cube with zero boundary conditions on one side. Moreover, since cpkk has L2

norm 1, the rescaled function has norm at most comparable to wk 2. Now

by elliptic interior and boundary regularity, we see that on half the cube f

is bounded by wk/2 times the distance to the boundary. This translates into

a bound on Wk of the form above with m = n/2 + 1.

Next, using (14.5), we have

Ic(x)I

Ek=1

C>k=l laklwk dist(x,COM)

< C(E'

la,Fe"'k)3(E001 wkme-`wk)i dist(x,8M)

< Ce' 12 dist (x, 8M).

In order to prove (14.4), note first that it follows from the triangle inequality

when ao is not comparable to 1. If, on the other hand, ao is comparable to 1,

then the estimate above shows that there is a neighborhood of 9M of volume

comparable to a-µ/2 on which IcpI < Iao77I/2. Therefore,

I I' + aorll IL-(M) >

ce-µ14,

which proves (14.4).

References

[1] V. Adolfsson and L. Escauriaza, C',* domains and unique continuation

at the boundary, Comm. Pure Appl. Math., vol. 50 (1997), 935-969.

Jerison and Lebeau

237

[2] V. Adolfsson, L. Escauriaza, and C. E. Kenig, Convex domains and unique

continuation at the boundary, Revista Matematica Iberoamericana, vol. 11

(1995), 513-525.

[3] N. Aronszajn, A unique continuation theorem for solutions of elliptic par-

tial differential equations or inequalities of second order, J. Math. Pures

et Appliquees 36 (1957)., 235-249.

[4] N. Aronszajn, A. Krzywicki, and J. Szarski, A unique continuation theo-

rem for exterior differential forms on Riemannian manifolds, Arkiv fiir

Matematik 4 (1963), 417-453.

[5) A. P. Calderon, Uniqueness in the Cauchy problem for partial differential

equations, Am. J. Math. 80 (1958), 16-36.

[6] H. Donnelly, Nodal sets for sums of eigenfunctions on Riemannian man-

ifolds, Proc A.M.S. 121 (1994), 967-973.

[7) H. Donnelly and C. Fefferman, Nodal sets of eigenfunctions on Rieman-

nian manifolds, Invent. Math. 93 (1988), 161-183.

[8] ,

Nodal sets of eigenfunctions: Riemannian manifolds with bound-

ary, Analysis, et cetera (Moser volume) (P. H. Rabinowitz, E. Zehnder,

ed.) Academic Press, Boston, 1990, pp. 251-262.

[9] E. B. Fabes, D. Jerison, and C. E. Kenig, Necessary and sufficient con-

ditions for absolute continuity of elliptic-harmonic measure, Ann. Math.

119 (1984), 121-141.

[10] R. A. Fefferman, C. E. Kenig, and J. Pipher, The theory of weights

and the Dirichlet problem for elliptic equations, Ann. Math. 134 (1991),

65-124.

[11] N. Garofalo and F: H. Lin, Monotonicity properties of variational inte-

grals, AP weights and unique continuation, Indiana Univ. Math. J. 35

(1986), 245-267.

[12] L. Hormander, Linear Partial Differential Operators, Springer-Verlag,

Berlin, 1963.

[13] D. Jerison and C. E.Kenig, Unique continuation and absence of positive

eigenvalues for Schrodinger operators, Ann. Math. 121 (1985), 463-488.

[14] F. John, A note on "improper" problems in partial differential equations,

Comm. Pure Appl. Math. 8 (1955), 591-594.

238

Chapter 14: Nodal Sets of Sums of Eigenfunctions

[15] ,

Continuous dependence on data for solutions of partial differ-

ential equations with a prescribed bound, Comm. Pure Appl. Math. 13

(1960), 443-492.

[16] C. E. Kenig and W. Wang, A note on boundary unique continuation

for harmonic functions in nonsmooth domains, Potential Analysis, vol. 8

(1998), 143-147.

[17] J. J. Kohn, Boundaries of complex manifolds, Proceedings Conf. on

Complex Manifolds, Minneapolis, 1964, pp. 81--94.

(18] I. Kukavica, Hausdorff measure of level sets for solutions of parabolic

equations, Internat. Math. Research Notes 13 (1995), 671-682.

[19] ,

Nodal volumes for eigenfunctions of analytic regular elliptic

problems, J. d'Analyse Math., vol. 67 (1995), 269-280.

[20] ,

Quantitative uniqueness for second-order elliptic operators, Duke

Math. J., vol. 91 (1998), 225-240.

[21] G. Lebeau and L. Robbiano, Controle exact de l'equation de la chaleur,

Comm. in P.D.E. 20 (1995), 335-356.

[22] F.-H. Lin, Nodal sets of solutions of elliptic and parabolic equations,

Comm. Pure App!. Math. 44 (1991), 287-308.

(23] K. Miller, Non-unique continuation for certain ODE's in Hilbert Space

and for uniformly parabolic and elliptic equations in self-adjoint divergence

form, Symposium on Non-Well-Posed Problems and Logarithmic Convex-

ity. Springer Lecture Notes 316 (R. J. Knops, ed.), Springer-Verlag, Berlin,

1973, pp. 85-101.

[24] C. B. Morrey and L. Nirenberg, On the analyticity of the solutions of

linear elliptic systems of partial differential equations, Comm. on Pure

Appl. Math. 10 (1957), 271-290.

[25] A. Plis, On non-uniqueness in the Cauchy problem for an elliptic second

order differential equation, Bull. Acad Sci Polon., Ser. Sci Math. Astro.

Phys. 11 (1963), 95-100.

126] F. Treves, Relations de domination entre operateurs differentiels, Acta

Math. 101 (1959), 1-139.

[27]

, Introduction to Pseudodifferential Operators and Fourier Inte-

gral Operators, Vol. 1, Plenum Press, New York, 1980, Theorem 5.4.

Jerison and Lebeau

239

[28] T. H. Wolff, Counterexamples with harmonic gradients in R3, Essays on

Fourier Analysis in Honor of Elias M. Stein (C. Fefferman et al., eds.),

Princeton University Press, Princeton, NJ, 1995, pp. 321-384.

[29]

, Recent work on sharp estimates in second-order elliptic unique

continuation problems, J. Geometric Analysis 3 (1993), 621-650.

Chapter 15

Large-time Behavior and

Self-similar Solutions of Some

Semilinear Diffusion Equations

Yves Meyer

In his Ph.D. dissertation, M. Cannone constructed self-similar solutions to

the Navier-Stokes equations. Then F. Planchon proved that these self-similar

solutions provide the asymptotic behavior at large scales of some global so-

lutions ([3], [18]).

Here we want to show that these results are not specific to the 3-D Navier-

Stokes equation but are also valid for the nonlinear heat equation

= Du + yu3

whatever be the sign of y and for the nonlinear Schrodinger equation.

This survey paper is organized as follows. We first review some well-

known material concerning the nonlinear heat equation = Du + u3 (blow

up in finite time and self-similar solutions) in §§15.1 to 15.6. We show that

these classical results are better understood when one is using a strategy

due to T. Kato [9]. This strategy amounts to finding a solution as a vector-

valued function u(x, t) of the time variable t > 0. More precisely, u will

belong to C((0, oo); E), and the novelty of our approach consists in replacing

the Lebesgue space E = L3(1R3) which was used by T. Kato by a suitable

Besov space E. Using such a Besov space offers many advantages. It permits

us to explain the role played by the oscillations of the initial value uo(x) in

the lifetime of the corresponding solution. This would not be possible if the

L3-norm were used. Moreover, the Besov spaces we will use contain functions

241

242

Chapter 15: Large-time Behavior of Semi-linear Diffusion Equations

which are homogeneous of degree -1. This is not the case for L3 (R3 ). Such

homogeneous u0 initial values will generate self-similar solutions.

In §§15.7 and 15.8 we show that the self-similar solutions which are con-

structed in the preceding sections are driving the large-scale behavior of most

of the global solutions to our nonlinear heat equation. In §15.9 we treat the

nonlinear heat equation with the opposite sign.

Sections 15.10 and 15.12 are devoted to Navier-Stokes equations which

obey the same scaling laws as our model nonlinear heat equations. The same

type of methods applies.

Finally in §15.13 we review some quite recent results obtained by T.

Cazenave and F. Weissler. These authors constructed self-similar solutions

for the nonlinear Schrodinger equation. The Besov spaces which we used in

the previous examples are now replaced by a new Banach space which looks

quite exciting.

15.1 A First Model Case: the Nonlinear Heat

Equation

Our first model case will be the following nonlinear heat equation

5 at = Du + u3 on 1[t3 x (0, oo),

u(x, 0) = uo(x)

(15.1)

where u = u(x, t) is a real-valued function of x E 1R3 and t > 0. Below we

will be much more specific about the functional spaces in which the solutions

will be constructed. For the time being we are considering classical solutions

to (15.1) with enough regularity and with appropriate size estimates. For

example, for the time being, all LP-norms in the x variable are supposed to

exist.

Multiplying (15.1) by u and integrating over R3 yields the equation 1 ae I Iui I2

_ -IIVuII2 + IIuII4 which means that the evolution results from a competi-

tion between IIuII4 and IIVuII2. This remark makes the following theorem

plausible.

Theorem 15.1 (J. Ball [1], H. A. Levine [13], and L. Payne [15]). If

uo E Co (I23), uo 0 0 and

IIDUoII2 :5 IIu0lI4,

(15.2)

then the corresponding solution to (15.1) blows up in finite time.

Meyer 243

It means that there exists a To < oo such that

lim sup I Iu(', t)

112 = +00.

0Tb

Let us make a few comments about this theorem. If u(x, t) is a solution

to (15.1), so are ua(x, t) = Au (Ax, A2t) for any A > 0. The L4-norm is not

invariant under this resealing and a condition of the type f f uof f 4 < 97 for

some small 77 cannot imply the existence of a global solution. Indeed, such

a condition would always be satisfied by one among the resealed solutions

u),, A > 0. However, the V-norm is invariant, and we will see below that

11u0113 < i7, 77 small enough, implies that the corresponding solution exists

globally in time.

A second remark is the following. If V E Co (R3) then for e > 0 small

enough, V(ex) = uo(x) will satisfy (15.2).

In contrast for any cp E Co (R3), there exists a positive wo = wo((p) such

that I'4 > wo, and uo(x) = e'"''xW(x) imply that the corresponding solution

u(x, t) to (15.1) is global in time.

Our next step is to find sufficient conditions on the initial value uo(x)

that imply that the corresponding solution u(x, t) will be global in time.

Such sufficient conditions will be of the type f f uOIIB < r7, where B is some

convenient Besov space which we will now describe.

15.2 Some Besov Spaces

We consider a function p belonging to the Schwartz class S(R3) with the

following two properties: cp(C) = 1 on f ff < 1 and cp(C) = 0 on off > 4/3.

Here and in what follows, f will denote the Fourier transform of f defined

by f (f) = f e"I f (x) dx.. Next Wj(x) = 23jW(21x), Sj(f) = f * wj, Oj =

Sj+1 - Sj, and O(x) = 23cp(2x) - W(x) in such a way that Oj(f) = f * 1/ij

If a > 0, 1 < q < oo, the homogeneous Besov space Bq °'° is defined by

the condition

f1Sj(f)Ifq :f- C2j°, j E Z.

(15.3)

The usual definition (which applies as well when a is any real number) is

IIOj (f) I Iq < C2j" (j E Z), but this standard definition is equivalent to (15.3)

if a > 0.

The drawback of the standard definition is the fact that any

polynomial P satisfies A j (P) = 0, which forces us to add some requirement

to get rid of such polynomials. This is not the case for the "clean definition"

given by (15.3).

When a > 0, Bq

is a Banach space consisting of tempered distribu-

tions. This Banach space is not separable but is the dual space of Bp ,

which