Chow T.L. Mathematical Methods for Physicists: A Concise Introduction
Подождите немного. Документ загружается.


Since
I
0;0
Z
1
ÿ1
e
ÿx
2
dx ÿ 1=2
p
;
we ®nd that
I
n;n
Z
1
ÿ1
e
ÿx
2
H
n
xH
n
xdx 2
n
n!
p
: 7:51
We can also use the generating function for the Hermite polynomials:
e
2txÿt
2
X
1
n0
H
n
xt
n
n!
; e
2sxÿs
2
X
1
m0
H
m
xs
m
m!
:
Multiplying these, we have
e
2txÿt
2
2sxÿs
2
X
1
m0
X
1
n0
H
m
xH
n
xs
m
t
n
m!n!
:
Multiplying by e
ÿx
2
and integrating from ÿ1 to 1 gives
Z
1
ÿ1
e
ÿxst
2
ÿ2st
dx
X
1
m0
X
1
n0
s
m
t
n
m!n!
Z
1
ÿ1
e
ÿx
2
H
m
xH
n
xdx:
Now the left hand side is equal to
e
2st
Z
1
ÿ1
e
ÿxst
2
dx e
2st
Z
1
ÿ1
e
ÿu
2
du e
2st
p
p
X
1
m0
2
m
s
m
t
m
m!
:
By equating coecients the required result follows.
It follows that the functions 1=2
n
n!
n
p
1=2
e
ÿx
2
H
n
x form an orthonormal set.
We shall assume it is complete.
Laguerre's equation
Laguerre's equation is
xD
2
y 1 ÿ xDy y 0: 7:52
This equation and its solut ions (Laguerre functions) are of interest in quantum
mechanics (e.g., the hydrogen problem). The origin x 0 is a regular singular
point and so we write
yx
X
1
k0
a
k
x
k
: 7:53
By substitution, Eq. (7.52) becomes
X
1
k0
k
2
a
k
x
kÿ1
ÿ k a
k
x
k
0 7:54
316
SPECIAL FUNCTIONS OF MATHEMATICAL PHYSICS

from which we ®nd that the indicial equation is
2
0. And then (7.54) reduces to
X
1
k0
k
2
a
k
x
kÿ1
ÿ ka
k
x
k
0:
Changing k ÿ 1tok
0
in the ®rst term, then renaming k
0
k, we obtain
X
1
k0
fk 1
2
a
k1
ÿ ka
k
gx
k
0;
whence the recurrence relations are
a
k1
k ÿ
k 1
2
a
k
: 7:55
When is a posit ive integer n, the recurrence relations give a
k1
a
k2
0,
and
a
1
ÿn
1
2
a
0
; a
2
ÿn ÿ 1
2
2
a
1
ÿ1
2
n ÿ 1n
1 2
2
a
0
;
a
3
ÿn ÿ 2
3
2
a
2
ÿ1
3
n ÿ 2n ÿ 1n
1 2 3
2
a
0
; etc:
In general
a
k
ÿ1
k
n ÿ k 1n ÿ k 2n ÿ 1n
k!
2
a
0
: 7:56
We usually choose a
0
ÿ1n!, then the polynomial solution of Eq. (7.52) is given
by
L
n
xÿ1
n
x
n
ÿ
n
2
1!
x
nÿ1
n
2
n ÿ 1
2
2!
x
nÿ2
ÿÿ1
n
n!
()
: 7:57
This is called the Laguerre polynomial of degree n. We list the ®rst four Laguerre
polynomials below:
L
0
x1; L
1
x1 ÿ x; L
2
x2 ÿ 4x x
2
; L
3
x6 ÿ 18x 9x
2
ÿ x
3
:
The generating function for the Laguerre polynomials L
n
x
This is given by
x; z
e
ÿxz=1ÿz
1 ÿ z
X
1
n0
L
n
x
n!
z
n
: 7:58
317
LAGUERRE'S EQUATION

By writing the series for the exponential and collecting powers of z, you can verify
the ®rst few terms of the series. And it is also straightforward to show that
x
@
2
@x
2
1 ÿ x
@
@x
z
@
@z
0:
Substituting the right hand side of Eq. (7.58), that is, x; z
P
1
n0
L
n
x=n!z
n
,
into the last equation we see that the functions L
n
x satisfy Laguerre's equation.
Thus we identify x; z as the generating function for the Laguerre polynomials.
Now multiplying Eq. (7.58) by z
ÿnÿ1
and integrating around the origin, we
obtain
L
n
x
n!
2i
I
e
ÿxz=1ÿz
1 ÿ zz
n1
dz; 7:59
which is an integral representation of L
n
x.
By diÿerentiating the generating function in Eq. (7.58) with respect to x and z,
we obtain the recurrence relations
L
n1
x2n 1 ÿ xL
n
xÿn
2
L
nÿ1
x;
nL
nÿ1
xnL
0
nÿ1
xÿL
0
n
x:
)
7:60
Rodrigues' formula for the Laguerre polynomials L
n
x
The Laguerre polynomials are also given by Rodrigues' formula
L
n
xe
x
d
n
dx
n
x
n
e
ÿx
: 7:61
To prove this formula, let us go back to the integral representation of L
n
x, Eq.
(7.59). With the transformation
xz
1 ÿ z
s ÿ x or z
s ÿ x
s
;
Eq. (7.59) becomes
L
n
x
n!e
x
2i
I
s
n
e
ÿn
s ÿ x
n1
ds;
the new contour enclosing the point s x in the s plane. By Cauchy's integral
formula (for derivatives) this reduces to
L
n
xe
x
d
n
dx
n
x
n
e
ÿx
;
which is Rodrigues' formula.
Alternatively, we can diÿerentiate Eq. (7.58) n times with respect to z and
afterwards put z=0, and thus obtain
e
x
lim
z!0
@
n
@z
n
1 ÿ z
ÿ1
exp
ÿx
1 ÿ z
hi
L
n
x:
318
SPECIAL FUNCTIONS OF MATHEMATICAL PHYSICS
But
lim
z!0
@
n
@z
n
1 ÿ z
ÿ1
exp
ÿx
1 ÿ z
hi
d
n
dx
n
x
n
e
ÿx
;
hence
L
n
xe
x
d
n
dx
n
x
n
e
ÿx
:
The orthogonal Laguerre functions
The Laguerre polynomials, L
n
x, do not by themselves form an orthogonal set.
But the functions e
ÿx=2
L
n
x are orthogonal in the interval (0, 1). For any two
Laguerre polynomials L
m
x and L
n
x we have, from Laguerre's equation,
xL
00
m
1 ÿ xL
0
m
mL
m
0;
xL
00
n
1 ÿ xL
0
n
mL
n
0:
Multiplying these equations by L
n
x and L
m
x respectively and subtracting, we
®nd
xL
n
L
00
m
ÿ L
m
L
00
n
1 ÿ xL
n
L
0
m
ÿ L
m
L
0
n
n ÿ mL
m
L
n
or
d
dx
L
n
L
0
m
ÿ L
m
L
0
n
1 ÿ x
x
L
n
L
0
m
ÿ L
m
L
0
n
n ÿ mL
m
L
n
x
:
Then multiplying by the integratin g factor
exp
Z
1 ÿ x=xdx expln x ÿ xxe
ÿx
;
we have
d
dx
fxe
ÿx
L
n
L
0
m
ÿ L
m
L
0
n
g n ÿ me
ÿx
L
m
L
n
:
Integrating from 0 to 1 gives
n ÿ m
Z
1
0
e
ÿx
L
m
xL
n
xdx xe
ÿx
L
n
L
0
m
ÿ L
m
L
0
n
j
1
0
0:
Thus if m 6 n
Z
1
0
e
ÿx
L
m
xL
n
xdx 0 m 6 n; 7:62
which proves the required result.
Alternatively, we can use Rodrigues' formula (7.61). If m is a positive integer,
Z
1
0
e
ÿx
x
m
L
m
xdx
Z
1
0
x
m
d
n
dx
n
x
n
e
ÿx
dx ÿ1
m
m!
Z
1
0
d
nÿm
dx
nÿm
x
n
e
ÿx
dx;
7:63
319
LAGUERRE'S EQUATION

the last step resulting from integrating by parts m times. The integral on the right
hand side is zero when n > m and, since L
n
x is a polynomial of degree m in x,it
follows that
Z
1
0
e
ÿx
L
m
xL
n
xdx 0 m 6 n;
which is Eq. (7.62). The reader can also apply Eq. (7.63) to show that
Z
1
0
e
ÿx
L
n
x
fg
2
dx n!
2
: 7:64
Hence the functions fe
ÿx=2
L
n
x=n!g form an orthonormal system.
The associ ated Laguerre polynomials L
m
n
x
Diÿerentiating Laguerre's equation (7.52) m times by the Leibnitz theorem we
obtain
xD
m2
y m 1 ÿ xD
m1
y n ÿ mD
m
y 0 n
and writing z D
m
y we obtain
xD
2
z m 1 ÿ xDz n ÿ mz 0: 7:65
This is Laguerre's associated equation and it clearly possesses a polynomial solu-
tion
z D
m
L
n
xL
m
n
xm n; 7:66
called the associated Laguerre polynomial of degree (n ÿ m). Using Rodrigues'
formula for Laguerre polynomial L
n
x, Eq. (7.61), we obtain
L
m
n
x
d
m
dx
m
L
n
x
d
m
dx
m
e
x
d
n
dx
n
x
n
e
ÿx
: 7:67
This result is very useful in establishing further properties of the associated
Laguerre polynomials. The ®rst few polynomials are listed below:
L
0
0
x1; L
0
1
x1 ÿ x; L
1
1
xÿ1;
L
0
2
x2 ÿ 4x x
2
; L
1
2
xÿ4 2x; L
2
2
x2:
Generating function for the associated Laguerre polynomials
The Laguerre polynomial L
n
x can be generated by the function
1
1 ÿ t
exp
ÿxt
1 ÿ t
X
1
n0
L
n
x
t
n
n!
:
320
SPECIAL FUNCTIONS OF MATHEMATICAL PHYSICS
Diÿerentiating this k times with respect to x, it is seen at once that
ÿ1
k
1 ÿ t
ÿ1
t
1 ÿ t
k
exp
ÿxt
1 ÿ t
X
1
k
L
k
x
!
t
: 7:68
Associated Laguerre function of inte gral order
A function of great importance in quantum mechanics is the associated Laguerre
function that is de®ned as
G
m
n
xe
ÿx=2
x
mÿ1=2
L
m
n
xm n: 7:69
It is signi®cant largely because jG
m
n
xj ! 0asx !1. It satis®es the diÿerential
equation
x
2
D
2
u 2xDu n ÿ
m ÿ 1
2
x ÿ
x
2
4
ÿ
m
2
ÿ 1
4
"#
u 0: 7:70
If we substitute u e
ÿx=2
x
mÿ1=2
z in this equation, it reduces to Laguerre's asso-
ciated equation (7.65). Thus u G
m
n
satis®es Eq. (7.70). You will meet this equa-
tion in quantum mechanics in the study of the hydrogen atom.
Certain integrals involving G
m
n
are often used in quantum mechanics and they
are of the form
I
n;m
Z
1
0
e
ÿx
x
kÿ1
L
k
n
xL
k
m
xx
p
dx;
where p is also an integer. We will not consider these here and instead refer the
interested reader to the following book: The Mathem atics of Physics and
Chemistry, by Henry Margenau and George M. Murphy; D. Van Nostrand Co.
Inc., New York, 1956.
Bessel's equation
The diÿerential equation
x
2
y
00
xy
0
x
2
ÿ
2
y 0 7:71
in which is a real and positive constant, is known as Bessel's equatio n and its
solutions are called Bessel functions. These functio ns were used by Bessel
(Friedrich Wilhelm Bessel, 1784±1864, German mathematician and astronomer)
extensively in a problem of dynamical astronomy. The importance of this
equation and its solutions (Bessel functions) lies in the fact that they occur fre-
quently in the boundary-value problems of mathematical physics and engineering
321
BESSEL'S EQUATION

involving cylindrical symmetry (so Bessel functions are sometimes called cylind-
rical functions), and many others. There are whole books on Bessel functio ns.
The origin is a regular singul ar point, and all other values of x are ordinary
points. At the origin we seek a series solution of the form
yx
X
1
m0
a
m
x
m
a
0
6 0: 7:72
Substituting this and its derivatives into Bessel's equation (7.71), we have
X
1
m0
m m ÿ 1a
m
x
m
X
1
m0
m a
m
x
m
X
1
m0
a
m
x
m2
ÿ
2
X
1
m0
a
m
x
m
0:
This will be an identity if and only if the coecien t of every power of x is zero. By
equating the sum of the coecients of x
k
to zero we ®nd
ÿ 1a
0
a
0
ÿ
2
a
0
0 k 0; 7:73a
ÿ 1a
1
1a
1
ÿ
2
a
1
0 k 1; 7:73b
k k ÿ 1a
k
k a
k
a
kÿ2
ÿ
2
a
k
0 k 2; 3; ...: 7:73c
From Eq. (7.73a) we obtain the indicial equation
ÿ 1 ÿ
2
ÿ 0:
The roots are . We ®rst determine a solution corresponding to the positive
root. For , Eq. (7.73b) yields a
1
0, and Eq. (7.73c) takes the form
k 2ka
k
a
kÿ2
0; or a
k
ÿ1
kk 2
a
kÿ2
; 7:74
which is a recurrence formula: since a
1
0 and 0, it follows that
a
3
0; a
5
0; ...; successively. If we set k 2m in Eq. (7.74), the recurrence
formula becomes
a
2m
ÿ
1
2
2
m m
a
2mÿ2
; m 1; 2; ... 7:75
and we can determine the coecients a
2
; a
4
, successivel y. We can rewrite a
2m
in
terms of a
0
:
a
2m
ÿ1
m
2
2m
m! m 2 1
a
0
:
322
SPECIAL FUNCTIONS OF MATHEMATICAL PHYSICS

Now a
2m
is the coecient of x
2m
in the seri es (7.72) for y. Hence it would be
convenient if a
2m
contained the factor 2
2m
in its denominat or instead of just 2
2m
.
To achieve this, we write
a
2m
ÿ1
m
2
2m
m! m 2 1
2
a
0
:
Furthermore, the factors
m 2 1
suggest a factorial. In fact, if were an integer, a factorial could be created by
multiplying numerator by ! . However, since is not necessarily an integer, we
must use not ! but its generalization ÿ 1 for this purpose. Then, except for
the values
ÿ1; ÿ2; ÿ3; ...
for which ÿ 1 is not de®ned, we can write
a
2m
ÿ1
m
2
2m
m! m 2 1ÿ 1
2
ÿ 1a
0
:
Since the gamma function satis®es the recurrence relation zÿzÿz 1, the
expression for a
2m
becomes ®nally
a
2m
ÿ1
m
2
2m
m!ÿ m 1
2
ÿ 1a
0
:
Since a
0
is arbitrary, and since we are looking only for particular solutions, we
choose
a
0
1
2
ÿ 1
;
so that
a
2m
ÿ1
m
2
2m
m!ÿ m 1
; a
2m1
0
and the series for y is, from Eq. (7.72),
yxx
1
2
ÿ 1
ÿ
x
2
2
2
ÿ 2
x
4
2
4
2!ÿ 3
ÿ
"#
X
1
m0
ÿ1
m
2
2m
m!ÿ m 1
x
2m
: 7:76
The function de®ned by this in®nite series is known as the Bessel function of the
®rst kind of order and is denoted by the symbol J
x. Since Bessel's equation
323
BESSEL'S EQUATION
of order has no ®nite singular points except the origin, the ratio test will show
that the series for J
x converges for all values of x if 0.
When n, an integer, solution (7.76) becomes, for n 0
J
n
xx
n
X
1
m0
ÿ1
m
x
2m
2
2mn
m!n m!
: 7:76a
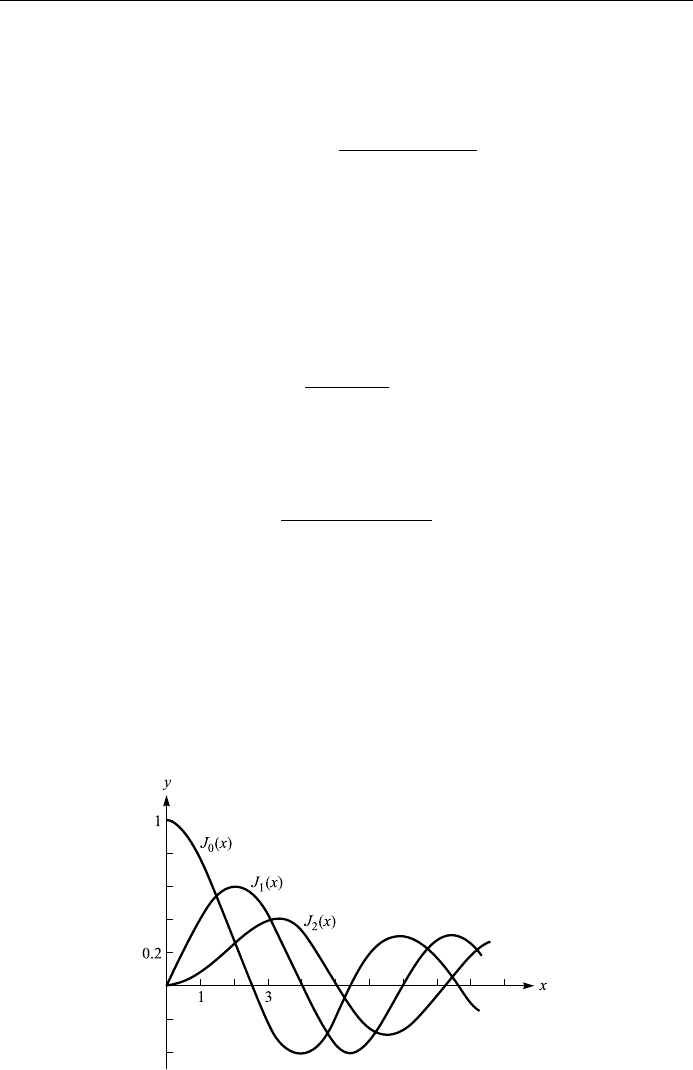
The graphs of J
0
x; J
1
x, and J
2
x are shown in Fig. 7.3. Their resemblance to
the graphs of cos x and sin x is interesting (Problem 7.16 illustrates this for the
®rst few terms). Fig. 7.3 also illustrates the important fact that for every value of
the equation J
x0 has in®nitely many real roots.
With the second root ÿ of the indicial equation, the recurrence relation
takes the form (from Eq. (7.73c))
a
k
ÿ1
kk ÿ 2
a
kÿ2
: 7:77
If is not an integer, this leads to an independent second solution that can be
written
J
ÿ
x
X
1
m0
ÿ1
m
m!ÿÿ m 1
x=2
ÿ2m
7:78
and the complete solution of Bessel's equation is then
yxAJ
xBJ
ÿ
x; 7:79
where A and B are arbitrary constants.
When is a positive integer n, it can be shown that the formal expression for
J
ÿn
x is equal to (ÿ1
n
J
n
x.SoJ
n
x and J
ÿn
x are linearly dependent and Eq.
(7.79) cannot be a general solution. In fact, if is a positive integer, the recurrence
324
SPECIAL FUNCTIONS OF MATHEMATICAL PHYSICS
Figure 7.3. Bessel functions of the ®rst kind.

relation (7.77) breaks down when 2 k and a second solution has to be found
by other methods. There is a diculty also when 0, in which case the two
roots of the indicial equation are equal; the second solution must also found by
other methods. These will be discussed in next section.
The results of Problem 7.16 are a special case of an important general theorem
which states that J
x is expressible in ®nite terms by means of algebraic and
trigonometrical functions of x whenever is half of an odd integer. Further
examples are
J
3=2
x
2
x
1=2
sin x
x
ÿ cos x
;
J
ÿ5=2
x
2
x
1=2
3 sin x
x
3
x
2
ÿ 1
cos x
:
The functions J
n1=2
x and J
ÿn1=2
x, where n is a positive integer or zero, are
called spherical Bessel functions; they have important applications in problems of
wave motion in whi ch spherical polar coordinates are appropriate.
Bessel functions of the second kind Y
n
x
For integer n; J
n
x and J
ÿn
x are linearly dependent and do not form a
fundamental system. We shall now obtain a second independent solution, starting
with the case n 0. In this case Bessel's equation may be written
xy
00
y
0
xy 0; 7:80
the indicial equation (7.73a) now, with 0, has the double root 0. Then we
see from Eq. (7.33) that the desired solution must be of the form
y
2
xJ
0
xln x
X
1
m1
A
m
x
m
: 7:81
Next we substitute y
2
and its derivatives
y
0
2
J
0
0
ln x
J
0
x
X
1
m1
mA
m
x
mÿ1
;
y
00
2
J
00
0
ln x
2J
0
0
x
ÿ
J
0
x
2
X
1
m1
mm ÿ 1A
m
x
mÿ2
into Eq. (7.80). Then the logarithmic terms disappear because J
0
is a solution of
Eq. (7.80), the other two terms containing J
0
cancel, and we ®nd
2J
0
0
X
1
m1
mm ÿ 1A
m
x
mÿ1
X
1
m1
mA
m
x
mÿ1
X
1
m1
A
m
x
m1
0:
325
BESSEL'S EQUATION
