Choudhry. Fixed Income Securities Derivatives Handbook

Подождите немного. Документ загружается.

52 Introduction to Bonds

The markets assume that the state variables evolve through a geomet-

ric Brownian motion, or Weiner process. It is therefore possible to model

their evolution using a stochastic differential equation. The market also

assumes that the cash ﬂ ow stream of assets such as bonds and equities is a

function of the state variables.

A bond is characterized by its coupon process, represented in (3.9).

Ct CXtXtXt X tt

()= () () () ()

[]

, , ,....., ,

123

(3.9)

The coupon process represents the cash ﬂ ow investors receive while

they hold the bond. Assume that a bond’s term can be divided into very

small intervals of length dt and that it is possible to buy very short-term

discount bonds, such as Treasury strips, maturing at the end of each

such interval and paying an annualized rate r (t). This rate is the short, or

instantaneous, rate, which in mathematical bond analysis is deﬁ ned as the

rate of interest charged on a loan taken out at time t that matures almost

immediately. The short rate is given by formulas (3.10) and (3.11).

rt rtt()=

()

, (3.10)

The short rate is the interest rate on a loan that is paid back almost

instantaneously; it is a theoretical construct. Equation (3.11) states this

mathematical notation in terms of the bond price.

Ptt()=−

∂

()

log ,

(3.11)

If the principal of the short-term security described above is continu-

ously reinvested at this short rate, the cumulative amount obtained at time

t is equal to the original investment multiplied by expression (3.12).

Mt rsds

()=

(

)

⎡

⎣

⎢

⎤

⎦

⎥

∫

exp

(3.12)

where M is a money market account that offers a return of the short rate

r (t). The bond principal is multiplied by an account and M(t) is the total

rate of return of such an account.

If the short rate is constant—that is, r (t) = r—then the price of a

risk-free bond that pays $1 on its maturity date T is given by expres-

sion (3.13).

PtT e

rT t

()

−−

()

(3.13)

Bond Pricing and Spot and Forward Rates 53

Expression (3.13) states that the bond price is a function of the con-

tinuously compounded interest rate. The right-hand side is the discount

factor at time t. At t = T—that is, on the redemption date—the discount

factor is 1, which is the redemption value of the bond and hence the price

of the bond at that time.

Consider a scenario in which a market participant can either

❑ invest

rT t−−

()

units of cash in a money market account, for a return

of $1 at time T, or

❑ purchase a risk-free zero-coupon bond that has a maturity value of

$1 at time T

The bond and the money market are both risk-free and have identical

payouts at time T, and neither will generate any cash ﬂ ow between now

and time T. Since the interest rates involved are constant, the bond must

have a value equal to the initial investment in the money market account:

rT t−−

()

. In other words, equation (3.13) must hold. This is a restriction

placed on the zero-coupon bond price by the requirement for markets to

be arbitrage-free.

If the bond were not priced at this level, arbitrage opportunities would

present themselves. Say the bond was priced higher than

rT t−−

()

. In this

case, investors could sell the bond short and invest et–r (T-t) of the sale

proceeds in the money market account. At time T, the short position

would have a value of –$1 (negative, because the bond position is short);

the money market, meanwhile, would have grown to $1, which the inves-

tors could use to close their short bond positions. And they would still

have funds left over from the short sale, because at time t

PtT e

rT t

()

−>

−−

()

So they would proﬁ t from the transaction at no risk to themselves.

A similar situation obtains if the bond price P(t, T ) is less than

rT t−−

()

In that case, the investors borrow

rT t−−

()

at the money market rate. They

then use P(t, T ) of this loan to buy the strip. At maturity the bond pays

$1, which the investors use to repay the loan. But they still have surplus

borrowed funds, because

ePtT

rT t−−

()

−

()

>,0

This demonstrates that the only situation in which no arbitrage proﬁ t

can be made is when

PtT e

rT t

()

−−

()

. (For texts providing more detail on

arbitrage pricing theory, see the References section.)

54 Introduction to Bonds

In the academic literature, the risk-neutral price of a zero-coupon

bond is expressed in terms of the evolution of the short-term interest rate,

r (t)—the rate earned on a money market account or on a short-dated

risk-free security such as the T-bill—which is assumed to be continuously

compounded. These assumptions make the mathematical treatment sim-

pler. Consider a zero-coupon bond that makes one payment, of $1, on its

maturity date T. Its value at time t is given by equation (3.14), which is the

redemption value of 1 divided by the value of the money market account,

given by (3.12).

PtT rsds

,exp

()

=−

(

)

⎡

⎣

⎢

⎤

⎦

⎥

∫

(3.14)

The price of a zero-coupon bond in terms of its yield is given by equa-

tion (3.15).

PtT T trT t,exp

()

=−−

()

−

()

⎡

⎣

⎤

⎦

(3.15)

Expression (3.14) is the formula for pricing zero-coupon bonds

when the spot rate is the nonconstant instantaneous risk-free rate r (s)

described above. This is the rate used in formulas (3.12), for valuing a

money market account, and (3.15), for pricing a risk-free zero-coupon

bond.

Stochastic Rates

In the academic literature, the bond price given by equation (3.15) evolves

as a martingale process under the risk-neutral probability measure



. This

process is the province of advanced ﬁ xed-income mathematics and lies

outside the scope of this book. An introduction, however, is presented in

chapter 4, which can be supplemented by the readings listed in the Refer-

ences section.

Advanced ﬁ nancial analysis produces the bond price formula (3.16)

(for the formula’s derivation, see Neftci (2000), page 417).

PtT E e

rsds

()

⎡

⎣

⎢

⎤

⎦

⎥

−

()

∫



(3.16)

The right-hand side of (3.16) is the randomly evolved discount factor

used to obtain the present value of the $1 maturity payment. The expres-

sion states that bond prices are dependent on the entire spectrum of short-

term interest rates r(s) during the period t < s < T. It also implies, given

Bond Pricing and Spot and Forward Rates 55

the view that the short rate evolves as a martingale process, that the term

structure at time t contains all the information available on future short

rates.

From (3.16) the discount curve, or discount function, at time t can be

derived as

TPt

→<,

. In other words, (3.16) states that the price of the

bond is the continuously compounded interest rate as applied to the zero-

coupon bond from issue date t to maturity T. The complete set of bond

prices assuming a nominal value of $1 is the discount function. Avellaneda

(2000) notes that the bond analysts usually replace the term T with a term

(T – t ), meaning time to maturity, so the function becomes

ττ

→>

,0, where τ =−

()

The relationship between the yield r (t, T ) of the zero-coupon bond

and the short rate r (t) can be expressed by equating the right-hand sides

of equations (3.16) and (3.3) (the formula for deriving the zero-coupon

bond price, repeated here as (3.17)). The result is (3.18).

PtT e

rtT T t

()

−

()

−

()

(3.17)

eEe

rTt T t

rsds

−

()

−

()

−

()

⎡

⎣

⎢

⎤

⎦

⎥

∫



(3.18)

Taking the logarithm of both sides gives (3.19).

rtT

rsds

log

()

−

⎡

⎣

⎢

⎤

⎦

⎥

−

()

∫



(3.19)

Equation (3.19) describes a bond’s yield as the average of the spot

rates that apply during the bond’s life. If the spot rate is constant, the

yield equals it.

For a zero-coupon bond, assuming that interest rates are positive,

P(t, T ) is less than or equal to 1. The yield of this bond is given by

(3.20).

rtT

PtT

log ,

()

=−

()

−

(3.20)

Rearranging (3.20) to solve for price results in (3.21).

PtT e

TtrTt

()

−−

()

−

()

(3.21)

56 Introduction to Bonds

In practice, this equation means that investors will earn r (t, T ) if they

purchase the bond at t and hold it to maturity.

Coupon Bonds

The price of coupon bonds can also be derived in terms of a risk-neutral

probability measure of the evolution of interest rates. The formula for this

derivation is (3.22).

PEe

rsds

⎛

⎝

⎜

⎞

⎠

⎟

−

()

−

()

∫∫

100



⎛⎛

⎝

⎜

⎞

⎠

⎟

∑

nt t

(3.22)

where

= the price of a coupon bond

C = the bond coupon

= the coupon payment dates, with

nN≤

, and t = 0 at the time of

valuation

w = the coupon frequency

(annual or semiannual for plain vanilla

bonds; monthly for certain ﬂ oating-rate notes and asset-backed securities),

expressed in number of times per year

T = the maturity date

Note that “100” on the right-hand side captures the fact that prices are

quoted per 100 of the bond’s principal, or nominal, value.

Expression (3.22) is written in some texts as (3.23).

Pe Ce

rN rn

−−

∫

100

(3.23)

Expression (3.22) can be simpliﬁ ed by substituting Df for the part of

the expression representing the discount factor. Assuming an annual cou-

pon, the result is (3.24).

PtT Df C Df

cNn

nt t

()

=× + ×

≥

∑

100

(3.24)

Expression (3.24) states that the market value of a risk-free bond on

any date is determined by the discount function on that date.

Bond Pricing and Spot and Forward Rates 57

Forward Rates

An investor can combine positions in bonds of differing maturities to

guarantee a rate of return that begins at a point in the future. The trade

ticket is written at time t to cover the period T to T + 1 where t < T.

The interest rate earned during this period is known as a forward rate.

The mechanism by which a forward rate is guaranteed is described below,

following Campbell et al (1997) and Jarrow (1996).

Guaranteeing a Forward Rate

Say an investor at time t simultaneously buys one unit of a zero-coupon

bond maturing at time T that is priced at P(t, T ) and sells P(t, T )/P(t, T +

1) units of zero-coupon bonds maturing at T + 1. Together these two trans-

actions generate a zero cash ﬂ ow: The investor receives a cash ﬂ ow equal to

one unit at time T and pays out P(t, T )/P (t, T + 1) at time T+ 1. These cash

ﬂ ows are identical to those that would be generated by a loan contracted at

time t for the period T to T + 1 at an interest rate of P (t, T )/P (t, T + 1).

Therefore P (t, T )/P(t, T + 1) is the forward rate. This is expressed formally

in (3.25).

ftT

PtT

()

(3.25)

Using the relationships between bond price and yield described earlier,

(3.25) can be rewritten in terms of yield as shown in (3.26), which repre-

sents the rate of return earned during the forward period (T, T + 1). This

is illustrated in

FIGURE 3.4.

FIGURE 3.4

Forward Rate Mechanics

TIME

Transactions t T T+1

Buy 1 unit of T-period bond –P (t,T ) +1

Sell P (t,T )/P (t, T+1) +[(P (t,T )/P (t, T+1)]P m (t, T+1) –P (t,T )/P (t, T+1)

T+1 period bonds

Net cash ﬂ ows 0 +1 –P (t,T )/P (t, T+1)

58 Introduction to Bonds

ftTT

PtT

rtT

()

(3.26)

Expression (3.25) can be rewritten as (3.27), which solves for bond

price in terms of the forward rates from t to T. (See Jarrow (1996), chapter

3, for a description of this derivation.)

PtT

ftk

()

−

∏

where

ftk fttftt ftT

, , , ..... ,

()

×× −

()

−

∏

(3.27)

Equation (3.27) states that the price of a zero-coupon bond is equal to

the nominal value, here assumed to be 1, receivable at time T, after it has

been discounted by the set of forward rates that apply from t to T.

Calculating a forward rate is equivalent to estimating what interest rate

will be applicable for a loan beginning at some point in the future. This

process exploits the law of one price, or no arbitrage. Consider a loan that

begins at time T and matures at T + 1. The process of calculating the rate

for that loan is similar to the one described above. Start with the simul-

taneous purchase at time t of one unit of a bond with a term of T + 1 for

price P (t, T+1) and sale of p amount of a bond with a term of T for price

P (t, T ). The net cash position at t must be zero, so p must be

PtT

()

To preclude arbitrage opportunities, the value of p amount of one

bond price divided by another must be the price of the T + 1–term bond

at time T. Therefore the forward yield for the period T to T + 1 is given

by expression (3.28).

ftT

PtT PtT

log , log ,

()

=−

()

−

(

)

()

−

(3.28)

If the period between T and the maturity of the longer-term bond is

progressively reduced, the result is an instantaneous forward rate, which is

given by formula (3.29).

Bond Pricing and Spot and Forward Rates 59

ftT

PtT, log ,

()

=−

∂

()

(3.29)

This is the price today of borrowing at time T. When T = t, the forward

rate is equal to the instantaneous short rate r (t); in other words, the spot

and forward rates for the period (t, t) are identical. For other terms, the

forward-rate yield curve will lie above the spot-rate curve if the spot curve

is positively sloping; below it, if the spot-rate curve is inverted. Campbell

et al (1997, pages 400–401) observes that this is a standard property for

marginal and average cost curves. That is, when the cost of a marginal

unit (say, of production) is above that of an average unit, the addition of a

marginal unit increases the average cost. Conversely, the average cost per

unit decreases if the marginal cost is below the average cost.

The Spot and Forward Yield Curve

From the preceding discussion of the relationships among bond prices,

spot rates, and forward rates, it is clear, given any one of these sets, that it

is possible to calculate the other two. As an illustration, consider the set of

zero-coupon rates listed in

FIGURE 3.5, which are assumed to be observed

in the market. From these ﬁ gures, the corresponding forward rates and

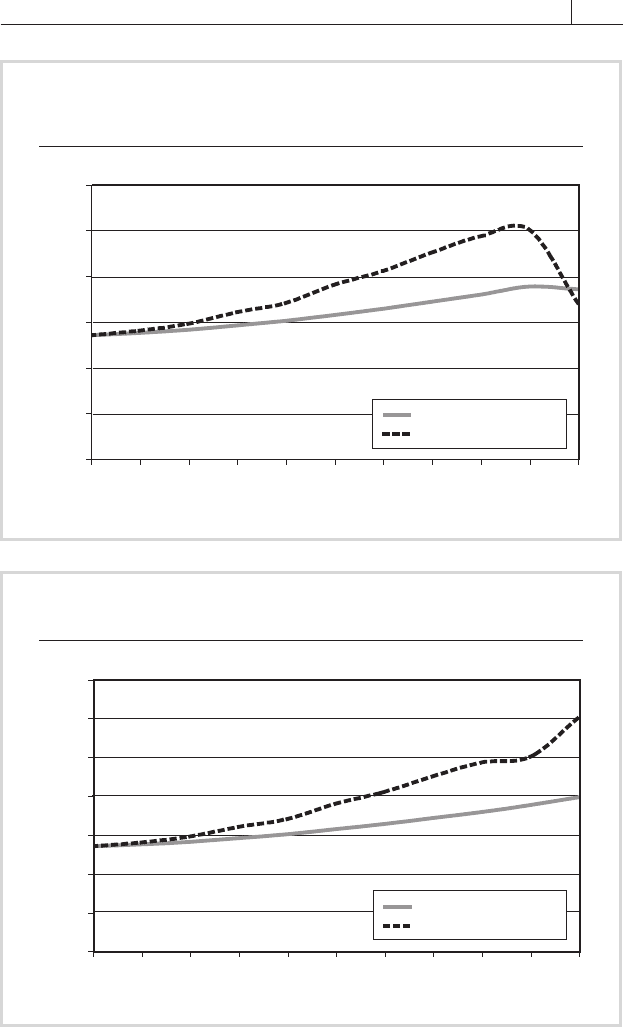
zero-coupon bond prices may be calculated.

FIGURES 3.6 and 3.7 show

the two derived curves plotted against the curve deﬁ ned by the observed

zero-coupon rates.

Note that the zero-coupon–yield curve has a positive, upward slope.

The forward-rate curve should, therefore, lie above it, as discussed earlier.

This is true until the later maturities, when the forward curve develops a

serious kink. A full explanation for why this occurs lies outside the scope

of this book. In simplest terms, though, it boils down to this: the forward

rate, or marginal rate of return, is equal to the spot rate, or average rate of

return, plus the rate of increase in the spot rate multiplied by the sum of

the increases between t and T. If the spot rate is constant (corresponding

to a ﬂ at curve), the forward-rate curve will equal it. An increasing spot-rate

curve, however, does not always generate an increasing forward curve, only

one that lies above it; it is possible for the forward curve to be increasing

or decreasing while the spot rate is increasing. If the spot rate reaches a

maximum level and then levels off or falls, the forward curve will begin

to decrease at a maturity point earlier than the spot curve high point. In

ﬁ gure 3.6 the rate of increase in the spot rate in the last period is magni-

ﬁ ed when converted to the equivalent forward rate; if the last spot rate had

been below the previous-period rate, the forward-rate curve would look

like that in ﬁ gure 3.7.

60 Introduction to Bonds

Calculating Spot Rates

It has been noted that a coupon bond may be regarded as a portfolio of

zero-coupon bonds. An implied zero-coupon interest rate structure can

therefore be derived from the yields on coupon bonds.

If the actual prices P

, P

, ….., P

of zero-coupon bonds with different

maturities and $1 nominal values are known, then the price P

of a coupon

bond of nominal value $1 and coupon C can be derived using (3.30).

PPCPC P C

=+++ +

()

1.....

(3.30)

Conversely if the coupon bond prices P

, P

,….., P

are known,

the implied zero-coupon term structure can be derived through an itera-

tive process using the relationship formalized in (3.30), as shown in (3.31)

and (3.32).

FIGURE 3.5

Hypothetical Zero-Coupon Yield and Forward Rates

TERM TO SPOT RATE r FORWARD RATE f BOND PRICE P

MATURITY (0, T ) (O, T )* (0, T )* (0, T )

0 1

1 1.054 1.054 0.94877

2 1.055 1.056 0.89845

3 1.0563 1.059 0.8484

4 1.0582 1.064 0.79737

5 1.0602 1.068 0.7466

6 1.0628 1.076 0.69386

7 1.06553 1.082 0.64128

8 1.06856 1.0901 0.58833

9 1.07168 1.0972 0.53631

10 1.07526 1.1001 0.48403

11 1.07929 1.1205 0.43198

*Interest rates are given as (1 + r )

Bond Pricing and Spot and Forward Rates 61

FIGURE 3.6

Hypothetical Zero-Coupon and Forward Yield

Curves

Term to maturity (years)

Interest rate (%)

1234567891011

Zero-coupon yield

Instantaneous forward rate

FIGURE 3.7

Hypothetical Spot and Forward Yield Curves

Term to maturity (years)

Interest rate (%)

Zero-coupon yield

Instantaneous forward rate

1 2 3 4 5 6 7 8 9 10 11