Choudhry. Fixed Income Securities Derivatives Handbook

Подождите немного. Документ загружается.

32 Introduction to Bonds

ﬂ ows generated during a bond’s life differ in value, however. The average

time to receipt would be a more accurate measure, therefore, if it were

weighted according to the cash ﬂ ows’ present values. The average maturity

of a bond’s cash ﬂ ow stream calculated in this manner provides a measure

of the speed at which a bond pays out its return, and hence of its price risk

relative to other bonds having the same maturity.

The average time until receipt of a bond’s cash ﬂ ows, weighted accord-

ing to the present values of these cash ﬂ ows, measured in years, is known

as duration or Macaulay’s duration, referring to the man who introduced

the concept in 1938—see Macaulay (1999) in References. Macaulay in-

troduced duration as an alternative for the length of time remaining before

a bond reached maturity.

Duration

Duration is a measure of price sensitivity to interest rates—that is, how

much a bond’s price changes in response to a change in interest rates. In

mathematics, change like this is often expressed in terms of differential

equations. The price-yield formula for a plain vanilla bond, introduced

in chapter 1, is repeated as (2.1) below. It assumes complete years to

maturity, annual coupon payments, and no accrued interest at the cal-

culation date.

()

.....

(2.1)

where

P = the bond’s fair price

C = the annual coupon payment

r = the discount rate, or required yield

N = the number of years to maturity, and so the number of interest

periods for a bond paying an annual coupon

M = the maturity payment

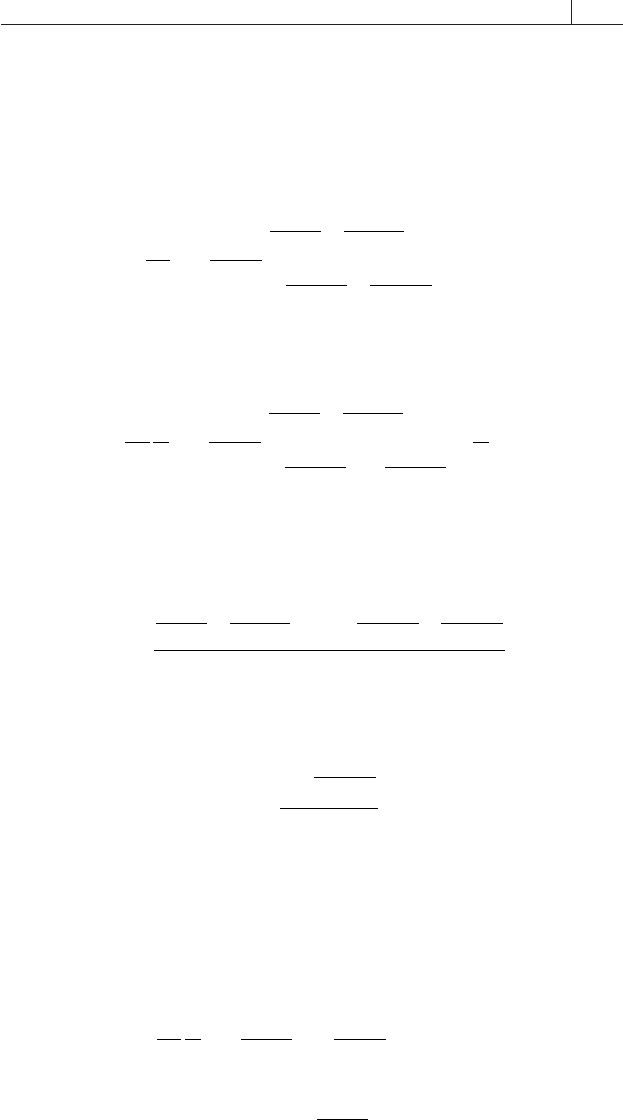
Chapter 1 showed that the price and yield of a bond are two sides of

the same relationship. Because price P is a function of yield r, we can dif-

ferentiate the price/yield equation at (2.1), as shown in (2.2). Taking the

ﬁ rst derivative of this expression gives (2.2).

−

()

−

()

−

(

)

()

−

(

)

(

111

23 1

.....

))

+n 1

(2.2)

Bond Instruments and Interest Rate Risk 33

Rearranging (2.2) gives (2.3). The expression in brackets is the aver-

age time to maturity of the cash ﬂ ows from a bond weighted according

to the present value of each cash ﬂ ow. The whole equation is the formula

for calculating the approximate change in price corresponding to a small

change in yield.

dr r

=−

()

⎡

⎣

⎢

.....

⎢⎢

⎢

⎤

⎦

⎥

(2.3)

Dividing both sides of (2.3) by P results in expression (2.4).

dr P r

=−

()

⎡

⎣

.....

⎢⎢

⎢

⎤

⎦

⎥

(2.4)

Dividing the bracketed expression by P gives expression (2.5), which is

the deﬁ nition of Macaulay duration, measured in years.

()

.....

(2.5)

Equation (2.5) can be simpliﬁ ed using

∑

, as shown in (2.6).

()

∑

(2.6)

where C

= the bond cash ﬂ ow at time n

If the expression for Macaulay duration, (2.5), is substituted into equa-

tion (2.4), which calculates the approximate percentage change in price,

(2.7) is obtained. This is the deﬁ nition of modiﬁ ed duration.

dr P r

=−

()

=−

mod

(2.7)

mod

()

(2.8)

34 Introduction to Bonds

Modiﬁ ed duration can be used to demonstrate that small changes in

yield produce inverse changes in bond price. This relationship is expressed

formally in (2.7), repeated as (2.9).

EXAMPLE: Calculating the Macaulay Duration for the 8 Percent

2009 Annual Coupon Bond

Issued 30 September 1999

Maturity 30 September 2009

Price $102.497

Yield 7.634 percent

PERIOD (N ) CASH FLOW PV AT CURRENT YIELD * N X PV

1 8 7.43260 7.4326

2 8 6.90543 13.81086

3 8 6.41566 19.24698

4 8 5.96063 23.84252

5 8 5.53787 27.68935

6 8 5.14509 30.87054

7 8 4.78017 33.46119

8 8 4.44114 35.529096

9 8 4.12615 37.13535

10 108 51.75222 517.5222

TOTAL 102.49696 746.540686

*Calculated as C/(1 + r )

Macaulay duration = 746.540686 / 102.497

= 7.283539998 years

Bond Instruments and Interest Rate Risk 35

dr P

=−

mod

(2.9)

It is possible to shorten the procedure of computing Macaulay dura-

tion longhand, by rearranging the bond-price formula (2.1) as shown in

(2.10), which, as explained in chapter 1, calculates price as the sum of the

present values of its coupons and its redemption payment. The same as-

sumptions apply as for (2.1).

−

⎡

⎣

⎢

⎤

⎦

⎥

()

(2.10)

Taking the ﬁ rst derivative of (2.10) and dividing the result by the

current bond price, P, produces an alternative formulation for modiﬁ ed

duration, shown as (2.11).

mod

−

⎡

⎣

⎢

⎤

⎦

⎥

−

()

(2.11)

Multiplying (2.11) by (1 + r) gives the equation for Macaulay duration.

The example on the following page shows how these shorthand formulas

can be used to calculate modiﬁ ed and Macaulay durations.

Up to this point the discussion has involved plain vanilla bonds. But

duration applies to all bonds, even those that have no conventional ma-

turity date, the so-called perpetual, or irredeemable, bonds (also known

as annuity bonds), which pay out interest for an indeﬁ nite period. Since

these make no redemption payment, the second term on the right side of

the duration equation disappears, and since coupon payments can stretch

on indeﬁ nitely, n approaches inﬁ nity. The result is equation (2.12), for

Macaulay duration.

(2.12)

where rc = (C/P

) is the running yield (or current yield ) of the bond

Equation (2.12) represents the limiting value to duration. For bonds

trading at or above par, duration increases with maturity, approaching

the value given by (2.12), which acts as a ceiling. For bonds trading at a

discount to par, duration increases to a maximum of around twenty years

and then declines toward the ﬂ oor given by (2.12). In general, duration

increases with maturity, with an upper bound given by (2.12).

36 Introduction to Bonds

Properties of Macaulay Duration

Duration varies with maturity, coupon, and yield. Broadly, it increases

with maturity. A bond’s duration is generally shorter than its maturity.

This is because the cash ﬂ ows received in the early years of the bond’s

life have the greatest present values and therefore are given the greatest

weight. That shortens the average time in which cash ﬂ ows are received.

A zero-coupon bond’s cash ﬂ ows are all received at redemption, so there is

no present-value weighting. Therefore, a zero-coupon bond’s duration is

equal to its term to maturity.

Duration increases as coupon and yield decrease. The lower the cou-

pon, the greater the relative weight of the cash ﬂ ows received on the ma-

turity date, and this causes duration to rise. Among the non–plain vanilla

types of bonds are some whose coupon rate varies according to an index,

usually the consumer price index. Index-linked bonds generally have

much lower coupons than vanilla bonds with similar maturities. This is

true because they are inﬂ ation-protected, causing the real yield required to

be lower than the nominal yield, but their durations tend to be higher.

EXAMPLE: Calculating the Modified and Macaulay Durations

as of 1999 of a Hypothetical Bond Having an Annual

Coupon of 8 Percent and a Maturity Date of 2009

Coupon 8 percent, paid annually

Yield 7.634 percent

n 10

Price $102.497

Plugging these values into the modiﬁ ed-duration equation

(2.11) gives

mod

()

−

()

⎡

⎣

⎢

⎤

⎦

⎥

−

()

0 07634

1 07634

10 100

0 07634

07634

102 497

()

mod

= 6.76695 years

To obtain the bond’s Macaulay duration, this modiﬁ ed duration

is multiplied by (1 + r ), or 1.07634, for a value of 7.28354 years.

Bond Instruments and Interest Rate Risk 37

Yield’s relationship to duration is a function of its role in discounting

future cash ﬂ ows. As yield increases, the present values of all future cash

ﬂ ows fall, but those of the more distant cash ﬂ ows fall relatively more. This

has the effect of increasing the relative weight of the earlier cash ﬂ ows and

hence of reducing duration.

Modified Duration

Although newcomers to the market commonly consider duration, much

as Macaulay did, a proxy for a bond’s time to maturity, this interpretation

misses the main point of duration, which is to measure price volatility, or

interest rate risk. Using the Macaulay duration can derive a measure of a

bond’s interest rate price sensitivity, i.e., how sensitive a bond’s price is to

changes in its yield. This measure is obtained by applying a mathematical

property known as a Taylor expansion to the basic equation.

The relationship between price volatility and duration can be made

clearer if the bond price equation, viewed as a function of r, is expanded

as a Taylor series (see Butler, pp. 112–114 for an accessible explanation of

Taylor expansions). Using the ﬁ rst term of this series, the relationship can

be expressed as (2.13).

∆P

D=−

()

⎡

⎣

⎢

⎤

⎦

⎥

××

Change in yield

(2.13)

where r = the yield to maturity of an annual-coupon-paying bond

As stated above, Macaulay duration equals modiﬁ ed duration multi-

plied by (1+r). The ﬁ rst two components of the right-hand side of (2.13)

taken together are therefore equivalent to modiﬁ ed duration, and equa-

tion (2.13) expresses the approximate percentage change in price as modi-

ﬁ ed duration multiplied by the change in yield.

Modiﬁ ed duration is a measure of the approximate change in bond

price for a 1 percent change in yield. The relationship between modiﬁ ed

duration and bond prices can therefore be expressed as (2.14). A negative is

used in this equation because the price movement is inverse to the interest

rate movement, so a rise in yields produces a fall in price, and vice versa.

∆∆PD rP=− ×

()

mod

(2.14)

The example on the following page illustrates how the relationships

expressed in these equations work.

Changes in yield are often expressed in terms of basis points, which

equal hundredths of a percent. For a bond with a modiﬁ ed duration of

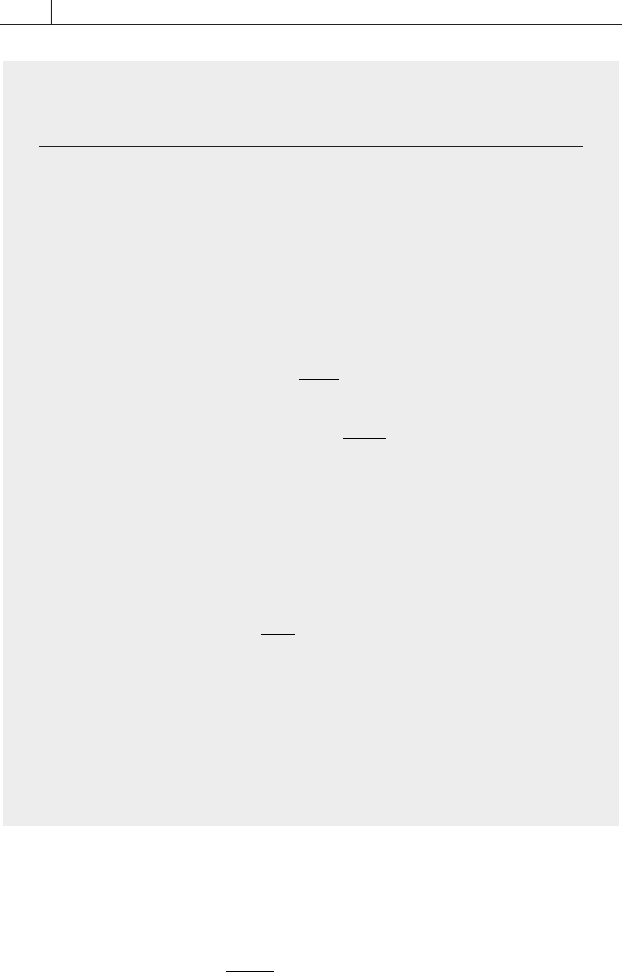
38 Introduction to Bonds

3.99, priced at par, an increase in yield of 1 basis point leads to a fall in

the bond’s price of

∆

−

⎛

⎝

⎜

⎞

⎠

⎟

()

324

100

0 0399

$. ,

+0.01 100.0

or 3.999 cents

In this example, 3.99 cents is the basis point value (BPV) of the bond:

the change in its price given a 1 basis point change in yield. The general

formula for deriving the basis point value of a bond is shown in (2.15).

EXAMPLE: Applying the Duration/Price Relationships to a

Hypothetical Bond

Coupon 8 percent, paid annually

Price par

Duration 2.74 years

If yields rise from 8 percent to 8.50 percent, the fall in the

price of the bond can be computed as follows:

∆

P=− ×

(

)

=− ×

⎛

⎝

⎜

⎞

⎠

⎟

(2.74)

0.005

1.080

100

−−$1.2685

That is, the price of the bond will fall to $98.7315.

The modiﬁ ed duration of a bond with a duration of 2.74 years

and a yield of 8 percent is

mod

.==

274

108

2 537 years

If a bond has a duration of 4.31 years and a modiﬁ ed dura-

tion of 3.99, a 1 percent move in the yield to maturity produces

a move (in the opposite direction) in the price of approximately

3.99 percent.

Bond Instruments and Interest Rate Risk 39

BPV

=×

mod

100 100

(2.15)

Basis point values are used in hedging bond positions. Hedging is done

by taking an opposite position—that is, one that will rise in value under

the same conditions that will cause the hedged position to fall, and vice

versa. Say you hold a 10-year bond. You might wish to sell short a similar

10-year bond as a hedge against your long position. Similarly, if you hold

a short position in a bond, you might hedge it by buying an equivalent

amount of a hedging instrument. A variety of hedging instruments are

available, for use both on- and off-balance sheet.

For a hedge to be effective, the price change in the primary instru-

ment should be equal to the price change in the hedging instrument. To

calculate how much of a hedging instrument is required to get this type of

protection, each bond’s BPV is used. This is important because different

bonds have different BPVs. To hedge a long position in, say, $1 million

nominal of a 30-year bond, therefore, you can’t simply sell $1 million of

EXAMPLE: Calculating Hedge Size Using Basis Point Value

Say a trader holds a long position of $1 million of the 8 per-

cent bond maturing in 2019. The bond’s modiﬁ ed duration is

11.14692, and its price is $129.87596. Its basis point value

is therefore 0.14477. The trader decides to protect the position

against a rise in interest rates by hedging it using the zero-coupon

bond maturing in 2009, which has a BPV of 0.05549. Assuming

that the yield beta is 1, what nominal value of the zero-coupon

bond must the trader sell?

The hedge ratio is

0 14477

0 05549

1 2 60894

.×=

To hedge $1 million of the 20-year bond, therefore, the trader

must sell short $2,608,940 of the zero-coupon bond. Using the

two bonds’ BPVs, the loss in the long position produced by a

1 basis point rise in yield is approximately equal to the gain in the

hedge position.

40 Introduction to Bonds

another 30-year bond. There may not be another 30-year bond with the

same BPV available. You might have to hedge with a 10-year bond. To

calculate how much nominal of the hedging bond is required, you’d use

the hedge ratio (2.16).

BPV

Change in yield for primary bond position

Change i

nn yield for hedge instrument

(2.16)

where

BPV

= the basis point value of the primary bond (the position to be

hedged)

BPV

= the basis point value of the hedging instrument

The second term in (2.16) is known as the yield beta.

FIGURE 2.1 shows how the price of the 8 percent 2009 bond changes

for a selection of yields. For a 1 basis point change in yield, the change

in price, indicated as “price duration for 1 basis point,” though not com-

pletely accurate because it is a straight line or linear approximation of

a non-linear relationship, as illustrated with ﬁ gure 1.5 of the price/yield

relationship, is a reasonable estimate of the actual change in price. For a

large move—say, 200 basis points—the approximation would be signiﬁ -

cantly off base, and analysts would accordingly not use it. This is shown

FIGURE 2.2.

Note that the price duration ﬁ gure, calculated from the modiﬁ ed dura-

tion measurement, underestimates the change in price resulting from a fall

in yields but overestimates the change from a rise in yields. This reﬂ ects

the convexity of the bond’s price-yield relationship, a concept that will be

explained in the next section.

FIGURE 2.1

The Modified Duration Approximation of

Bond Price Change at Different Yields

PRICE

DURATION

MATURITY MODIFIED OF 1 BASIS

BOND (YEARS) DURATION POINT 6.00% 6.50% 7.00%

8% 2009 10 6.76695 0.06936 114.72017 110.78325 107.02358

Bond Instruments and Interest Rate Risk 41

Convexity

Duration is a ﬁ rst-order measure of interest rate risk, using ﬁ rst-order

derivatives. If the relationship between price and yield is plotted on a

graph, it forms a curve. Duration indicates the slope of the tangent at any

point on this curve. A tangent, however, is a line and, as such, is only an

approximation of the actual curve—an approximation that becomes less

accurate the farther the bond yield moves from the original point. The

magnitude of the error, moreover, depends on the curvature, or convexity,

of the curve. This is a serious drawback, and one that applies to modiﬁ ed

as well as to Macaulay duration.

Convexity represents an attempt to remedy the drawbacks of du-

ration. A second-order measure of interest rate risk uses second-order

derivatives. It measures the curvature of the price-yield graph and the

degree to which this diverges from the straight-line estimation. Convex-

7.50% 7.99% 8.00% 8.01% 8.50% 9.00% 10.00%

103.43204 100.0671311 100.00000 99.932929 96.71933 93.58234 87.71087

FIGURE 2.2

Approximation of the Bond Price Change

Using Modified Duration

ESTIMATE USING

YIELD CHANGE PRICE CHANGE PRICE DURATION

Down 1 bp 0.06713 0.06936

Up 1 bp 0.06707 0.06936

Down 200 bp 14.72017 13.872

Up 200 bp 12.28913 13.872