Chattaraj P.K. (Ed.) Quantum Trajectories
Подождите немного. Документ загружается.

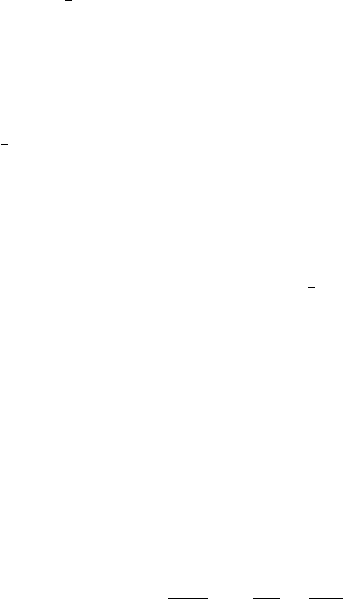
74 Quantum Trajectories
spacetime. Our purpose here is to show how the scheme may be extended to include
the derivation of the dynamics of quantum fields from a trajectory model. It turns out
that a unified description embracing both bosons and fermions may be developed by
generalizing the configuration space of the known scheme for first quantized systems
to a Riemannian manifold. We shall illustrate this for the simplest non-trivial systems
that exhibit the key algebraic features, namely, non-relativistic free spinless boson
and fermion fields. In the fluid description the two types of statistics are distinguished
by the choice of the configuration space and associated Riemannian metric, together
with the potentials to which the flow is subject. Specifically, the trajectories in the case
of bosons will be described by translational time-dependent oscillator coordinates,
and an analogous system of rotational time-dependent coordinates is employed in the
fermion case. We thus call attention to the value of considering flows in spaces other
than physical (Euclidean) space.
In extending the continuum-mechanical construction of the wave equation to
fermionic fields we shall exploit the fact that the anticommutative fermion algebra is
essentially an algebra of SU(2) angular momentum operators. It is necessary then to
include spin
1
2
in the hydrodynamical description. This problem has been addressed
previously and indeed the inclusion of spin was the initial motivation for develop-
ing the Riemannian generalization that we use [4, 5]. In presenting this method here
we generalize the previous treatment slightly to include external potentials, which
are necessary for the application to both boson and fermion fields. The key point in
the fermion case is that in the Lagrangian picture the fluid particles are treated as
spin
1
2
rotators described by sets of Euler angles. The necessity of this model arises
because the usual textbook approach to spin employing spinors is not suitable for our
purposes. We shall discuss this problem first.
5.2 SPIN AND TRAJECTORIES
An Eulerian hydrodynamic picture for spin
1
2
follows in a fairly straightforward way
for systems governed by the Pauli equation (e.g., Ref. [6] and references therein).
However, the hydrodynamic equations in this approach to spin are rather complicated
and difficult to interpret, at least in comparison with the simplicity of the spin 0
Madelung model, and there are ambiguities in identifying suitably defined phases of
the spinor components. An additional problem in connection with our constructive
program is that it is not clear how to develop a suitable Lagrangian-coordinate, or
trajectory, version of the theory. We may see this with a simple example. For a spinor
field ψ
a
(x), a = 1, 2, the associated probability density is
ρ = ψ
†
ψ (5.1)
and the flow lines are naturally defined as the integral curves of the velocity field
v
i
(x) =
2miρ
ψ
†
∂ψ
∂x
i
−
∂ψ
†
∂x
i
ψ
, i = 1, 2, 3. (5.2)
Undoubtedly, these paths provide considerable insight into spin phenomena [6]. The
problem in the present context is that the ensemble of paths generated by varying

Quantum Field Dynamics from Trajectories 75
the initial position does not contain sufficient information to construct the four real
components of the spinor field according to the method set out above and described
in [2]. To see the nature of the information that is missing, consider the following two
wavefunctions (ψ
obeys the free Pauli equation if ψ does):
ψ(x, t ) =
ψ
1
ψ
2
, ψ
(x, t ) = σ
1
ψ(x, t ) =
ψ
2
ψ
1
(5.3)
where we have used one of the Pauli matrices σ
i
, i = 1, 2, 3 (any one would do
in this example). These wavefunctions may be chosen to have non-trivially distinct
time dependences. But the implied probability density and velocity are the same
for each, given by the Equations 5.1 and 5.2. To capture the further information in
the wavefunction not exhibited by the trajectories one needs to introduce additional
hydrodynamic-like variables built from the spinor. The spin vector field s
i
(x, t ) =
(/2ρ)ψ
†
σ
i
ψ plays such a role; denoting by s
i
the spin vector associated with ψ
, the
two states (Equation 5.3) are distinguished as follows:
s
1
= s
1
, s
2
=−s
2
, s
3
=−s
3
. (5.4)
Unfortunately, while such quantities suitably extend the Eulerian hydrodynamic des-
cription, this does not assist us since the additional functions do not have Lagrangian
counterparts. That is, although one can potentially associate additional structure with
the trajectories defined by Equation 5.2 by evaluating the functions along them, the
trajectories exhibit no features that could be set in correspondence with the functions.
One can partially ameliorate the situation by seeking to redefine the velocity so that
it depends on the additional variables whilst corresponding to the same density. Just
such an additional term is, in fact, required by relativistic considerations [7] and this
leads to the following modified non-relativistic velocity:
v
i
=
2miρ
ψ
†
∂ψ
∂x
i
−
∂ψ
†
∂x
i
ψ
+
1
mρ
j,l
ε
ijl
∂
∂x
j
(ρs
l
). (5.5)
It remains the case, however, that we cannot uniquely infer from the modified trajec-
tories the full time-dependent wavefunction.
The problem is compounded for many-body systems. As the number of particles
increases, reproducing all the information in the wavefunction through additional
hydrodynamic variables requires ever more complex quantities that lack physical
interpretation and corresponding Lagrangian representatives.
It has been argued that the origin of the problems with the spin
1
2
hydrodynamic the-
ory in this context—its complexity and lack of an adequate Lagrangian formulation—
is that the standard approach to spin on which it is based works with a (angular
momentum) representation of the quantum theory in which the rotational freedoms
appear as discrete indices in the wavefunction [6]. The local fluid quantities (such
as density and velocity) are defined by summing, or “averaging,” over these indices,
and as a result essential information contained in the wavefunction is lost. The alter-
native procedure advocated in Reference [6] is to start from the angular coordinate

76 Quantum Trajectories
representation in which the spin freedoms are represented as continuous parameters
(α
1
, α
2
, α
3
) (Euler angles) in the wavefunction, on the same footing as the spatial
variables x: ψ(x, α, t). Specifically, we write
ψ(x, α, t) =
a=1,2
ψ
a
(x, t )u
a
(α), (5.6)
where
u
1
(α) = (2
√
2π)
−1
cos(α
1
/2)e
−i(α
2
+α
3
)/2
,
u
2
(α) =−i(2
√
2π)
−1
sin(α
1
/2)e
i(α
2
−α
3
)/2
,
(5.7)
are spin
1
2
basis functions with
u
∗
a
(α)u
b
(α)dΩ = δ
ab
,
dΩ = sin α
1
dα
1
dα
2
dα
3
, α
1
∈[0, π], α
2
∈[0, 2π], α
3
∈[0, 4π]. (5.8)
This implies a physically clearer and simpler hydrodynamic model. The phase S of
the wavefunction is immediately identifiable and the equations for the fluid paths
are defined in terms of the gradient of S with respect to all the coordinates, an obvi-
ous generalization of the spin 0 theory. The paths thus comprise both spatial and
angular components. The principal advantage of the angular coordinate approach for
our considerations here is that it provides a sufficiently detailed Lagrangian picture
that allows us to extend the constructive method to include the wavefunction (Equa-
tion 5.6). The enhanced detail is indicated, for example, by the fact that the velocity
field (Equation 5.2) is the mean of the new velocity over the angles:
v
i
(x, t ) = (1/ρ)
|ψ(x, α, t)|
2
1
m
∂S(x, α, t)
∂x
i
dΩ. (5.9)
Having obtained the wavefunction, the components of the time-dependent spinor field
may be extracted by using Equation 5.8 to invert Equation 5.6:
ψ
a
(x, t ) =
ψ(x, α, t)u
∗
a
(α)dΩ. (5.10)
In our application to fermions here the space variables are not relevant and we
need consider only the angular freedoms.
5.3 TRAJECTORY CONSTRUCTION OF THE WAVE EQUATION
IN A RIEMANNIAN MANIFOLD
5.3.1 L
AGRANGIAN PICTURE
We describe here the Lagrangian-coordinate hydrodynamic derivation of the wave
equation in an N-dimensional Riemannian manifold M with generalized coordinates
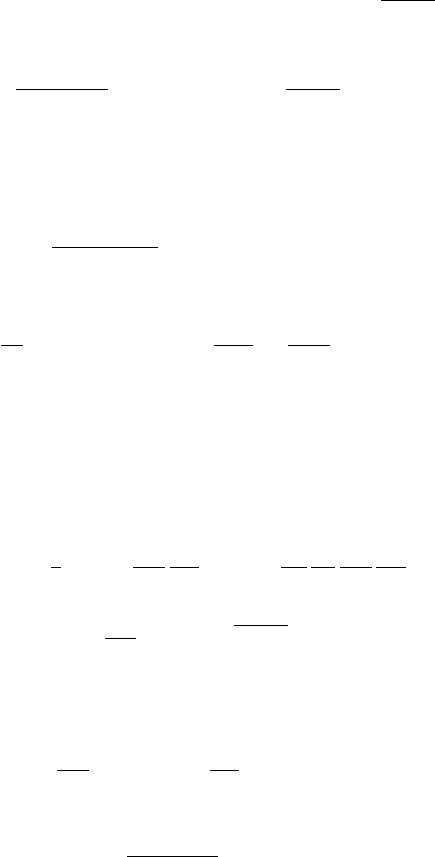
Quantum Field Dynamics from Trajectories 77
x
µ
and (static) metric g
µv
(x), µ, v, ... = 1, ...,N . In this space, the history of
the fluid is encoded in the positions ξ(ξ
0
, t) of the distinct fluid elements at time t,
each particle being distinguished by its position ξ
0
at t = 0. We assume that the
mapping between these two sets of coordinates are single-valued and differentiable
with respect to ξ
0
and t to whatever order is necessary, and that the inverse mapping
ξ
0
(ξ, t) exists and has the same properties.
Let P
0
(ξ
0
) be the initial density of some continuously distributed quantity in M
(number of particles in our application) and g = detg
µv
. Then the quantity in an
elementary volume d
N
ξ
0
attached to the point ξ
0
is given by P
0
(ξ
0
)
|g(ξ
0
)|d
N
ξ
0
.
The conservation of this quantity in the course of the motion of the fluid element is
expressed through the relation
P (ξ(ξ
0
, t))
|g(ξ(ξ
0
, t))|d
N
ξ(ξ
0
, t) = P
0
(ξ
0
)
|g(ξ
0
)|d
N
ξ
0
(5.11)
or
P (ξ
0
, t) = D
−1
(ξ
0
, t)P
0
(ξ
0
) (5.12)
where
D(ξ
0
, t) =
|g(ξ)|/|g(ξ
0
)|J (ξ
0
, t), 0 <D<∞, (5.13)
and J is the Jacobian of the transformation between the two sets of coordinates:
J =
1
N!
µ
1
,...,µ
N
v
1
,...,v
N
ε
µ
1
···µ
N
ε
v
1
···v
N
∂ξ
µ
1
∂ξ
v
1
0
···
∂ξ
µ
N
∂ξ
v
N
0
. (5.14)
In our later application where we take the limit N →∞it is assumed that the
determinant converges.
We assume that the Lagrangian for the set of fluid particles comprises a kinetic
term, an internal quantum potential energy that represents a certain kind of particle
interaction, and terms due to external scalar and vector potentials:
L =
P
0
(ξ
0
)
µ,v
1
2
mg
µv
(ξ)
∂ξ
µ
∂t
∂ξ
v
∂t
− g
µv
(ξ)
2
8m
1
P
2
∂P
∂ξ
µ
∂P
∂ξ
v
+
µ
A
µ
(ξ)
∂ξ
µ
∂t
− V (ξ)
|g(ξ
0
)|d
N
ξ
0
. (5.15)
Here P
0
, A
µ
, V, and g
µv
are prescribed functions, ξ = ξ(ξ
0
, t) and we substitute for
P from Equation 5.12 and write
∂
∂ξ
µ
= J
−1
v
J
v
µ
∂
∂ξ
v
0
(5.16)
where
J
v
µ
=
∂J
∂(∂ξ
µ
/∂ξ
v
0
)
(5.17)
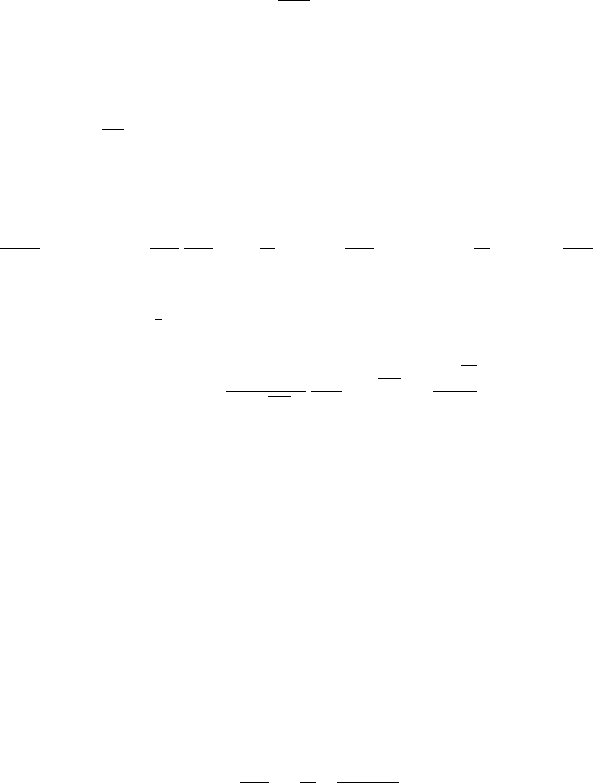
78 Quantum Trajectories
is the cofactor of ∂ξ
µ
/∂ξ
v
0
. The latter satisfies
µ
∂ξ
µ
∂ξ
v
0
J
σ
µ
= J δ
σ
v
. (5.18)
It is assumed that P
0
and its derivatives take values such that the surface terms in the
variational principle vanish. The parameter m will later be identified with the mass
of the quantum system and should not be confused with the mass of a fluid particle,
which is mP
√
|g|d
N
ξ.
Varying the coordinates, the Euler–Lagrange equations of motion for the ξ
0
th fluid
particle take the form of Newton’s second law in general coordinates:
∂
2
ξ
µ
∂t
2
+
v,σ
µ
vσ
!
∂ξ
v
∂t
∂ξ
σ
∂t
=−
1
m
v
g
µv
∂
∂ξ
v
(Q + V ) +
1
m
v
F
µv
∂ξ
v
∂t
(5.19)
where
"
µ
vσ
#
=
ρ
1
2
g
µρ
(∂g
σρ
/∂ξ
v
+ ∂g
vρ
/∂ξ
σ
− ∂g
vσ
/∂ξ
ρ
), F
µv
= ∂A
v
/∂ξ
µ
−
∂A
µ
/∂ξ
v
, and
Q =
µ,v
−
2
2m
√
|g|P
∂
∂ξ
µ
|g|g
µv
∂
√
P
∂ξ
v
(5.20)
is the quantum potential. Here we have written the force terms on the right-hand side
of Equation 5.19 in condensed form and substituting for P from Equation 5.12 and
for the derivatives with respect to ξ from Equation 5.16 we obtain a highly complex
fourth-order (in ξ
0
) local nonlinear partial differential equation. We shall see that from
the solutions ξ = ξ(ξ
0
, t), subject to specification of ∂ξ
µ
0
/∂t whose determination is
discussed next, we may derive solutions to Schrödinger’s equation.
5.3.2 I
NITIAL CONDITIONS
To obtain a flow that is representative of Schrödinger evolution we choose the initial
conditions of Equation 5.19 so that P
0
is the square of the amplitude of the initial
wavefunction and the initial covariant components of the velocity field are given by
v
g
µv
(ξ
0
)
∂ξ
v
0
∂t
=
1
m
∂S
0
(ξ
0
)
∂ξ
µ
0
− A
µ
(ξ
0
)
(5.21)
where S
0
(ξ
0
) is the phase of the initial wavefunction. To show that these assumptions
imply motion characteristic of quantum evolution we first demonstrate that the form
Equation 5.21 is preserved by the dynamical Equation 5.19. To this end, we use a
generalization of the method employed in classical hydrodynamics based on Weber’s
transformation [8].
We first multiply Equation 5.19 by
σ
g
σµ
∂ξ
σ
/∂ξ
ρ
0
and integrate between the time
limits (0, t). The term involving the Christoffel symbols {
µ
vσ
}drops out and we obtain
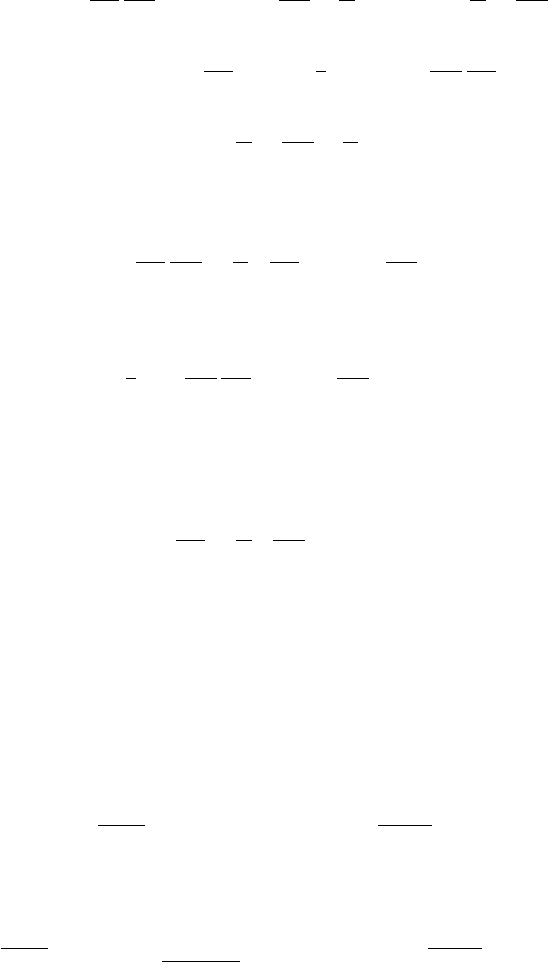
Quantum Field Dynamics from Trajectories 79
σ,µ
g
σµ
(ξ(ξ
0
, t))
∂ξ
σ
∂ξ
ρ
0
∂ξ
µ
∂t
=
µ
g
ρµ
(ξ
0
)
∂ξ
µ
0
∂t
+
1
m
A
ρ
(ξ
0
) −
µ
1
m
A
µ
∂ξ
µ
∂ξ
ρ
0
+
∂
∂ξ
ρ
0
t
0
µ,v
1
2
g
µv
(ξ(ξ
0
, t))
∂ξ
µ
∂t
∂ξ
v
∂t
+
µ
1
m
A
µ
∂ξ
µ
∂t
−
1
m
(Q + V )
dt. (5.22)
Then, substituting Equation 5.21, we get
σ,µ
g
σµ
∂ξ
σ
∂ξ
ρ
0
∂ξ
µ
∂t
=
1
m
∂S
∂ξ
ρ
0
−
µ
A
µ
∂ξ
µ
∂ξ
ρ
0
(5.23)
where
S = S
0
+
t
0
µ,v
1
2
mg
µv
∂ξ
µ
∂t
∂ξ
v
∂t
+
µ
A
µ
∂ξ
µ
∂t
− Q − V
dt. (5.24)
The left-hand side of Equation 5.23 gives the covariant velocity components at time t
with respect to the ξ
0
-coordinates. To obtain the ξ-components we multiply by J
−1
J
ρ
v
and use Equations 5.16 and 5.18 to get, at time t,
v
g
µv
∂ξ
v
∂t
=
1
m
∂S
∂ξ
µ
− A
µ
(ξ)
(5.25)
where S =S(ξ
0
(ξ, t), t). We conclude that the covariant velocity of each particle
retains forever the form Equation 5.21 if it possesses it at any moment.
5.3.3 E
ULERIAN PICTURE
We can transform the nonlinear dynamical equation into a linear one by changing
the independent variable from the particle label ξ
0
to a fixed space point x. The link
between the particle (Lagrangian) and wave-mechanical (Eulerian) pictures is defined
by the following expression for the Eulerian density:
P (x, t)
|g(x)|=
δ(x −ξ(ξ
0
, t))P
0
(ξ
0
)
|g(ξ
0
)|d
N
ξ
0
. (5.26)
The corresponding formula for the Eulerian velocity is contained in the expression
for the current:
P (x, t)
|g(x)|v
µ
(x, t ) =
∂ξ
µ
(ξ
0
, t)
∂t
δ(x −ξ(ξ
0
, t))P
0
(ξ
0
)
|g(ξ
0
)|d
N
ξ
0
. (5.27)
These relations express both the change of picture and the temporal propagation of
the system. Evaluating the integrals, Equations 5.26 and 5.27 are equivalent to the

80 Quantum Trajectories
following local expressions
P (x, t)
|g(x)|=J
−1
|
ξ
0
(x,t)
P
0
(ξ
0
(x, t ))
|g(ξ
0
(x, t ))| (5.28)
v
µ
(x, t ) =
∂ξ
µ
(ξ
0
, t)
∂t
$
$
$
$
ξ
0
(x,t)
. (5.29)
These formulas enable us to translate the Lagrangian flow equations into Eulerian
language and solve the latter using the trajectories. Differentiating Equation 5.26 with
respect to t and using Equation 5.27 we deduce the continuity equation
∂P
∂t
+
1
√
|g|
µ
∂
∂x
µ
(P
|g|v
µ
) = 0. (5.30)
Next, differentiating Equation 5.27 and using Equations 5.19 and 5.30 we get the
analog of Euler’s classical equation:
∂v
µ
∂t
+
v
v
v
∂v
µ
∂x
v
+
v,σ
µ
vσ
!
v
v
v
σ
=−
1
m
v
g
µv
∂
∂x
v
(Q + V ) +
1
m
v
F
µv
v
v
(5.31)
where Q is given by Equation 5.20 with ξ replaced by x. Finally, the Formula 5.25
for the velocity becomes
v
µ
=
1
m
v
g
µv
∂S(x, t)
∂x
v
− A
µ
(x)
. (5.32)
Formulas 5.28 and 5.29 give the general solution of the coupled continuity and Euler
Equations 5.30 and 5.31 in terms of the paths and initial wavefunction.
To establish the connection between the Eulerian equations and Schrödinger’s
equation we note that, using Equation 5.32, 5.31 implies
∂S
∂t
+
1
2m
µ,v
g
µv
∂S
∂x
µ
− A
µ
∂S
∂x
v
− A
v
+ Q + V = 0 (5.33)
where we have absorbed a function of time in S. Combining Equation 5.33 with Equa-
tion 5.30 (where we substitute Equation 5.32) we find that the function Ψ(x, t) =
√
P exp(iS/) obeys the Schrödinger equation in external scalar and vector poten-
tials in general coordinates:
i
∂Ψ
∂t
=
−
2
2m
√
|g|
µ,v
∂
∂x
µ
− (i/)A
µ
|g|g
µv
∂Ψ
∂x
v
− (i/)A
v
Ψ
+ V ψ.
(5.34)
We have thus deduced the linear wave equation from the nonlinear collective particle
motion.
The explicit construction of the time-dependent wavefunction in terms of the tra-
jectories follows straightforwardly from Equation 5.28 (which gives the amplitude)
and Equations 5.29 and 5.32. The latter give ∂S/∂x
µ
and S is fixed up to an additive
constant by Equation 5.33.

Quantum Field Dynamics from Trajectories 81
5.4 BOSONS
To make contact with the field theory for bosons we let M = R⊗···⊗R with ξ
µ
a set of
Cartesian coordinates and g
µv
= δ
µv
. We assume moreover that the coordinate index
is shorthand for three indices: µ ≡ k
i
= (k
1
, k
2
, k
3
) where k
i
=−2πn/L, ...,2πn/L,
i = 1, 2, 3, with L the side of a box of volume L
3
and N = (2n +1)
3
. We shall write
k for k
i
. Choosing A
µ
= 0 and V =
k
1
2
mω
2
k
ξ
2
k
where ω
k
= k
2
/2m, the equation
of motion 5.19 of the ξ
k0
th particle becomes
∂
2
ξ
k
∂t
2
+ ω
2
k
ξ
k
=−
1
m
∂Q
∂ξ
k
, Q =
k
−
2
2m
√
P
∂
2
√
P
∂ξ
2
k
. (5.35)
The fluid is therefore a continuum of linear oscillators coupled by quantum force terms.
The corresponding classical system is obtained when the quantum force −∂Q/∂ξ
k
may be neglected.
For notational convenience we write q in place of x in passing to the Eulerian
picture. The Schrödinger Equation 5.34 implied by Equation 5.35 is
i
∂Ψ
∂t
=
k
−
2
2m
∂
2
Ψ
∂q
2
k
+
1
2
mω
2
k
q
2
k
Ψ
. (5.36)
Writing p
k
=−i∂/∂q
k
and using the q
k
, p
k
commutation relations, the quantities
a
k
=
1
2
|k|q
k
− p
k
/i|k|
, a
†
k
=
1
2
|k|q
k
+ p
k
/i|k|
(5.37)
define a set of bosonic creation and annihilation operators:
[a
k
, a
†
k
]
−
= δ
kk
, [a
k
, a
k
]
−
=[a
†
k
, a
†
k
]
−
= 0. (5.38)
Using these operators, the Hamiltonian in Equation 5.36 becomes
H =
k
E
k
a
†
k
a
k
(5.39)
where E
k
=
2
k
2
/2m. To complete the derivation of the quantum field dynamics
we take the limit n →∞and define a complex field operator in space through the
Fourier series
ψ(x) = L
−3/2
k
a
k
e
ik.x
, k
i
= 2πn
i
/L, n
i
∈ Z, i = 1, 2, 3. (5.40)
The relations Equation 5.38 imply that the field operators obey the commutation
relations
[ψ(x), ψ
†
(x
)]
−
= δ(x − x
), [ψ(x), ψ(x
)]
−
=[ψ
†
(x), ψ
†
(x
)]
−
= 0 (5.41)
82 Quantum Trajectories
and the Hamiltonian Equation 5.39 is that of a free boson field:
H =
−
2
2m
ψ
†
(x)∇
2
ψ(x)d
3
x. (5.42)
We have thus derived the dynamics of a free quantum boson field from the trajectory
theory of a continuum of harmonic oscillators coupled by forces derived from the
quantum potential.
5.5 FERMIONS
In the case of fermions we make the following identification of the manifold sup-
porting the flow: M = SU(2) ⊗···⊗SU(2). Here the index µ = r, k
i
where
r = 1, 2, 3 and k
i
is defined as in Section 5.4. The coordinates ξ
rk
i
=
√
3/2|k|
−1
θ
r
k
i
where θ
r
k
i
= (θ
1
k
i
, θ
2
k
i
, θ
3
k
i
) are, for each k, a set of three Euler angles (for the defi-
nition see Ref. [6]), and the k-dependent coefficient is chosen in order to achieve a
particular form for the moment of inertia. The metric on M is g
µv
= g
rk
i
sk
j
with
g
rk
i
sk
j
= G
rs
(θ
k
)δ
k
i
k
j
and
G
rs
=
10 0
0 1 cos θ
1
k
0 cos θ
1
k
1
, G
rs
=
10 0
0
1
sin
2
θ
1
k
−
cos θ
1
k
sin
2
θ
1
k
0 −
cos θ
1
k
sin
2
θ
1
k
1
sin
2
θ
1
k
. (5.43)
Thus g =
+
k
det G
rs
(θ
k
) =
+
k
sin
2
θ
1
k
.
Instead of expressing the law of motion Equation 5.19 directly in terms of the
Euler angles, we can more clearly appreciate the physical model if we use the angular
velocity vector and other quantities carrying vector indices i, j , . . . with respect to
which the metric is δ
ij
. We may pass between the angle and vector descriptions using
the matrices
X
i
r
=
−cos θ
2
k
0 −sin θ
1
k
sin θ
2
k
sin θ
2
k
0 −sin θ
1
k
cos θ
2
k
0 −1 −cos θ
1
k
,
X
r
i
=
−cos θ
2
k
cot θ
1
k
sin θ
2
k
−cosecθ
1
k
sin θ
2
k
sin θ
2
k
cot θ
1
k
cos θ
2
k
−cosecθ
1
k
cos θ
2
k
0 −10
.
(5.44)
Then, for each k, the angular velocity is ω
i
k
=
r
X
i
r
∂θ
r
k
/∂t and the metrics are given
by G
rs
=
i
X
i
r
X
i
s
and δ
ij
=
r
X
r
i
X
r
j
. Writing A
rk
(θ
k
) =−
i
√
mI
k
X
i
r
B
ik
(θ
k
)
where I
k
= 3m/2k
2
we have
Quantum Field Dynamics from Trajectories 83
µ
A
µ
∂ξ
µ
∂t
=−
i,k
I
k
B
ik
ω
i
k
. (5.45)
Next, we introduce a set of angular momentum operators for each k,
ˆ
M
ik
=−i
r
X
r
i
∂/∂θ
r
k
, i = 1, 2, 3, (5.46)
so that
−
2
µ,v
g
µv
(ξ)
∂P
∂ξ
µ
∂P
∂ξ
v
=−
2
r,s,k
2k
2
3
G
rs
(θ
k
)
∂P
∂θ
r
k
∂P
∂θ
s
k
=
i,k
2k
2
3
ˆ
M
ik
P
ˆ
M
ik
P . (5.47)
Putting all this together in Equation 5.15, the Lagrangian may be written
L =
P
0
(θ
k0
)
i,k
1
2
I
k
ω
i
k
ω
i
k
+
1
8I
k
P
2
ˆ
M
ik
P
ˆ
M
ik
P −I
k
B
ik
(θ
k
)ω
i
k
− V (θ
k
)
,
k
dΩ
k0
(5.48)
where dΩ
k0
= sin θ
1
k0
dθ
1
k0
dθ
2
k0
dθ
3
k0
. This is the Lagrangian of a collection of spher-
ical rotators of moment of inertia I
k
subject to external magnetic-type fields B
ik
and
a scalar potential V . Their quantum-mechanical character is expressed through the
quadratic interaction potential and the constraints implied by the initial wavefunc-
tion. In terms of these variables the (final) Lorentz force-type term in Equation 5.19
contributes several terms including a Larmor-type torque and the equation of motion
of the θ
k0
th particle is
∂ω
i
k
∂t
=
j,l
ε
ijl
B
jk
ω
l
k
+
1
iI
k
ˆ
M
ik
(Q + V ) +
1
i
j
ω
j
k
(
ˆ
M
ik
B
jk
−
ˆ
M
jk
B
ik
). (5.49)
The fluid is therefore a continuum of spin
1
2
rotators subject to Larmor (the first
term on the right-hand side of Equation 5.49), quantum and external torques. The
corresponding classical system is obtained when the quantum torque
ˆ
M
ik
Q/i may
be neglected.
The justification for this construction is seen on passing to the Eulerian picture
where we write x
rk
i
=
√
3/2|k|
−1
α
r
k
i
. First, we note that the Schrödinger Equa-
tion 5.34 implied by Equation 5.49 describes a set of spherical rotators, in external
“magnetic” and scalar potentials, in the angular coordinate representation:
i
∂Ψ
∂t
=
i,k
1
2I
k
(
ˆ
M
ik
+ I
k
B
ik
)(
ˆ
M
ik
+ I
k
B
ik
)Ψ + V Ψ (5.50)
