Casing/Tubing design manual october 2005 Chevron
Подождите немного. Документ загружается.

()
()
()
CF
EE
F
EE
CF
EE
F
EE
CF
EE
F
EE
CC
F
rr
s
y
ts
rz
s
y
ts
rz
zz
s
y
ts
zr
rr
=−
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
−−
=−
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
−−
=−
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
−−
==−
11
4
11
2
11
4
11
2
11
4
11
2
2
1
2
2
2
2
2
2
σ
σσσ
σ
σσσ
σ
σσσ
θ
θθ θ
θ
θθ
'
'
'
,
,
,
()()
()()
EE
F
EE
CC
F
EE
F
EE
CC
F
EE
F
EE
s
y
ts
rz rz
rz zr
s
y
ts
rzzr
zz
s
y
ts
−
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
−− −−
==− −
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
−− −−
==− −
⎛
⎝
⎜
⎞
⎠
⎟
+−
⎛
⎝
⎜
⎞
⎠
⎟
1
4
11
22
2
11
4
11
22
2
11
4
11
2
2
2
σ
σσσ σσσ
σ
σσσ σσσ
σ
θθ
θθ
θθ
'
'
'
,
,
()()
22
σσσ σσσ
θθ
−− −−
rz zr
,
(D-78)
and for the incremental theory of plasticity,
()
()
()
C
F
EE
C
F
EE
C
F
EE
rr
y
t
rz
y
t
rz
zz
y
t
zr
=−
⎛
⎝
⎜
⎞
⎠
⎟
−−
=−
⎛
⎝
⎜
⎞
⎠
⎟
−−
=−
⎛
⎝
⎜
⎞
⎠
⎟
−−
4
11
2
4
11
2
4
11
2
2
2
2
2
2
2
σ
σσσ
σ
σσσ
σ
σσσ
θ
θθ θ
θ
'
'
'
,
,
,
()()
()()
()()
CC
F
EE
CC
F
EE
CC
F
EE
rr
y
t
rz rz
rz zr
y
t
rzzr
zz
y
t
rz zr
θθ θ θ
θθ
θθ θ θ
σ
σσσ σσσ
σ
σσσ σσσ
σ
σσσ σσσ
== −
⎛
⎝
⎜
⎞
⎠
⎟
−− −−
== −
⎛
⎝
⎜
⎞
⎠
⎟
−− −−
== −
⎛
⎝
⎜
⎞
⎠
⎟
−− −−
4
11
22
4
11
22
4
11
22
2
2
2
'
'
'
,
,
,
(D-79)
Notice in equation (D-77) that, because of the history or path dependence of
inelastic behavior, it is necessary to consider increments in load rather than total
load. In the expressions for the material coefficients listed in equations (D-78)
and (D-79),
′
σ
y
is the largest value of
′
σ
y
experienced up to and including the
current state of loading. The moduli
E
, , and are the previously
E
t
E
s
D-32 Casing/Tubing Design Manual
October 2005
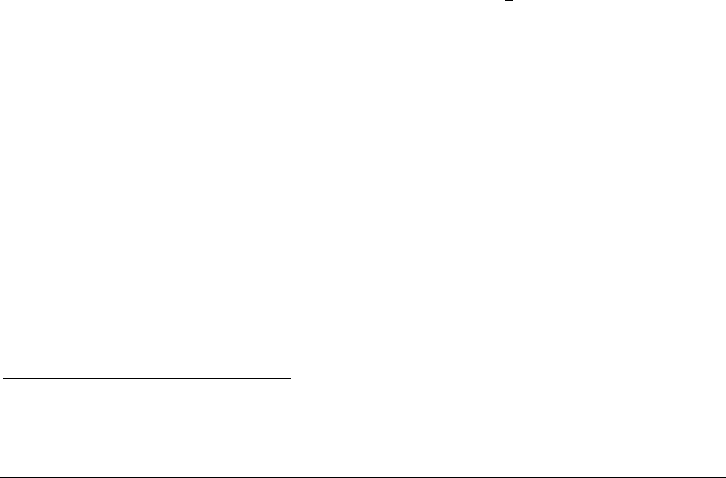
introduced measures of material stiffness as determined from a uniaxial stress-
strain curve for the material in question.
Careful examination of either equation (D-78) or (D-79) will reveal a characteristic
of the relations, the resolution of which is not immediately obvious. Notice that
these sets of equations, intended for use in a state of multi-dimensional inelastic
deformation, contain moduli that are valid only for uniaxial loads. The implication
is that the correspondence between these two stress states is of critical
significance in the solution of practical problems. In fact, this correspondence is
of such a crucial nature that we will postpone a presentation of the complete set
of elastic-plastic constitutive equations until we have resolved this interrelation.
D.5.6 The Effective Stress
Throughout the previous three sections, the complex relations for inelastic
behavior under multi-dimensional loading have been discussed by providing
analogies between simple uniaxial loading and more complex stress states (in
particular, recall Figures D-8 and D-10). In practice, the correspondence is even
more intimate.
Consider the question of the appropriate material parameters to be used in a
problem involving complex loading. For pure elastic behavior, the answer is
relatively simple. Because of the constant values of the elastic material
parameters
E
and
µ
, and the linearity of the governing equations, a simple
superposition argument can be employed to arrive at a full set of elastic
constitutive relations using only the results of a uniaxial tension test.
Unfortunately, after initial yield occurs, the material parameters are no longer
constant and the governing equations are no longer linear. However, there is still
a need to predict inelastic response using a minimum of experimental data.
Fortunately, within the confines of a reasonably accurate plasticity theory, this
need can be realized.
Let us introduce the effective stress,
σ
e
, defined by:
()
σ σσσσσσσσσ
e
= ++− − −
1
2
2
2
3
2
12 13 23
1
2
(D-80)
Comparing equation (D-80) with equations (D-73) and (D-75) we arrive at the
following alternate expressions for the yield condition,
σσσσ
yey
''
,=≥
y
(D-81)
f
ey
=−=
σσ
22
0
'
(D-82)
and the correspondence
13
summarized in Table D-4 and Figure D-11.
13
Note that for uniaxial loading, for example,
σ
σ
σ
123
00
≠
=
=
, , the effective stress
and the nonzero uniaxial stress are tantamount.
Casing/Tubing Design Manual D-33
October 2005
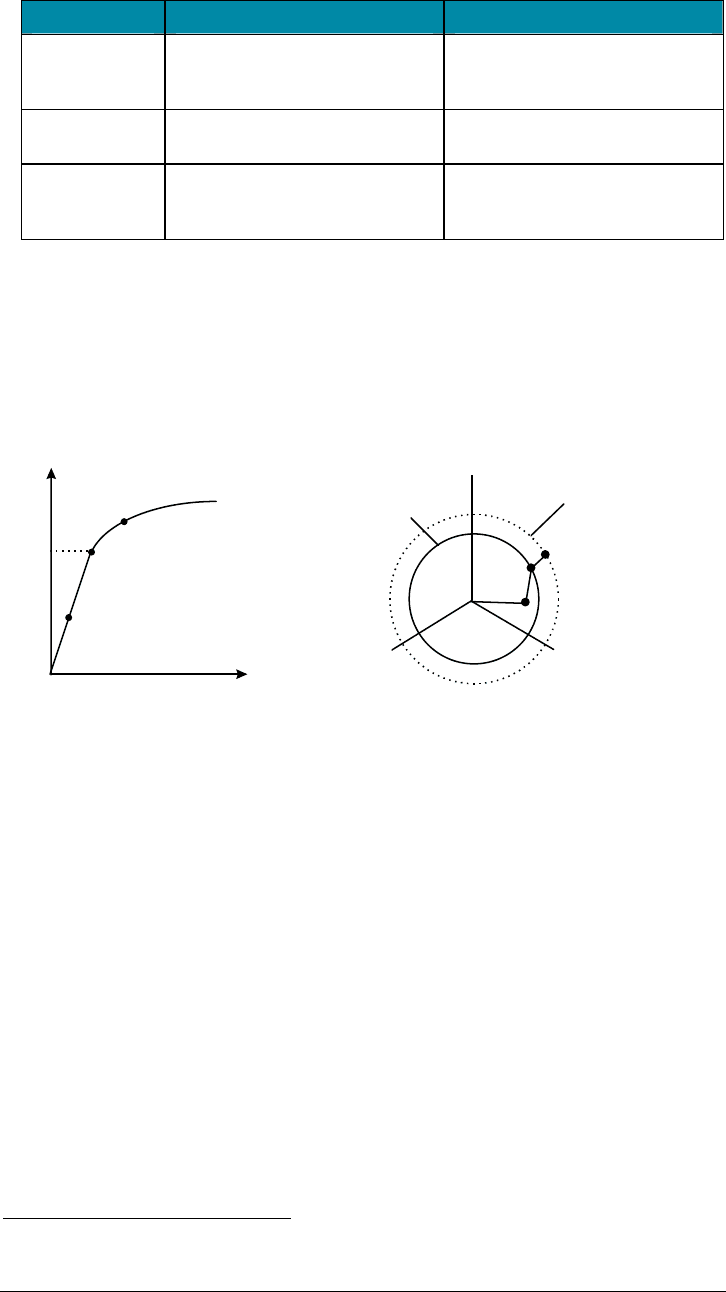
Table D-4. Correspondence for Effective Stress between Uniaxial and Multi-
Dimensional Loading
Point Uniaxial Stress Multi-Dimensional Stress
A
Stress
σ
e
is less than yield
stress
σ
y
Stress state is interior to initial
yield surface
B
Uniaxial yield,
σ
σ
ey
=
Multi-dimensional yield,
equation (D-72)
C Subsequent yield increases
yield stress above its initial
value
Subsequent yield expands initial
yield surface
More importantly, notice that for uniaxial plastic deformation, material functions
14
E
t
and are readily identified. By appropriate arguments, they may be used to
complete a description of constitutive behavior in the presence of inelastic
deformation, as indicated in equations (D-78) and (D-79). This result places us in
a position to outline the procedure for analyzing problems involving inelastic
deformation.
E
s
b. Multi-dimensional stressa. Uniaxial (effective) stress
σ
σ
y
ε
Initial Yield Surface
σ
2
= σ
θ
σ
3
= σ
z
σ
1
= σ
r
Subsequent Yield Surface
A
B
C
A
B
C
Figure D-11. Illustration of Correspondence between Effective Stress and Multi-
Dimensional Stress State at a Point
D.5.7 Elastic-Plastic Behavior
Given a prescribed load path and the uniaxial stress-strain behavior, the
following procedure may be used to determine the response of a tubular
structure:
• Determine any constraints on or simplifications to the system that may
reduce the complexity of the governing equations (examples: axisymmetric
loading, plane strain deformation).
• Increment the loads according to the prescribed load history.
• Calculate the effective stress (equation (D-80)).
14
Note the absence of an inelastic material parameter corresponding to Poisson’s ratio.
The plastic portion of the deformation produces no volume change.
D-34 Casing/Tubing Design Manual
October 2005

• Determine the elastic-plastic state (equations (D-82) and (D-76)):
o For elastic behavior or unloading,
F
=
0
, determine the strain
increment from equations (D-54) though (D-56) and (D-60) through
(D-62). The incremental elasticity equations are simply the
referenced expressions with
ε
replaced by d
ε
and
σ
replaced by
d
σ
.
o For neutral loading or loading,
F
=
1
, determine the total strain
increment from equation (D-74) with equations (D-54) through (D-56)
and (D-60) through (D-62) and either (D-78) or (D-79). In the latter
equations,
and are determined from the uniaxial stress-strain
curve and the current value of
E
t
E
s
σ
e
.
• Return to the second step, increment the load, and repeat until the total load
path is traced.
It is important to note that specific problem conditions may drastically alter the
above outline. Notwithstanding these possibilities, the outline here should
present some degree of closure to this section on constitutive relations.
D.6 Loading and Deformation of a Thick
Cylinder
Figure D-12 shows the tubular model that will be employed throughout the
remainder of this text. In the coordinate system shown, the tube is considered to
be of infinite length,
−
∞
<
<
∞
z (D-83)
finite thickness,
rrr
io
≤
≤
(D-84)
tr r
oi
=
−
(D-85)
and loaded by internal pressure,
σ
ri
patr r
i
=
−
=
(D-86)
external pressure,
σ
ro
patr r
o
=
−
=
(D-87)
and some form of axial load to be defined later.
D.6.1 Elastic, Axisymmetric Deformation
Let the cylinder described in the previous section be loaded so that all variables
are independent of the
θ
-coordinate. The strain-displacement relations for this
special deformation are given by equation (D-12). Using equation (D-12)
5
in
equations (D-60) and (D-62), one arrives at the conclusion that
σ
σ
θθ
rz
==0 .
Casing/Tubing Design Manual D-35
October 2005
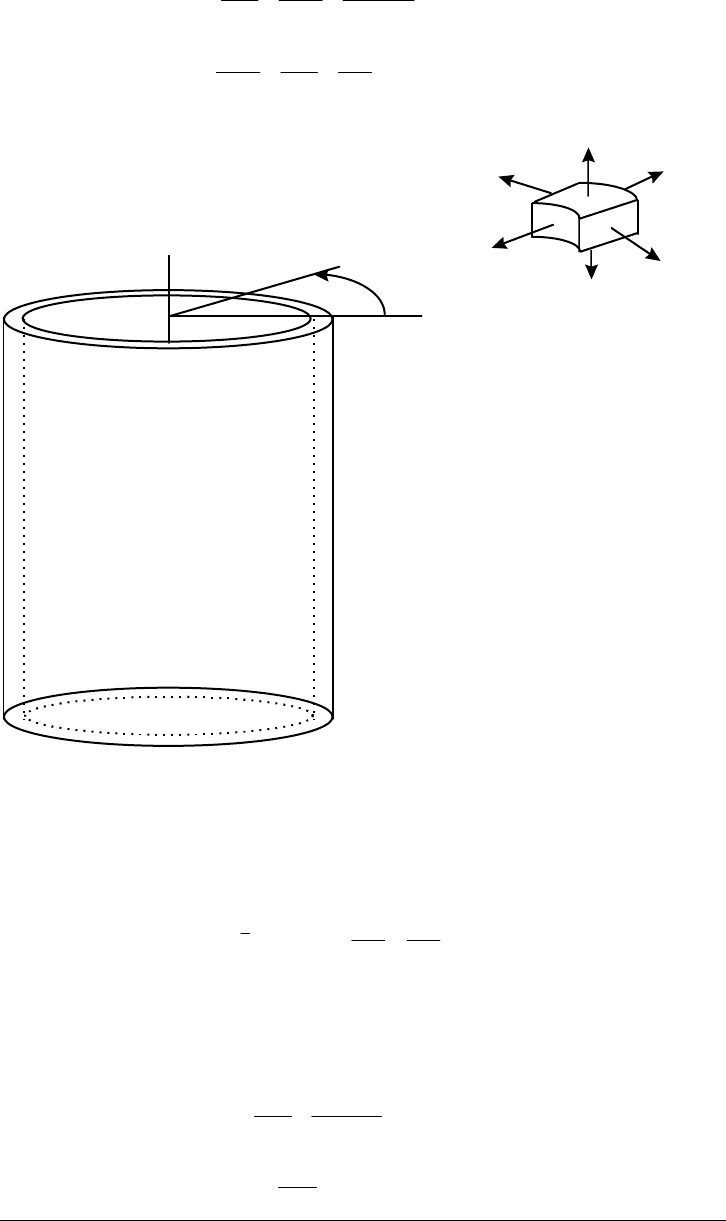
Finally, let it be assumed that the only nonzero component of body force lies
along the
-axis. Under the assumptions of this paragraph, the equations of
equilibrium (D-32) and (D-35) become:
z
∂σ
∂
∂σ
∂
σσ
θ
rzr
r
rz r
++
−
= 0
(D-88)
∂σ
∂
∂σ
∂
σ
χ
zr z zr
z
rzr
+++=0
(D-89)
z
θ
σ
θ
σ
z
σ
r
r
Figure D-12. The Thick Cylinder Model
The equation of equilibrium in the
θ
-direction, equation (D-34), vanishes
identically.
Now let the displacement of the cylinder be further restricted by the conditions:
εε
∂
∂
∂
∂
z
rz
constant
u
z
u
r
== = =,0(D-90)
which is commonly referred to as a condition of generalized plane strain. From
equations (D-90) and (D-12),
is a function of only and is a function of
only. From equation (D-61),
u
z
z u
r
r
σ
rz
=
0 , and the equations of equilibrium become
d
dr r
r
r
σ
σσ
θ
+
−
= 0 (D-91)
d
dz
z
z
σ
χ
+=0(D-92)
D-36 Casing/Tubing Design Manual
October 2005

Equation (D-91) is now an ordinary, rather than a partial differential, equation.
This may be verified by substituting the displacement field into the definitions of
strain and then calculating the stresses
σ
r
and
σ
θ
from equations (D-57) and
(D-58). Consider first the solution of equation (D-91) which can be rewritten in the
form:
()
d
dr
r
r
σσ
θ
−=0
(D-93)
Substituting equations (D-57) and D-58) into equation (D-93),
() ( )()
[]
{}
() ( )(){}
011
11
=∆+−++−−
∆+−++−
T
Tr
dr
d
zr
zr
αµεεµεµ
αµεεµεµ
θ
θ
(D-94)
Now using the strain-displacement relations from equations (D-12) and the
restrictions of equation (D-90), and simplifying the resulting expression,
r
du
dr
du
dr
u
r
rrr
2
2
0+−=
(D-95)
The solution of this equation is of the form
. Substituting this form into
equation (D-95),
ur
r
m
=
()
0
1
1
12
=−+−
−− mmm
r
r
rmrmrm
(D-96)
or
[
]
mr
m21
1−
−
0=
(D-97)
equation (D-97) is satisfied for arbitrary values of
if and only if . Thus, r
m =±1
uArBr
r
=+
−1
(D-98)
where A and B are constants. All the information necessary to compute the
nonzero components of stress and strain is now available,
ε
r
r
du
dr
A
B
r
==−
2
(D-99)
ε
θ
==+
u
r
A
B
r
r
2
(D-100)
()( )
()
()
()( )
()
σ
µµ
µµε
µ
α
µε
µµ µ
µ
α
µ
α
r
E
A
B
r
A
B
r
E
T
EA
EB
r
E
T
C
C
r
E
T
=
+−
−−
⎛
⎝
⎜
⎞
⎠
⎟+ + +
⎛
⎝
⎜
⎞
⎠
⎟
⎡
⎣
⎢
⎤
⎦
⎥
−
−
=
+
+−
−
+
−
−
=− −
−
112
1
12
112 1
1
12
12
22
2
1
2
2
∆
∆
∆
,
,
,
(D-101)
Casing/Tubing Design Manual D-37
October 2005
σ
µ
α
θ
=+ −
−
C
C
r
E
T
1
2
2
12
∆
(D-102)
σµ ε
µ
α
z
CE
E
T=+−
−
2
12
1
∆
(D-103)
Where:
()
()( )
(
)
(
)
C
EA
A
C
E
1
1
112
112
=
+
+−
=
+−
−
µε
µµ
µµ
µε
,
(D-104)
()
(
)
C
EB
B
C
E
2
2
1
1
=
+
=
+
µ
µ
,
(D-105)
Using the boundary conditions for stress given in equations (D-86) and D-87),
σ
µ
α
r
i
i
C
C
r
E
Tpatr=− −
−
=− =
1
2
2
12
∆
i
r
(D-106)
σ
µ
α
r
o
o
C
C
r
E
Tpatrr=− −
−
=− =
1
2
2
12
∆
o
(D-107)
the values of the constants C
1
and C
2
are:
C
pr p r
rr
E
T
ii oo
oi
1
22
22
12
=
−
−
+
−
µ
α
∆
(D-108)
(
)
C
pprr
rr
ioio
oi
2
22
22
=
−
−
(D-109)
Using equations (D-108) and (D-109) in equations (D-101) and (D-103),
(
)
σ
r
ii oo
oi
ioio
oi
pr p r
rr
pprr
rr r
=
−
−
−
−
−
22
22
22
22 2
1
(D-110)
(
)
σ
θ
=
−
−
+
−
−
pr p r
rr
pprr
rr r
ii oo
oi
ioio
oi
22
22
22
22 2
1
(D-111)
(
σµ εα
z
ii oo
oi
pr pr
rr
E=
−
−
+−2
22
22
∆
)
T
(D-112)
Notice in equations (D-110) and (D-111) that both
σ
r
and
σ
θ
are proportional to
1 r
2
; whereas, under the assumptions employed in this analysis, equation (D-
112) indicates that
σ
z
is constant across any cross section.
Using equations (D-104) and (D-105) to determine the constants A and B, the
radial displacement
can also now be more explicitly written as: u
r
D-38 Casing/Tubing Design Manual
October 2005

()
()
[
()
()
u
E
rr
pr pr r
pp
rr
r
rT
r
oi
ii oo
io
io
=
+
−
−−
+−
⎤
⎦
⎥
⎥
−++
1
1
12
1
22
22
22
µ
µ
µε µ α
∆
r
(D-113)
As a final step, let us return to equation (D-92), set
χ
z
w
s
=
−
, and solve for the
additional effect of gravity on
σ
z
15
. By straightforward integration of equation (D-
92):
σ
zs
wz C
=
−
+
(D-114)
where C is a constant that will depend on the location of the origin of the
axis
and the axial stress at that point. For example, at some value of
, say , let
the axial stress be
z
z z
0
σ
z0
. Using this condition in equation (D-114),
(
)
(
)
σσ σ
zszosozos
wz wz w z z=− + + = − −
o
(D-115)
D.6.2 Initial Yield, Axisymmetric Deformation
In solving the equilibrium equation (D-91) for the stress field, it was necessary to
employ the elastic constitutive equations. After any point in the cylinder yields,
the results of the previous section have to be modified. In determining the
condition for initial yield in the cross section, it is assumed that the von Mises
yield condition presents an adequate description of initial yield. Recall that (1) if
σ
σ
σ
θθ
rrzz
===0 , then
σ
r
,
σ
θ
,
σ
z
are principle stresses and may be used as
the coordinate axes in the stress space of Figure D-8b, and (2) the von Mises
criterion appears as a right circular cylinder in principle stress space governed by
equation (D-72).
Further simplification of equation (D-72) can be realized. Prior to yield, the entire
cross section of the cylinder will exhibit elastic behavior. Therefore, the external
loads resulting in yield may be determined by substituting equations (D-101) and
(D-102) into (D-72). Performing this substitution and manipulating terms, the
condition of initial yield can be written:
C
ET
C
r
C
ET
zz1
2
2
2
4
2
1
2
12
32
12
−
−
⎛
⎝
⎜
⎞
⎠
⎟
++−−
−
⎛
⎝
⎜
⎞
⎠
⎟
=
α
µ
σ
α
µ
σσ
∆∆
y
(D-116)
Notice that equation (D-116) is a function of the radius. To find the maximum
value of the left-hand side of equation (D-116), let us differentiate with respect to
the radial coordinate,
15
The -axis is assumed to be positive downward. Notice that if the cylinder is loaded
by gravity only,
z
σ
σ
θ
r
=
=
0 and gravity will have no additional effect on the radial and
circumferential stresses.
Casing/Tubing Design Manual D-39
October 2005

d
dr
C
ET
C
r
C
ET
C
r
zz1
2
2
2
4
2
1
2
2
5
12
32
12
12
0
−
−
⎛
⎝
⎜
⎞
⎠
⎟
++−−
−
⎛
⎝
⎜
⎞
⎠
⎟
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
=− <
α
µ
σ
α
µ
σ
∆∆
(D-117)
The implications from equation (D-117) are (1) because the derivative is always
negative, no local minimum exists and (2) the negative derivative implies that the
maximum value of the left-hand side of equation (D-117) will always occur at the
minimum value of
. For axisymmetric deformation, regardless of the values of
the external loads, first yield will always occur at the inner radius. Using this fact,
and the values of C
r
1
and C
2
given in equations (D-108) and (D-109), the initial
yield condition can be rewritten in the form:
(
)
()
pr p r
rr
ppr
rr
pr pr
rr
ii oo
oi
ioo
oi
z
ii oo
oi
zy
22
22
2
2
4
22
2
2
22
22
2
3
2
−
−
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
+
−
−
+
−
−
−
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
=
σ
σσ
(D-118)
which can be rewritten:
2
2
1
3
4
2
22
2
1
2
r
rr
pp p p
o
oi
io
y
zi
y
zi
y
−
−
=
+
±−
+
⎛
⎝
⎜
⎜
⎞
⎠
⎟
⎟
⎡
⎣
⎢
⎢
⎤
⎦
⎥
⎥
σ
σ
σ
σ
σ
(D-119)
1.0
-1.0
-1.0 1.0
2
2
22
r
rr
pp
o
oi
io
y
−
−
σ
σ
σ
zi
y
p+
2
Figure D-13. The Ellipse of Plasticity
Figure D-13 is a plot of equation (D-119). The ellipse in Figure D-13 is
sometimes called the “ellipse of plasticity.” It is important to realize that this
D-40 Casing/Tubing Design Manual
October 2005
ellipse is simply another form of the initial yield surface for any point on the inner
radius of the cylinder.
16
For any combination of , and
p
i
p
o
σ
z
lying interior to
the ellipse, behavior at the inner radius (and, thus, throughout the cylindrical
cross section) will be elastic. Any combination of
, and
p
i
p
o
σ
z
lying on the
ellipse indicates incipient plastic deformation at the inner radius.
D.7 Approximations for a Thin Cylinder
Instances will arise where the relations presented in the previous section lead to
expressions that are unnecessarily cumbersome. For this reason, it is instructive
to consider additional simplifications that can result under the assumption that
the tubular is a thin, rather than thick, cylinder.
Strictly speaking, the diameter-to-thickness ratio range common to oil field
tubulars (
10 30≤≤Dt ) is not large enough to permit these structures to be
classified as thin cylinders. However, for an appreciable number of problems, the
error introduced by the thin cylinder approximation is not sufficient to cause
concern. Further, the degree of error introduced by the thin cylinder
approximation is often less than the error tolerance on the magnitude of the
anticipated loads and/or the geometry of the cross section. (T.e., the thickness of
API casing can vary as much as 12.5% from its nominal value and still be
considered acceptable.) For this reason, whenever appropriate, the
simplifications introduced below will be accompanied by an estimate of the
possible error introduced.
D.7.1 Ignoring Radial Stress
A common assumption in dealing with thin tubes is that the radial stress, in
comparison with the circumferential and axial stresses, is small. Consider a
cylinder loaded only by an internal pressure,
. The maximum value of the
radial stress will be at the inner radius where
p
i
σ
r
p
i
=
−
. On the other hand, the
circumferential stress will vary between the values:
σ
σ
θ
θ
=
−
+
−
=
+
−
=
=
−
+
−
=
−
=
pr
rr
pr
rr
p
rr
rr
at r r
pr
rr
pr
rr
p
r
rr
at r r
ii
oi
io
oi
i
io
oi
i
ii
oi
ii
oi
i
i
oi
o
2
22
2
22
22
22
2
22
2
22
2
22
2
(D-120)
Re-expressing equation (D-120) in terms of the diameter: thickness ratio of the
cross section,
1
2
22
1
1
2
1
1
22
D
t
D
t
D
t
p
D
t
D
t
D
t
p
ii
⎛
⎝
⎜
⎞
⎠
⎟
−+
−
≤≤
⎛
⎝
⎜
⎞
⎠
⎟
−+
−
σ
θ
(D-121)
16
The initial yield surface appears as an ellipse, rather than a cylinder because the axes in
Figure D-13 are not in the π-plane.
Casing/Tubing Design Manual D-41
October 2005
