Casing/Tubing design manual october 2005 Chevron
Подождите немного. Документ загружается.

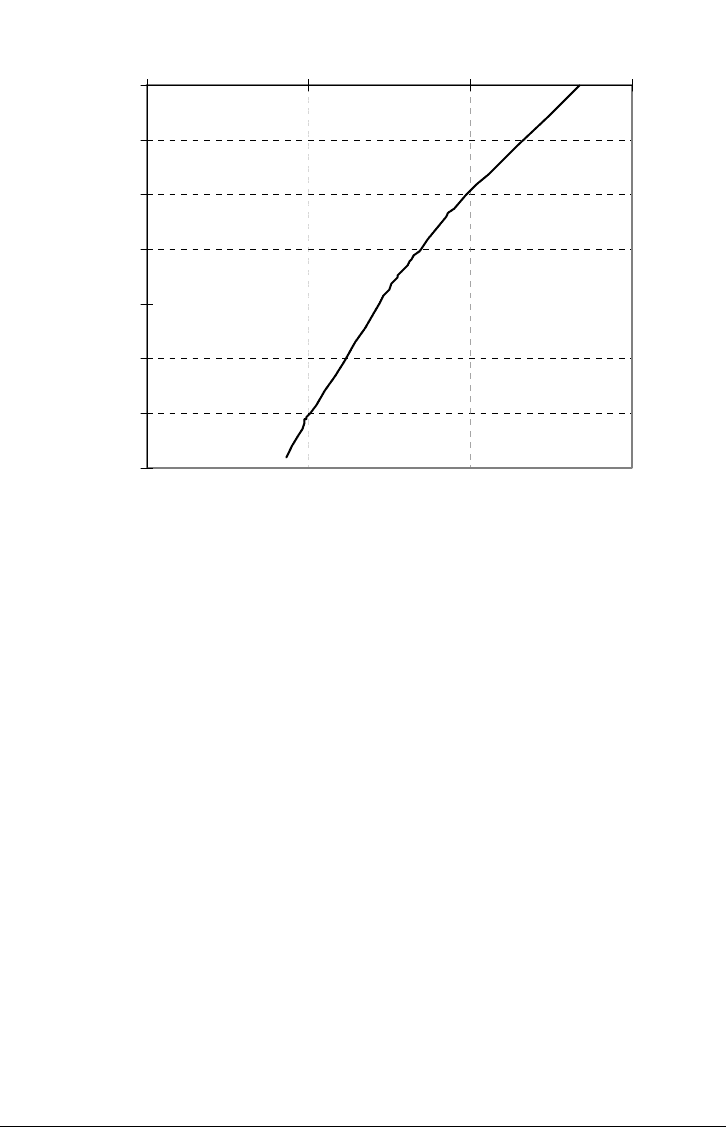
• A trial-and-error solution is, therefore, necessary because the depth to which
the 43.16-kg/m (29-ppf) N-80 is run is balanced with the tension due to
casing suspended below. This procedure is illustrated graphically in Figure
9-3. The point at which the collapse resistance of the tube just equals the
design collapse differential pressure is 2,749 m (9,020 ft).
• This trial-and-error procedure, particularly when combined with the API
procedure for adjusting collapse resistance for axial load, can be extremely
time consuming and is best performed with computer software.
6000
6500
7000
7500
8000
8500
9000
9500
0.75 1 1.25 1.5
Collapse Load/Collapse Resistance
TVD
Figure 9-3. Illustration of Trial-and-Error Solution for Crossover in a Collapse
Design
We now have a depth at which we may cross over from the 47.62 kg/m (32 ppf)
N-80 to the less expensive 43.16 kg/m (29 ppf) N-80. Repeating the procedure,
we now ask at what depth we may abandon the 43.16 kg/m (29 ppf) N-80 for a
less expensive weight/grade combination, in this case it is 38.69 kg/m (26 ppf) N-
80. This procedure proceeds uphole until the entire wellbore has been traversed.
The results are summarized in Figure 9-2.
One complication to the procedure outlined above occurs in this example. Notice
that in determining the cross over from 43.16 kg/m (29 ppf) N-80 to 38.69 kg/m
(26 ppf) K-55 we encounter the cement top, which results in a step increase in
the design collapse differential pressure. There is a short interval near the
cement top where the axial load adjusted collapse resistance of the 38.69 kg/m
(26 ppf) N-80 is inadequate (see the curve in Figure 9-2 labeled “First Pass”). To
remedy this deficiency, we must run 43.16 kg/m (29 ppf) N-80 to a point slightly
above the cement top. This point is determined in a manner identical to that
described above, the only adjustment being to use the 1.0 design factor for
determining the design differential pressure when considering the cross over
from 43.16 kg/m (29 ppf) N-80 to 38.69 kg/m (26 ppf) N-80.
Casing/Tubing Design Manual 9-23
October 2005

We now have, within the limits of our design constraints and available inventory,
the least expensive combination of weights and grades that will perform
adequately in collapse. It now remains to check other load conditions.
9.5.1.2 Burst Design
The design for internal pressure differential takes the collapse design as its
starting point. Starting with the collapse design string, a check of each section
against the design internal pressure differential is performed and, where
appropriate, amendments to the design string are made.
The preliminary design for internal pressure is less complicated than that for
external pressure as tension enhances burst resistance and may, therefore, in
the name of conservatism be ignored.
Figure 9-4 summarizes the burst design procedure. Plotting design internal
differential pressure versus depth involves two steps:
1. Given the appropriate load case, compute the appropriate internal and
external pressure profiles. For production casing, the basic load case is a
surface tubing leak. From the pore pressure distribution, the reservoir
pressure is based on a 1,761-kg/m
3
(14.7-ppg) gradient. Subtracting a
gas gradient of 2.26 kPa/m (0.1 psi/ft), the resulting surface pressure is
43.47 MPa (6,305 psi). The external pressure is set to the pore pressure
gradient.
2. Apply the design factor for this load case to the difference of the internal
and external pressure profiles to arrive at a design internal differential
pressure. In this particular design, the burst design factor is the Chevron
default of 1.2.
9-24 Casing/Tubing Design Manual
October 2005
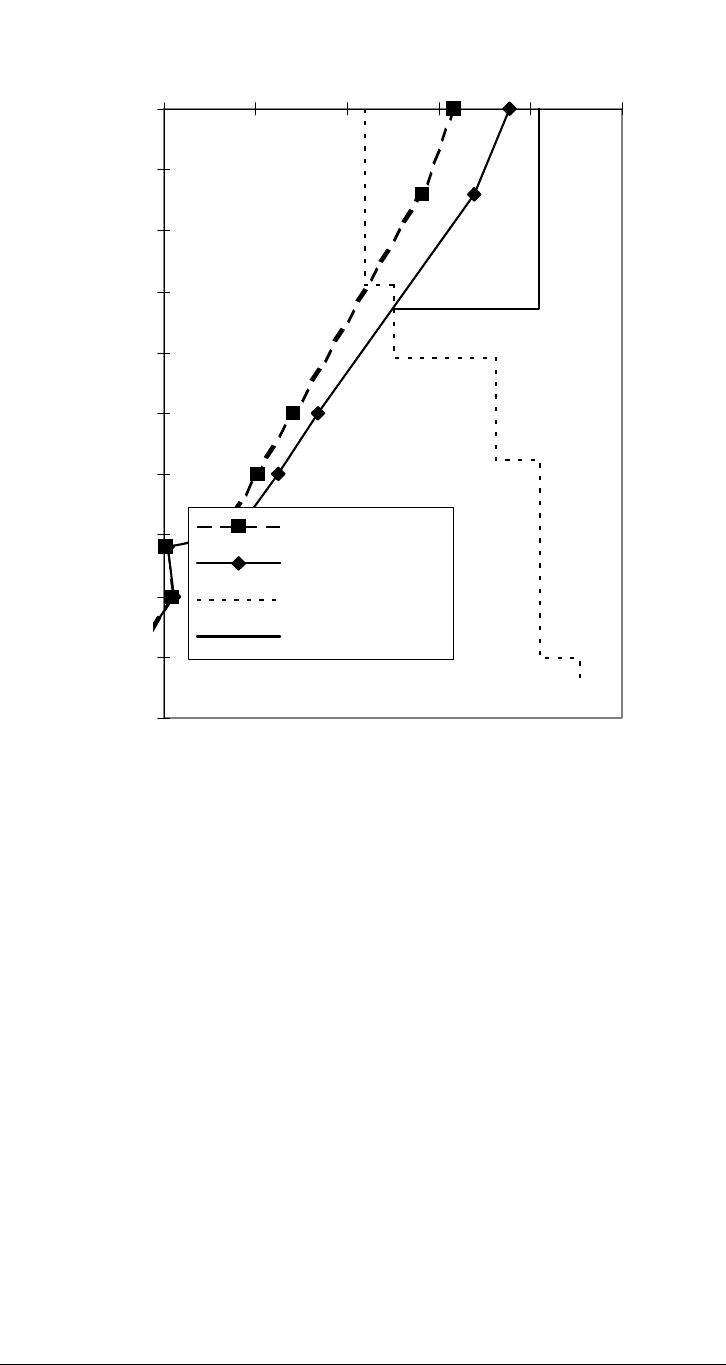
0
1000
2000
3000
4000
5000
6000
7000
8000
9000
10000
0 2000 4000 6000 8000 10000
Differential Pressure (psi)
TVD (ft)
Differential Load
Load w/DF
Collapse Design
Burst Design
29,N
26,N
26,K
23,K
32,N
29,N
Figure 9-4. Burst Design for Production Casing Example
As seen in Figure 9-4, the burst design check suggests a large portion of the
38.69-kg/m (26-ppf) K-55 and all of the 34.23-kg/m (23-ppf) K-55 must be
replaced.
In each case we replace the inadequate tube with a weight/grade combination
that has:
• A higher burst resistance
• At least as high a collapse resistance.
For both of these tubes the replacement is 43.16-kg/m (29-ppf) N-80.
Casing/Tubing Design Manual 9-25
October 2005
We now have, within the limits of our design constraints and available inventory,
the least expensive combination of weights and grades that will perform
adequately in both collapse and burst. The design string as this point is shown in
Table 9-12.
Table 9-12. Production Casing Following Burst Design
Weight kg/m(lb/ft) Grade Interval m (ft) Length m (ft)
43.16
(29)
N-80 0-1,006
(0-3,300)
1,006
(3,300)
38.69
(26)
K-55 1,006-1,241
(3,300-4,070)
235
(770)
38.69
(26)
N-80 1,241-1,756
(4,070-5,760)
515
(1,690)
43.16
(29)
N-80 1,756-2,749
(5,760-9,020)
994
(3,260)
47.62
(32)
N-80 2,749-2,896
(9,020-9,500)
146
(480)
9.5.1.3 Axial Load Design
The axial load check in this example will be simple. We will assume that a
connection as strong as the tube body is to be considered. Figure 9-5
summarizes the axial load design procedure. Plotting design axial load versus
depth involves two steps:
1. Compute the axial tension at each depth as the weight in air of casing below
that point.
2. Apply the design factor for this load case to the axial tension. In this
particular design the tension design factor is the Chevron default of 1.5.
9-26 Casing/Tubing Design Manual
October 2005
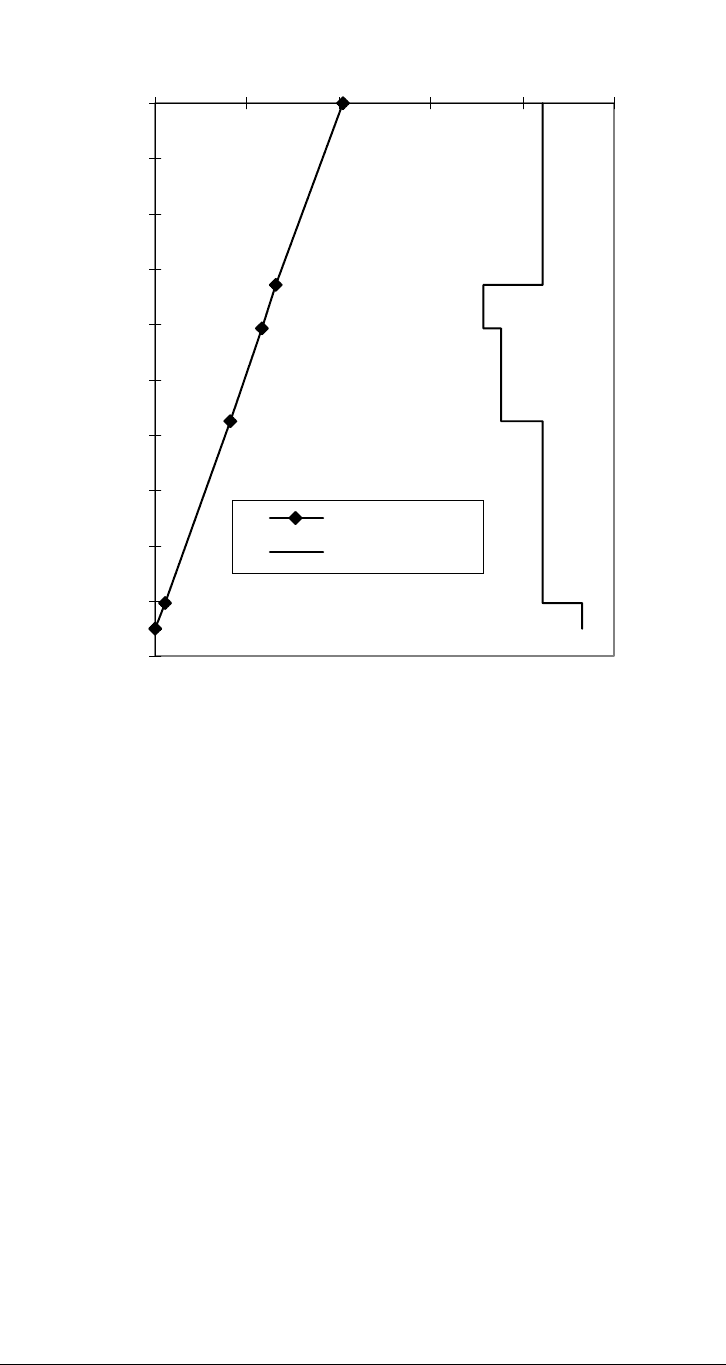
0
1000
2000
3000
4000
5000
6000
7000
8000
9000
10000
0 200000 400000 600000 800000 1000000
Axial Load (lb)
TVD (ft)
Load w/DF
Burst Design
29,N
26,N
32,N
29,N
26,K
Figure 9-5. Axial Load Design for Production Casing Example
At every depth the current design is adequate and no further changes need be
made.
9.5.1.4 Qualitative Considerations
As it now exists, the preliminary design string is adequate to meet all design
loads from the preliminary design. The string is not, however, practical. Additional
points worth considering are covered in the sections to follow. In each case,
altering the current string description will increase the cost of the design. The
decision to implement the change is based on an assessment of the risk of
operational problems should the design proceed as is.
9.5.1.5 Minimum Segment Length, Number of Segments
A design that looks good on paper may not look good sitting on the pipe rack at
the well site. A large number of weight/grade and, possibly, thread combinations
can complicate field operations. Here are some design considerations:
• Manipulating the combinations to ensure the tubes are on the rack in the
correct running order is both time consuming, particularly offshore where
space is cramped and error prone.
• Having the same weight of tube, but different grades, means tube markings
must be clear and clearly understood.
Casing/Tubing Design Manual 9-27
October 2005
• Varying the threaded connection from string section to string section
increases the number of crossovers and backups needed. For some
connection designs, crossovers are required on a change in wall thickness
even if the connection type is unchanged.
The primary danger is running the wrong weight/grade/thread combination out of
order and placing it in a section of the string for which it has inadequate strength.
With exceptional cases possible, no more than three weight/grade combinations
should be run on onshore wells, and usually only one weight/grade should be run
offshore.
One method of limiting the number of sections in a design string is to designate a
minimum segment length. In fact, such a stipulation could have been appended
to the preliminary design procedure above. In such a case, each time a new
weigh/grade/thread combination is added, some minimum length of this
combination is run before another less expensive alternative is considered.
According to the design conditions, such a procedure can significantly complicate
a hand design, but is an insignificant addition to computer software.
In the current design, even assuming the same threaded connection throughout,
we have four weight/grade combinations to be run in five distinct sections. This is
too many to be practical. A number of change options exist, but probably the
most straightforward is to replace the 38.69-kg/m (26-ppf) K-55 with 38.69-kg/m
(26-ppf) N-80. This change has minimal effect on cost, but eliminates the
possibility of confusing grades at the well site. The amended design is shown in
Table 9-13.
Table 9-13. Production Casing Following Reduction in Sections
Weight kg/m
(lb/ft)
Grade Interval m (ft) Length m (ft)
43.16
(29)
N-80 0-1,006
(0-3,300)
1,006
(3,300)
38.69
(26)
N-80 1,006-1,756
(3,300-5,760)
750
(2,460)
43.16
(29)
N-80 1,756-2,749
(5,760-9,020)
994
(3,260)
47.62
(32)
N-80 2,749-2,896
(9,020-9,500)
146
(480)
9.5.1.6 Gauge Rings
Collapse considerations often dictate that a higher wall thickness casing is run at
the bottom of the string. In this regard, it is common practice to run one or two
joints of this higher wall thickness casing out of sequence at the top of the string.
This avoids the embarrassment (and lost rig time) of running a tool entirely to
bottom before discovering that it will not pass through the higher wall thickness
tube. Although gauge rings are a good idea, they should only be run with due
consideration given the following:
• An extra crossover may be required.
• A check should be made to ensure the gauge tube is of adequate strength.
For example, the gauge tube may be of high wall thickness, but of low grade,
9-28 Casing/Tubing Design Manual
October 2005
and may actually be weaker than a higher grade tube originally intended for
the upper extremity of the wellbore.
• Care should be exercised if, below the gauge ring, plans are to run a tubing
or other hanger in a lighter tube. Hangers are often designed to work in a
range of tube inside diameters. If, however, the discrepancy between the
gauge ring and the tube at the hanger depth is sufficient, it may be that a
hanger sufficiently small to pass the gauge ring will not anchor properly in the
larger inside diameter below. One remedy is to replace the gauge ring, and
the deeply set tube it represents, with a smaller wall thickness, higher grade
tube having the same performance properties.
In the current design, the 47.62-kg/m (32-ppf) tube at the bottom of the string
suggests a gauge ring (two joints) of this tube should be run at the top of the
string.
9.5.2 Final Design Check
With a preliminary design in hand, we are now in a position, with all geometries
given, to check the design for the effect of changing environment. The procedure
is in two steps:
1. Determine the initial state of the casing string. The initial state is that state
existing immediately following WOC.
2. One-by-one, submit the casing to the changes in environment associated
with each load case. The differential pressure loads will be identical to those
considered in the preliminary design. Changes in pressure and temperature
will, however, change the axial load. This affects not only the axial design,
but also collapse and burst resistance. In addition, changes in the axial load
may initiate column buckling, which introduces bending stress, even in a
vertical wellbore.
In the final design check, burst and axial yield of the tube body are considered
simultaneously by using the von Mises yield check. The API offers no correction
to burst or internal pressure resistance for the presence of axial load. The von
Mises yield check remedies this oversight. See Table 9-14 for casing string
examples.
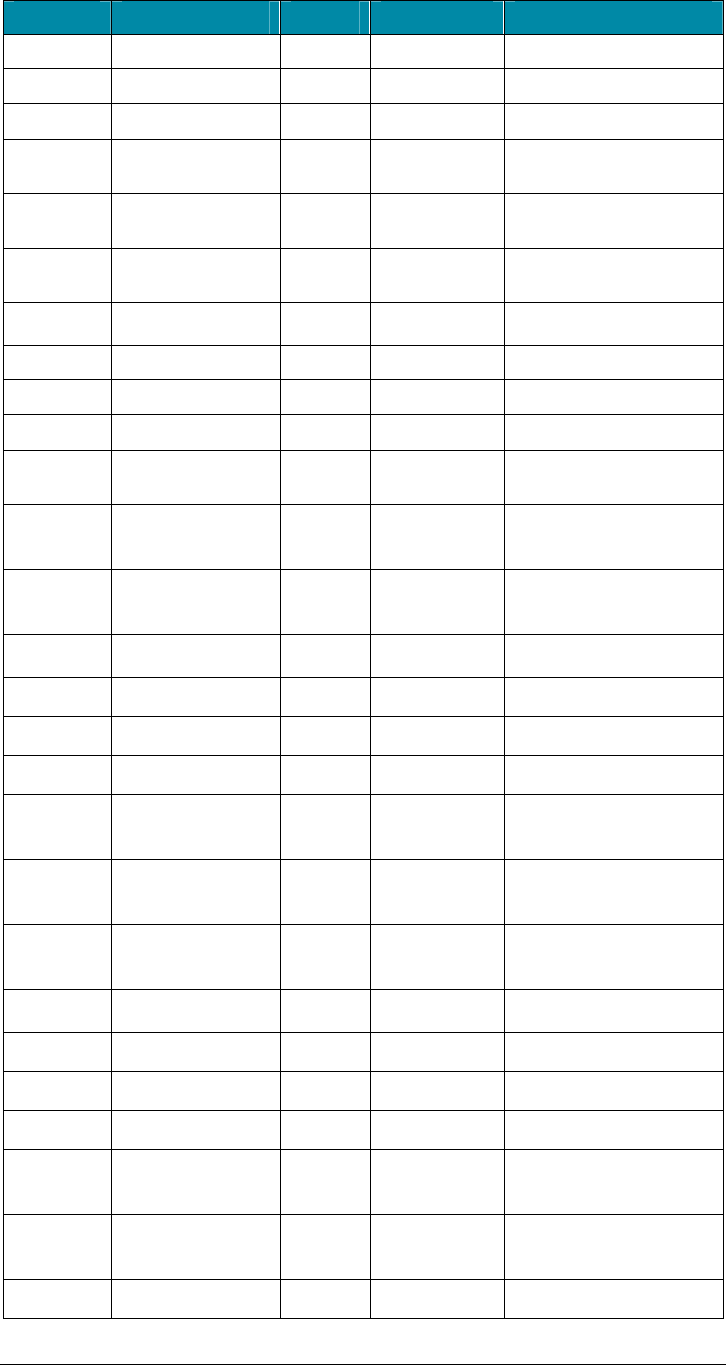
Table 9-14. Example Production Casing String (Top to Bottom)
Segment Attribute Symbol Metric English
1 Length
L
1,006 m 3,300 ft
Outside Diameter
D
177.8 mm 7 in
Wall Thickness
t
10.36 mm 0.408 in
Grade (Minimum
Yield Strength)
σ
y
551.6 MPa 80,000 psi
Weight with
Connection
w
s
43.16 kg/m 29 lb/ft
Connection Joint
Efficiency
100% 100%
Radial Clearance
r
c
19.49 mm 0.768 in
Casing/Tubing Design Manual 9-29
October 2005
Segment Attribute Symbol Metric English
2 Length
L
750 m 2,460 ft
Outside Diameter
D
177.8 mm 7 in
Wall Thickness
t
9.19 mm 0.362 in
Grade (Minimum
Yield Strength)
σ
y
551.6 Mpa 80,000 psi
Weight with
Connection
w
s
38.69 kg/m 26 lb/ft
Connection Joint
Efficiency
100% 100%
Radial Clearance
r
c
19.49 mm 0.768 in
3 Length
L
73.2 m 240 ft
Outside Diameter
D
177.8 mm 7 in
Wall Thickness
t
10.36 mm 0.408 in
Grade (Minimum
Yield Strength)
σ
y
551.6 Mpa 80,000 psi
Weight with
Connection
w
s
43.16 kg/m 29 lb/ft
Connection Joint
Efficiency
100% 100%
Radial Clearance
r
c
19.49 mm 0.768 in
4 Length
L
920 m 3,020 ft
Outside Diameter
D
177.8 mm 7 in
Wall Thickness
t
10.36 mm 0.408 in
Grade (Minimum
Yield Strength)
σ
y
551.6 Mpa 80,000 psi
Weight with
Connection
w
s
43.16 kg/m 29 lb/ft
Connection Joint
Efficiency
100% 100%
Radial Clearance
r
c
19.49 mm 0.768 in
5 Length
L
146 m 480 ft
Outside Diameter
D
177.8 mm 7 in
Wall Thickness
t
11.51 mm 0.453 in
Grade (Minimum
Yield Strength)
σ
y
551.6 Mpa 80,000 psi
Weight with
Connection
w
s
47.62 kg/m 32 lb/ft
Connection Joint 100% 100%
9-30 Casing/Tubing Design Manual
October 2005

Segment Attribute Symbol Metric English
Efficiency
Radial Clearance
r
c
19.49 mm 0.768 in
9.5.3 Cemented Casing versus Packer
Completions
The sections to follow compute the effects of changes in the temperature and
pressure environment on the integrity of the example casing string. Several
differences should be noted between these calculations and those of a packer
completion:
• The uncemented interval knows nothing of changes occurring below the
cement top. In a packer completion, pressure and fluid density changes
affect the pressure at the packer altering the axial load at the packer which is
consequently transmitted to the tubular string above the packer. In a
cemented completion, and following the setting time for the cement, it is
assumed that axial loads below the cement top are absorbed by the shear
bond between the casing and cement sheath. Therefore, loads generated
below the cement top have no effect on portions of the tubular string above
the cement top.
• The uncemented and cemented portions of a casing string must be handled
separately. In a packer completion, the entire extent of the tubing string
above the packer acts as an elastic unit, with each position in the string
experiencing the effects of the elastic response of its neighbors, even its
distant neighbors. This is also the case above the cement top in a casing
string. Below the cement top, however, each position is isolated from its
neighbors and must absorb the consequences of its inability to move axially.
Below the cement top, temperature and pressure changes have local affects
specific to the depth at which they occur.
As an example, consider a position in a tubular string that undergoes a local
temperature increase. If the string is completed with a packer or if the string is
casing above the cement top, the force generated by the tendency of the tube to
expand axially is distributed over the entire (laterally) unsupported length. If, on
the other hand, the string is cemented, the locale at which the temperature
change occurs is solely responsible for responding to the force generated by the
potential expansion.
Of course, in reality, the cement sheath is also elastic and any local effects, such
as those described above, would dissipate over a few diameters of axial length.
Such stress distributions, however, are beyond the scope of classic casing
design. In conventional design calculations, both the cement stiffness and the
shear bond between the cement and tubular are taken to be infinite in magnitude.
Cement plays a unique role in casing design calculations. The cement is
considered in the suite of initial fluids and its density is used when computing the
hydrostatic load at the bottom of the casing during cement setting. However, the
mud in which the casing is set (usually the mud displaced by the cement) or,
alternately, pore pressure is used to compute the external fluid gradient in load
Casing/Tubing Design Manual 9-31
October 2005
conditions. The assumption is that the cement solidifies sufficiently to restrain the
casing axially, but it is not competent to provide a permanent fluid seal to isolate
the casing from formation pressures (sometimes approximated by the displaced
mud).
One area of continuing controversy in cemented casing design regards the
contribution of the cement slurry density to the hydrostatic environment of the
casing during WOC. Field measurement indicates that during cement thickening
and solidification, the hydrostatic pressure within the slurry approaches the
adjacent pore pressure. This would suggest that, below the cement top, pore
pressure rather than cement slurry density should be used to compute the
hydrostatic pressure acting at the bottom of the casing string. Unfortunately, it is
not clear at what point the cement offers sufficient resistance to stop axial
movement of the casing and, therefore, set the initial state. Here, it will be
assumed that the density of the cement slurry fixes the hydrostatic load at the
bottom of the casing string and, thus, the initial axial load. Using pore pressure,
however, is acceptable as a matter of local or personal design preference.
9.5.3.1 Initial Conditions
The initial state of the casing immediately following WOC is summarized in Table
9-15.
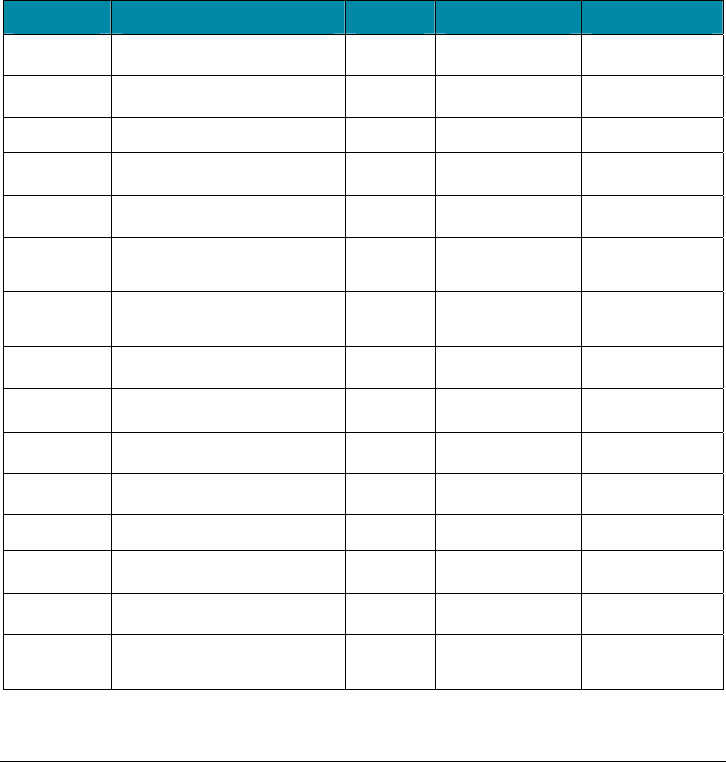
Table 9-15. Conditions Immediately Following Installation
Segment Attribute Symbol Metric English
1 Internal area
A
i
1.938x10
4
mm
2
30.04 in
2
External area
A
o
2.483x10
4
mm
2
38.48 in
2
Moment of inertia
I
1.918x10
7
mm
4
46.07 in
4
Axial force at bottom
F
z
434.01 kN 97,563 lb
Effective force at bottom
F
e
543.56 kN 122,187 lb
Internal pressure at
bottom
p
i
20.09 Mpa 2,914 psi
External pressure at
bottom
p
o
20.09 Mpa 2,914 psi
Effective weight
w
e
32.05 kg/m 21.53 lb/ft
Average temperature
T
ave
31.4°C 88.5°F
2 Internal area
A
i
1.996x10
4
mm
2
30.94 in
2
External area
A
o
2.483x10
4
mm
2
38.48 in
2
Moment of inertia
I
1.736x10
7
mm
4
41.70 in
4
Axial force at bottom
F
z
161.16 kN 36,227 lb
Effective force at bottom
F
e
331.99 kN 74,628 lb
Internal pressure at
bottom
p
i
35.07 Mpa 5,087 psi
9-32 Casing/Tubing Design Manual
October 2005
