Carlip S. Quantum Gravity in 2+1 Dimensions

Подождите немного. Документ загружается.

86 4

Geometric structures

and

Chern-Simons theory

explicitly. We find

T°[m,

= cosh ——,

[m,

= -(ma +

nb)

smh

2 2

(4.87)

The functions T° and T

clearly determine A, /i, a, and b, and can thus

be used to label points in the space Jt of classical solutions. Moreover,

it is now an easy exercise to check explicitly that the Poisson brackets

(4.84) of the holonomy variables imply the symplectic structure (3.82)

derived in chapter 3, once again confirming the consistency of our various

descriptions.

For spatial topologies of

genus

g >

no simple expression analogous to

(4.87) is known. However, Loll has recently found a complete set of inde-

pendent loop variables sufficient to parametrize the full phase space T* Jf

[178].

Loll's 6g

—

6 T° variables are written in terms of Fenchel-Nielsen

coordinates, a set of coordinates on higher-genus moduli space analogous

to the modulus

of the torus; her conjugate T

variables are expressed as

differential operators that satisfy the appropriate commutation relations.

Cambridge Books Online © Cambridge University Press, 2009

Canonical quantization in reduced phase space

Having examined the classical dynamics of (2+l)-dimensional gravity, we

are now ready to turn to the problem of quantization. As we shall see

in the next few chapters, there are a number of inequivalent approaches

to quantum gravity in 2+1 dimensions. In particular, each of the the

classical formalisms of the preceding chapters - the ADM representation,

the Chern-Simons formulation, the method of geometric structures -

suggests a corresponding quantum theory.

The world is not (2+l)-dimensional, of course, and the quantum theories

developed here cannot be taken too literally. Our goal is rather to learn

what we can about general features of quantum gravity, in the hope that

these lessons may carry over to 3+1 dimensions. Fortunately, many of the

basic conceptual issues of quantum gravity do not depend on the number

of dimensions, so we might reasonably hope that even a relatively simple

model could provide useful insights.

After a brief introduction to some of

the

conceptual issues we will face, I

will devote this chapter to a quantum theory based on the ADM represen-

tation of chapter 2. As we saw in that chapter, the ADM decomposition

and the York time-slicing make it possible to reduce (2+l)-dimensional

gravity to a system of finitely many degrees of freedom. Quantum gravity

thus becomes quantum mechanics, a subject we believe we understand

fairly well. This approach has important limitations, which are discussed

at the end of this chapter, but it is a good starting place.

5.1 Conceptual issues in quantum gravity

The assumption of a fixed spacetime background pervades ordinary quan-

tum theory. It appears in the definition of equal time commutators, in the

normalization of wave functions on spacelike surfaces, in the imposition

of causality requirements, even in the choice of fundamental observables.

88 5 Canonical quantization in reduced phase space

In general relativity, on the other hand, the universe is dynamical, and

quantum gravity requires quantization of the structure of spacetime

itself.

It should come as no surprise that attempts to formulate such a theory

quickly bring deep conceptual issues to the fore.

One serious difficulty arises as soon as an attempt is made to determine

the quantum mechanical observables. A coordinate system in general

relativity has no objective physical meaning - the theory is invariant

under diffeomorphisms - and physical observables must therefore be

independent of the choice of coordinates. As we saw in chapter 2,

the diffeomorphisms are generated by the Hamiltonian and momentum

constraints in classical general relativity. In the quantum theory, Poisson

brackets become commutators, and diffeomorphism invariance becomes

the requirement that observables commute with the constraints and that

physical states be annihilated by them. So far, however, it has proven

difficult to find any observables that meet this requirement, much less a

complete set. In particular, observables cannot be local functions of the

coordinates, since diffeomorphisms shuffle points around but must leave

observables unchanged.

A particularly strong form of this dilemma goes under the name of the

'problem of time'. (For good reviews, see references [160] and [171].) In

conventional approaches to canonical quantization, observables in quan-

tum gravity can no more depend on time than on the spatial coordinates:

the Hamiltonian constraint generates translations in coordinate time, and

must commute with observables and annihilate physical states.* Observ-

ables must therefore be time-independent - that is, they must be constants

of motion! On the other hand, general relativity is clearly a dynamical

theory, and quantum gravity must somehow describe this dynamics. One

may try to circumvent this problem by choosing a 'physical time', for in-

stance by slicing spacetime into hypersurfaces of constant mean extrinsic

curvature. But such a selection seems arbitrary, and the resulting quantum

theory is likely to depend on the choice of slicing. One may try to build

physical 'clocks' out of matter, but two different clocks will not always

agree: any clock constructed from a quantum mechanical field with a

nonnegative Hamiltonian has a finite probability of occasionally running

backwards

[261].

Alternatively, one may try to single out the Hamiltonian

constraint for special treatment, using it as an equation of motion rather

than a restriction on physical states, but this seems to violate at least the

spirit of general covariance.

Even if one could find a complete set of physical observables, it would

remain necessary to interpret their values in terms of information about

Strictly speaking, we saw in chapter 2 that the Hamiltonian constraint (2.13) generates

diffeomorphisms only on shell, creating a further difficulty in interpretation.

5.2

Quantization

the reduced phase space

geometry. In a sense, this is equivalent to completely integrating the

classical equations of

motion:

one would have to reconstruct the geometry

of spacetime from a set of diffeomorphism-invariant constants of motion.

Needless to say, this is not an easy task.

Past attempts to solve these and similar problems have given birth to

a plethora of techniques. One may start with the path integral or with

canonical quantization. In the path integral approach, one may fix the

topology of spacetime or sum over some or all topologies. In canonical

quantization, one may solve the constraints classically or impose them

as conditions on physical states. In either approach, one may choose

among a wide assortment of fundamental variables, and given a choice of

phase space variables, one may further select among 'polarizations' into

positions and momenta. In 3+1 dimensions, it is not clear that any of

these approaches works, and it is certainly not obvious which of them, if

any, are equivalent.

One long-term aim of (2+l)-dimensional gravity is to sort out some

of these conceptual issues. Beyond this, we can also explore some of the

qualitative features that might be expected in a more realistic theory of

quantum gravity. Can the topology of space change in time? What is

the effect of summing over spacetime topologies? Can gravity cut off the

ultraviolet divergence of quantum field theory? Why is the cosmological

constant so nearly zero? Do quantum effects prevent the formation of

closed timelike curves and 'time machines'?

5.2 Quantization of the reduced phase space

The starting point of any quantum theory is a classical formulation to be

'quantized'. This is perhaps unfortunate - presumably the quantum theory

should be fundamental, and the classical theory derived - but we do not

know how to proceed otherwise.

A simple starting point for quantum gravity in 2+1 dimensions is the

ADM formalism of chapter 2 in the York time-slicing. We begin by

assuming that spacetime has the (fixed) topology [0,1] x Z, where £ is

a closed surface of genus g > 0. As we saw in chapter 2, the dynamics

of the physical degrees of freedom is described by a reduced phase space

action

T I

A'T

)

&•

(

~P\ I /C

where

(5.2)

Hred

= [

Canonical quantization

reduced phase space

and X is a complicated function of m, p, and T obtained by solving

the elliptic differential equation (2.35). The positions m

parametrize the

moduli space Jf of E, and the momenta p

are cotangent vectors, so the

ADM phase space has the structure of a cotangent bundle T* Jf.

In principle, the quantization of the action (5.1) is straightforward.

We simply promote the m

and p

to operators on the Hilbert space of

square-integrable functions on Jf, with equal time commutation relations

(5.3)

The resulting quantum mechanical system then has simple states, but the

dynamics is extremely complicated. As discussed in chapter 3, for spatial

topologies of genus g >

it is not clear that equation (2.35) can be solved

for X in closed form, although there is some hope of finding the exact

solution for genus 2. But even if a solution can be found, the resulting

Hamiltonian will be an explicitly time-dependent, nonpolynomial function

of both coordinates and momenta, leading to a highly ambiguous operator

ordering.

As in the classical analysis, however, these difficulties largely disappear

if Z has the topology of a torus. We can then use the Hamiltonian of

equation (3.66) to write down a Schrodinger equation^

with

red

= (T

-4A)-

((p

)

= (T

(5.6)

Here

(5.7)

is the standard scalar Laplacian for the metric (3.67) on moduli space,

ds\ =

\dxdx.

(5.8)

t A 'Klein-Gordon' version of this equation, with T replaced by its conjugate variable V,

was found by Martinec as early as 1984

[189].

5.2 Quantization of the reduced phase space 91

This metric has constant negative curvature, and is defined on the upper

half-plane 12 > 0; we saw in chapter 3 that the classical solutions were

geodesies with respect to this geometry. Recall also that the large diffeo-

morphisms generate an additional symmetry, that of the mapping class

(or modular) group, generated by the transformations

T : t -+ r + 1, (5.9)

which leave the Laplacian (5.7) invariant. Classical observables are in-

variant under these transformations, which are, after all, merely diffeo-

morphisms; it is reasonable to assume that wave functions should also be

invariant, or at least covariant.

The Laplacian acting on such invariant functions is known to mathe-

maticians as the Maass Laplacian of weight zero, and its eigenfunctions

are weight zero Maass forms [111, 222, 226, 255]. It may be shown that

Ao is self-adjoint and has a nonnegative spectrum, so the square root in

equation (5.6) can be defined by the spectral decomposition. That is, any

modular invariant function

can be expanded in terms of eigenfunctions

of Ao,

I/>(T,T) = ^C

VVV

(T,T), (5.10)

where

the

are

weight zero Maass forms,

(T,

1/>V(T,

T),

+ 1) =

(

--) = \p

(z, z).

T T

(5.11)

We can define the action of

T,T).

(5.12)

A general time-dependent wave function - a solution of equation (5.5) -

then takes the form

T) = 2^

Uv l

(z,

T),

(5.13)

where t is the time coordinate of equation (3.68),

92 5 Canonical quantization in reduced phase space

The eigenfunctions of the Laplacian (5.7) can be found explicitly by

separation of variables. One set of eigenfunctions is

u^(x

) = T2

1/2

(2n\n\T

2nim

\ (5.15)

where K\

is a modified Bessel function. The eigenfunction u^ has

eigenvalue v

+ \, independent of n, and if n is an integer, uffi is clearly

invariant under the transformation T : x\

—•

x\ + 1. An additional set of

eigenfunctions with the same eigenvalues is

^ (5.16)

and the functions h^ are trivially T-invariant. The u^ and hf^ are not,

however, invariant under the transformation S : x

—>

—

1/T.

A weight zero

Maass form must therefore be a superposition

p7fc7.

(5.17)

Modular invariant superpositions of the form (5.17) exist for all values

of v. The continuous part of the spectrum of Ao comes from a collection

of eigenfunctions

(TI,T2) known as Eisenstein series, whose coefficients

are known explicitly. Additional eigenfunctions v

(xi

X2)

known as

cusp forms, occur for particular values of v, adding a discrete compo-

nent to the spectrum. The corresponding coefficients p

and eigenvalues

are known only numerically. The cusp forms are square integrable

with respect to the measure determined by the metric (5.8), while the

Ev(xwz2) are not. In general, however, both are needed in the expansion

of an arbitrary modular invariant function

[255]:

the Roelcke-Selberg

spectral decomposition theorem states that any modular invariant square

integrable function \p(x

x) has an expansion

5> ^JT,T)

(5.18)

where

—.f(T,T)

(T,T). (5.19)

Equation (5.18) may be understood as the proper form of the expansion

(5.10),

with both the discrete and the continuous spectra taken into

account.

The requirement of modular invariance introduces a rather peculiar

feature to the quantum theory. Since the modulus x is not itself invariant,

x is no longer, strictly speaking, an admissible observable: that is, if

I/?(T,T)

5.3

Automorphic

forms and Maass

operators

is an invariant wave function, TT/;(T,T) is not. A similar phenomenon

occurs in the quantization of a particle moving on a circle: the angle 9

is not itself periodic, and cannot be made into a self-adjoint operator.

This is not a serious problem, of course, since the trigonometric functions

cos

and sin

are perfectly good operators, which together give us exactly

the information we need from 9. Similarly, for the model of quantum

gravity developed here we can obtain complete information about

from

an appropriate set of modular invariant operators. The analogs of the

trigonometric functions are now the 'modular functions', which have been

studied extensively by mathematicians. A standard example is the modular

function J(x) of Dedekind and Klein, defined by [225]

(5.20)

J(x)

(60G

(T))

-27(140G

(T))

where the G2/C(T) are Eisenstein series,

(The prime means that the value m = n = 0 is excluded from the sum.) It

may be shown that any meromorphic modular function is a rational func-

tion of

J(T).

Such functions are certainly less familiar than trigonometric

functions, but in principle they are no more extraordinary.

5.3 Automorphic forms and Maass operators

The quantum theory described above was based on wave functions that

were invariant under the action (5.9) of the mapping class group. This

may be too strong a requirement, however: it should be sufficient to

demand that wave functions transform under a unitary representation,

so that the inner products that give transition amplitudes are invariant.

To understand the range of possible quantum theories, we must take a

short digression into the theory of representations of the torus mapping

class group. This group is also known as the modular group, and it is

represented on spaces of functions known as automorphic forms (strictly

speaking, 'automorphic forms for the modular group', or in the case of

holomorphic representations, 'modular forms'). The study of automorphic

forms is a major topic in mathematics, and we shall only touch on the

highlights here; for more detail, see, for example, reference

[255].

Recall first that the modulus

the^

torus lies in the upper half-plane

> 0. This is the Teichmiiller space Jf(T

) of the torus; more generally,

the space of constant curvature metrics jjn a surface Z modulo small dif-

feomorphisms is the Teichmiiller space ^T(Z), as discussed in appendix A.

Canonical quantization in reduced phase space

The mapping class group - the group of large diffeomorphisms - acts on

^(S),

and the quotient by this action is the moduli space JV(L).

The mapping class group of the torus is the group generated by the

transformations S and T of equation (5.9). An arbitrary element of this

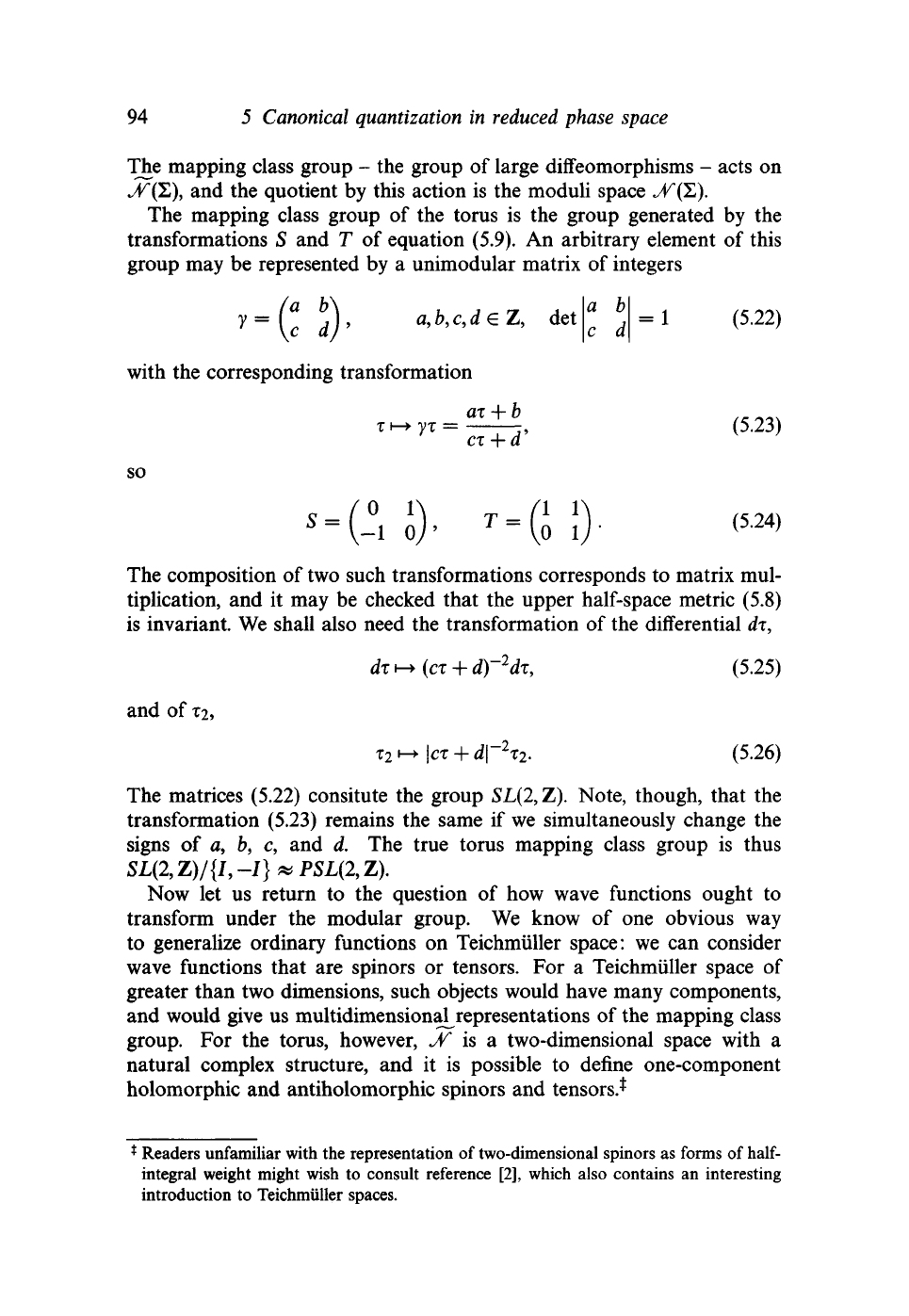
group may be represented by a unimodular matrix of integers

y =

a b

c d

b,c,d

Z, det

a b

= 1

with the corresponding transformation

S =

xi—>

yx =

0 1

-l oy

ax + b

cx + d'

T =

l l

17'

(5.22)

(5.23)

(5.24)

The composition of two such transformations corresponds to matrix mul-

tiplication, and it may be checked that the upper half-space metric (5.8)

is invariant. We shall also need the transformation of the differential dx,

I—•

(ex + d)

dx,

and of

T2,

>—•

\CX

+ d\ X2

-2

(5.25)

(5.26)

The matrices (5.22) consitute the group SL(2,Z). Note, though, that the

transformation (5.23) remains the same if we simultaneously change the

signs of a, b, c, and d. The true torus mapping class group is thus

SL(2,Z)/{/,-/}«PSL(2,Z).

Now let us return to the question of how wave functions ought to

transform under the modular group. We know of one obvious way

to generalize ordinary functions on Teichmiiller space: we can consider

wave functions that are spinors or tensors. For a Teichmiiller space of

greater than two dimensions, such objects would have many components,

and would give us multidimensional^representations of the mapping class

group. For the torus, however, Jf is a two-dimensional space with a

natural complex structure, and it is possible to define one-component

holomorphic and antiholomorphic spinors and tensors.*

Readers unfamiliar with the representation of two-dimensional spinors as forms of

half-

integral weight might wish to consult reference [2], which also contains an interesting

introduction to Teichmiiller spaces.

5.3 Automorphic forms and Maass operators 95

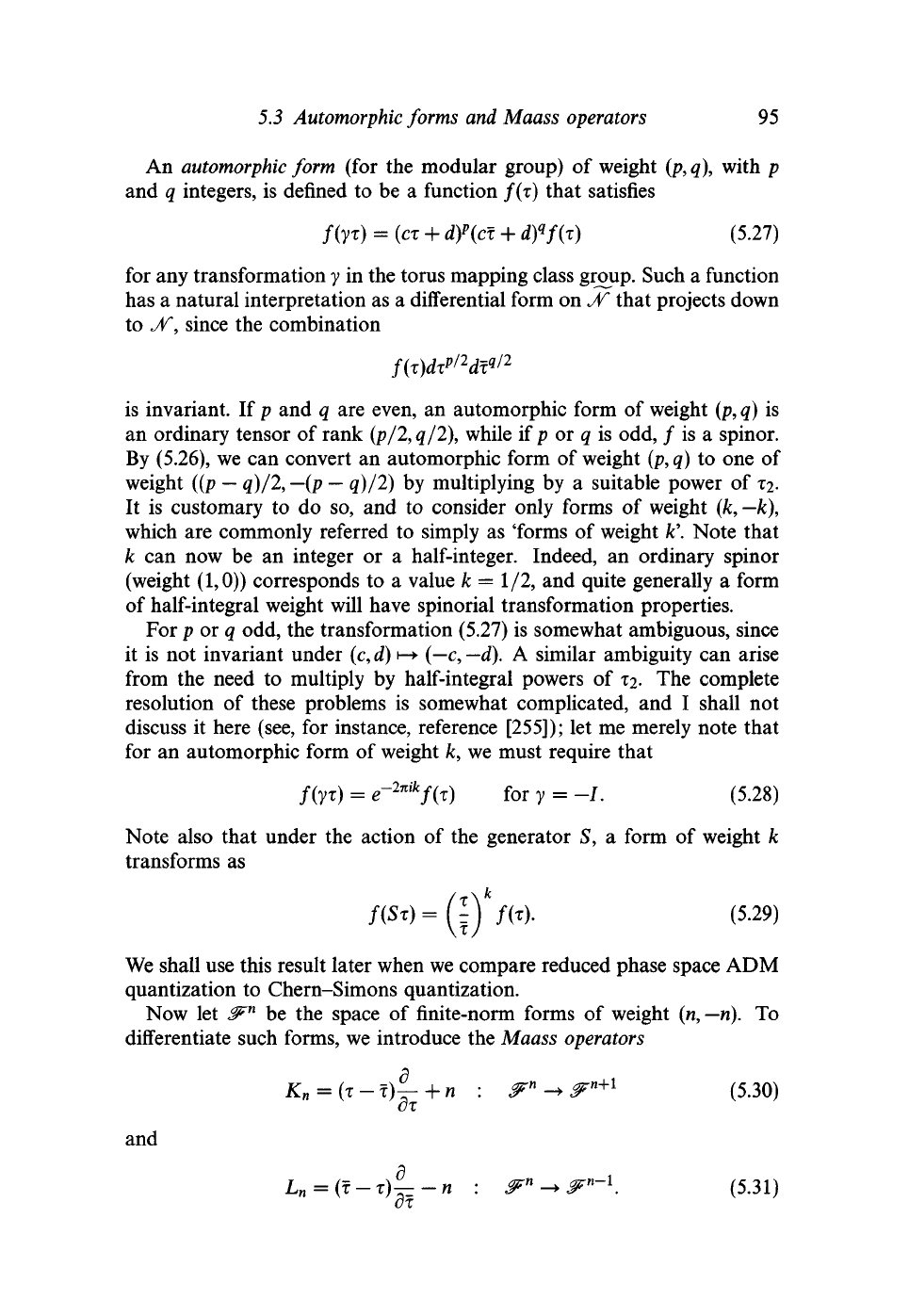
An automorphic form (for the modular group) of weight (p,g), with p

and q integers, is defined to be a function

/(T)

that satisfies

j (yx)

= (ex +

{cT

+ ay]

(T)

(5.27)

for any transformation y in the torus mapping class group. Such a function

has a natural interpretation as a differential form on Jf that projects down

to Jf, since the combination

/(T)^

is invariant. If p and q are even, an automorphic form of weight

(p,

g) is

an ordinary tensor of rank (p/2,q/2), while if p or q is odd, / is a spinor.

By (5.26), we can convert an automorphic form of weight (p,q) to one of

weight ((p

—

q)/2

— (p

—

q)/2) by multiplying by a suitable power of 12-

It is customary to do so, and to consider only forms of weight

(/c,

—

k),

which are commonly referred to simply as 'forms of weight k\ Note that

k can now be an integer or a half-integer. Indeed, an ordinary spinor

(weight (1,0)) corresponds to a value k = 1/2, and quite generally a form

of half-integral weight will have spinorial transformation properties.

For p or q odd, the transformation (5.27) is somewhat ambiguous, since

it is not invariant under

(c,

d) \->

(—c, —

d).

A similar ambiguity can arise

from the need to multiply by half-integral powers of

%2-

The complete

resolution of these problems is somewhat complicated, and I shall not

discuss it here (see, for instance, reference [255]); let me merely note that

for an automorphic form of weight fc, we must require that

) = e-

2nik

f(T) for y = -/. (5.28)

Note also that under the action of the generator S, a form of weight k

transforms as

'"^/(T).

(5.29)

We shall use this result later when we compare reduced phase space ADM

quantization to Chern-Simons quantization.

Now let J^

be the space of finite-norm forms of weight

(n,

—n).

differentiate such forms, we introduce the Maass operators

T)—

+ n : ^

-• J^

(5.30)

and

(T-r)—-n

: &

J^""

(5.31)