Cain G., Meyer G.H. Separation of Variables for Partial Differential Equations: An Eigenfunction Approach
Подождите немного. Документ загружается.


page_152
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_152.html[22/02/2009 23:52:59]
< previous page page_152 next page >
Page 152
denote the error of the approximation. Then
e(x, t)
is the solution of
(6.8)
If we multiply the differential equation by
e(x, t)
and integrate with respect to
x,
we obtain
After integrating the first term by parts the following equation results:
Example 4.20 proves that
This inequality allows the following estimate for the mean square error at time
t:
(6.9)
where for convenience we have set
Applying the algebraic-geometric mean inequality
with
we obtain the estimate
With this estimate for (6.9) we have the following error bound:
(6.10)
< previous page page_152 next page >

page_153
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_153.html[22/02/2009 23:53:00]
< previous page page_153 next page >
Page 153
E
(0)=
||w
0−
PNw0||
2
where || || is the usual norm of L2(0,
L
). Inequalities like (6.10) occur frequently in the qualitative study
of ordinary differential equations. If we express it as an equality
for some nonnegative (but unknown) function
g(t),
then this equation has the solution
or finally
(6.11)
This inequality is known as Gronwall’s inequality for (6.10). Thus the error due to projecting the initial
condition depends entirely on how well
w
0 can be approximated in and can be made as
small as desirable by taking sufficiently many eigenfunctions. In addition, this contribution to the overall
error decays with time. The approximation of the source term
G
also converges in the mean square
sense. If we can assert that
||G(x, t)−PNG(x, t)||
→0 uniformly in
t,
then we can conclude that
E(t)
→0
uniformly in
t
as
N
→∞.
As an illustration consider
It is straightforward to compute that
Using Parseval’s identity
< previous page page_153 next page >

page_154
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_154.html[22/02/2009 23:53:00]
< previous page page_154 next page >
Page 154
we obtain the estimate
where the last inequality follows from the integral test
The mean square error is
In general we cannot expect much more from our approximate solution because initial and boundary
data may not be consistent so that the Gibbs phenomenon precludes a uniform convergence of
wN
to
w.
However, as we saw in Chapter 4, when the data are smooth, then their Fourier series will converge
uniformly. In this case it is possible to establish uniform convergence with the so-called maximum
principle for the heat equation. Hence let us assume that
w
0
(x)
and
G(x, t)
are such that
and
as Here
T
is considered arbitrary but fixed. Then the error
e(x, t)
satisfies (6.8) with continuous
initial/boundary data and a smooth source term. The inequality (1.10) of Section 1.4 translates into the
following error estimate for this one-dimensional problem:
where the constant
K
depends on the length of the interval. In other words, if the orthogonal
projections converge uniformly to the data functions, then the approximate solution likewise will
converge uniformly to the true solution on the computational domain [0,
L
]×[0,
T
].
We conclude our discussion of convergence with the following characterization of
wN
.
Theorem 6.13
Let w be the analytic solution of
(6.7).
If for t
>0
the derivatives wxxand
then
PNw(x, t)=wN(x, t).
< previous page page_154 next page >

page_155
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_155.html[22/02/2009 23:53:01]
< previous page page_155 next page >
Page 155
Proof. Since
wxx (x, t)−wt(x, t)=G(x, t)
w
(0,
t
)=
w(L, t)
=0
w
(
x,
0)=
w
0
(x),
we see that
PN(wxx −wt)=PNG
PNw
(
x,
0)=
PNw
0
(x).
Writing out the projections we obtain for each
n
Integration by parts shows that
and of course
Hence the term
satisfies the initial value problem for
αn
. Since its solution is unique, it follows
that and hence that
wN(x, t)=PNw(x, t).
Theorem 6.13 states that the computed
wN
for any
t
is the orthogonal projection of the unknown exact
solution
w
onto the span of the first eigenfunctions in other words,
wN
is the best possible
approximation to
w
in span
Conditions on the data for the existence of wxx and discussed, for example, in [5]. In
particular, it is necessary that the consistency conditions
w
0(0)=
w
0
(L)
=0 hold. This is a severe
restriction on the data and not satisfied by many of our examples.
6.3 Influence of the boundary conditions and Duhamel’s solution
The formulas derived for the solution of (6.6) involve the function
v
used to zero out the boundary
conditions. Since there are many
v
which may be used, and since the analytic solution is uniquely
determined by the boundary data
< previous page page_155 next page >

page_156
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_156.html[22/02/2009 23:53:02]
< previous page page_156 next page >
Page 156
and is independent of
v,
it may be instructive to see how
v
actually enters the computational solution.
We recall the original problem is transformed into
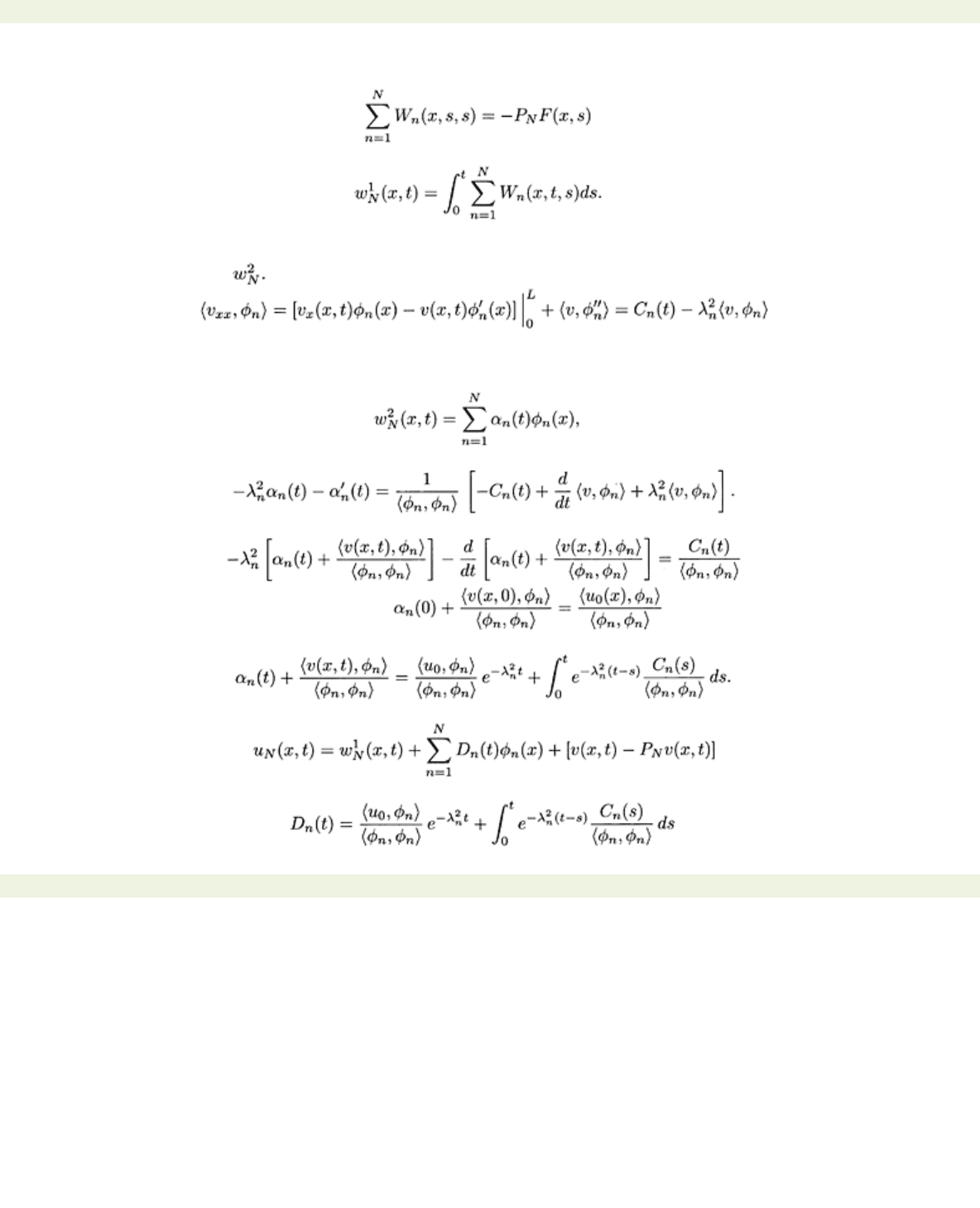
Let us write the approximate solution
wN(x, t)
in the form
where
and where
accounts for the influence of the initial and boundary conditions
The above discussion of the Dirichlet problem shows that
where
We remark that is identical to the solution obtained with Duhamel’s principle since by inspection
the function
solves the problem
< previous page page_156 next page >
page_157
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_157.html[22/02/2009 23:53:03]
< previous page page_157 next page >
Page 157
so that
and
In other words, the eigenfunction expansion is identical to the solution obtained with Duhamel’s
superposition principle.
We shall now compute
Integration by parts shows that
where for the data of (6.6)
Cn(t)
=λ
n[A(t)−B(t) cos
λ
nL
].
Cn(t)
is independent of the choice of
v
since only the boundary data appear. If we write
then
It follows that
so that
Thus
where
< previous page page_157 next page >

page_158
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_158.html[22/02/2009 23:53:04]
< previous page page_158 next page >
Page 158
is again independent of the choice of
v.
Hence the solution
uN(x, t)
at time
t
depends on the data of the original problem and on the difference
between
v
and its orthogonal projection into the span of the eigenfunctions. In all of our examples any
function
v
with continuous
vxx
and
vt
on [0,
L
]×[0,
T
] will be admissible to zero out nonhomogeneous
boundary conditions. Functions linear (or possibly quadratic) in
x
will cause the fewest technical
complications for the eigenfunctions of Table 3.1. However, for the boundary layer problem of Example
6.8 the linear function
v(x)
=1
−x
is not advantageous. We recall that the eigenfunctions of this example are
which are complete and orthogonal in
In this case
where
It is apparent that for
the Fourier coefficients are of order which makes the
projection
PNv(x)
impossible to compute numerically because we have to sum large alternating terms.
On the other hand, if we choose
then
which is, of course, manageable and allows us to resolve the boundary layer of Example 6.8.
< previous page page_158 next page >

page_159
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_159.html[22/02/2009 23:53:04]
< previous page page_159 next page >
Page 159
Exercises
6.1)
i) Find
t*
when
u
(1,
t
) reaches its maximum,
ii) Find
t**
when
ut
(1,
t
) reaches its maximum.
6.2)
i) Find
t*
when |
ux
(1,
t
)| reaches its maximum,
ii) Find
t**
when |
uxt
(1,
t
)| reaches its maximum.
6.3)Verify that the solution for the composite slab in Example 6.11 is independent of
X
for
α
1
=α
2 and
k
1
=k
2.
6.4)Show that the problem
i)
can be transformed (i.e., made nondimensional) into
ii)
where
D
depends on the parameters of problem i).
< previous page page_159 next page >

page_160
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_160.html[22/02/2009 23:53:05]
< previous page page_160 next page >
Page 160
problem ii) with an eigenfunction expansion. Determine
D
such that
U
(.5, .5) −.5.
What do
U
(.5, .5)=.5 and the value of
D
imply about the relationship between
u, x, t,
and the
parameters of problem
i
).
6.5)Determine a quadratic
P
2
(x)
such that the solution of
minimizes
6.6)Solve in the subspace of the first N eigenfunctions the following problem:
Find
u
0
(x)
such that the solution of
satisfies
PNu
(
x,
1)=
PNx
2(2−
x
).
Compute the solution for
N
=1, 2, 3 and infer its behavior as
N
becomes large.
6.7)Combine numerical integration and differential equations methods, if necessary, to solve in the
subspace of the first two eigenfunctions the reaction diffusion problems
i)
and
ii)
both subject to
ux
(0,
t
)=
ux
(1,
t
)=0
u
(
x,
0)=cos
πx
.
< previous page page_160 next page >

page_161
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_161.html[22/02/2009 23:53:06]
< previous page page_161 next page >
Page 161
Chapter 7
One-Dimensional Wave Equation
7.1 Applications of the eigenfunction expansion method
Following the format of Chapter 6 we examine the application of the eigenfunction expansion approach
to the one-dimensional wave equation. Examples involve vibrating strings, chains, and pressure and
electromagnetic waves. We comment on the convergence of the approximate solution to the analytic
solution and conclude by showing that the equations to be solved in the eigenfunction expansion
method are identical to those which arise when Duhamel’s principle is combined with the traditional
product ansatz of separation of variables.
Example 7.1 A vibrating string with initial displacement.
The vibrating string problem served in Chapter 5 to introduce eigenfunction expansions for the wave
equation. Here we shall use this simple setting to examine the method in some detail.
Suppose that a string of length
L
with fixed ends is displaced from its equilibrium position and let go at
time
t
=0. We wish to study the subsequent motion of the string.
Newton’s second law and a small amplitude assumption lead to the following mathematical model for
the displacement
u(x, t)
of the string at point
x
and time
t
from the equilibrium
(7.1)
< previous page page_161 next page >
