Blank L., Tarquin A. Engineering Economy (McGraw-Hill Series in Industrial Engineering and Management)
Подождите немного. Документ загружается.

SECTION 3.3 Calculations for Shifted Gradients
Figure 3- 1
Ob
and c partitions these two series. Gradient year 2
is
placed in year 5
of
the
entire sequence
in
Figure 3-10c.
It
is clear that n = 5 for the
piG
factor. The P G = ?
arrow
is
correctly placed
in
gradient year 0, which is year 3
in
the cash flow series.
The general relati.
on
for
P.,.
is
taken from Equation [2.18]. The uniform series A =
$100 occurs for all 8 years, and the G = $50 gradient present worth appears
in
year
3.
P
T
= P
A
+ P
G
= 100(pIA,i,8) + 50(pIG,i,5)(pIF,i,3)
The
PIG
and
AI
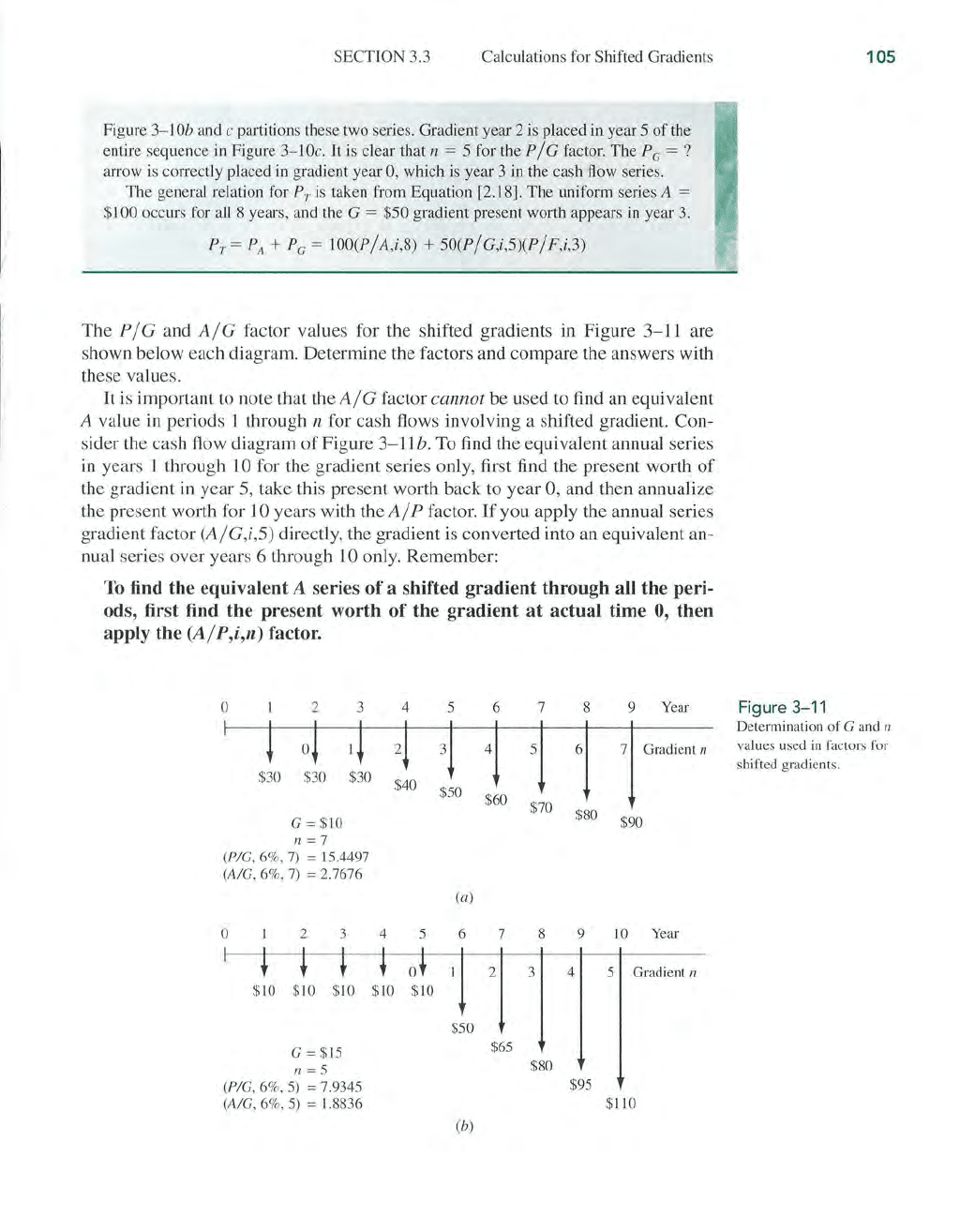
G factor values for the shifted gradients in Figure 3-11 are
shown below each diagram. Determine the factors and compare the answers with
these values.
It is important to note that the
AI
G factor
cannot
be used to find an equivalent
A value in periods 1 through n for cash flows involving a shifted gradient. Con-
sider the cash flow diagram
of
Figure 3-11h. To find the equivalent annual series
in years 1 through 10 for the gradient series only, first find the present worth
of
the gradient in year 5, take this present worth back to year 0, and then annualize
the present worth for
10 years with the
Alp
factor.
If
you apply the annual series
gradient factor
(AIG,i,5)
directly, the gradient is converted into an equivalent an-
nual series over years 6 through 10 only. Remember:
To
find the equivalent A series
of
a shifted
gradient
through
all
the
peri-
ods, first find the
present
worth
of the
gradient
at
actual time 0,
then
apply the
(AIP,i,n)
factor.
0
2 3
4
5 6 7 8 9
Year
3 4 5 6 7 Gradient
n
$30
$30
$30
$40
$50
$60
$70
$80
C =
$10
$90
n=7
(PIC, 6%, 7) = 15.4497
(AlC
, 6%, 7) = 2.7676
(a)
0
2
3
4
5 6 7 8 9
10 Year
2
3
4
5
Gradient
n
$ 10 $ 10
$10 $10
$10
$50
C = $ 15
$65
n=5
$80
(PIC, 6%, 5) = 7.9345
$95
(AlC
, 6%, 5) = 1.8836
$110
(b)
105
Figure
3-
11
Determination
of
C and n
values used
in
factors for
shifted gradients.
106
s
Q-Solv
(PIA,g
,i
,n)
fact
or
CHAPTER 3 Combining Factors
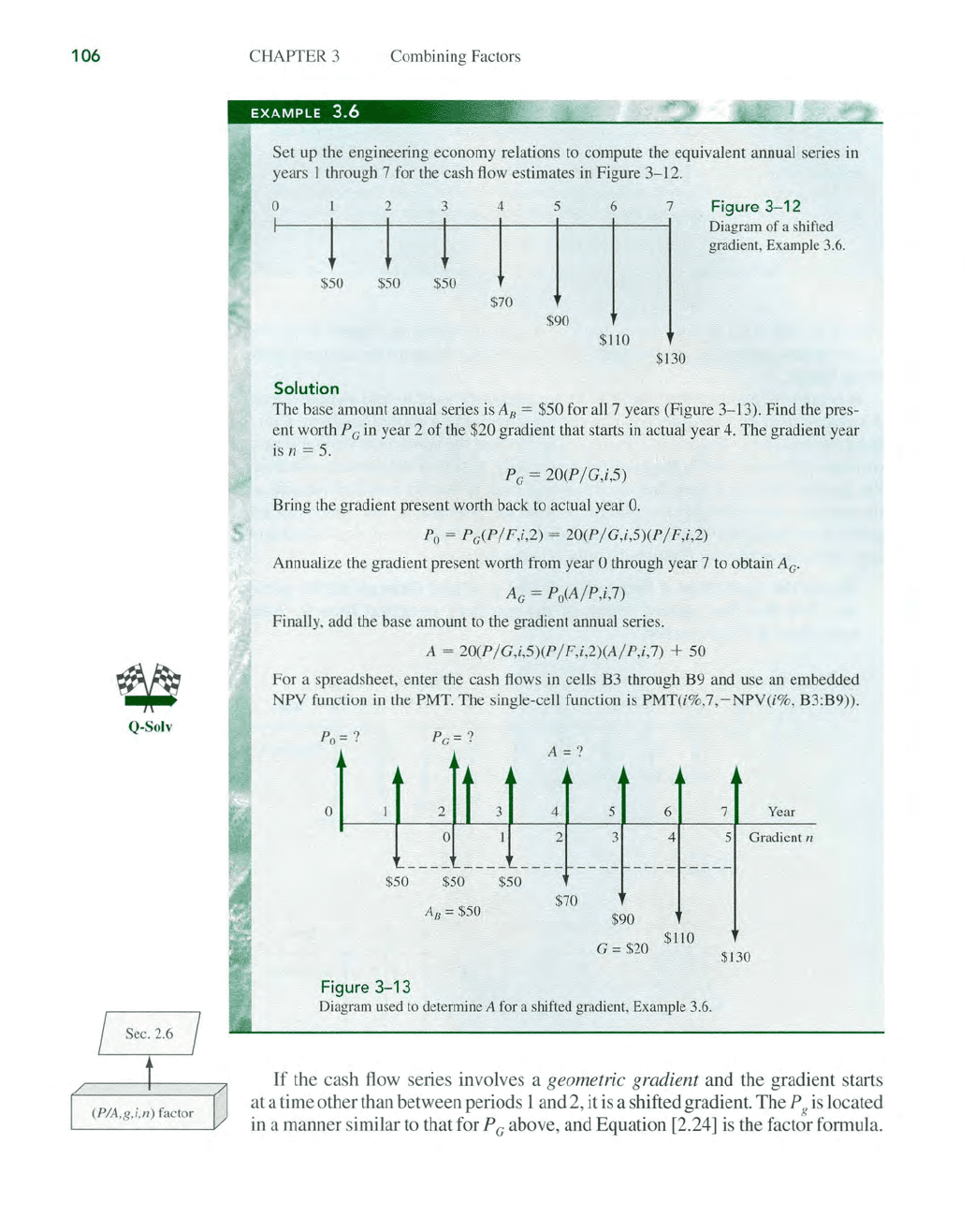
EXAMPLE
3.6
Set
up
the engineering economy relations to com
pu
te
the equivalent
an
nu
al series
in
years"] throu
gh
7 for the cash flow estimates
in
Figure
3-12.
0
2
3
4
5
6
7
Figure
3-12
Diagram
of
a shifted
gradient,
Example 3.6.
$50 $50
$50
$70
$90
$1
10
$130
Solution
The base amount annual series is
A8
=
$SO
for all 7 years (Figure 3-13). Find
th
e pres-
ent worth P G
in
year 2
of
the $20 gradient that star
ts
in
actual year
4.
The gradient year
is
n = S.
P G = 20(P I
G,
i,S)
Bring the gradient present worth back
to
actu
al
year
O.
Po
= P
G
(PIF,i,2) = 20(PIG,i,S)(PIF,i,
2)
Annualize the gradient present worth from year 0 throu
gh
year 7 to obtain A
G
.
AG
=
Po(A
I P,i,7)
Finally, add the base amount to the gradient
an
nu
al
series.
A
= 20(P I G,i,S)(P I F,i,2)(AI P,i,7) +
SO
For a spreadsheet, enter the cash flows in ce
ll
s B3 throu
gh
B9
an
d use
an
embedded
NPY function
in
the PMT. The single-ce
ll
function is PMT(i%,7,
-NPY(i
%, B3:B9».
P
o
= ?
Pc=?
A = ?
O
~!
___
~
~
_
-
_-
__
~_
~
--_
4'2
~
---~-
~
---:
~
----:
+-
G-r
-
:-~-:-·t-n
$50
$50
AIJ
= $50
Figure
3-13
$50
$70
$90
$1
10
G = $20
Diagram used
to
determine A for a shifted gradient, Exam
pl
e 3.6.
$130
If
the
ca
sh flow series involves a geometric gradient
and
the gradient starts
at a time other than between periods 1 and 2, it is a shifted gradient.
The
P
g
is located
in
a manner similar to that for P G above, and Equation [2.24]
is
the factor formula.
SECTION 3.3 Calculations for Shifted Gradients
EXAMPLE
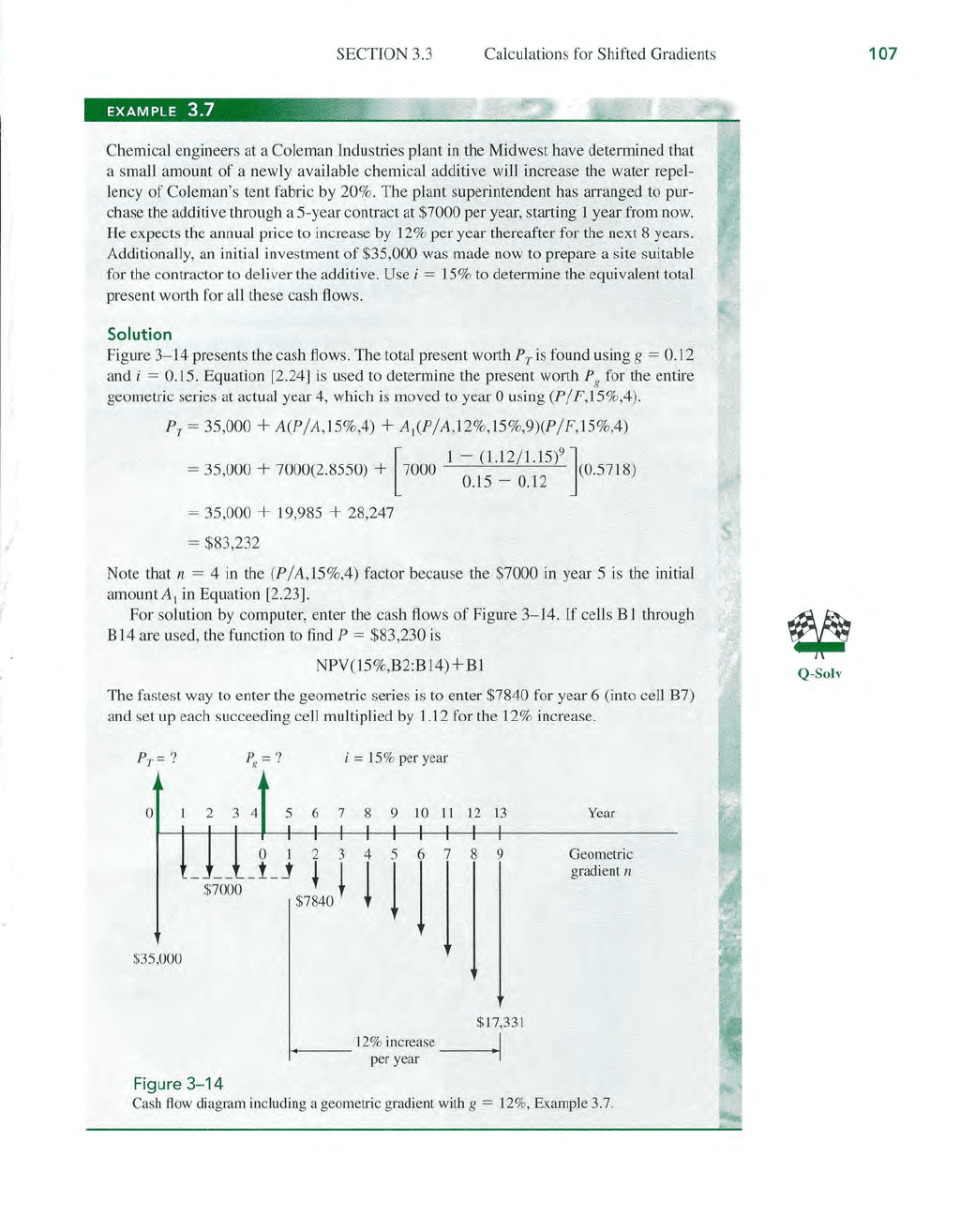
3.7
':!~
Chemical engineers at a Coleman Industries plant in the Midwest have determined that
a small amount
of
a newly available chemical additive will increase the water repel-
lency
of
Coleman's tent fabric by 20%.
The
plant superintendent has arranged
to
pur-
chase the additive through a 5-year contract at
$7000 per year, starting I year from now.
He expects the annu
al
price to increase by 12% per year thereafter for the next 8 years.
Additionally, an initial investment
of
$35,000 was made now to prepare a site suitable
for the contractor to deliver the additive. Use i = 15% to determine the equivalent total
present worth for all these cash flows.
Solution
Figure 3- 14 presents the cash flows.
The
total present worth P
y
is
found using g = 0.12
and i = 0.15. Equation [2.24] is used
to
determine the present worth P
g
for the entire
geometric se
ri
es at actual year 4, which is moved to year 0 using (P / F,15%,4).
P
y
= 35,000 + A(P/A,15%,4) + A
1
(P/A,12%,15%,9)(P/F,15%,4)
= 35,000 + 7000(2.8550) + 7000 (0.5718)
[
1
-
(l.12/1.15)9]
0.15 - 0.12
= 35,000 + 19,985 + 28,247
= $83,232
Note that
n = 4 in the (P / A,15%,4) factor because the $7000 in year 5 is the initial
amount
A I
in
Equation [2.23].
For
solution by computer, enter the cash flows
of
Figure
3-14.
If
cells B 1 through
B 14 are used, the function to find
P = $83,230 is
NPV(
15
%,B2:BI
4)+
BI
The fastest way
to
enter the geometric series
is
to enter $7840 for year 6 (into cell B7)
and set
up
each succeeding cell multiplied by 1.12 for the 12% increase.
P
T
= ?
0 2 3
$7000
$35,000
F
igure
3-14
P
g
=?
i = 15% per year
4
5 6 7 8 9 10
11
12
13
0
1 2
3
4
5 6
7
8 9
_Lj
$7tO
+
t
I
j
j
$17,331
12 % increase I
1
--
----+-i-
per year
Year
Geometric
gradient
n
Cash flow diagram including a geometric gradient with g = 12%, Example 3.7.
107
Q-Solv
108
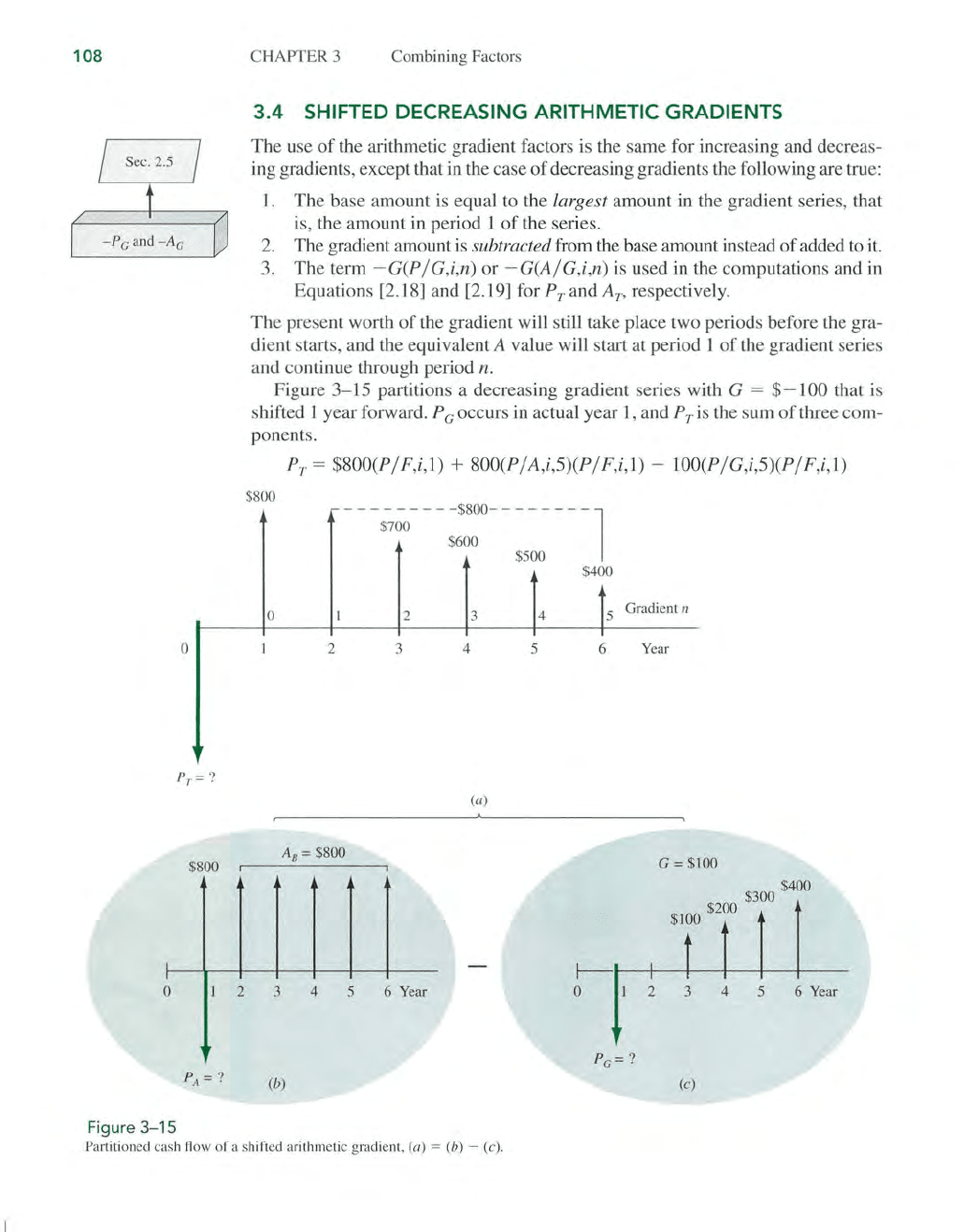
- Pc and -
Ac
0
P
T
= ?
$800
o
Figure
3-15
CHAPTER 3 Combining Factors
3.4
SHIFTED DECREASING ARITHMETIC GRADIENTS
The use
of
the arithmetic gradient factors is the same for increasing and decreas-
ing gradients, except that
in the case
of
decreasing gradients the following are true:
I . The base amount is equal to the largest amount in the gradient series, that
is, the amount in period 1
of
the series.
2. The gradient amount
is
subtracted from the base amount instead
of
added
to
it.
3.
The
term -
G(P/G,i,n)
or
-G(A/G,i,n)
is used in the computations and
in
Equations [2.18] and [2.19] for
PTandA
T
, respectively.
The
present worth
of
the gradient will still take place two periods before the gra-
dient starts, and the equi valent A value will start at period I
of
the gradient series
and continue through period
n.
Figure 3-
15
partitions a decreasing gradient series with G =
$-100
that
is
shifted 1 year forward. P G occurs
in
actual year 1, and P T
is
the sum
of
three com-
ponents.
P T = $800(P / F,i,
1)
+ 800(P / A,i,5)(P /
F,i,])
- 1
OO(P
/ G ,i,5)(P /
F,i,])
$8
00
--
-
$~
~O---
~
::O-
- :
5~
-
--l
0 2 3
4
2
3 4 S
(a)
A8
=
$800
2 3 4 5 6 Year
(b)
$4
00
ts
6
Gr
adient n
Year
G = $1
00
$400
$200 $300
$100
~
~
-r--r--r--r--r---
o
2 3 4 S 6 Year
Pc = ?
(e
)
Partitioned cash now
of
a shifted arithmetic gradient, (a) = (b) - (e
).
SEC
TI
ON 3
.4
Shifted Decreas
in
g
Ari
thmetic Gradients
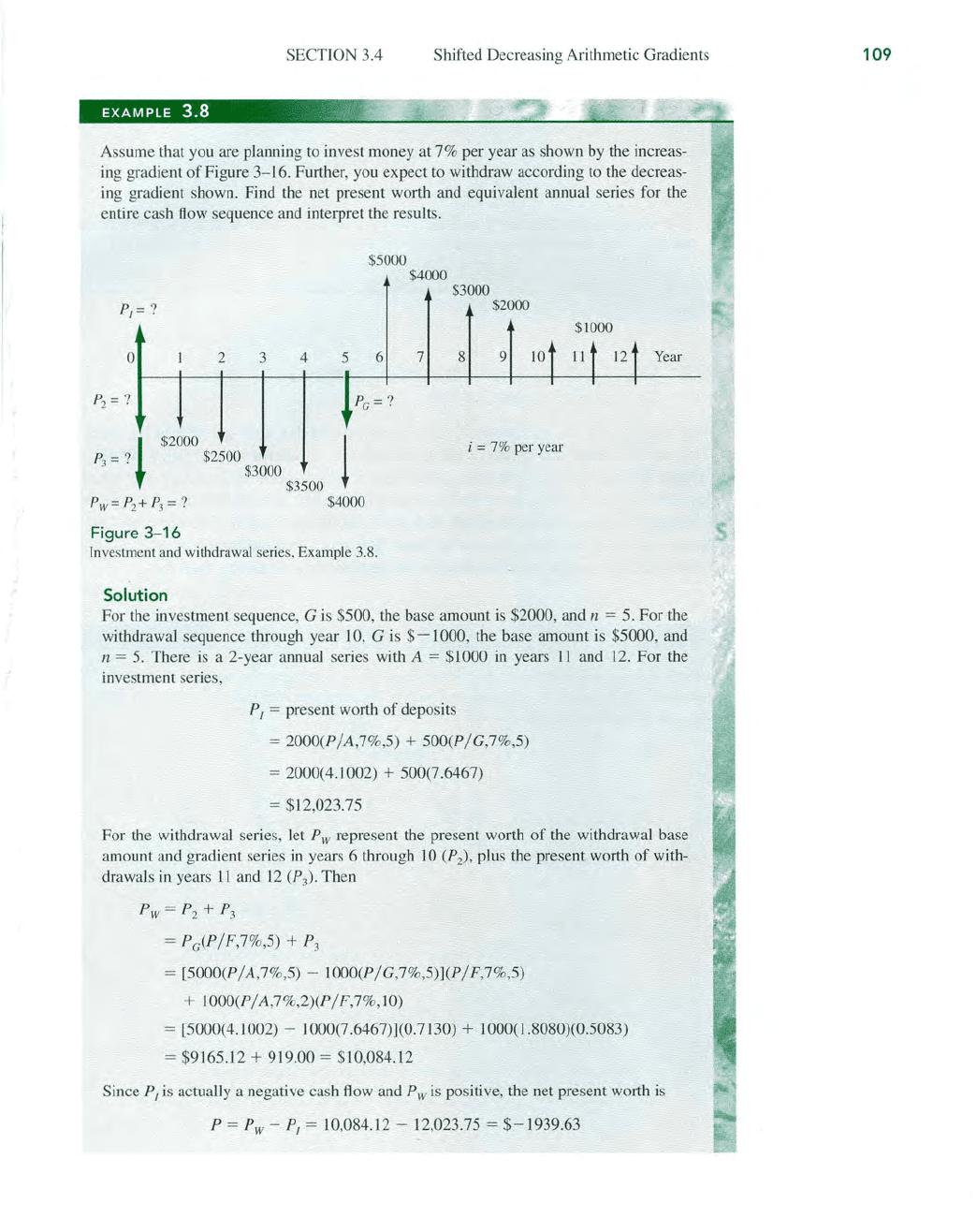
EXAMPLE 3.8
.'ft:
Ass
um
e
th
at you are pla
nn
in
g to invest money at 7% per year as shown
by
the increas-
in
g gradient of Figure 3-
16.
Further, you expect to withdraw according to the decreas-
in
g gradient shown. Find the net present worth and equivalent annual series for the
entire cash
flow
sequence a
nd
interpret
th
e results.
$
500
0
$
4000
$3
000
= ?
$2
000
$
1000
o
I 2
3
4
5
6 7 8 9
lO
t
11 t 12 t
Year
~
Pc = ?
$
2000
i = 7% per year
$2500
3 0
$ 0 0 I
$
3500
$
4000
Figure
3-16
In
ves
tm
ent a
nd
withdrawal se
ri
es, Example 3.8.
Solution
For
th
e
in
ves
tm
ent sequence, G is $
500
, the base amount is $2000, and n = 5. For the
withdrawal se
qu
ence through year
10
, G is
$-
1000, the base amount is $5000, and
n = 5. There is a 2-year annual se
ri
es
widl A = $
LOOO
in
years
11
a
nd
12
. For the
in
ves
tm
ent series,
P, = present worth of deposits
= 2000(P/A,7
%,
S) +
SOO
(P/
G,7
%,
S)
= 2000(4.1002) + 500(7.6467)
= $12,023.
75
For
th
e withdrawal series, let P
w
represent the present worth
of
the withdrawal base
amount and gradient series in years 6 through
10
(P
z
),
pl
us
the present worth
of
with-
drawals
in
years
11
and l2 (P
3
).
Then
P
w
= P
2
+ P
3
= P
c
(P/F,7
%,
S)
+ P
3
=
[SOOO
(P/A,7
%,
S) - 1
000CP
/ G,7
%,
S)
]CP/F,7%,
S)
+ 1000(P/A,7
%,
2)
(P/ F,7
%,
1O
)
= [5000(4.1002) - 1000(7.64
67
)]CO.7130) + 1000(1.8080)(0.5083)
= $916S.l2 +
91
9.00 = $
10
,084
.1
2
S
in
ce
P,
is ac
tu
a
ll
y a nega
ti
ve cash
fl
ow a
nd
P w is positive, the net present worth is
P = P
w
-
P,
=
10
,08
4.1
2 -
12
,023.75 =
$-
1939.63
109
110
~
E-Solve
CHAPTER 3 Combining Factors
The
A value may be computed using the
(AI
P,7%,
12)
factor.
A = P(AIP,7%,
12)
=
$-244.20
The interpretation
of
these results is as follows:
In
present-worth equivalence, you w
ill
invest $1939.63 more than you expect to withdraw. This
is
equivalent to
an
annual sav-
ings
of
$244.20 per year for the 12-year period.
3.5 SPREADSHEET
APPLICATION-USING
DIFFERENT FUNCTIONS
The
example below compares a solution by computer with a solution by hand.
The cash flows are two shifted uniform series for
which the total present worth is
sought. Naturally, only one set
of
relations for the solution by hand, or one set
of
functions for the computer solution, would
be
used to find P
T'
but the example il-
lu
strates the different approaches and work involved
in
each. The computer so-
lution is faster, but the solution by hand helps in the understanding
of
how the
time value
of
money is accounted for by engineering economy factors.
EXAMPLE
3.9
<:~
Q-So
lv
Determine the total present worth
P)'
in
period 0 at
15
% per year for the two shifted uni-
form series
in
Figure 3-17. Use two approaches:
by
computer with different functions and
by
hand using three different factors.
o
I
2
I
Figure
3-1
7
i =
15
% per year
345
t t t
Al
= $1000
6
I
7
I
8
I
9 to
II
12
13
I I I I I
A2 = $1500
Un
iform series used
to
compute prese
nt
worth by several methods, Example 3.9.
Solution by
Computer
Figure
3-18
finds P)' using the NPY and PY functions.
NPV function: This
is
by far the easiest way to determine P
T
= $3370. The cash flows
are entered into cells, and the NPY function is developed using the format
NPY(i%,second_
cell:lasccelI)
+ first_ cell
or
NPY(Bl,B6:B
18)+
B5
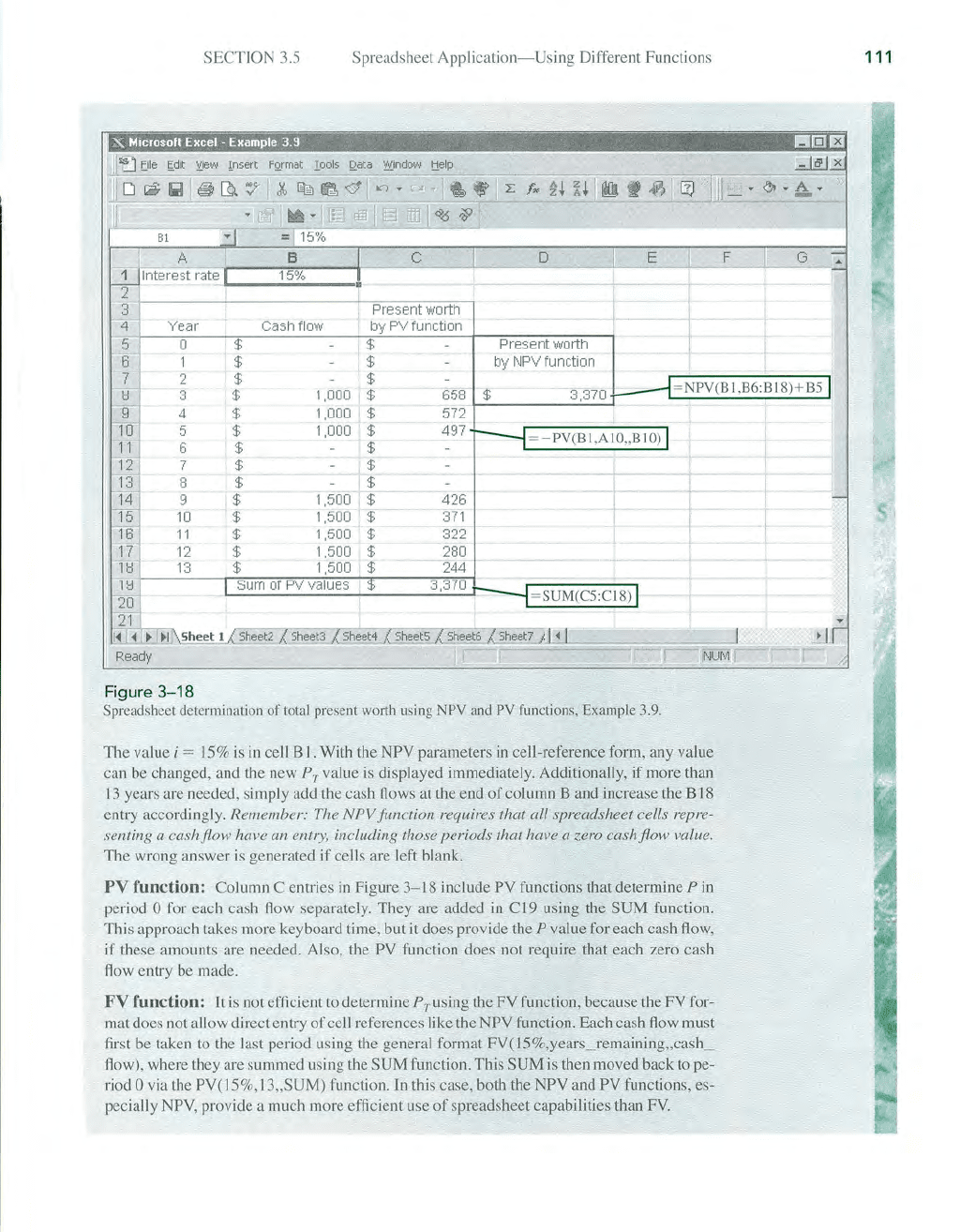
SECTION 3.5
Spreadsheet
Application-Using
Different Functions
Cash flu.".
on
Present worttl
$
by
I~PV
function
$
1,000 $ 658 $ 3,370
4 1,000 , $ 572
5
1,000 $ 497
6
$
7
, $
8
: $
t
9
1,500 $
426
10
$
371
i
11
$
12 $
$
Figure
3-18
Spreadsheet determination
of
total present worth using NPV and PV functions, Example 3.9.
Tbe value i = 15%
is
in cell B I. With the NPV parameters
in
cell-reference form, any value
can be changed, and the new
PI"
value is displayed immediately. Additionally,
if
more than
13
years are needed, simply add the casb flows at the end
of
column B and increase the B I 8
entry accordingly.
Remember: The
NPV
function requires that all spreadsheet cells repre-
senting a cashjlow have an entry, including those periods that have a zero cash flow value.
The wrong answer
is
generated if cells are left blank.
PV
function: Column C entries
in
Figure
3-18
include
PV
functions that determine P in
period
0 for each cash flow separately. They are added in C
19
using the SUM function.
Tbis approach takes more keyboard time, but it does provide the
P value for each cash flow,
if
these amounts are needed. Also, the PV function does not require that each zero cash
flow entry be made.
FV function:
It
is
not efficient
to
determine P
T
using the
FY
function, because the FV for-
mat does not allow direct entry
of
cell references like the NPV function. Each cash flow must
first be taken to the last period using the general format FV(l5%,years_remaining"cash_
flow), where they are summed using the
SUM function. This SUM
is
then moved back to pe-
riod
0 via the PV(J5%, J3"SUM) function. In this case, both the NPV and PV functions, es-
pecially
NPV, provide a much more efficient use
of
spreadsheet capabilities than
FY.
111

112
CHAPTER 3 Combining Factors
Solution by Hand
There are numerous ways
to
find
PT.
The two simplest are probably the present worth and
future worth methods. For a third method, use year 7 as an anchor point. This
is
called
th
e
intermediate year method.
P
T
= ?
P
A2
= ? t
P"'?
Gt
o 1 2 t-
-+j--jl----+I--6t-
'
_+~_8_
t-
_+9
__
1t-0_+1J
_ _ 1t-2---i13
AI
=$1000
A2 = $l500
(a) Present worth method
P
r
= ?
o 3 6 2
4 5
7 8 9
~
10
11
12
13
FA2
= ?
~ ~
AI = $1000
A
z
= $
1500
(b) Future worth method
F AI = ?
pJ?
P;
,
.?
o
t--
-t_-+
2_
~
3
1---t4_5-+_~61--_
7
~t--
-t9
__
l+0_~1t-l_+J2_~1
3
AI = $1000
A2 = $
1500
(c) Intermediate year method
Figure
3-19
Computa
ti
on
of
th
e prese
nt
wor
th
of
Figure
3-
l7
by
three method
s,
Examples 3.9.

SECTION 3
.5
Spreadsheet Applica
ti
on
-Us
in
g Different F
un
ctions
Present worth method: See Figure 3-19a.. The use
of
P j A factors for the
un
iform
series, followed by the use
of
P j F factors to obtain the present worth in year 0, finds P
T"
P
Al
=
P
~
,(P
j
F
,
15
%
,2)
= A,(PjA,
15
%,
3)
(PjF,15
%,
2)
= 1000(2.2832)(0.7561)
= $1726
P
A2
=
P
~i
P
j
F
,
15
%,
8)
= A
2
(P
jA,
15
%,
5)(Pj F,
15
%,
8)
= 1500(3.3522)(0.3269)
= $1644
P
T
= 1726 + 1644 = $3370
Future worth method: See Figure
3-l9b.
Us
e the FjA, Fj P, and Pj F factor
s.
P
T
= (FA' + F
A2
)(Pj F,
15
%,
13)
FA' =
F~,(F
j
P,15
%,
8)
= A,(FjA,15%,3)(Fj P,l5%,8)
= 1000(3.4725)(3.0590) = $10,622
F
A2
= A
2
(FjA,
15
%,
5) = 1500(6.7424) = $10,
113
P
T
= (FA, + F
A2
)(PjF,
15
%
,13
) = 20,735(0.1625) = $3369
Intermediate year method: See Figure 3- 19c. Find the equivalent worth
of
both se-
ries at year 7 and then use the
P j F factor.
The
P
A2
value is computed as a present worth; but to find the total value P
-r
at year 0,
it
mu
st
be treated as
an
F value. Thus,
FA'
=
F~,(FjP,15
%,
2)
=
A,(FjA,l5%,3)(F/P
,
15
%,2)
= 1000(3.4725)(1.3225) = $4592
P
A2
=
P
~2
(P
j
F
,
15
%
,l
)
= A
2
(P
jA,l5
%,
5)(Pj F,l5
%,
1)
= 1500(3.3522)(0.8696) = $4373
P-r
=
(FA,
+ P
A2
)(PjF,15%,7)
= 8965(0.3759) = $3370
113
114
CHAPTER 3 Combining Factors
ADDITIONAL
EXAMPLE
EXAMPLE 3.10
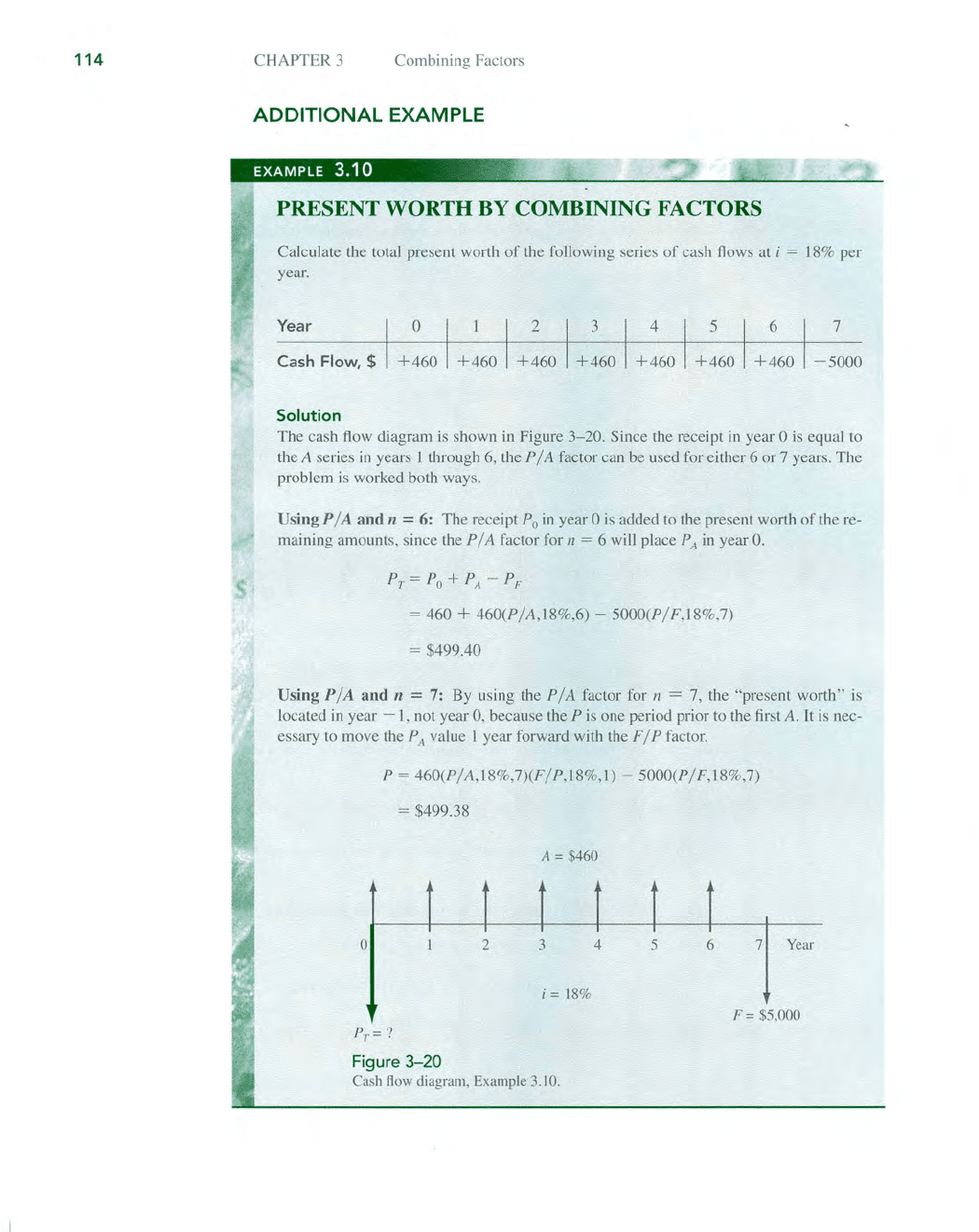
PRESENT WORTH BY COMBINING FACTORS
Calculate the total present worth
of
the following series
of
cash flows at i =
18
% per
year.
Year
7
Cash Flow, $
-5000
Solution
The cash flow diagram is shown
in
Figure 3-20. Since the receipt
in
year 0
is
equal
to
the A series
in
years I through
6,
the
PIA
factor can be lIsed for either 6 or 7 years. The
problem
is
worked both ways.
Using PIA and
Il
=
6:
The
receipt
Po
in
year 0
is
added to the present worth
of
the re-
maining amounts, since the P
IA
factor for n = 6 will place P
A
in year
O.
= 460 +
460(PIA,
18
%,6) -
5000(P
I F,
18
%,7)
= $499.40
Using
PIA
and
Il
=
7:
By using the
PIA
factor for n =
7,
the "present worth"
is
located
in
year
-1,
not year 0, because the P is one period prior
to
the first A. It is nec-
essary to move the P
A
value I year forward with the F I P factor.
P =
460(P
IA,
18
%,
7)(F
I P,18%, I) -
5000(PIF,18
%,7)
= $499.38
A = $460
o 2 3 4 5
6
7 Year
i =
18
%
F=
$5,000
P.,.=
?
Figure
3-20
Cash
flow
diagram, Example 3.
10.
