Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

s14.1 Definitions of Heat Transfer Coefficients

427

from which

aD2 (To

Tbl)

This gives us the formula for calculating h1 from the data given above.

Analogously, use of Eqs. 14.1-3 and 14.1-4 gives

("2

(r)

---

nD2

(To

Tb)a

for obtaining

and h,, from the data.

To evaluate

h,,,,

we have to use the preceding data to construct a continuous curve Tb(z),

as in Fig. 14.1-2, to represent the change in bulk temperature with

in the longest (96-in.)

tube. Then Eq. 14.1-10 becomes

By differentiating this expression with respect to

and combining the result with

Eq.

14.1-5,

we get

Since To is constant, this becomes

The derivative in this equation is conveniently determined from a plot of In(T,

Tb) versus

z/L. Because a differentiation is involved, it is difficult to determine

hlo,

precisely.

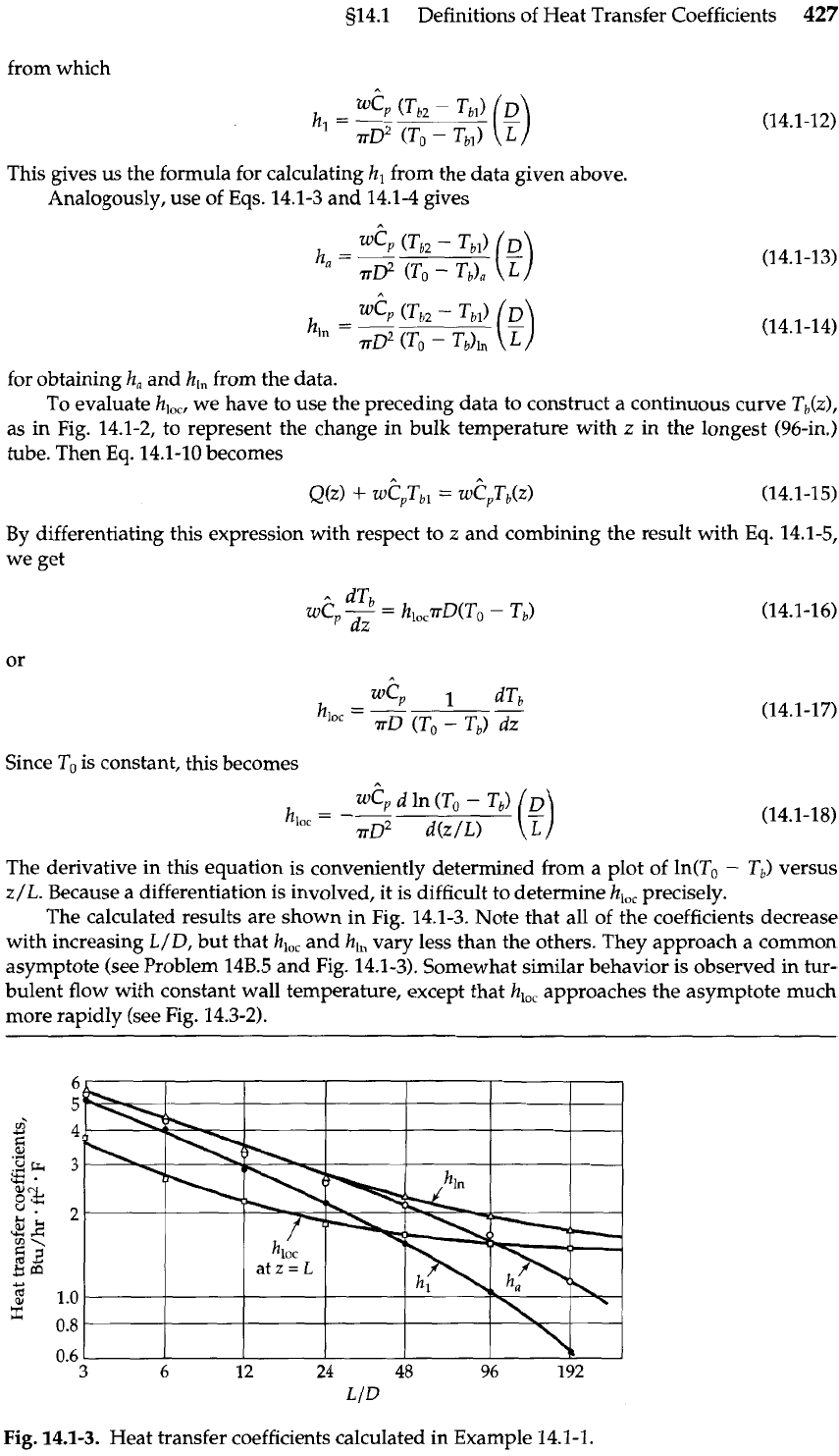
The calculated results are shown in Fig. 14.1-3. Note that all of the coefficients decrease

with increasing LID, but that

hi,,

and

hl,

vary less than the others. They approach a common

asymptote (see Problem 14B.5 and Fig. 14.1-3). Somewhat similar behavior is observed in tur-

bulent flow with constant wall temperature, except that h,, approaches the asymptote much

more rapidly (see Fig. 14.3-2).

LID

Fig.

14.1-3.

Heat transfer coefficients calculated in Example 14.1-1.

428

Chapter

Interphase Transport in Nonisothermal Systems

314.2

ANALYTICAL CALCULATIONS OF

HEAT

TRANSFER

COEFFICIENTS FOR FORCED CONVECTION

THROUGH TUBES AND SLITS

Recall from Chapter

where we defined and discussed friction factors, that for some

very simple laminar flow systems we could obtain analytical formulas for the (dimen-

sionless) friction factor as a function of the (dimensionless) Reynolds number. We would

like to do the same for the heat transfer coefficient, h, which, however, is not dimension-

less. Nonetheless we can construct with it a dimensionless quantity, Nu

hD/k, the

Nusselt number, using the fluid thermal conductivity

and a characteristic length

that

must be specified for each flow system. Two other related dimensionless groups are

commonly used: the Stanton number, St

Nu/RePr, and the Chilton-Colbum j-fnctor for

heat transfer,

Nu/~e~r"~. Each of these dimensionless groups may be "decorated

with subscript 1, a, In, or

corresponding to the subscript on the Nusselt number.

way of illustration, let us return to 310.8 where we discussed the heating of

fluid in laminar flow in a tube, with all the fluid properties being considered constant.

From Eq. 10.8-33 and

Eq.

10.8-31 we can get the difference between the wall temperature

and the bulk temperature:

in which

and

are the radius and diameter of the tube. Solving for the wall flux we

get

Then making use of the definition of the local heat transfer coefficient hlo,-namely, that

h,,,(To

T&we find that

This result is the entry in Eq.

(L)

of Table 14.2-1-namely, for the laminar flow of a con-

stant-property fluid with a constant wall heat flux, for very large

The other entries in

Table 14.2-1 and 2 may be obtained in a similar way.' Some Nusselt numbers for New-

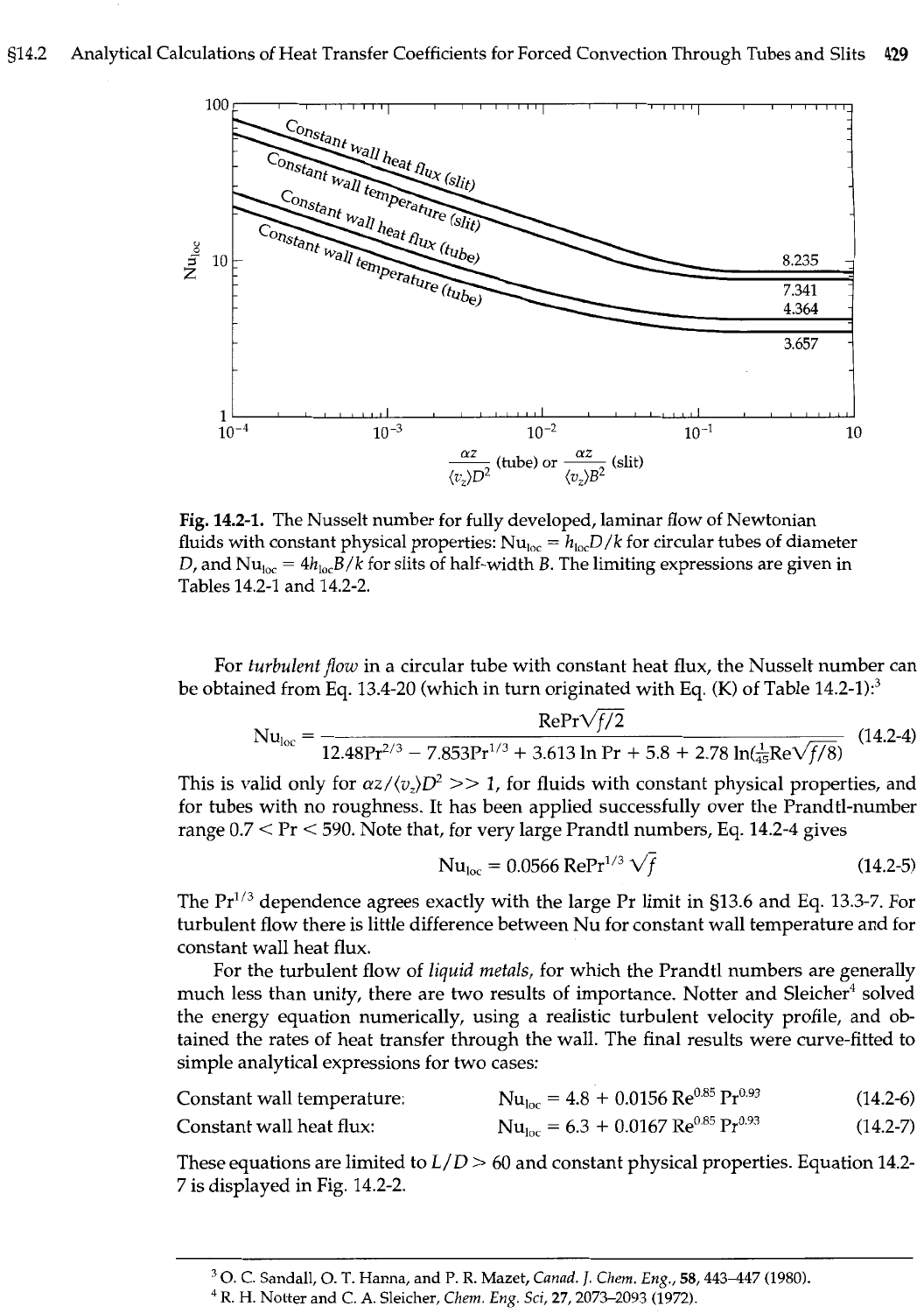
tonian fluids with constant physical properties are shown in Fig. 14.2-1.'

These tables are taken from

B. Bird,

C. Armstrong, and

Hassager,

Dynamics

Polymeric

Liquids,

Vol.

Fluid Mechanics,

1st edition, Wiley, New York, (1987), pp. 212-213. They are based, in turn,

Beek and

Eggink,

De Ingenieur,

74,

(35) Ch. 81-Ch. 89 (1962) and

Valstar and W.

Beek,

De Ingenieur,

75,

(I), Ch. 1-Ch.

(1963).

The correspondence between the entries of Tables 14.2-1 and 2 and problems in this book is as

follows (0

circular tube,

plane slit):

Eq.

(C)

Problem 12D.4 0; 12D.5

Laminar Newtonian

Eq.

(F)

Problem 12D.3 0; 12D.5

Laminar Newtonian

Eq.

(G)

Problem 10B.9(a)

10B.9(b)

Plug flow

Eq.

(I)

Problem 12D.7

12D.6

Laminar Newtonian

Eq.

(K)

Problem 10D.2

Laminar non-Newtonian

Eq.

(L)

Problem 12D.6

Laminar Newtonian

Equations analogous to Eqs.

(K)

in Tables 14.2-1 and

are given for turbulent flow in Eqs. 13.4-19 and

13C.1-1.

s14.2

Analytical Calculations of Heat Transfer Coefficients for Forced Convection Through Tubes and Slits

429

ruz

crz

(tube)

(slit)

(v,D2

(%P2

Fig.

14.2-1.

The Nusselt number for fully developed, laminar flow of Newtonian

fluids with constant physical properties: NuIoc

hlOcD/k for circular tubes of diameter

and Nul,,

4hlocB/k for slits of half-width

The limiting expressions are given in

Tables 14.2-1 and 14.2-2.

For

turbulent

pow

in a circular tube with constant heat flux, the Nusselt number can

be obtained from Eq. 13.4-20 (which in turn originated with Eq.

(K)

of Table 14.2-I):3

This is valid only for

az/(v,)D2

1, for fluids with constant physical properties, and

for tubes with no roughness. It has been applied successfully over the Prandtl-number

range 0.7

590. Note that, for very large Prandtl numbers, Eq. 14.2-4 gives

The Pr1l3 dependence agrees exactly with the large Pr limit in 513.6 and Eq. 13.3-7. For

turbulent flow there is little difference between Nu for constant wall temperature and for

constant wall heat flux.

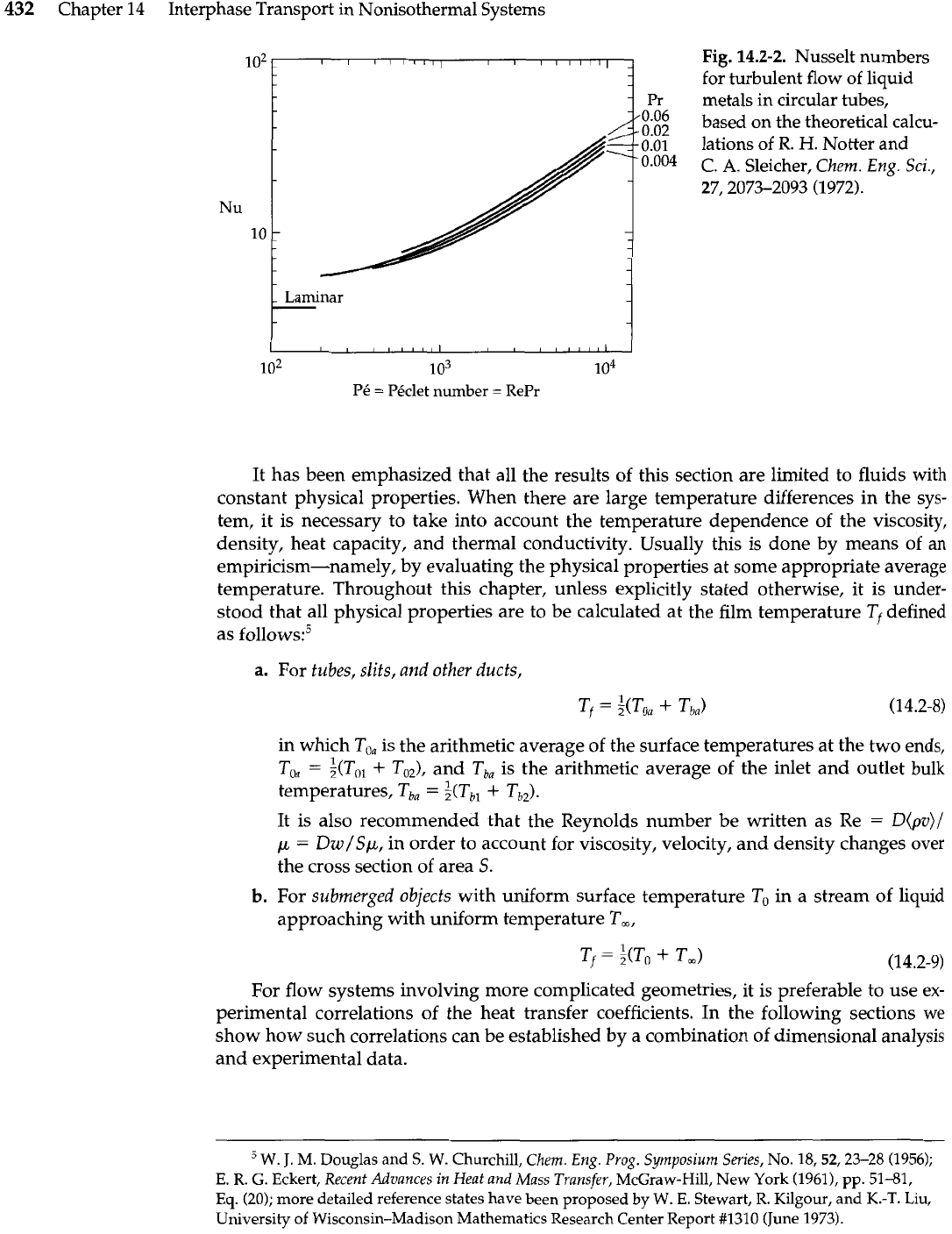
For the turbulent flow of

liquid metals,

for which the Prandtl numbers are generally

much less than unity, there are two results of importance. Notter and Sleicher4 solved

the energy equation numerically, using a realistic turbulent velocity profile, and ob-

tained the rates of heat transfer through the wall. The final results were curve-fitted to

simple analytical expressions for two cases:

Constant wall temperature: Nul,,

4.8

0.0156 (14.2-6)

Constant wall heat flux:

Nu,,,

6.3

0.0167 ~e"-"~

(14.2-7)

These equations are limited to

L/D

60 and constant physical properties. Equation 14.2-

7 is displayed in Fig. 14.2-2.

Sandall,

Hanna, and

Mazet,

Canad.

Chem.

Eng.,

58,443447 (1980).

Notter

and

Sleicher,

Chem.

Eng.

Sci,

27,2073-2093 (1972).

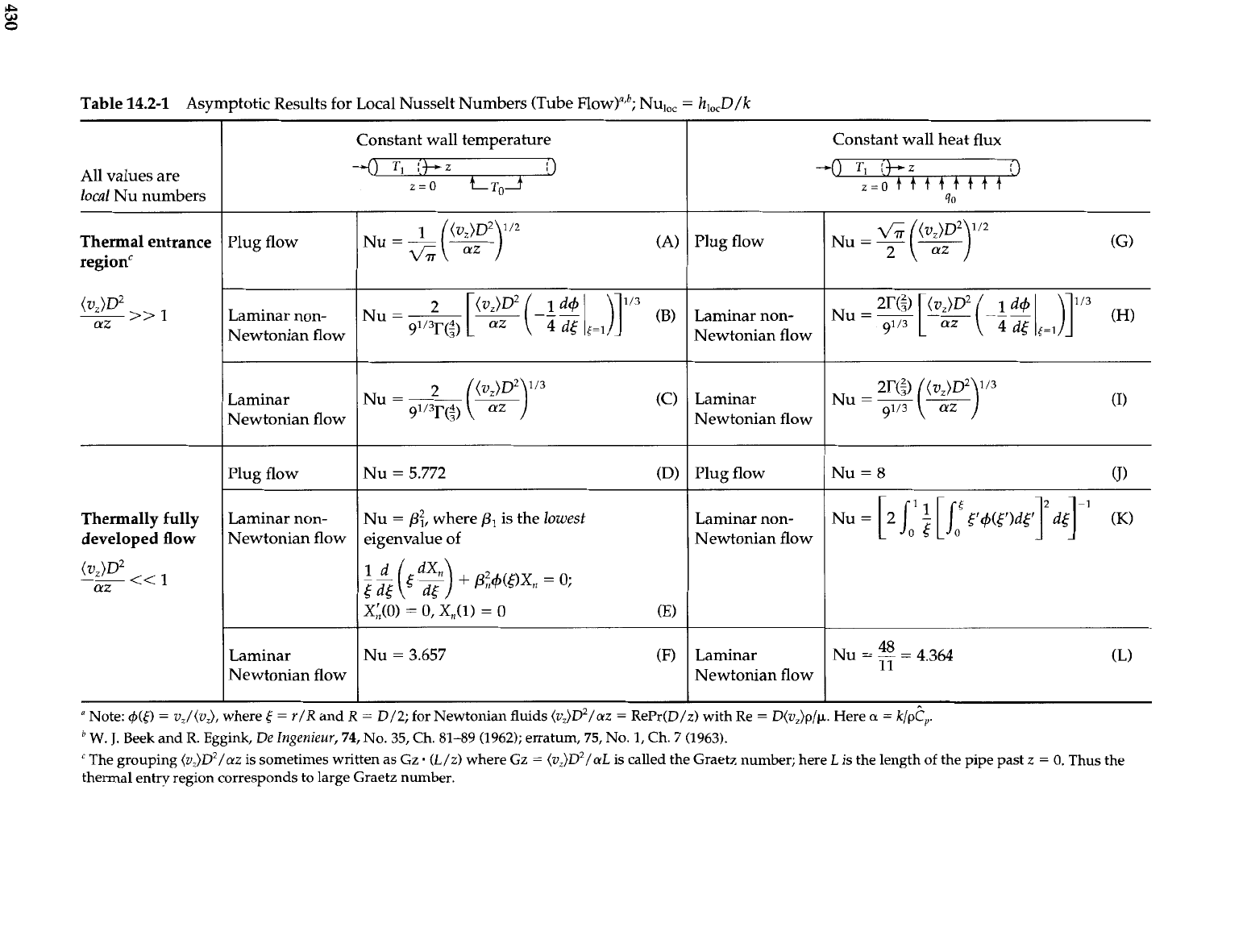
Table

14.2-1

Asymptotic Results for Local Nusselt Numbers (Tube

low)".^;

Nu,,,

h,,,~/k

Constant wall heat flux

Constant wall temperature

[+z

z=o

To2

All values are

local

Nu numbers

Thermal entrance

regionc

Plug flow

Laminar non-

Newtonian flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

Laminar

Newtonian flow

Plug flow

where

is the

lowest

eigenvalue of

Laminar non-

Newtonian flow

Thermally fully

developed flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

Laminar

Newtonian flow

Note:

c#&$)

v,/(v,), where

r/R and R

D/2; for Newtonian fluids (v,)D2/az

RePr(D/z) with Re

D(v,)p/p. Here

k/&.

Beek and R. Eggink, De

Ingenieur,

74,

No.

35,

Ch. 81-89 (1962); erratum,

75,

No. 1, Ch.

(1963).

The grouping (v,)D2/az is sometimes written as Gz (L/z) where Gz

(v,)D2/cuL is called the Graetz number; here

is the length of the pipe past

Thus the

thermal entry region corresponds to large Graetz number.

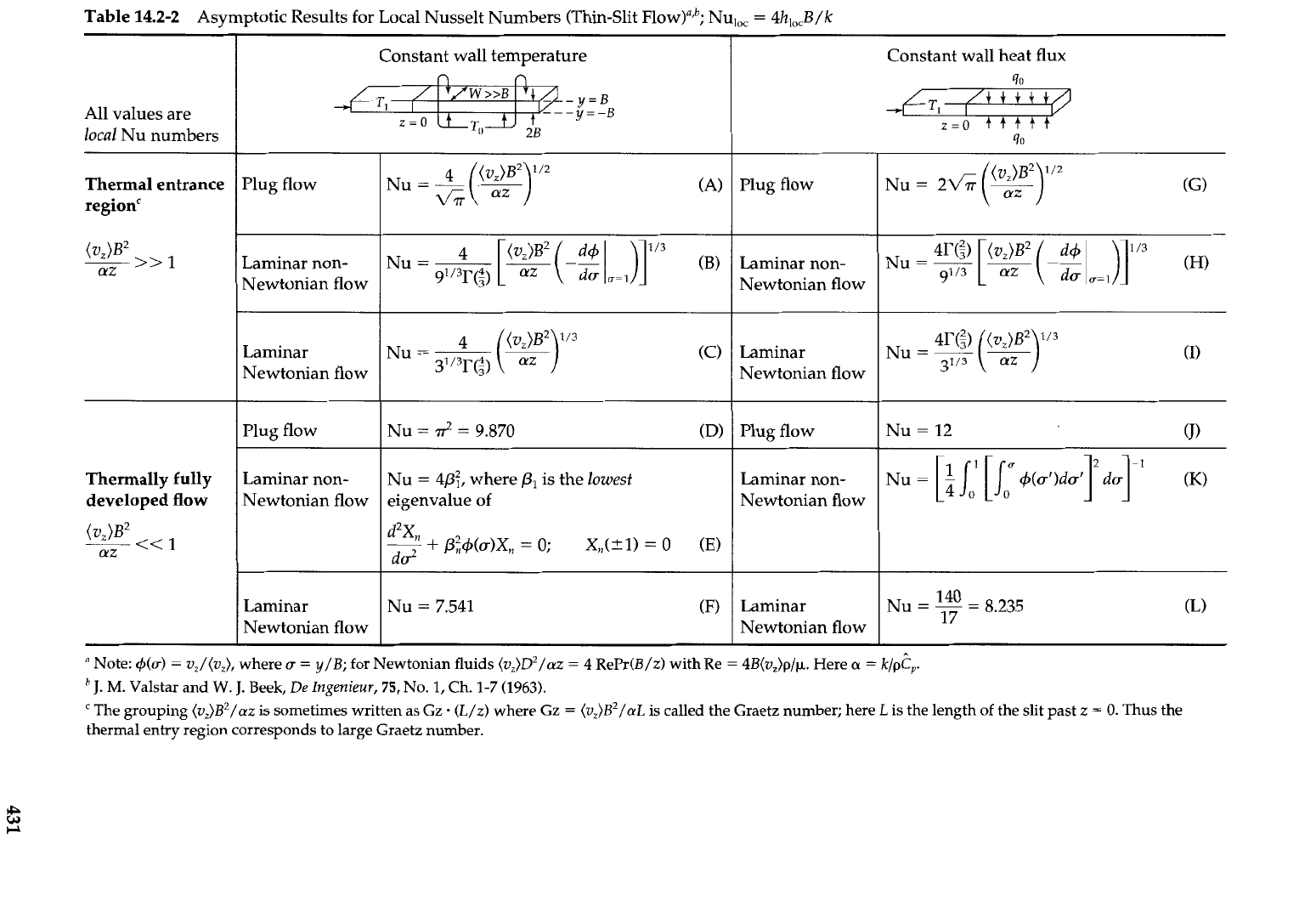
Table

14.2-2

Asymptotic Results for Local Nusselt Numbers (Thin-Slit

low)".^;

Nul,,

4hl,,B/k

All values are

local

Nu numbers

Thermal entrance

regionc

Thermally

fully

developed flow

Constant wall temperature

Plug flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

Plug flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

4&,

where

is the

lowest

eigenvalue of

Plug flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

Plug flow

Laminar non-

Newtonian flow

Laminar

Newtonian flow

Constant wall heat flux

Note:

vv,/(vz),

where

for Newtonian fluids

(vz)D2/cuz

RePr(B/z)

with Re

4B(v,)p/p.

Here

klp~,.

Valstar and

Beek,

Ingenieur,

75,

No.

Ch.

1-7 (1963).

The grouping

(vz)B2/az

is sometimes written

(L/z)

where Gz

(vz)B2/aL

is called the Graetz number; here

the length

the slit past

Thus the

thermal entry region corresponds to large Graetz number.

432

Chapter

Interphase Transport in Nonisothermal Systems

It has been emphasized that all the results of this section are limited to fluids with

constant physical properties. When there are large temperature differences in the sys-

tem, it is necessary to take into account the temperature dependence of the viscosity,

density, heat capacity, and thermal conductivity. Usually this is done by means of

empiricism-namely, by evaluating the physical properties at some appropriate average

temperature. Throughout this chapter, unless explicitly stated otherwise, it is under-

stood that all physical properties are to be calculated at the film temperature

defined

follow^:^

For tubes, slits, and other ducts,

Fig.

14.2-2.

Nusselt numbers

for turbulent flow of liquid

metals in circular tubes,

/,/0-06

based on the theoretical calcu-

0.02

<-0.01

lations of

Notter and

'-0.004

Sleicher, Chem.

Eng.

Sci.,

27,2073-2093 (1972).

lo2

in which

To,

is the arithmetic average of the surface temperatures at the two ends,

To,

;(T,,

To,),

and

Tb,

is the arithmetic average of the inlet and outlet bulk

temperatures,

Tb,

;(T~,

Tb2).

lo2

104

PCclet number

RePr

I I,,

,1111

//@@@;

Laminar

It is also recommended that the Reynolds number be written as Re

D(pv)/

Dw/Sp, in order to account for viscosity, velocity, and density changes over

the cross section of area

For submerged objects with uniform surface temperature

in a stream of liquid

approaching with uniform temperature

T,,

$(T,

T,)

For flow systems involving more complicated geometries, it is preferable to use ex-

perimental correlations of the heat transfer coefficients.

the following sections

show how such correlations can be established by a combination of dimensional analysis

and experimental data.

J. M. Douglas and

W. Churchill,

Chem. Eng.

Pvog.

Symposium Series,

No. 18,52,23-28 (1956);

Eckert,

Recent Advances in Heat and Mass Transfer,

McGraw-Hill, New York (1961),

pp.

51-81,

Eq.

(20); more detailed reference states have been proposed by

Stewart,

Kilgour, and K.-T. Liu,

University

Wisconsin-Madison Mathematics Research Center Report #I310 (June 1973).

g14.3

Heat Transfer Coefficients for Forced Convection in Tubes

433

514.3

HEAT TRANSFER COEFFICIENTS FOR

FORCED CONVECTION IN TUBES

In the previous section we have shown that Nusselt numbers for some laminar flows can

be computed from first principles. In this section we show how dimensional analysis

leads us to a general form for the dependence of the Nusselt number on various dimen-

sionless groups, and that this form includes not only the results of the preceding section,

but turbulent flows as well. Then we present a dimensionless plot of Nusselt numbers

that was obtained by correlating experimental data.

First we extend the dimensional analysis given

511.5 to obtain a general form for

correlations of heat transfer coefficients in forced convection. Consider the steadily driven

laminar or turbulent flow of a Newtonian fluid through a straight tube of inner radius

as shown in Fig. 14.3-1. The fluid enters the tube at

with velocity uniform out to very

near the wall, and with a uniform inlet temperature TI

Tbl). The tube wall is insulated

except

the region

where a uniform inner-surface temperature To is main-

tained by heat from vapor condensing 02 the outer surface. For the moment, we assume

constant physical properties

k, and

C,.

Later we

will

extend the empiricism given in

$14.2 to provide a fuller allowance for the temperature dependence of these properties.

We follow the same procedure used in 56.2 for friction factors. We start by writing

the expression for the instantaneous heat flow from the tube wall into the fluid in the

system described above,

which is valid for laminar or turbulent flow (in laminar flow,

would, of course, be in-

dependent of time). The

sign appears here because the heat is added to the system in

the negative

direction.

Equating the expressions for

given in Eqs. 14.1-2 and 14.3-1 and solving for

h,,

we get

Next we introduce the dimensionless quantities

r/D,

z/D, and

To)/

(Tbl

TO), and multiply by D/k to get an expression for the Nusselt number Nul

hlD/k:

Thus the (instantaneous) Nusselt number is basically a dimensionless temperature gradient

averaged

over

the heat transfer surface.

Condenser

Fluid

enters

4bj_

Fluid leaves

at uniform

with bulk

temperature

Tb2

Heated section

"1"

with uniform surface

"2"

temperature

Fig.

14.3-1.

Heat transfer in the entrance region of

tube.

434

Chapter

Interphase Transport in Nonisothermal Systems

The dimensionless temperature gradient appearing in Eq. 14.3-3 could, in principle,

be evaluated by differentiating the expression for

obtained by solving Eqs. 11.5-7, 8,

and 9 with the boundary conditions

where

v/(uZ), and

91)/p(v,)~. As in s6.2, we have neglected the

d2/di2

terms of the equations of change on the basis of order-of-magnitude reasoning similar to

that in 94.4. With those terms suppressed, upstream transport of heat and momentum

are excluded, so that the solutions upstream of plane 2 in Fig. 14.3-1 do not depend on

L/D.

From Eqs. 11.5-7,8, and

and these boundary conditions, we conclude that the

di-

mensionless instantaneous temperature distribution must be of the following form:

?(?,

Re, Pr, Br)

for 0

L/D

(14.3-9)

Substitution of this relation into Eq. 14.3-3 leads to the conclusion that

NU,(^)

Nul(Re,

Pr, Br, L/D,

i).

When time-averaged over an interval long enough to include all the tur-

bulent disturbances, this becomes

Nul

Nul(Re, Pr, Br,

L/D)

(14.3-10)

similar relation is valid when the flow at plane

is fully developed.

If, as is often the case, the viscous dissipation heating is small, the Brinkman number

can be omitted. Then Eq. 14.3-10 simplifies to

Nu,

Nul(Re, Pr,

(14.3-1

Therefore, dimensional analysis tells us that, for forced-convection heat transfer in circu-

lar tubes with constant wall temperature, experimental values of the heat transfer coeffi-

cient h1 can be correlated by giving Nu, as a function of the Reynolds number, the

Prandtl number, and the geometric ratio L/D. This should be compared with the similar,

but simpler, situation with the friction factor (Eqs. 6.2-9 and 10).

The same reasoning leads us to similar expressions for the other heat transfer coeffi-

cients we have defined. It can be shown (see Problem 14.B-4) that

Nu,

Nu,(Re, Pr, LID)

Nul,

Nu,(Re, Pr, LID)

Nuloc

Nul,,(Re, Pr,

in which Nu,

h,D/k, Nul,

hl,D/k, and Nu,,,

hl,,D/k. That

is,

to each of the heat

transfer coefficients, there is a corresponding Nusselt number. These Nusselt numbers

are, of course, interrelated (see Problem 14.B-5). These general functional forms for the

Nusselt numbers have a firm scientific basis, since they involve only the dimensional

analysis of the equations of change and boundary conditions.

Thus far we have assumed that the physical properties are constants over the tem-

perature range encountered in the flow system. At the end of s14.2 we indicated that

evaluating the physical properties at the film temperature is a suitable empiricism. How-

ever, for very large temperature differences, the viscosity variations may result in such a

large distortion of the velocity profiles that it is necessary to account for this by introduc-

ing an additional dimensionless group,

pb/po,

where

is the viscosity at the arithmetic

s14.3

Heat Transfer Coefficients for Forced Convection in Tubes

435

average bulk temperature and

is the viscosity at the arithmetic average wall tempera-

ture.' Then we may write

Nu(Re, Pr,

pb/po)

(14.3-15)

This type of correlation seems to have first been presented by Sieder and Tate.2 If, in ad-

dition, the density varies significantly, then some free convection may occur. This effect

can be accounted for in correlations by including the Grashof number along with the

other dimensionless groups. This point is pursued further in 914.6.

Let us now pause to reflect on the significance of the above discussion for con-

structing heat transfer correlations. *The heat transfer coefficient

depends on

eight

physical quantities

(D,

(v),

PO,

pb,

Cp,

L).

However, Eq. 14.3-15 tells us that this de-

pendence can be expressed more concisely by giving Nu as a function of only

four

di-

mensionless groups (Re, Pr,

LID,

pb/pO).

Thus, instead of taking data on

for 5 values

of each of the eight individual physical quantities (58 tests), we can measure

for 5

values of the dimensionless groups (5"ests)-a rather dramatic saving of time and

effort.

good global view of heat transfer in circular tubes with nearly constant wall tem-

perature can be obtained from the Sieder and Tate2 correlation shown in Fig. 14.3-2. This

is of the form of

Eq.

14.3-15. It has been found empiri~ally~,~ that transition to turbulence

usually begins at about Re

2100, even when the viscosity varies appreciably in the ra-

dial direction.

For

highly turbulent

flow,

the curves for LID

10 converge to a single curve. For

20,000 this curve is described by the equation

This equation reproduces available experimental data within about ?20% in the ranges

lo4

105and0.6

100.

For

laminar

flow,

the descending lines at the left are given by the equation

One can arrive at the viscosity ratio

inserting into the equations of change a temperature-

dependent viscosity, described, for example, by a Taylor expansion about the wall temperature:

When the series is truncated and the differential quotient is approximated

a difference quotient, we get

Thus, the viscosity ratio appears in the equation of motion and hence in the dimensionless correlation.

Sieder and

Tate,

Ind.

Eng.

Chem.,

28,1429-1435

(1936).

Colburn,

Trans.

AIChE,

29,174-210 (1933).

Alan

Philip

Colburn

(1904-1955),

provost at the

University of Delaware

(1950-1955),

made important contributions to the fields of heat and mass transfer,

including the "Chilton-Colburn relations."

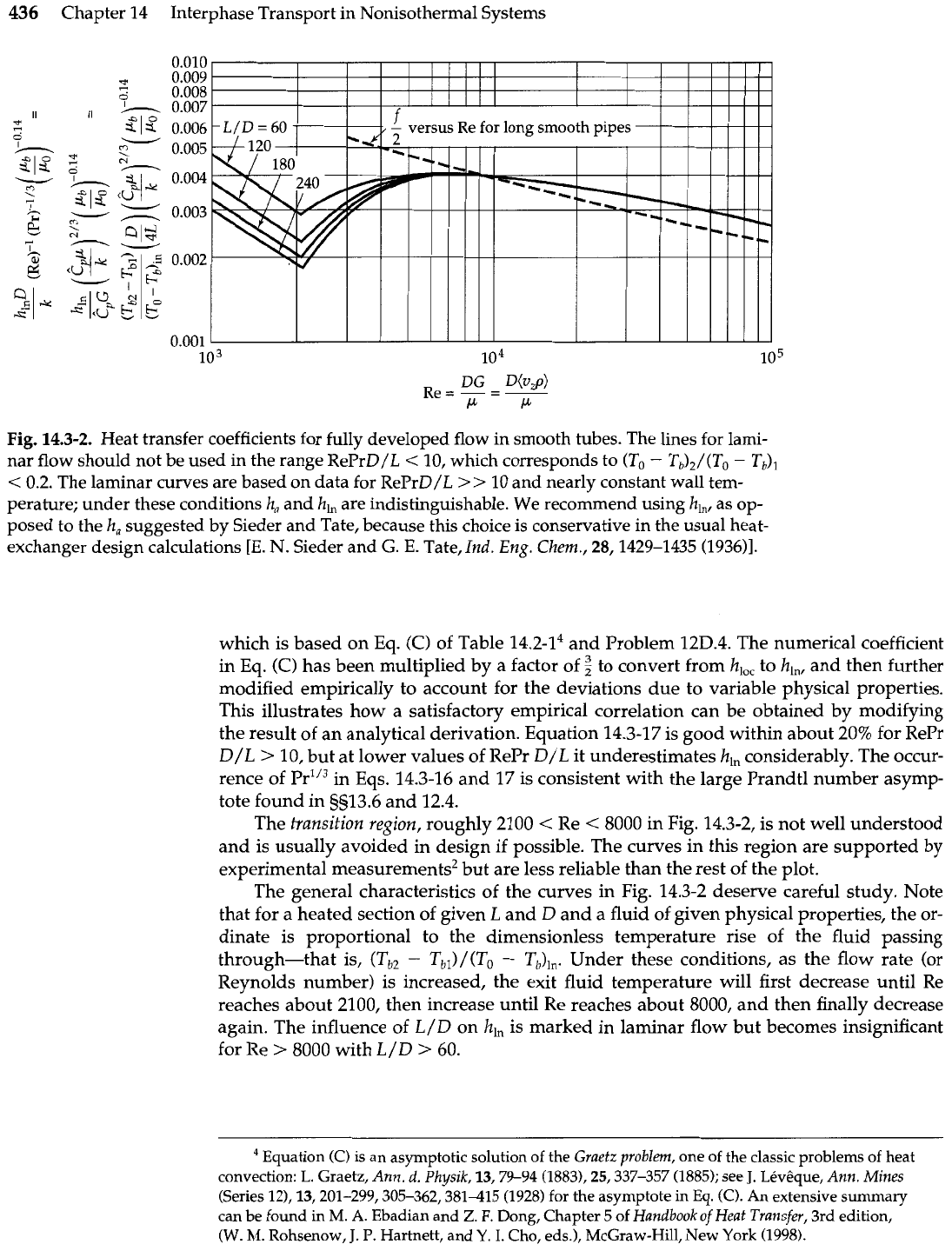
436

Chapter 14 Interphase Transport in Nonisothermal Systems

Fig.

14.3-2.

Heat transfer coefficients for fully developed flow in smooth tubes. The lines for lami-

nar flow should not be used in the range RePrD/L

10, which corresponds to (To

Tb),/(T0

T,),

0.2. The laminar curves are based on data for RePrD/L

10 and nearly constant wall tem-

perature; under these conditions

and

kl,

are indistinguishable. We recommend using k,, as op-

posed to the

suggested

Sieder and Tate, because this choice is conservative in the usual heat-

exchanger design calculations

[E.

Sieder and

Tate,

Ind.

Eng.

Chem.,

28,1429-1435 (1936)l.

which is based on Eq.

(C)

of Table 14.2-l4 and Problem 12D.4. The numerical coefficient

in Eq.

(C)

has been multiplied by a factor of

to convert from h,,, to

hln,

and then further

modified empirically to account for the deviations due to variable physical properties.

This illustrates how a satisfactory empirical correlation can be obtained by modifying

the result of an analytical derivation. Equation 14.3-17 is good within about 20% for RePr

D/L

10, but at lower values of RePr

D/L

it underestimates

hl,

considerably. The occur-

rence of Pr1'3 in Eqs. 14.3-16 and 17 is consistent with the large Prandtl number asymp-

tote found in 9913.6 and 12.4.

The transition region, roughly 2100

8000 in Fig. 14.3-2, is not well understood

and is usually avoided in design if possible. The curves in this region are supported by

experimental measurements2 but are less reliable than the rest

the plot.

The general characteristics of the curves in Fig. 14.3-2 deserve careful study. Note

that for a heated section of given

and

and a fluid of given physical properties, the or-

dinate is proportional to the dimensionless temperature rise of the fluid passing

through-that is,

(T,,

T,,)/(T,

Tb)ln

Under these conditions, as the flow rate (or

Reynolds number) is increased, the exit fluid temperature will first decrease until Re

reaches about 2100, then increase until Re reaches about 8000, and then finally decrease

again. The influence of

L/D

on h,, is marked in laminar flow but becomes insignificant

for Re

8000 with

LID

60.

Equation

(C)

is an asymptotic solution of the

Graetz problem,

one of the classic problems of heat

convection:

Graetz,

Ann.

Physik,

13,79-94 (1883), 25,337-357 (1885); see

Leveque,

Ann. Mines

(Series 12),

13,201-299,305-362,381415

(1928) for the asymptote in

Eq.

(C).

An extensive summary

can be found in

Ebadian and

Dong, Chapter

Handbook

Heat Transfer,

3rd

edition,

(W.

Rohsenow,

Hartnett, and

Cho, eds.), McGraw-Hill, New York (1998).