Bichop R.H. (Ed.) Mechatronic Systems, Sensors, and Actuators: Fundamentals and Modeling

Подождите немного. Документ загружается.

8-2 Mechatronic Systems, Sensors, and Actuators

where we have assumed that the mass does not change over time. The angular momentum of a particle

with respect to an arbitrary reference point O is defined as

(8.3)

where r

is the position vector between points O and P (see Figure 8.1a). The balance of angular momentum

for a single infinitesimally small particle is automatically satisfied as a result of (8.1). In the case of multiple

particles (a rigid body composed of infinite number of particles), the linear and angular momenta are

defined as the sum (integral) of the momentum of individual particles (Figure 8.1b):

(8.4)

The second fundamental law of classical mechanics states that the rate of change of angular momentum

is equal to the sum of all moments acting on the body:

(8.5)

where M

are the applied external force-couples in addition to the forces F

. If the reference point O is

chosen to be the center of mass of the body G, the linear and angular momentum balance law take a

simpler form:

(8.6)

(8.7)

where

is the instantaneous vector of angular velocity and

is the moment of inertia about the center

of mass. Equations 8.6 and 8.7 are called equations of motion and play a central role in the dynamics of

rigid bodies. If there is no motion (linear and angular velocities are zero), one is faced with a

statics problem

Conversely, when the accelerations are large, we need to solve the complete system of Equations 8.6 and

8.7 including the inertial terms. In mechatronic systems the mechanical response is generally slower than

the electrical one and therefore determines the overall response. If the response time is critical to the

application, one needs to consider the inertial terms in Equations 8.6 and 8.7.

8.1.2 Equations of Motion of Deformable Bodies

Rigid bodies do not change shape or size during their motion, that is, the distance between the particles

they are made of is constant. In reality, all objects deform to a certain extent when subjected to external

forces. Whether a body can be treated as rigid or deformable is dictated by the particular application.

FIGURE 8.1 Difinition of velocity and position vectors for single particle (a) and rigid body (b).

(a) (b)

mv()×=

Lvm and H

v× md

×+

9258_C008.fm Page 2 Tuesday, October 9, 2007 9:01 PM

Structures and Materials 8-3

In this section we will review the fundamental equations describing the motion of deformable bodies.

These equations also result from the balance of linear and angular momentum applied to an infinitesi-

mally small portion of the material volume dV. Each element dV is subjected not only to external body

force f, but also to internal forces originating from the rest of the body. These internal forces are described

by a second order tensor T, called stress tensor. The balance of linear momentum can then be stated in

integral form for an arbitrary portion of the body occupying volume V as

(8.8)

where

is the mass density,

is the velocity of the element

, and

is the force per unit volume acting

upon

. The above balance law states that the rate of change of linear momentum is equal to the sum of the

internal force flux (stress) acting on the boundary of

and the external body force, distributed inside

Applying the transport theorem to (8.8) along with the mass conservation law reduces the above to

(8.9)

Since (8.9) is valid for an arbitrary volume, it follows that the integrands are also equal. Thus the local

(differential) form of linear momentum balance is

or with index notation

= T

ij,j

+ f

(8.10)

Using analogous procedure, the balance of angular momentum can be shown to reduce to a simple

symmetry condition of the stress tensor

(8.11)

which is valid for materials without external body couples. It should be mentioned that in certain

anisotropic materials, the polarization or magnetization vectors can develop body couples, for example,

when E × P ≠ 0. In these cases the stress tensor is nonsymmetric and its vector invariant is equal to the

body couple. Equation 8.10 are usually used in one of the three most common coordinate systems. For

example, using rectangular coordinates we have

(8.12)

and in cylindrical coordinates

(8.13)

where (x, y, z) and (r,

, z) are the three coordinates, f ’s are the corresponding body force densities, and

a’s are the accelerations. In addition to Equations 8.12 or 8.13, a relation between the stress and the

displacement is needed in order to determine the deformation. Since the rigid body translations and

-----

v vd

Tn⋅ A f vd

∂V

∇ T⋅ v f vd

∇ Tf+⋅=

∂

----------

∂

----------

∂

----------

+++

, T

∂

----------

∂

----------

∂

----------

+++

, T

∂

----------

∂

----------

∂

----------

+++

, T

∂

----------

θθ

–

--------------------

∂

∂θ

----------

∂

----------

++++

,= T

∂

----------

∂

θθ

∂θ

-----------

∂

----------

+++ +

,= T

∂

----------

∂

∂θ

----------

∂

----------

+++ +

,= T

9258_C008.fm Page 3 Tuesday, October 9, 2007 9:01 PM

8-4 Mechatronic Systems, Sensors, and Actuators

rotations do not cause deformation of the body, they do not affect the internal stress field either. In fact,

the latter is a function of the gradient of the displacement, called deformation gradient. When this

gradient is small, a linear relationship between the displacements and strains can be used

(8.14)

The conservation of momentum and kinematic relations does not contain any information about the

material. Constitutive laws provide this additional information. The most common such law describes a

linear elastic material and can be conveniently expressed using a symmetric matrix c

, called stiffness matrix:

(8.15)

In the most general case, the matrix c

has 21 independent elements. When the material has a crystal

symmetry, the number of independent constants is reduced. For example, single crystal Si is a common

structural material in MEMS with a cubic symmetry. In this case there are only three independent

constants:

(8.16)

If the material is isotropic (amorphous or polycrystalline), the number of independent elastic constants

is further reduced to two by the relation c

= (c

− c

)/2. The elastic constants of several most commonly

used materials are listed in Table 8.1 (from Kittel 1996).

Additional information on other symmetry classes can be found in Nye (1960).

TABLE 8.1 Elastic Constants of Several Common Cubic

Crystals

Stiffness Constants at Room Temperature, 10

N/m

Crystal c

W 5.233 2.045 1.607

Ta 2.609 1.574 0.818

Cu 1.684 1.214 0.754

Ag 1.249 0.937 0.461

Au 1.923 1.631 0.420

Al 1.608 0.607 0.282

K 0.0370 0.0314 0.0188

Pb 0.495 0.423 0.149

Ni 2.508 1.500 1.235

Pd 2.271 1.761 0.17

Si 1.66 0.639 0.796

∂

--------

∂

--------

∂

--------

∂

--------

∂

--------

∂

--------

∂

--------

∂

--------

∂

--------

+=+=+====

symm. c

⋅=

000

symm. c

⋅

9258_C008.fm Page 4 Tuesday, October 9, 2007 9:01 PM

Structures and Materials 8-5

8.1.3 Electric Phenomena

In the previous section the laws governing the motion of rigid and deformable bodies were reviewed.

The forces entering these equations are often of electromagnetic origin; thus one has to know the

distribution of electric and magnetic fields. The electromagnetic field is governed by a set of four coupled

equations known as Maxwell’s equations. Similarly, to the momentum equations, these can also be

postulated in integral form. Here we only give the local form

(8.17)

where E is the electric field, D is the electric displacement, B is the magnetic induction, H is the magnetic

field strength, i is the electric current density, and q

is the free charge volume density. Equations 8.17

require constitutive laws specifying the current density, electric displacement, and magnetic field in terms

of electric field and magnetic induction vectors. A linear form of these laws is given by

(8.18)

where

is the electrical resistance. The coupling between electrical and mechanical fields can be linear

or nonlinear. For example, piezoelectricity is a linear phenomenon describing the generation of electric

field as a result of the application of mechanical stress. Electrostriction on the other hand is a second

order effect, resulting in the generation of mechanical strain proportional to the square of the electric

field. Other effects include piezoresistivity, i.e., a change of the electrical resistance due to mechanical

stress. In addition to these material properties, electromechanical coupling can be achieved through direct

use of electromagnetic forces (Lorentz force) as is commonly done in conventional electrical machines.

Lorentz force per unit volume is given by

(8.19)

where q

is the volume charge density. Equation 8.19 accounts for the forces acting on free charge only.

If the fields have strong gradients, the above expression should be modified to include the polarization

and magnetization terms (Maugin 1988).

(8.20)

Equation 8.19 or 8.20 can be used in the momentum equation 8.10 in place of the body force f.

As mentioned earlier, piezoelectricity and piezoresistivity are the other commonly used effects in

electromechanical systems. The piezoelectric effect occurs only in materials with certain crystal structure.

Common examples include BaTiO

and lead zirconia titanate (PZT). In the quasi-electrostatic approxi-

mation (when the magnetic effects are neglected) there are four variables describing the electromechanical

state of the body—electric field E and displacement D, mechanical stress T and strain ε. The constitutive

laws of piezoelectricity are given as a set of two matrix equations between the four field variables, relating

one mechanical and one electrical variable to the other two in the set

(8.21)

where s

ijkl

is the elastic compliance tensor, d

ijk

is the piezoelastic tensor, Ξ

is the electric permittivity tensor.

If the electric field and the polarization vectors are co-linear, the stress and strain tensors are symmetric,

and the number of independent coefficients in s

ijkl

is reduced from 81 to 21 and for the piezoelastic ten-

sor d

ijk

from 27 to 18. If further, the piezoelectric is poled in one direction only (e.g., index 3), the only

∇ E×+ 0=

∇ D⋅ q

∇ HD

–× i=

∇ B⋅ 0=

----

, D

EP+ , B

M+==

H==

EvB×+()=

∂

------

BP∇E ∇BM⋅+⋅+×+=

ijkl

ijk

, D

+ d

ikl

+==

9258_C008.fm Page 5 Tuesday, October 9, 2007 9:01 PM

8-6 Mechatronic Systems, Sensors, and Actuators

nonzero elements are

Numerical values for the coefficients in (8.22) for bulk BaTiO

crystals can be found in Zgonik et al. 1994.

8.2 Common Structures in Mechatronic Systems

Microelectromechanical systems traditionally use technology developed for the manufacturing of inte-

grated circuits. As a result, the employed mechanical structures are often planar devices—springs, coils,

bridges, or cantilever beams, subjected to in-plane and out-of-plane bending and torsion. Using high

aspect ratio reactive ion etching combined with fusion bonding of silicon, it is possible to realize true

three-dimensional structures as well. For example Figure 8.2 shows an SEM micrograph of a complex

capacitive force sensor designed to accept glass fibers in an etched v-groove. In this section, we will review

the fundamental relationships used in the initial designs of such electromechanical systems.

8.2.1 Beams

Microcantilevers are used in surface micromachined electrostatic switches, as “cantilever tip” for scanning

probe microscopy (SPM) and in myriad of sensors, based on vibrating cantilevers. The majority of the

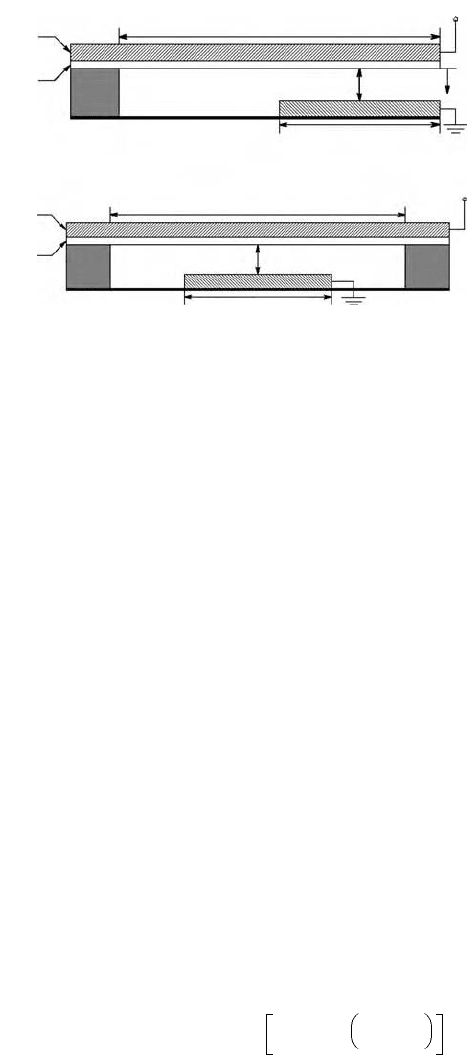
surface micromachined beams fall into two cases—cantilever beams and bridges. Figure 8.3 illustrates a

two-layer cantilever beam (Figure 8.3a) and a bridge (Figure 8.3b). The elastic force required to produce

deflection d at the tip of the cantilever beam, or at the center of the bridge, is given by

elast

= K

eff

d (8.22)

where

(8.23)

are the effective spring constants of the composite beams for cantilever and bridge beams, respectively.

The effective stiffness of the beam in both cases can be calculated from

(8.24)

FIGURE 8.2 Capacitive force sensor using 3D micromachining.

113

, d

223

, d

333

, d

232

322

, d

131

313

, d

123

213

===

eff

24 EI()

eff

/5()6 ll

–()l

12 ll

–()

8 ll

–()

++ +

----------------------------------------------------------------------------------------------------------

= and K

eff

360 EI()

eff

30l

45l– l

5 l

/l()– 3l

-------------------------------------------------------------

EI()

eff

-------------

+()

4 E

+()

--------------------------------------------

++=

9258_C008.fm Page 6 Tuesday, October 9, 2007 9:01 PM

Structures and Materials 8-7

where w is the width of the beam, t

the thickness of the top beam, t

the thickness of insulating layer

(silicon oxide, silicon nitride), l the length of the beam, l

the length of fixed electrode, E

the Young’s

modulus of the top layer, E

the Young’s modulus of insulating layer.

8.2.2 Torsional Springs

Torsion of beams is used primarily in rotating structures such as micromirrors for optical scanning, or

projection displays. The micromirror array developed by Texas Instruments for example uses polycrys-

talline silicon beams as hinges of the micromirror plate.

The torsion problems can be solved in a closed form for beams with elliptical or triangular cross sections

(Mendleson 1968). In the case of an elliptical cross section, the moment required to produce an angular

twist (angle or rotation per unit length of the beam)

[rad/m] is equal to

(8.25)

where G is the elastic shear modulus, and a and b are the lengths of the two semi-axes of the ellipse. The

maximum shear stress in this case is

(8.26)

The torsional stiffness of rectangular cross-section beams can be obtained in terms of infinite power

series (Hopkins 1987). If the cross-section has dimension a × b, b < a, the first three term of this series

result in an equation similar to 8.25

M = 2KG

, where (8.27)

8.2.3 Thin Plates

Pressure sensors are one of the most popular electromechanical transducers. The basic structure used to

convert mechanical pressure into electrical signal is a thin plate subjected to a pressure differential.

Piezoresistive gauges are used to convert the strain in the membrane into change of resistance, which is

FIGURE 8.3 Surface micromachined beams: (a) Two-layer composite beam with electrostatic actuation; (b) two-

layer composite bridge with electrostatic actuation.

, t

(a)

(b)

----------------

max

-------------------

, ab>=

Kab

-- 0.21

12a

----------–– .=

9258_C008.fm Page 7 Tuesday, October 9, 2007 9:01 PM

8-8 Mechatronic Systems, Sensors, and Actuators

read out using a conventional resistive bridge circuit. The initial pressure sensors were fabricated via

anisotropic etching of silicon, which results in a rectangular diaphragm. Figure 8.4 shows a thin-plate,

subjected to normal pressure q, resulting in out-of-plane displacement w(x, y). The equilibrium condition

for w(x, y) is given by the thin plate theory (Timoshenko 1959):

(8.28)

where D = Eh

/12(1 −

) is the flexural rigidity, E is the Young’s modulus,

is the Poisson ratio, and h

is the thickness of the plate. The edge-moments (moments per unit length of the edge) and the small

strains are

(8.29)

Using (8.29), one can calculate the maximum strains occurring at the top and bottom faces of the plate

in terms of the edge-moments:

(8.30)

In the case of a pressure sensor with a diaphragm subjected to a uniform pressure, the boundary conditions

are built-in edges: w = 0,

∂

x = 0 at x = ±a/2 and w = 0,

∂

y = 0 at y = ±b/2, where the diaphragm

has lateral dimensions a × b. The solution of this problem has been obtained by Evans (1939), showing

that the maximum strains are at the center of the edges. The values of the edge-moments and the

displacement of the center of plate are listed in Table 8.2.

TABLE 8.2 Deflection and Bending Moments of Clamped Plate under Uniform Load q

b/aW(x = 0, y = 0) M

(x = a/2, y = 0) M

(x = 0, y = b/2) M

(x = 0, y = 0) M

(x = 0, y = 0)

1 0.00126qa

/D −0.0513qa

−0.0513qa

0.0231qa

1.5 0.00220qa

/D −0.0757qa

−0.0570qa

0.0368qa

0.0203qa

2 0.00254qa

/D −0.0829qa

−0.0571qa

0.0412qa

0.0158qa

∞ 0.00260qa

/D −0.0833qa

−0.0571qa

0.0417qa

0.0125qa

Source: Evans 1939.

FIGURE 8.4 Thin plate subjected to positive pressure q.

∂

---------

∂

----------------

∂

---------

----

x, y() D

∂

---------

∂

---------

–

,–=

x, y() D

∂

---------

∂

---------

–

,–=

x, y()D(1

)

∂

x∂y

------------

– ,=

x, y, z()z

∂

---------

–=

x, y, z()z

∂

---------

–=

x, y, z()z

∂

------------

–=

max

x, y, z()

12z

--------

–()

z=h

--------

–()==

max

x, y, z()

12z

--------

–()

z=h

--------

–()==

9258_C008.fm Page 8 Tuesday, October 9, 2007 9:01 PM

Structures and Materials 8-9

8.3 Vibration and Modal Analysis

As mentioned earlier, the time response of a continuum structure requires the solution of Equation 8.10

with the acceleration terms present. For linear systems this solution can be represented by an infinite

superposition of characteristic functions (modes). Associated with each such mode is also a characteristic

number (eigenvalue) determining the time response of the mode. The analysis of these modes is called

modal analysis and has a central role in the design of resonant cantilever sensors, flapping wings for

micro-air-vehicles (MAVs) and micromirrors, used in laser scanners and projection systems. In the case

of a cantilever beam, the flexural displacements are described by a fourth-order differential equation

(8.31)

where I is the moment of inertia, E is the Young’s modulus,

is the density, and A is the area of the cross

section. When the thickness of the cantilever is much smaller than the width, E should be replaced by

the reduced Young’s modulus E

= E/(1 −

). For a rectangular cross section, (8.31) is reduced to

(8.32)

where h is the thickness of the beam. The solution of (8.32) can be written in terms of an infinite series

of characteristic functions representing the individual vibration modes

(8.33)

where the characteristic functions Φ

are expressed with the four Rayleigh functions S, T, U, and V:

(8.34)

The coefficients a

, b

, c

, d

, and

are determined from the boundary and initial conditions of (8.34).

For a cantilever beam with a fixed end at x = 0 and a free end at x = L, the boundary conditions are

(8.35)

Since (8.35) are to be satisfied by each of the functions Φ

, it follows that a

= 0, b

= 0 and

cosh(

L)cos(

L) = −1 (8.36)

-------

∂

wx, t()

∂

-----------------------

∂

wx, t()

∂

-----------------------

+ 0=

---------

∂

wx, t()

∂

-----------------------

∂

wx, t()

∂

-----------------------

+ 0=

w Φ

x()

+()sin

i=1

∞

x()b

x()c

x()d

x()+++=

Sx()

xxcos+cosh(), Tx()

xxsin+sinh()==

Ux()

xxcos–cosh(), Vx()

xxsin–sinh(),

-------

===

w 0, t()0,

∂

wL, t()

∂

-----------------------

0==

∂

w 0, t()

∂

--------------------

∂

wL, t()

∂

-----------------------

0==

9258_C008.fm Page 9 Tuesday, October 9, 2007 9:01 PM

8-10 Mechatronic Systems, Sensors, and Actuators

From this transcendental equation the

’s and the circular frequencies

are determined (Butt et al.

1995).

(8.37)

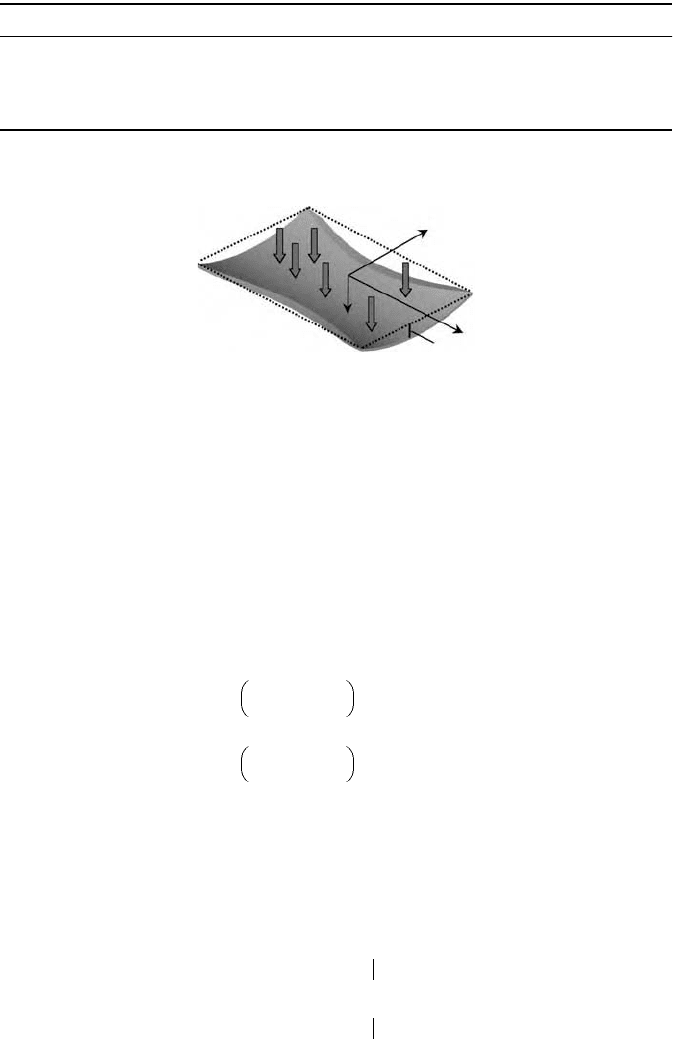
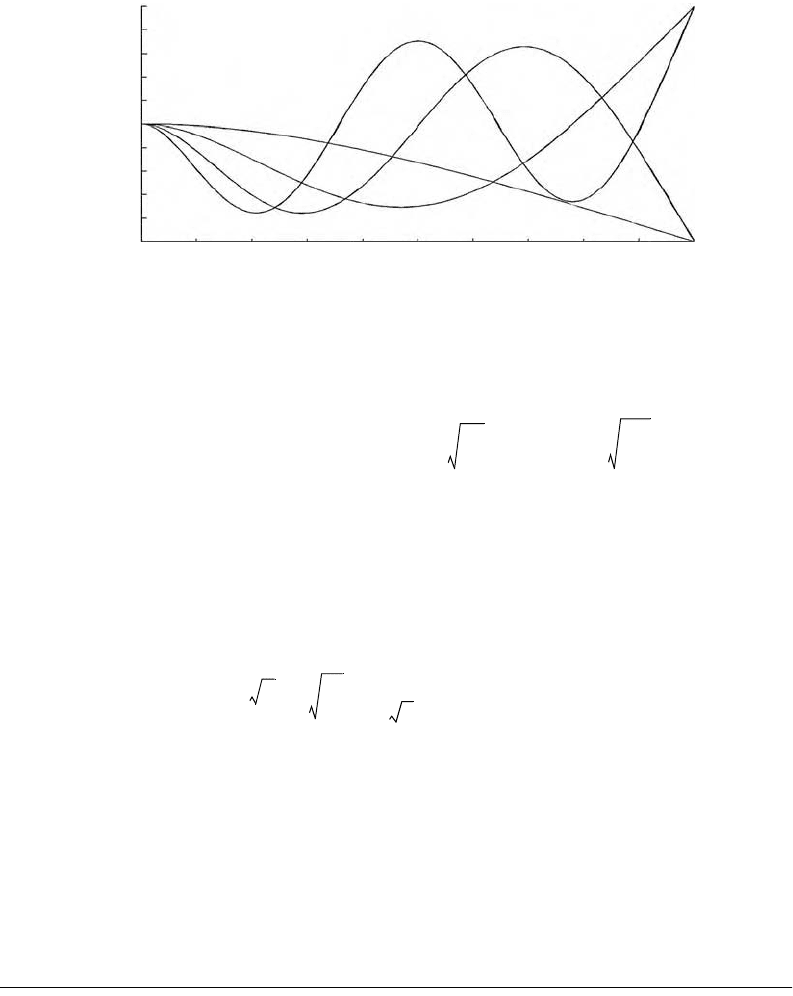
Figure 8.5 shows the first four vibrational modes of the cantilever. An important result of the modal

analysis is the calculation of the amplitude of thermal vibrations of cantilevers. As the size of the

cantilevers is reduced to nanometer scale, the energy of random thermal excitations becomes comparable

with the energy of the individual vibration modes. This effect leads to a thermal noise in nanocantilevers.

Using the equipartition theorem (Butt et al. 1995) showed that the root mean square of the amplitude

of the tip of such cantilever is

(8.38)

Similar analysis can be performed on vibrations of thin plates such as micromirrors. The free lateral

vibrations of such a plate are described by

(8.39)

The interested reader is referred to Timoshenko (1959) for further details on vibrations of plates.

8.4 Buckling Analysis

Structural instability can occur due to material failure, For example, plastic flow or fracture, or it can

also occur due to large changes in the geometry of the structure (e.g., buckling, wrinkling, or collapse).

The latter is the scope of this section. When short columns are subjected to a compressive load, the stress

in the cross section is considered uniform. Thus for short columns, failure will occur when the plastic

yield stress of the material is reached. In the case of long and slender beams under compression, due to

manufacturing imperfections, the applied load or the column will have some eccentricity. As a result the

force will develop a bending moment proportional to the eccentricity, resulting in additional lateral

deflection. While for small loads the lateral displacement will reach equilibrium, above certain critical

FIGURE 8.5 First four vibration modes of a cantilever beam.

0.8

0.6

0.4

0.2

–0.2

–0.4

–0.6

–0.8

–1

0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1

2i 1–()

----------------------

≅

2i 1–()

--------------------------

-------

2i 1–()

--------------------------

---------==

------

0.64 Å

---------------

, K

Ewh

------------

== =

∂

wx, y, t()

∂

-----------------------------

∂

wx, y, t()

∂

-----------------------------

∂

wx, y, t()

∂

-----------------------------

------

∂

wx, y, t()

∂

-----------------------------

–=

9258_C008.fm Page 10 Tuesday, October 9, 2007 9:01 PM

Structures and Materials 8-11

load, the beam will be unable to withstand the bending moment and will collapse. Consider the beam

in Figure 8.5, subjected to load F with eccentricity e, resulting in lateral displacement of the tip

According to the beam bending equation

(8.40)

where the boundary conditions are w(0) = 0,

∂

x |

x=0

= 0. The corresponding solution is

(8.41)

From w(L) =

one has

= e(1/coskL − 1), where k = . This solution looses stability when

grows

out of bound, that is, when cos kL = 0, or kL = (2n + 1)

/2. From this condition the smallest critical load is

(8.42)

The above analysis and Equation 8.42 were developed by Euler. Similar conditions can be derived for

other types of beam supports. A general formula for the critical load can be written as

(8.43)

where several values of the coefficient K are given in Table 8.3.

8.5 Transducers

Transducers are devices capable of converting one type of energy into another. If the output energy is

mechanical work the transducer is called an actuator. The rest of the transducers are called sensors,

although in most cases, a mechanical transducer can also be a sensor and vice versa. For example the

capacitive transducer can be used as an actuator or position sensor. In this section the most common

actuators used in micromechatronics are reviewed.

8.5.1 Electrostatic Transducers

The electrostatic transducers fall into two main categories—parallel plate electrodes and interdigitated

comb electrodes. In applications where relatively large capacitance change or force is required, the parallel

plate configuration is preferred. Conversely, larger displacements with linear force/displacement charac-

teristics can be achieved with comb drives at the expense of reduced force. Parallel plate actuators are

used in electrostatic micro-switches as illustrated in Figure 8.1. In this case the electrodes form a parallel

plate capacitor and the force is described by

(8.44)

where A is the area of overlap between the two electrodes; t

is the thickness of insulating layer (silicon

dioxide, silicon nitride); l

is the length of fixed electrode;

is the relative permittivity of insulating layer;

V is the applied voltage; d

is the initial separation between the capacitor plates; and d is downward

TABL E 8 .3 Critical Load Coefficients

End Conditions

One end built-in, other free

Both ends built-in Pin-joints at both ends

K coefficient 1/4 4 1

∂

---------

ew++()==

+()1 IE/Fx()cos–[]=

IE/F

IE/4L

IE/L

elec

2 t

d–()+[]

-------------------------------------------

9258_C008.fm Page 11 Tuesday, October 9, 2007 9:01 PM