Bhattacharjee J.K., Bhattacharyya S. Non-Linear Dynamics Near and Far from Equilibrium
Подождите немного. Документ загружается.

50 2 Models of Dynamics
2.7 Turbulence
In this section, we discuss the classic problem in nonlinear dynamics - turbulence
in fluids. The velocity field of a fluid satisfies Navier-Stokes equation which reads
∂ v
∂t
+(v.
∇)v =−
∇P
ρ
+ν∇
2
v (2.7.1)
with
∇.v =0 (2.7.2)
for an incompressible flow. The above equation needs boundary conditions and ini-
tial conditions. If v =0 at infinite distances or bounding surface, then Eqns.(2.7.1)
and (2.7.2) lead to
∂
∂t
1
2
v
2
d
3
r =−ν
∂v
i
∂x
j
∂v
i
∂x
j
d
3
r ≤0 (2.7.3)
Thus, unless there is an external force the motion ultimately ceases. For maintained
turbulence there must exist an external force such that
f.vd
3
r =ν
∂v
i
∂x
j
∂v
i
∂x
j
d
3
r (2.7.4)
This makes possible a steady state. We will assume that the external force puts
energy per unit mass into the system at the rate
=
f.vd
3
r.
We now turn to a complication exhibited by Eq.(2.7.1). If the nonlinear term dom-
inates (which it does if R
e
=
vL
ν
1, where v is a characteristic velocity and L a
characteristic length), the solutions of Eq.(2.7.1) are chaotic. This means that they
are sensitive to initial conditions and that, in turn, means that talking about v(r,t)is
not sensible. One has to talk about average values. One has to prescribe how to take
the averages. One way would be to consider an ensemble where different realiza-
tions correspond to different initial conditions and averaging over the realizations
gives v(r,t). The specification of the velocity field now requires the knowledge of
all the correlation functions - the two point correlation function v(x +r,t)v(x,t)
and the correlation of all the composite operators e.g v
2
(x +r,t)v
2
(x,t)etc. The
problem of turbulence thus becomes a problem in statistical mechanics. Calculat-
ing correlation functions is facilitated by having a stochastic differential equation,
as we have had in all our previous examples. To set up a Langevin equation in this
case, we split the velocity field into a mean part and a fluctuation
v(r,t)=v(r,t)+u(r,t)=
V(r,t)+u(r,t) (2.7.5)
2.7 Turbulence 51
Substituting Eq.(2.7.5) into Eq.(2.7.1)
∂
V
∂t
+
∂ u
∂t
+(
V.
∇)
V +(
V.
∇)u +(u.
∇)
V +(u.
∇)u =−
∇P
ρ
+ν∇
2
V +ν∇
2
u
(2.7.6)
If we now consider ensemble averages, then
∂
V
∂t
+(
V.
∇)
V +(u.
∇)u=−
∇P
ρ
+ν∇
2
V (2.7.7)
This transforms Eq.(2.7.6) to
∂ u
∂t
+(u.
∇)u =−
∇P
ρ
+ν∇
2
u +
T (2.7.8)
where
T =−(
V.
∇)u −(u.
∇)
V −(u.
∇)u+
∇P
ρ
(2.7.9)
The force
T includes the interaction between the mean flow and the fluctuation
and thus contains the mechanism for transferring energy into the fluctuating field.
In Eq.(2.7.8) for the fluctuating field, we will replace the force
T by a fluctuating
force
f which is operative mainly at the large distance scales. Thus,
∂ u
∂t
+(u.
∇)u =−
∇P
ρ
+ν∇
2
u +
f
with
∇.v = 0 (2.7.10)
The random force f will exhibit spatial correlation since it will be operative mainly
at the large distance scales and we take
f
α
(r
1
,t
1
)f
β
(r
2
,t
2
)=D
0
P
αβ
|r
1
−r
2
|
y−4
δ(t
2
−t
1
) (2.7.11)
where P
αβ
is the projection operator
P
αβ
=δ
αβ
−
∂
α
∂
β
∇
2
(2.7.12)
It is more usual to write the above in momentum space, where for the Fourier
component u(
k, t), we have the equation of motion in the form
∂
∂t
u
α
(
k, t) =
p
M
αβγ
(k) u
β
( p) u
γ
(
k −p) −νk
2
u
α
+f
α
(2.7.13)

52 2 Models of Dynamics
where
M
αβγ
(k) =i[k
β
P
αγ
(k) +k
γ
P
αβ
(k)] (2.7.14)
with the projection operator
P
αβ
(k) =δ
αβ
−
k
α
k
β
k
2
(2.7.15)
and the noise correlation
f
α
(
k
1
,t
1
)f
β
(
k
2
,t
2
)=
D
0
k
D−4+y
P
αβ
(k) δ(
k
1
+
k
2
)δ(t
2
−t
1
) (2.7.16)
It is the properties of Eqns.(2.7.13) - (2.7.16), that we will be investigating in
Chapter 8. Here we discuss what is the phenomenology that one would like to
reproduce.
The phenomenology of homogeneous isotropic turbulence was formulated by
Kolmogorovmore than fifty years ago. The important thing to do first is to recognize
the existence of three different regimes:
• i) a long wavelength regime, where the energy needed to maintain the turbu-
lence is inserted. The wave vectors in this regime are of the order of L
−1
, where
L is the characteristic size of the system.
• ii) a short wave length regime, where the energy inserted by the stirring forces
is dissipated by molecular forces. This length scale is formed out of the en-
ergy input rate and the molecular viscosity ν and is given by k
−1
D
, where
k
D
=(/ν
3
)
1/4
. The short wavelength region corresponds to wavenumbers
k ≥O(k
D
).
• iii) an inertial range, corresponding to wavenumbers k such that
1
L
k k
D
.
In this range of wavelengths, the boundaries and the energyinput mechanism or
the dissipative mechanisms and molecular viscosity do not affect the dynamics
which is consequently expected to be universal. In this inertial range the energy
cascades from one scale to another at the constant rate . We note that k
D
L =
(/ν
3
)
1/4
L ∼(
VL
ν
)
3/4
, implying a large inertial range for Re 1.
The Kolmogorov phenomenology deals with the supposedly universal inertial
range, where it predicts the correlation functions by a dimensional analysis. The
two point structure factor,
C(k)=v(
k, t)v(−
k, t) (2.7.17)
is the most commonly investigated one. From C(k), we construct the energy spec-
trum by the relation
E =
C(k)d
3
k =
E(k)dk (2.7.18)

2.7 Turbulence 53
In the above E is the total energy per unit mass with dimensions L
2
/T
2
. The
quantity E(k) is called the energy spectrum and has the dimension E(k)∼L
3
/T
2
.
The universality of the inertial range requires that E(k) needs to be determined by
and k alone and dimensional analysis immediately yields
E(k) =C
k
2/3
k
−5/3
(2.7.19)
where C
k
is a universal dimensionless constant. In coordinate space, this implies
that the two-point function has the scaling behaviour
[v(x +r)−v(x)]
2
=C
2
2/3
r
2/3
(2.7.20)
Can one actually probe the correlation function of Eq.(2.7.20) or equivalently the
energy spectrum of Eq.(2.7.19)? Unlike the accurate measurements that can be
carried out in critical phenomena, the early measurements in turbulence that were
carried out as late as the nineteen seventies, have a certain real world charm. The
first requirement is high Reynold’s number which we have already noticed is V L/ν
It is difficult to raise the Reynold’s number by increasing the speed. So one depends
on L. This is why the experiments are carried out in large water bodies like rivers or
sea inlets. The classic study of Grant, Stuart and Moillet was set near Vancouver
Island in Canada and the data were taken at different points of the Campbell river.
The data at different Reynolds number show the same slope for E(k) and the slope
is very close to −5/3.
For the higher order structure factors, this dimensional analysis leads to
|v(x +r)−v(x)|
p
=C
p
p/3
r
p/3
(2.7.21)
For the third order structure factor
|v(x +r)−v(x)|
3
=−
4
5
r (2.7.22)
The Kolmogorov spectrum was considered correct when confronted with early
experimental data, but later more accurate determination of the higher order struc-
ture factors showed clear cut departures from Kolmogorov scaling. The dissipation
rate which was assumed to be constant in the Kolmogorov picture, showed strong
intermittent fluctuations when looked at closely. This phenomenon is called in-
termittency. It shows up the departure from Kolmogorov scaling in a particularly
transparent matter. The energy dissipation rate can be written as v
2
/t ∼v
3
/L.If
we define a local dissipation rate (x) by
(x) =ν
over a ball
surrounding x
∂v
i
∂x
j
+
∂v
j
∂x
i
2
d
3
r (2.7.23)
54 2 Models of Dynamics
then the dissipation correlation function k
(r) is defined as
k
(r) =(x +r)(x) (2.7.24)
With ∼v
3
/L, the Kolmogorov scaling of Eq.(2.7.21) would imply k
(r) is in-
dependent of r. Consequently with
k
(r) ∼r
−µ
(2.7.25)
where µ is called the intermittency exponent, Kolmogorov scaling gives µ =0.
A non-zero value of µ is a very clear indication of departure from Kolmogorov
scaling. In general, taking the intermittency phenomenology into account, we have
|v(x +r)−v(x)|
p
∼r
ζ
p
(2.7.26)
where ζ
p
=p/3. The departure of ζ
p
from p/3 is linkedto the existence of coherent
structures.
The coherent structures have of late brought interest back into the turbulence
exhibited by the forced Burgers equation. Burgers equation, often studied in one
dimension, gives the equation of motion for the velocity as
∂u
∂t
+u
∂u
∂x
=ν
∂
2
u
∂x
2
(2.7.27)
The coherent structures in this equation are shocks and have been well studied.
The forced Burgers equation acquires the form
∂u
∂t
+u
∂u
∂x
= ν
∂
2
u
∂x
2
+f
with f(x
1
,t
1
)f (x
2
,t
2
)=−2D
0
∇
2
δ(x
1
−x
2
)δ(t
1
−t
2
) (2.7.28)
Interestingly enough, the KPZ equation that we discussed before can be cast in
the Burgers equation by the transformation u =
∂h
∂x
. This can be seen by taking the
gradient in Eq(2.7.27) and setting u =
∇h.Weget
∂ u
∂t
= ∇
2
u +λ
∇u
2
+
∇f
= ∇
2
u +λ
∇u
2
+
ξ (2.7.29)
where
ξ
α
(x
1
,t
1
)ξ
β
(x
2
,t
2
)=−2D
0
∇
2
δ(x
1
−x
2
)δ(t
1
−t
2
)δ
αβ
(2.7.30)
In D =1, this is the same as Eq.(2.7.28).

2.7 Turbulence 55
References
Langevin and Fokker-Planck Dynamics
1. H. Risken Fokker-Planck Equation Springer-Verlag, Berlin. (1984)
2. R. Dorfman, Introduction to Chaos in Non Equilibrium Statistical Mechanics Cam-
bridge University Press, New York. (1999)
Dynamical Critical Phenomena
1. P. C. Hohenberg and B. I. Halperin, Rev. Mod. Phys. 49 435 (1977)
2. P.M. Chaikin and T.C. Lubensky, Principles of Condensed Matter Physics. Cambridge
University Press. (1995)
3. A. Onuki, Phase Transition Dynamics. Cambridge University Press, New York (1998)
Systems Far From Equilibrium
1. A. J. Bray, “Theory of Phase Ordering Kinetics” Adv. in Phys. 43 357 (1994)
Growth Models
1. J. Krug and H. Spohn, Solids Far From Equilibrium ed. C. Godr
´
eche, Cambridge
University Press, Cambridge (1990)
2. T. Halpin-Healy and Y. C. Zhang Phys. Rep. 254 215 (1995).
3. A. L. Barabasi and H. E. Stanley Fractal Concepts in Surface Growth Cambridge
University Press, Cambridge (1995)
Turbulence
1. H. Tennekes and J. L. Lumley, A First Course in Turbulence M.I.T. Press, Cam-
bridge(MA) (1972)
2. U. Frisch, Turbulence Cambridge University Press, New York (1996)
Antiferromagnets
1. A. Tucciarone et.al. Phys.Rev.B 4 3204 (1971) (For experiment
)
2. R. Freedman and G. F. Mazenko Phys. Rev. Letts. 34 1575 (1975), Phys. Rev. B13 4967
(1976), Phys. Rev. B18 2281 (1978) (For theory)
Fluids
1. K. Kawasaki Ann. Phys. 61 1 (1970) (For theory)
2. H. L. Swinney and D. L. Henry, Phys. Rev. A8 2486 (1973) (For experiment)
Ferromagnet
1. P. Resibois and C. Piette, Phys. Rev. Lett. 24 514 (1970) (For theory)
2. O.W. Dietrich, J. Als-Nielsen and L. Passell, Phys. Rev. B14 4923 (1976)
3
The Renormalization Group
3.1 Introduction
Critical phenomena had posed a challenge for a long time since it is an intrinsically
strong coupling problem where traditional perturbation techniques did not work.
The difficulty is enshrined in the infinite correlation length at the critical point. The
renormalization group (RG) is a technique that is specifically designed to lead to an
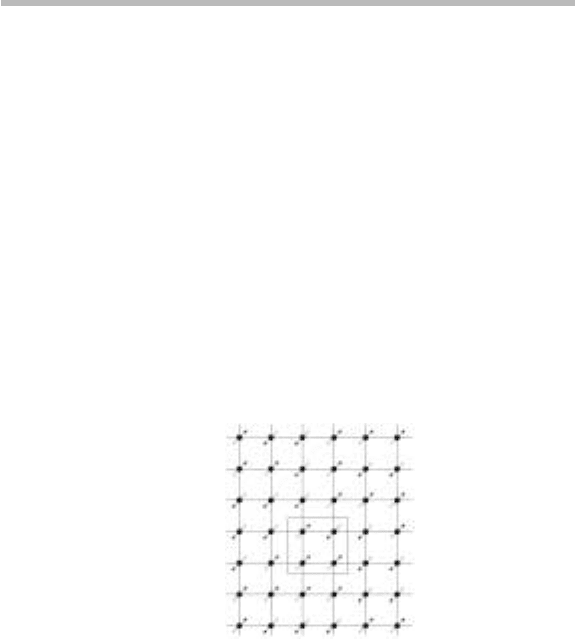
infinite correlation length. The idea is best set out using the Kadanoff construction
in the two dimensional Ising model shown in Fig. 3.1.
Figure 3.1. Making blocks of Ising spins
The Hamiltonian for the two dimensional Ising model is given by
H =−J
S
i,j
S
i+1,j +1
(3.1.1)
where S
i,j
is the spin at the lattice site (i, j). The idea is to bunch together some
spins and form a new spin (the spins shown inside the dotted square in Fig. 3.1 and
58 3 The Renormalization Group
form an effective lattice, where the lattice spacing is double the previous lattice
spacing. As the spins change to an effective spin S
i,j
, the coupling constant changes
toanewJ
, such that
J
=f(J) (3.1.2)
The correlation length, which is ξ(J) in terms of the lattice spacing undergoes a
transformation when the spin regrouping occurs. The new correlation length ξ(J
)
will clearly be half of the previous correlation length (since the lattice spacing is
doubled) and we have
ξ(J
) =
1
2
ξ(J) (3.1.3)
One can repeat this transformation and the important question is what will happen
as the transformation is repeated again and again. There is the possibility that
ultimately we will reach a fixed point J
∗
, where
J
∗
=f(J
∗
) (3.1.4)
and further regrouping does not change J anymore. If this occurs, then Eq.(3.1.3)
tells us that ξ =∞or 0. Thus this transformation is designed to lead to an infinite
correlation length, if the fixed point is arrived at and this corresponds to the critical
point. If, we examine Eq.(3.1.2) near the fixed point J
∗
,wefind
J
= J
∗
+
∂f
∂J
J =J
∗
(J −J
∗
) +...
or δJ
=
∂f
∂J
J =J
∗
δJ =λ
1
δJ (3.1.5)
If |λ
1
|> 1, then δJ grows on iteration and to reach criticality, we have to hold
δJ =0. Such a variable is called a relevant variable - the fixed point is unstable in
the direction of the relevant variable. Turning to Eq.(3.1.3), one can now write
ξ(δJ) = 2ξ(δJ
)
= 2ξ(λ
1
δJ)
= 2
2
ξ(λ
2
1
δJ)
.............
= 2
n
ξ(λ
n
1
δJ) (3.1.6)
Since λ
1
is greater than unity, we can choose n large enough so that however small
δJ may be (δJ is very small close to the critical point) λ
n
1
δJ ∼1. For this choice,
we have
n =
ln(
1
δJ
)
ln(λ
1
)
(3.1.7)
3.1 Introduction 59
with this value of n Eq.(3.1.6) becomes
ξ(δJ)=2
[ln(
1
δJ
)/ln(λ
1
)]
ξ(1) (3.1.8)
Now, ξ(1) is an ordinary number say ξ
0
and we have
ξ(δJ)=ξ
0
(δJ )
−
ln2
lnλ
1
=ξ
0
(δJ )
−ν
(3.1.9)
Since δJ is a relevant variable, which has to be zero at criticality, we identify δJ
with δT =
T −T
c
T
c
, and one arrives at
ξ(T −T
c
) = ξ
0
T −T
c
T
c
−ν
where ν =
ln2
lnλ
1
(3.1.10)
To get an actual value of ν, one must have a calculational scheme that will give
us the function f of Eq.(3.1.1). The simple steps that we have gone through have
yielded the scaling law of the first of Eq.(3.1.10) and illustrates the basic working
of the renormalization group.
In general, we would need to start out with a larger Hamiltonian, e.g. one
that would include four spin interaction, two spin interaction with next nearest
neighbour and so on. We denote the coupling constants of these various interactions
by the set {K}=(K
1
,K
2
.....). Under the scaling transformations described above
all the K
i
’s reach the fixed point values K
∗
i
- that is the entire Hamiltonian reaches
a fixed point. The transformation that we describe takes us from J, {K}→J
, {K
}
and in general J =f(J,{K}) and similarly for the set {K}. From the set J,{K}we
can form linear combinations {u
i
}, called scaling fields, such that when linearized
about the fixed point
δu
i
=λ
i
δu
i
(3.1.11)
for each i. For |λ
i
|> 1, we have a relevant variable that we have discussed before,
while for |λ
i
|< 1, the variables are irrelevant. Marginal variables correspond to
|λ
i
|=1. The existence of the irrelevant variables leads to universality. If one starts
out with a general Hamiltonian, then under the iterations described above, the
irrelevant variables die out and one ends up with the critical Hamiltonian where
only relevant variables matter. Thus a huge number of Hamiltonians end up giving
the same critical behaviour thus leading to universality.
We summarize the above discussion by repeating that a convenient way of de-
scribing critical phenomena is to set up a transformation which removes degrees
of freedom on the small scale (the lattice spacing is doubled in the transformation)
and focusses on the change in the coupling constants that this entails. The transfor-
mation is called a renormalization group transformation (RGT) - the Hamiltonian
after the transformation has the same structure as before (notice that the spins have
60 3 The Renormalization Group
to be rescaled as well so that they are always ±1) but with “renormalized” coupling
constants. In the next section, we will see how one implements this procedure for
continuous fields - our primary concern in this book.
3.2 Renormalization Group: General Framework
In this section, we show how to implement the RGTs for a continuum model.
The order parameter field φ(x) (we will restrict ourselves to a scalar field for the
present) determines the free energy density which in turn determines the probability
distribution of the field φ(x). The free energy density is given, as is well known, by
the Ginzburg - Landau free energy density of Eq.(2.3.16). We will drop the quartic
term to begin with and consider the Gaussian model with the free energy
F =
d
D
x
1
2
m
2
φ
2
+
1
2
(
∇φ)
2
(3.2.1)
which can be exactly solved.
We will first discuss the method of implementing the transformation procedure.
This discussion will be general and independent of the model. To appreciate the
existence of all scales in the field φ(x), we carry out the Fourier decomposition
φ(x)=
1
(2π)
D/2
φ(
k)e
i
k.x
d
D
k (3.2.2)
The momentum integration is essentially over the entire momentum range - the
cutoff corresponds to inverse lattice spacing. Thus φ(x) is composed of Fourier
components φ(k), where k can range from 0 to . This shows the existence of
all length scales (k
−1
corresponds to length). The Fourier components with low
values of k are called the slow variables while the components with high values of k
are called the fast variables. Quantitatively we call all components with 0 <k<
b
(b>1)theslowvariablesand the components with
b
<k<arethe fast variables.
The slow variables will be denoted by φ
<
(k) and the fast variables by φ
>
(k). The
first step of the RGT is now clear - integrate out the fast variables and write the free
energy as a function of φ
<
(k). But one is not ready to compare coupling constants
yet - the new Hamiltonian (a term which will be used interchangeably with free
energy) has φ(k) with k ranging only up to
b
. The previous Hamiltonian had
φ(k) with k ranging up to . To be able to compare the two Hamiltonians, the
momentum after the elimination of the degrees of freedom has to go up to . This
requires scaling all momenta by a factor b, i.e. k →k
=bk. In terms of k
, the
range is now 0 to . We now need to rescale the field φ(x), so that the coefficient
of (
∇φ)
2
remains fixed at 1/2. This ensures that the new Hamiltonian does not
become the old Hamiltonian by a mere rescaling of φ. At this point, one is ready
to compare the coupling constants. Thus the three steps of the RGT are
