Bhattacharjee J.K., Bhattacharyya S. Non-Linear Dynamics Near and Far from Equilibrium
Подождите немного. Документ загружается.

7.6 Growth with Surface Diffusion 213
By looking at the correlation function, we find that the change δN in the coefficient
of the noise correlation is
δN =
λ
2
n
2
2κ
3
d
D
p
p
4
|
k −p|
4
[p.(
k −p]
2
p
4
+|
k −p|
4
(7.6.29)
From the structure of Eq.(7.6.28), it is clear that as b →1, a perturbative change
occurs in κ for D =4 and we have
δκ =
λ
2
N
κ
2
6
D
−1
ln b (7.6.30)
The noise correlator on the other hand is not affected and as in the KPZ equation
the vertex correction is assumed to be unaffected (this is not exactly true, it is true to
a very good approximation because the coefficient of the correction term happens
to be very small). We now need to restore the cutoff to by carrying out a scale
transformation. The result of that can be read off from Eq.(7.6.12) and we find
dκ
dl
= κ
z −4 +
λ
2
N
κ
3
S
D
6 −D
D
dN
dl
= N[z −2α −D]
dλ
dl
= λ[z +α −4] (7.6.31)
It should be noted that unlike the first of Eq.(7.6.31) above the two following
equations are exact. The diagrammatic expansion in powers of λ do not contribute
to N at all and very insignificantly to λ and hence flow is determined by na
¨
ıve
dimensional scaling. Hence we have the exact ‘results’
α +z = 4
2α −z =−D
which lead to α =
1
3
(4 −D) and z =
1
3
(8 +D) as had been obtained before
(Eq.(7.6.16)) by a completely different argument.
It is instructive to look at the problem from the self-consistent mode coupling
point of view as well. One proceeds exactly as in the case of the KPZ equation and
obtains in analogy with Eq.(7.5.4)
G
−1
(k, ω) =−iω +κk
4
+(k,ω) (7.6.32)
where
(k, ω) = 4λ
2
k
2
d
D
p
(2π)
D
dω
1
2π
p
2
[p.(
k −p)][
k.(
k −p]
×G( p, ω
1
)C(
k −p, ω −ω
1
) (7.6.33)

214 7 Surface Growth
Once again it is tacitly assumed that λ does not renormalize. We first enquire in
what situation will (k, ω) dominate κk
4
.If(k, ω) scales as k
z
, then a power
count of the R.H.S of Eq.(7.6.31) shows that the integral scales as k
8−2α−z
=k
4−α
.
Consequently, so long as α>0, (k, ω) will dominate k
4
.
Let us now explore the region of the integrand where p
k. In this approxi-
mation the integral is
k
2
G(
k) =
d
D
p
(2π)
D
dω
1
2π
(
k. p)
2
C(p
,ω−ω
)
∝ k
2
G(k)
d
D
p
(2π)
D
(
k. p)
2
(p
)
D+2α
= k
4
G(k)
C
D
(2π)
D
1
D
dp
p
2α−1
(7.6.34)
The integral diverges (ultraviolet i.e. short distance divergence because k is small)
if α ≥1, i.e. D ≤1. Consequently, the scaling laws that we have obtained will not
be valid is D ≤1.
How does this difficulty show up in the RG treatment of the model? If we
include higher order terms in the equation of motion, then we would have
˙
h =−κ∇
4
h +λ∇
2
(
∇h)
2
+λ
1
∇
2
(
∇h)
4
+...
+λ
n
∇
2
(
∇h)
2n
+........ +f (7.6.35)
If α =1, the dimensionality of all the operators (
∇h)
2n
become the same and hence
all the terms are potentially relevant in the RG sense for α>1 (i.e. D<1). It is
clear that we can no longer have α =1 at D=1. There is some indication that z=4 for
D=1 and that α =3/2. The difficulty that we see over here is interestingly enough
similar to the difficulty that one encounters in turbulence (see Chapter 8).
7.7 Discrete Models
The first of the discrete models for surface growth in the presence of diffusion
was introduced by Wolf and Villain and by Das Sarma and Tamborenea. The two
models differ in the sticking rules for atoms. We illustrate the action of the model
for a one dimensional substrate. If we imagine the interface at any instant as shown
in Fig. 7.5 The solid circles indicate the particles which have been deposited. The
randomly dropped new particle (open circle) has to decide which site it will settle
on. For this it looks at the nearest neighbor sites. If at least one of the nearest
neighbour sites is occupied it stays at the position at which it has landed. If none
of the nearest neighbours are occupied the particle will move. If only one nearest
neighbour site has its neighbouring site occupied, then the particle moves to that
nearest neighbour position. If both the nearest neighbours have neighbouring sites

7.7 Discrete Models 215
Figure 7.5. The growth rule in the DasSarma Tamborenea model
occupied or unoccupied, then the particle will move randomly to its left or right.
These are the rules for Das Sarma - Tamborenea model. In the Wolf-Villain model,
the particle moves to the site which has the largest number of nearest neighbours.
An unrealistic aspect of such growth models could be the formation of large local
slopes. The particles have the freedom to travel as far down as possible to maximize
the number of nearest neighbours. This generally does not happen in the growth
of crystals by Molecular Beam Epitaxy (MBE) which the discrete models are
supposed to mimic.
The numerical simulations study the width W of the interface which is the mean
square deviation form the average height
W(L,t)=[
1
N
N
i=1
(h
i
−
¯
h)
2
]
1/2
(7.7.1)
The expected scaling behaviour is W(L,t)∼L
α
f(
t
1/z
L
) with f(x)∼x
α
for small
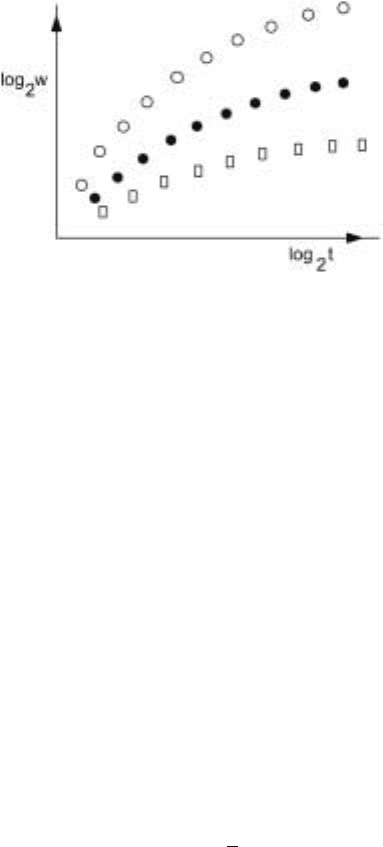
x and saturating for x 1. Consequently, a typical data set for the width appears
shown in Fig. 7.6 The small time measurement yields β =
α
z
0.365 for the Wolf-
Villain Model. The scaling of the saturation width with L gives α 1.4. These
results are in agreement with those obtained in Eq.(7.6.8) for the linear theory and
disagree with the results of the nonlinear theory expressed in Eq.(7.6.16). A two
dimensional generalizaton of the Wolf-Villain model yielded β 0.20 and α
0.66 in agreement with the nonlinear theory and disagreeing with the predictions
of the linear theory. The surprising result that the nonlinear theory works in D =2
and does not work in D =1 has to do with the fact that the nonlinear model that we
are working with cannot be trusted at D =1 where an infinite number of nonlinear
terms are equally important.
The unusual features associated with D =1 are further stressed by the work of
Krug who went on to study the distribution of step sizes. The step size is defined
216 7 Surface Growth
Figure 7.6. Interface width as a function of time in the DasSarma Tamborenea model
as the nearest neighbour height difference. The various moments of the step size
are defined as
σ
q
=|h(x +1) −h(x)|
q
1/q
(7.7.2)
which is clearly a local quantity. There is the width variable which is a global
quantity and the generalized width fluctuation moment W
q
is defined as
W
q
=(h −
¯
h)
q
1/q
(7.7.3)
The scaling of both sets of moments is in terms of a lateral correlation length ξ
which increases as t
1/z
, where t is the deposition time. This holds for small times
when ξ<<L, the system size. For ξ L, the scaling is controlled by L. The local
quantity σ
q
scales as ξ
α
q
where α
q
is an increasing function of q, while the global
W
q
scales as ξ
3/2
independent of q. The local and global regimes are connected
by the r-dependent correlation function
G
q
(r) =|h(x +r)−h(x)|
q
1/q
=ξ
α
q
r
ζ
q
f
q
(r/ξ ) (7.7.4)
The property of f
q
(x) is that for x →0 f
q
(x) tends to a constant, while for x →∞
f
q
(x) ∼x
−ζ
q
.Nowifr =1, x =1/xi →0 then f(x)→constant. Consequently,
G
q
(1) ∼ξ
α
q
.Ifr ξ , so that one is in the global regime, we have
G
q
(r ξ) ∼ ξ
α
q
r
ζ
q
(
ξ
r
)
ζ
q
=ξ
α
q
+ζ
q
= ξ
3/2
(7.7.5)
provided α
q
+ζ
q
=3/2. Thus ζ
q
becomes q dependent because of α
q
. The G
q
are
the analogues of the structure factors in turbulence and the nonlinear q-dependence
of ζ
q
is the signature of turbulence in this case. The step size distribution was
found to be non-Gaussian (a stretched exponential), once again in analogy with
intermittency in turbulence. A clear understanding of the basic issues here is still
not absolutely clear.

7.8 Growth Models with Correlated Noise 217
7.8 Growth Models with Correlated Noise
The KPZ model is certainly of great importance as a generic model for non equi-
librium phase transitions. However, one has not yet come up with an experimental
system that confirms the predictions of the KPZ model satisfactorily. This discrep-
ancy has motivated various modifications on the original model. First of all, one
may question the validity of the uncorrelated Gaussian noise. In a real system, the
noise could be correlated, non-gaussian or even quenched. Quite generally, in a
genuine non equilibrium system, the form of the noise correlations in an effective
Langevin type description is a crucial ingredient of the modelling. This is in con-
trast with equilibrium dynamics, where the functional form of the noise as well
as its strength are fixed via an Einstein relation which ensures that asymptotically
the probability distribution will be an equilibrium one. Uncorrelated white noise
is often a straightforward choice but the sensitivity and stability of the ensuing
results need to be carefully tested against modifications of the noise correlations.
The motivation is not to introduce a realistic growth model but to see how much
change can such perturbations cause over the KPZ results. We can focus on D =1.
In momentum space the model reads (compare Eqns.(7.4.1) and (7.4.2))
−iωh(
k, ω)=−νk
2
h(
k, ω)
−
λ
2
p,ω
1
p.(
k −p)h( p, ω
1
)h(
k −p, ω −ω
1
) +η(
k, ω) (7.8.1)
with
η(
k, ω)η(
k
,ω
)=
2N
k
2−β
δ(
k +
k
)δ(ω +ω
) (7.8.2)
In coordinate space, the noise correlation reads
η( x
1
,t
1
)η( x
2
,t
2
)=2N|x
1
−x
2
|
β−2−1
δ(t
1
−t
2
) (7.8.3)
In D-dimensional space the generalization would be
η( x
1
,t
1
)η( x
2
,t
2
)=2N|x
1
−x
2
|
β−2−D
δ(t
1
−t
2
) (7.8.4)
For β =2, we have the KPZ system, while for β<2 we have the generalized KPZ
system. The basic ingredients of the calculation are the Green’s function
δh(
k, ω)
δη(
k
,ω
)
1
δ(
k +
k
)δ(ω +ω
)
and the correlation function
h(
k, ω)h(
k
,ω
)
δ(
k +
k
)δ(ω +ω
)

218 7 Surface Growth
Dyson’s equation gives
G
−1
=−iω +νk
2
+(k,ω)
and the self-consistent scheme (or the spherical limit) gives
(k, ω)=λ
2
dp
2π
dω
2π
pk(k −p)
2
G(p, ω
)C(k −p, ω −ω
) (7.8.5)
The correlation function at the same order of accuracy is
C(k,ω) =|G(k, ω)|
2
2Nk
β−2
+λ
2
|G(k, ω)|
2
dp
2π
dω
2π
p
2
(k −p)
2
×C(p,ω
)C(k −p, ω −ω
) (7.8.6)
We note that a similar approximation for the KPZ equation with white noise has
been studied by Frey et.al, who not only considered the scaling behaviour but the
entire scaling function. We now make the following scaling Ansatz for (k, ω)
and C(k,ω):
(k, ω) = k
z
g
ω
k
z
(7.8.7)
C(k,ω) = k
−(1+2α+z)
f
ω
k
z
(7.8.8)
where z is the dynamic scaling exponent and α is the roughness exponent. The
self-energy (k, ω) will determine the relaxation rate in the long wavelength limit
if z<2. Similarly in Eq.(7.8.6) the noise strength 2N will not be renormalized (i.e.
acquire k,ω dependence) if the second term is no more singular than the first. If
the second term dominates in the long wavelength limit, Eq.(7.8.6) reduces to
C(k,ω) = λ
2
|G(k, ω)|
2
dp
2π
dω
2π
p
2
(k −p)
2
×C(p,ω
)C(k −p, ω −ω
) (7.8.9)
With G
−1
(k, ω) =−iω +(k, ω), we can solve for α and z between Eqns.(7.8.5)
and (7.8.9). This leads to α =1/2 and z =3/2.
On the other hand, if N is not renormalized, then Eq.(7.8.6) shows 2α =1 −
β +z and Eq.(7.8.5) leads to z =1 +
β
3
. We now need to settle when N will be
renormalized. This follows from a simple power count of Eq.(7.8.6). The second
term on the R.H.S dominates the first if z ≤1 +
β
3
. The dominance of the second
term implies z =3/2 and hence one will see z =3/2 for β ≥3/2.
We now check for the finiteness of (k, ω) as given by Eq.(7.8.5). There is no
divergence coming from the region where p>k. We next check the region p k,
and find
7.9 Growth models with Nonlocality 219
(k, 0) λ
2
k
2
G(k, 0)
dq
2π
dω
2π
q
2
C(q, ω
) (7.8.10)
(k, 0) λ
2
k
2
[(k)]
−1
dq
2π
q
2
N
q
3−2β/3
= λ
2
k
2
[(k)]
−1
N
dq
q
1−2β/3
(7.8.11)
For β>0, the integral converges and we have z =1 +β/3, but for β<0, the
integral needs to be cut off at a lower limit k
0
and hence (k) ∼kk
β/3
0
, giving z=1.
Thus,
z = 3/2,α=1/2, for 2 ≥β ≥3/2
z = 1 +β/3,α=1 −β/3, for 3/2 ≥β ≥0
z = 1, for β<0 (7.8.12)
These are precisely the results obtained by Medina et.al. For β<0, the dynamics
is governed by the sweeping of small-scale fluctuations by the large-scale ones.
We see that for a range of β around the KPZ value (i.e. if the spatial correlation
is weak) the KPZ value for z is obtained. In this case, we may safely say that the long
ranged nature of the noise correlation is an irrelevant perturbation. For the range
of β between 3/2 and 0, one settles on a result dominated by the noise correlation.
The surprising thing is that for β<0 Eq.(7.8.12) gives z =1 i.e. One has reached
the ballistic limit. However, the numerical work of Hayot and Jayaprakash which
verifiedthese predictions in the ranged 2 ≤β ≤0, showed that z =1 +β/3 holds for
β<0. This is once again reminiscent of Kolmogorov scaling in turbulence(z =2/3
there) and like the problem of turbulence this situation is not fully understood.
7.9 Growth models with Nonlocality
The fact that experiments never record a KPZ like exponent has given rise to modi-
fications in the deterministic part of the equation as well. Many of the experimental
situations involve complex processes which go beyond the idealization of the de-
position of non interacting particles. This could be especially true if medium or
fluctuation induced interactions interfere with the process as for example in sys-
tems involving proteins, colloids or latex particles. The major interaction that is
involved is long ranged hydrodynamic interaction. Can such interactions be rele-
vant to the roughness of the surface? Once again this question is akin to the one
asked in the last section where the issue was whether spatial correlation in the noise
could be a relevant perturbation.
The first question is how does one incorporate such interactions. The answer
proposed by Mukherji and Bhattacharjee is to view the gradient of the height as a
measure of the local density and incorporate the long range interaction by coupling
220 7 Surface Growth
the gradient at two different points. This means that the nonlinear term (
∇h)
2
in
the KPZ equation gets replaced by
d
D
r
∇h(r).
∇h(
r
)V (r −
r
).
The usual model Eq.(7.3.3) obtains if we set
V(r −
r
) =
λ
2
δ(r −
r
)
i.e. make the interaction absolutely short ranged. The proposed model is
˙
h(r,t)=ν∇
2
h +
1
2
d
D
r
∇h(r).
∇h(
r
)V (r −
r
) +η (7.9.1)
η(r,t)η(
r
,t
) =2Nδ(r −
r
)δ(t −t
) (7.9.2)
V(r −
r
) =λδ(r −
r
) +λ
1
|r −
r
|
ρ−D
(7.9.3)
The rescaling of r by b, t by b
z
and h by b
α
i.e.
r
=
r
b
t
=
t
b
z
h
=
h
b
α
leads to
˙
h
(
r
1
,t
) = νb
z−2
∇
2
h
+
λ
2
b
α+z−2
(
∇
h
)
2
+
λ
1
2
b
α+z−2+ρ
×
|
r
1
−
r
2
|
ρ−D
(
∇
h
(
r
1
)).(
∇
h
(
r
1
))d
D
r
2
+b
µ+z
η
(
r
1
,t
)
(7.9.4)
The coupling constant λ renormalizes according to λ
=λb
α+z−2
and the constant
λ
1
according to λ
1
=λ
1
b
α+z−2+ρ
. At the Gaussian fixed point, α =1 −
D
2
and
z =2, which means λ
=λb
1−
D
2
and λ
1
=λ
1
b
1−
D
2
+ρ
. Consequently, for D<2,λ is
relevant (as before) and the long range interaction is relevant for D<2(1 +ρ).For
2 <D<2(1 +ρ), only the long range interactions give a relevant perturbation for
ρ>0. Thus it is expected that if ρ>0, the roughening exponent will be determined
by the long-range-interaction-fixed-point and consequently the exponents α and z
will depend upon the parameter ρ. The complete picture can be obtained only if we

7.10 Roughening Transition 221
carry out a full RG transformation. We refer the reader to the original publication
for the details and end this section by quoting the flow equations in D =1
dν
dl
= ν
z −
2 +
NS
D
ν
3
˜
V(2)
˜
V(1)
(D −2) +3f(1)
4D
(7.9.5)
dN
dl
=
z −D −2α
N +
N
2
S
D
4ν
3
˜
V(2)
2
(7.9.6)
where
˜
V(k)=λ +λ
1
k
−ρ
is the Fourier transform of the interaction V(r
1
−r
2
). The
function f(x)is the logarithmic derivative
∂ ln
˜
V(k)
∂ ln k
evaluated at k =x. The analysis
of the fixed point structure of the above flow equations confirms the qualitative
statements made above.
7.10 Roughening Transition
The mechanism for roughening so far has been the random deposition process.
Crystal surfaces can, however, be rough under the equilibrium conditions. At low
temperatures, thermal fluctuations have no effect on the shape of the crystal with
all atoms remaining at their mean positions and the crystal planes looking quite
flat. As temperature increases, the probability of an atom breaking its bond with its
neighbours increases and atoms can hop onto neighbouring sites, causing roughness
on an atomic scale. One can expect a gradual transition to a rough morphology
with more atoms hopping from their positions and eventually causing the surface to
melt. This is true for the short range correlation but the long range correlation gets
broken much earlier. If one focuses on the long distance scales, then there exists
a critical temperature T
R
(much below the melting temperature) above which the
crystal facet is no longer smooth.
Fluctuating about the flat surface cause the surface area and hence the surface
energy to increase. The energy of a two dimensional surface is proportional to the
area and can be written as
H =ν
d
2
x
(1 +
∇h)
2
(7.10.1)
In the absence of height fluctuations (no local slopes)
∇h =0 and the flat surface
area is H
0
=νL
2
with ν being the ’surface tension’ or the stiffness of the surface.
If the gradient is not too large anywhere, we can expand and get
H =H
0
+
1
2
ν
d
2
x(
∇h)
2
+....... (7.10.2)
This Hamiltonian is the same as that of the EW model. The lesson from that
model is that the equilibrium (saturated i.e t L
z
) system is rough with the height

222 7 Surface Growth
correlation increasing logarithmically. This is independent of the length scale and
hence it cannot account for the roughening temperature.
The shortcoming of Eq.(7.10.2) can be seen from the fact that this energy
expression has continuous symmetry but the crystal has a discrete symmetry in
terms of the lattice spacing
a
0
. This can be incorporated by adding a periodic term
to Eq.(7.10.2) and writing the excess energy
δH =
1
2
ν(
∇h)
2
−V
0
cos
2π
a
0
d
2
x (7.10.3)
The equilibrium statistical mechanics of the system is done by writing down the
partition function
Z =
D[h]e
−
δH
k
B
T
(7.10.4)
The minimization of δH yields h constant from the first term in Eq.(7.10.3) which
requires
∇h =0 for a minimum. The second term is minimized if h is an integral
multiple of a
0
. Thus, the minimization of δH yields a flat surface which is the
mean field result. It is the fluctuation around this mean field value that needs to be
studied. This is done by Eq.(7.10.4) in the momentum space modes of h(r), which
yields
Z =
k=0
dh(k)e
−
1
2
[
k
νk
2
|h
k
|
2
+V(h
k
)]
(7.10.5)
One now writes down the RG flows for ν/T and V
0
/T by eliminating the modes
lying between /b and and then rescaling the coordinates and fields so that the
original cut off restored. These flow equations are quite difficult to derive but
can now be found in standard text books and review articles on critical phenomena.
It is customary to define the two variables
x =
2νa
2
0
πk
B
T
and y =
4πV
0
k
B
Tλ
2
(7.10.6)
and write the flow equations in terms of them as
dy
dl
= 2y
x −1
x
(7.10.7)
dx
dl
=
y
2
2x
F
2
x
(7.10.8)
where F(z) is a complicated function.
We note that Eq.(7.10.7) has a fixed point at x =1, corresponding to T =T
R
given by
