Benzoni-Gavage S., Serre D. Multi-dimensional Hyperbolic Partial Differential Equations: First-order Systems and Applications

Подождите немного. Документ загружается.

Shock waves 405

More generally, what can we say for Bethe–Weyl ﬂuids (of which Smith ﬂuids

are special cases) about the relationship between compressivity, the entropy

criterion (13.4.37) and the existence of a planar shock layer? An easy result

is the following.

Proposition 13.4 For Bethe–Weyl ﬂuids, dissipative shock layers converging

fast enough to their endstates, that is, heteroclinic orbits between hyperbolic ﬁxed

points of (13.4.42) with κ ≥ 0 and λ +2µ ≥ 0, satisfy the entropy criterion in

(13.4.37).

Proof For simplicity we denote by D the diﬀusion tensor

D := (λ ∇·u) I

+ µ ( ∇u +

(∇u)).

Along smooth solutions of (13.4.41), because of (13.1.1) the entropy per unit

volume S satisﬁes

∂

S + ∇·(S u)=

( ∇·( κ ∇T )+D : ∇u ) , (13.4.44)

which is reminiscent from (13.2.27), with additional terms due to dissipation.

Looking at travelling-wave solutions of (13.4.44) for which ρ (u − σ) ≡ j and

u ≡

∞

, we ﬁnd that

jTs



=(κT



)



+(λ +2µ)(u



)

(13.4.45)

along heteroclinic orbits of (13.4.42). (This equation can of course be obtained

directly from (13.4.42), using (13.1.1) in the form Ts



= e



+ pv



.) Integrating

(13.4.45) then yields, if the orbit converges suﬃciently fast towards its endstates,

j [s]=



+∞

−∞









(λ +2µ)(u



)



dξ, (13.4.46)

which is obviously non-negative. 

So, for Bethe–Weyl ﬂuids a reasonable admissibility criterion is the existence

of a shock layer, and under the additional condition (13.1.15), it is equivalent to

compressivity.

For other kinds of ﬂuids, like van der Waals ﬂuids below a critical temper-

ature, for instance, alternate admissibility criteria are needed. As a matter of

fact, on the one hand, it is well-known that dynamical phase transitions can

be compressive (condensation) but also expansive (vapourization). On the other

hand, layers for dynamical phase transitions, also called diﬀuse interfaces, cannot

be obtained from the purely dissipative system (13.4.41). Some dispersion has

to be added in connection with capillarity eﬀects. This approach was proposed

independently by Slemrod [196] and Truskinovsky [214] in the 1980s and led to

the so-called viscosity–capillarity criterion. We shall not enter into more detail

here (see [10]).

406 The Euler equations for real ﬂuids

The Liu criterion

Even with monotone isentropes, the admissibility of shocks is a tough problem

in lack of convexity (that is, without the assumption G > 0). It was investi-

gated in the 1970s by Tai Ping Liu [119] and independently by Ling Hsiao

and coworkers [224]. This led them to introduce what is now called the Liu

criterion and is a generalization of the Ole˘ınik criterion – originally introduced

for scalar conservation laws – formulated in terms of shock speeds along Hugoniot

loci.

Deﬁnition 13.3 (Liu) A discontinuity between W

and W

,ofspeedσ, satisﬁes

the Liu criterion if

for all W ∈ H (W

; W

), the part of the Hugoniot locus issued from W

and arriving at W

, the corresponding speed σ(W

; W) satisﬁes

σ = σ(W

; W

) ≤ σ(W

; W),

for all W ∈ H (W

; W

), the part of the Hugoniot locus issued from W

and arriving at W

, the corresponding speed σ(W

; W) satisﬁes

σ(W

; W) ≤ σ(W

; W

)=σ.

Using this criterion, we have the following result, analogous to Theorem 13.2

without the restriction G > 0.

Theorem 13.3 (Liu–Pego) For ﬂuids with positive pressure and temperature

satisfying

γ>0 ,δ>0 , Γ > 0,

all discontinuities satisfying Liu’s criterion admit purely viscous shock layers,

that is, with identically zero heat conductivity κ. If, additionally,

Γ ≥ δ,

this is true for arbitrary heat conductivity: discontinuities satisfying Liu’s crite-

rion admit general shock layers.

We refer to the original papers [119, 157] for the proof. The ﬁrst part was

proved by Liu [119], and the second one by Pego [157]. To help the reader with

the way those authors stated their assumptions, we just point out the following

equivalences, where the right-hand inequalities are precisely the ones required by

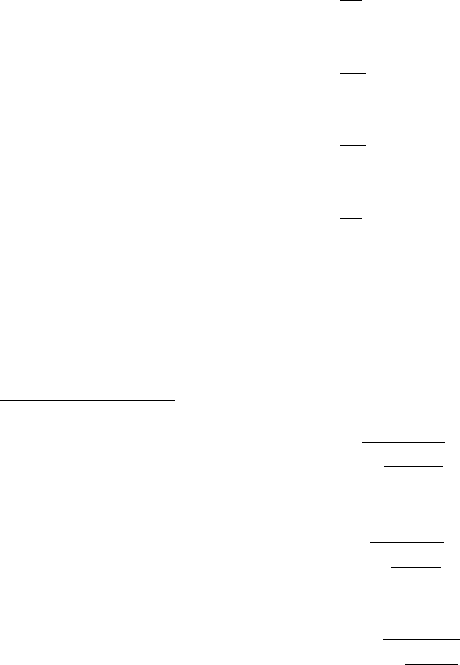
Shock waves 407

Liu and Pego. For positive pressure and temperature

γ>0 ⇐⇒

∂p

∂v



< 0 ,

δ>0 ⇐⇒

∂e

∂T



> 0 ,

δ Γ > 0 ⇐⇒

∂p

∂T



> 0 ,

Γ ≥ δ ⇐⇒

∂e

∂v



≥ 0 .

The latter inequality is in fact an equality for ideal gases, and a strict inequality

for van der Waals ﬂuids.

Interestingly, the Liu criterion allows expansive discontinuities. Taking

on the same adiabat and such that the graph of p is above its

chord on the interval [v

], and u

, u

compatible with (13.4.33) and j :=



−(p

− p

)/(v

− v

) > 0, we obtain an expansive discontinuity of speed

σ = u

l,r

− v

l,r

−

− p

− v

And so for intermediate states W,

σ(W

; W)=u

− v

−

p − p

v − v

≥ σ,

while

σ(W

; W)==u

− v

−

p − p

v − v

≤ σ.

This means that the discontinuity meets the Liu criterion, though being expan-

sive.

However, expansive discontinuities may lack internal structure: for instance,

as was pointed out by Pego [157], when heat conductivity dominates viscosity

and the condition Γ ≥ δ fails, there are expansive discontinuities having no layer,

though meeting Liu’s criterion.

The Lax shock criterion

As a byproduct of the Liu criterion, we obtain the celebrated (weak) Lax shock

inequalities

; n) ≤ σ(W

; W

) ≤ λ

; n),

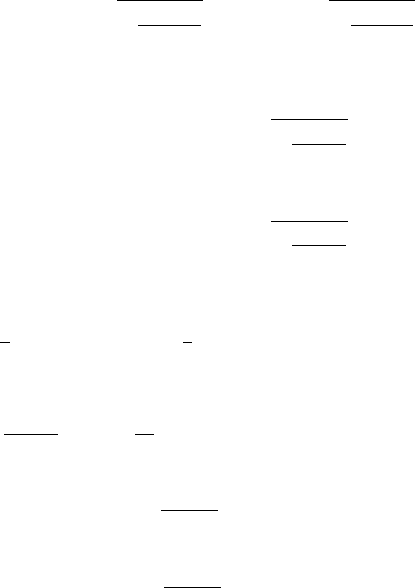
408 The Euler equations for real ﬂuids

either for i = 1 or for i = 3. This is a standard result in the theory of hyperbolic

conservation laws, relying on the fact that shock speeds tend to characteristic

speeds as the amplitudes of shocks go to 0. It can be checked in a most direct way

for ﬂuids. As a matter of fact, two states W

and W

connected by a dynamical

discontinuity of speed σ are such that

σ = u

± v

−

− p

− v

= u

± v

−

− p

− v

Assume for instance that ± here above is a +. Then by a connectedness argument

we have

σ(W

; W)=u

+ v

−

p − p

v − v

along H (W

; W

), and

σ(W

; W)=u

+ v

−

p − p

v − v

along H (W

; W

). And from (13.4.38) and (13.4.39) we have

( v +

Γ(v − v

))dp =(

Γ(p − p

) − γp)dv (13.4.47)

along {h

=0}, which contains H (W

; W

). Therefore,

−

p − p

v − v

−→ γ

when W

H (W

)

−−−−−−−−→ W

and so

σ(W

; W) −→ u

√

= u

+ c

= λ

; n).

For the same reason,

σ(W

; W) −→ u

√

= u

+ c

= λ

; n)

when

H (W

)

−−−−−−−−→ W

More precisely, i-Lax shock inequalities read

; n) <σ<λ

; n) ,λ

i−1

; n) <σ<λ

i+1

; n).

or equivalently, for i =1,

− c

<σ<u

− c

,σ<u

, (13.4.48)

and for i =3,

+ c

<σ<u

+ c

,σ>u

. (13.4.49)

Shock waves 409

A discontinuity satisfying either one of these sets of inequalities is called a Lax

shock. More precisely, in one space dimension, a discontinuity satisfying the i-

Lax shock inequalities is usually called an i-shock. However, in several space

dimensions this distinction is irrelevant, because the choice of left and right is

arbitrary, and exchanging W

and W

amounts to changing n into −n, hence the

1-Lax shock inequalities into the 3-ones. (Recall that the notation u stands for

u · n, and that σ is a normal speed and therefore depends also on the direction of

n.) Still, it is possible to distinguish between the two states of a Lax shock from

an intrinsic point of view, and more precisely, we may speak of the state behind

the shock and the state ahead of the shock. Indeed, the motion of the shock with

respect of the ﬂuid ﬂow on either side of the shock goes from the state indexed

by r to the state indexed by l if we have (13.4.48) whereas it goes from l to r if

we have (13.4.49). In other words, the state behind the shock is indexed by r in

the ﬁrst case and by l in the second one. In both cases, the Lax shock inequalities

imply that the state behind the shock is subsonic, and the other one, supersonic,

according to whether the Mach number

l,r

− σ|

l,r

(13.4.50)

is less than or greater than one. Indeed, (13.4.48) implies M

< 1 <M

and

(13.4.49) implies M

< 1 <M

. To summarize, Lax shocks are characterized

a non-zero mass-transfer ﬂux across the discontinuity,

a subsonic state behind the discontinuity, and a supersonic state ahead of

the discontinuity.

Now, what is the relationship between the Lax shock criterion and the other

criteria? For concave S, a standard Taylor expansion shows that weak shocks

satisfying the entropy criterion (13.4.37) are necessarily Lax shocks. (See [109].)

What can we say for shocks of arbitrary strength? The answer is not easy. One

diﬃculty is that Mach numbers involve slopes of isentropes, since by (13.4.31)

and the deﬁnition of sound speed,

−

∂p

∂v



, (13.4.51)

and isentropes are diﬀerent from shock curves. However, it is possible to reformu-

late the Lax shock criterion in terms of the slopes of Hugoniot adiabats, thanks

to the following result, which is in fact the continuation of Proposition 13.3.

Proposition 13.5 For a Smith ﬂuid, the Hugoniot adiabat, {h

(p, s)=0},

issued from (p

) (where h

is deﬁned as in (13.4.36)), is a curve parametrized

410 The Euler equations for real ﬂuids

by p in the (v, p)-plane. The non-dimensional quantity

R :=

p − p

v − v

∂v

∂p



. (13.4.52)

achieves the value 1 on that curve if and only if

(p − p

)/(v − v

)

∂p

∂v



(13.4.53)

does so at the same point.

(Observe that, thanks to (13.4.34), the equality in (13.4.53) is equivalent to

(13.4.51).)

Proof It relies on (13.4.47), valid along {h

=0}. Under the assumption

(13.1.15), the coeﬃcient of dv in (13.4.47) is always non-zero, which proves the

ﬁrst claim and yields the formula

R =

p − p

v − v

v +

Γ(v − v

)

Γ(p − p

) − γp

Hence, R = 1 if and only if

p − p

v − v

= −

γp

Since

−

γp

∂p

∂v



by (13.1.10), we get the conclusion. 

Of course the equalities R =1andM

= 1 occur simultaneously at p

.But

we also know (by Taylor expansion) that M

is less than 1 on the compression

branch {p>p

} of the Hugoniot locus close to the reference state. So by

Proposition 13.5 this property persists as long as R does not achieve 1 along the

corresponding branch of the Hugoniot adiabat. Similarly, M

is greater than 1 on

the expansion branch. More precisely, using the fact that (p − p

)(v − v

) < 0

along the Hugoniot locus, we can see that (M

− 1) (R − 1) ≥ 0, with equality

only at (p

Corollary 13.1 For a Smith ﬂuid, Lax shocks are characterized by R<1

behind the discontinuity and R>1 ahead.

BOUNDARY CONDITIONS FOR EULER EQUATIONS

We now turn to the Initial Boundary Value Problem (IBVP) for Euler equations.

We provide below a classiﬁcation of IBVPs according to various physical situ-

ations, and discuss possible boundary conditions ensuring well-posedness. (For

similar discussions in the case of viscous compressible ﬂuids, the reader may

refer in particular to [152, 159, 201]; also see the review paper by Higdon [84].)

We shall also give an explicit and elementary construction of Kreiss symmetrizers

for uniformly stable Boundary Value Problems.

14.1 Classiﬁcation of ﬂuids IBVPs

As far as smooth domains are concerned, a crucial issue is the well-posedness

of IBVPs in half-spaces (obtained using co-ordinate charts). To ﬁx ideas, we

consider IBVPs in the half-space {x

≥ 0 } (without loss of generality, the Euler

equations being invariant by rotations).

We recall from Section 13.2 (Proposition 13.1) that the characteristic speeds

of the Euler equations (13.2.18) in the direction n =(0,...,0, 1)

are

= u − c, λ

= u, λ

= u + c,

where u := u · n is the last component of u and c is the sound speed. When

the boundary is a wall, u is clearly zero. Otherwise, if u = 0, we can distinguish

between incoming ﬂows, for which u>0, and outgoing ﬂows, for which u<0.

Another distinction to be made concerns the Mach number

M :=

|u|

The ﬂow is said to be subsonic if M<1andsupersonic if M>1.

This yields the following classiﬁcation, when c is non-zero.

Non-characteristic problems

Out-Supersonic (u<0andM>1, hence λ

, λ

< 0). There is no

incoming characteristic. No boundary condition should be prescribed.

Out-Subsonic (u<0andM<1, hence λ

, λ

< 0, λ

> 0). There is one

incoming characteristic. One and only one boundary condition should be

prescribed.

412 Boundary conditions for Euler equations

In-Subsonic (u>0andM<1, hence λ

< 0, λ

, λ

> 0). There are

(d + 1) incoming characteristics, counting with multiplicity. This means that

(d + 1) independent boundary conditions are needed.

In-Supersonic (u>0andM>1, hence λ

, λ

> 0). All characteristics

are incoming. This means that all components of the unknown W should be

prescribed on the boundary.

Characteristic problems

Slip walls (u = 0, hence λ

< 0, λ

=0,λ

> 0). One and only one boundary

condition b(W) should be prescribed. For the IBVP to be normal,the2-

eigenﬁeld should be tangent to the level set of b.

Out-Sonic (u = −c, hence λ

, λ

< 0, λ

= 0). No boundary condition should

be prescribed.

In-Sonic (u = c, hence λ

=0,λ

, λ

> 0). A set of (d + 1) boundary condi-

tions b

(W), ...b

d+1

(W) should be prescribed, and the 1-eigenﬁeld should be

tangent to the level sets of b

, ...b

d+1

14.2 Dissipative initial boundary value problems

In this section, we look for dissipative boundary conditions. This notion depends

on the symmetrization used. For concreteness, we use the simplest symmetriza-

tion, in (p, u,s) variables, given in Section 13.2.3. We recall indeed that, away

from vacuum, the Euler equations can be rewritten as

S(p, u,s)(∂

+ A(p, u,s; ∇))









=0,

where S(p, u,s) is symmetric positive-deﬁnite and

S(p, u,s) A(p, u,s; n)=







u · n

ρc

n ρ (u · n) I

00u · n







In what follows we take n =(0,...,0, 1)

. According to Deﬁnition 9.2, dissi-

pativeness of a set of boundary conditions encoded by a non-linear mapping

b :(p, u,s) → b(p, u,s) requires that −SA

be non-negative on the tangent bun-

dle of the manifold B = {b(p, u,s)=b

}, while strict dissipativeness requires

that −SA

be coercive on the same bundle.

A straightforward computation shows that for

U =(˙p,

u, ˙u, ˙s)

(SA

U)=ρu



( u ˙u + v ˙p )

−

(1 − M

)˙p

+ 

u

+ v ˙s



(14.2.1)

if u =0.

Dissipative initial boundary value problems 413

We can now review the diﬀerent cases.

Supersonic outﬂow (u<0andM>1). We see that −SA

is coercive on

the whole space. So this case is harmless.

Subsonic outﬂow (u<0andM<1). The restriction of −SA

to the hyper-

plane { ˙p =0} is obviously coercive. Thus a strictly dissipative condition is

obtained by prescribing the pressure p at the boundary. Another possible,

simple, choice is to prescribe the normal velocity u,since−SA

is also coercive

when restricted to the hyperplane { ˙u =0}.

Subsonic inﬂow (u>0andM<1). This is the most complicated case.

Prescribing the pressure among the boundary conditions would obviously be

a bad idea, for the same reason as it is a good one for subsonic outﬂows.

On the other hand, the easiest way to cancel some bad terms is to prescribe

the tangential velocity

u and the entropy s, which leaves only one boundary

condition to be determined in such a way that u du + v dp = 0 on the tangent

bundle of B. Recalling that, by (13.1.1), the speciﬁc enthalpy h = e + pv is

such that

dh = T ds + v dp,

we see that the above requirement is achieved by

+ h along isentropes.

Hence a strictly dissipative set of boundary conditions is

(

+ h,

u ,s) .

Other boundary conditions may be exhibited that are more relevant from a

physical point of view – for instance using concepts of total pressure and total

temperature, see [11].

Supersonic inﬂow (u>0andM>1). We see that SA

(instead of −SA

)

is coercive. But the tangent spaces are reduced to {0}. So this case is also

harmless.

Slip walls (u = 0). The kernel of A

is the d-dimensional subspace {(0,

u, 0, ˙s)},

which is part of the tangent subspace { ˙u =0} associated with the natural

boundary condition on u – as required by the normality criterion. The matrix

is null on { ˙u =0}, which means that the boundary condition is dissipa-

tive but of course not strictly dissipative.

Out-Sonic (u = −c). The matrix −SA

is non-negative but has isotropic

vectors (deﬁned by u ˙u + v ˙p =0,

u =0,˙s =0).

In-Sonic (u = c). The only possible choice of dissipative boundary conditions

is the one described for subsonic inﬂows, which cancels all remaining terms in

(SA

U)(since−(1 − M

)˙p

is zero). The normality criterion is met by

those boundary conditions because

Ker SA

= {(˙p, ˙u, ˙s); u ˙u + v ˙p =0,

u =0, ˙s =0}.

414 Boundary conditions for Euler equations

We now turn to a more systematic testing of boundary conditions, which is

known (and will be shown) to be less restrictive.

14.3 Normal modes analysis

Our purpose is to discuss boundary conditions from the Kreiss–Lopatinski˘ıpoint

of view, for general ﬂuids equipped with a complete equation of state. To get

simpler computations, we choose the speciﬁc volume v and the speciﬁc entropy

s as thermodynamic variables, and rewrite the Euler equations as











∂

v + u ·∇v − v ∇·u =0,

∂

u +(u ·∇)u + vp



∇v + vp



∇s =0,

∂

s + u ·∇s =0,

(14.3.2)

with the short notations



∂p

∂v





∂p

∂s



Later, we shall make the connection with the non-dimensional coeﬃcients γ and

Γ, in that



= −γ



=Γ

Alternatively, we recall that



= −

where c is the sound speed deﬁned in (13.1.10). Our minimal assumption is that

c is real (positive). Furthermore, we have seen in Section 14.1 that boundary

conditions for supersonic ﬂows are either trivial or absent. A normal modes

analysis is irrelevant in those cases. And sonic IBVP are so degenerate that a

normal modes analysis is also useless. So from now on we concentrate on the

subsonic case, assuming that

0 < |u| <c. (14.3.3)

14.3.1 The stable subspace of interior equations

Linearizing (14.3.2) about a reference state (v, u =(

u,u),s), we get











( ∂

u ·

∇ + u∂

)˙v − v ∇·

u =0,

( ∂

u ·

∇ + u∂

)

u + vp



∇˙v + vp



∇˙s =0,

( ∂

u ·

∇ + u∂

)˙s =0,

(14.3.4)