Beer F.P., Johnston E.R., DeWolf J.T., Mazurek D.F. Mechanics of Materials

Подождите немного. Документ загружается.

Apago PDF Enhancer

Since the element had originally a unit volume, the quantity e repre-

sents the change in volume per unit volume; it is referred to as the dila-

tation of the material. Substituting for P

, P

, and P

from Eqs. (2.28)

into (2.30), we write

e 5

1 s

e 5

1 2 2n

1 s

(2.31)†

A case of special interest is that of a body subjected to a uni-

form hydrostatic pressure p. Each of the stress components is then

equal to 2p and Eq. (2.31) yields

e 52

1 2 2n

(2.32)

Introducing the constant

1 2 2n

(2.33)

we write Eq. (2.32) in the form

e 52

(2.34)

The constant k is known as the bulk modulus or modulus of compres-

sion of the material. It is expressed in the same units as the modulus

of elasticity E, that is, in pascals or in psi.

Observation and common sense indicate that a stable material

subjected to a hydrostatic pressure can only decrease in volume; thus

the dilatation e in Eq. (2.34) is negative, from which it follows that

the bulk modulus k is a positive quantity. Referring to Eq. (2.33), we

conclude that 1 2 2n . 0, or n ,

. On the other hand, we recall

from Sec. 2.11 that n is positive for all engineering materials. We

thus conclude that, for any engineering material,

0, n ,

(2.35)

We note that an ideal material having a value of v equal to zero could

be stretched in one direction without any lateral contraction. On the

other hand, an ideal material for which n 5

, and thus k 5 `, would

be perfectly incompressible (e 5 0). Referring to Eq. (2.31) we also

note that, since n ,

in the elastic range, stretching an engineering

material in one direction, for example in the x direction (s

. 0,

5 s

5 0), will result in an increase of its volume (e . 0).‡

*2.13 Dilatation; Bulk Modulus

†Since the dilatation e represents a change in volume, it must be independent of the ori-

entation of the element considered. It then follows from Eqs. (2.30) and (2.31) that the

quantities P

1 P

and s

1 s

are also independent of the orientation of the

element. This property will be verified in Chap. 7.

‡However, in the plastic range, the volume of the material remains nearly constant.

bee80288_ch02_052-139.indd Page 97 11/8/10 9:34:11 PM user-f494bee80288_ch02_052-139.indd Page 97 11/8/10 9:34:11 PM user-f494 volume 201/FREE048/work%0/indd%0/volume 201/FREE048/work%0/indd%0/

Apago PDF Enhancer

2.14 SHEARING STRAIN

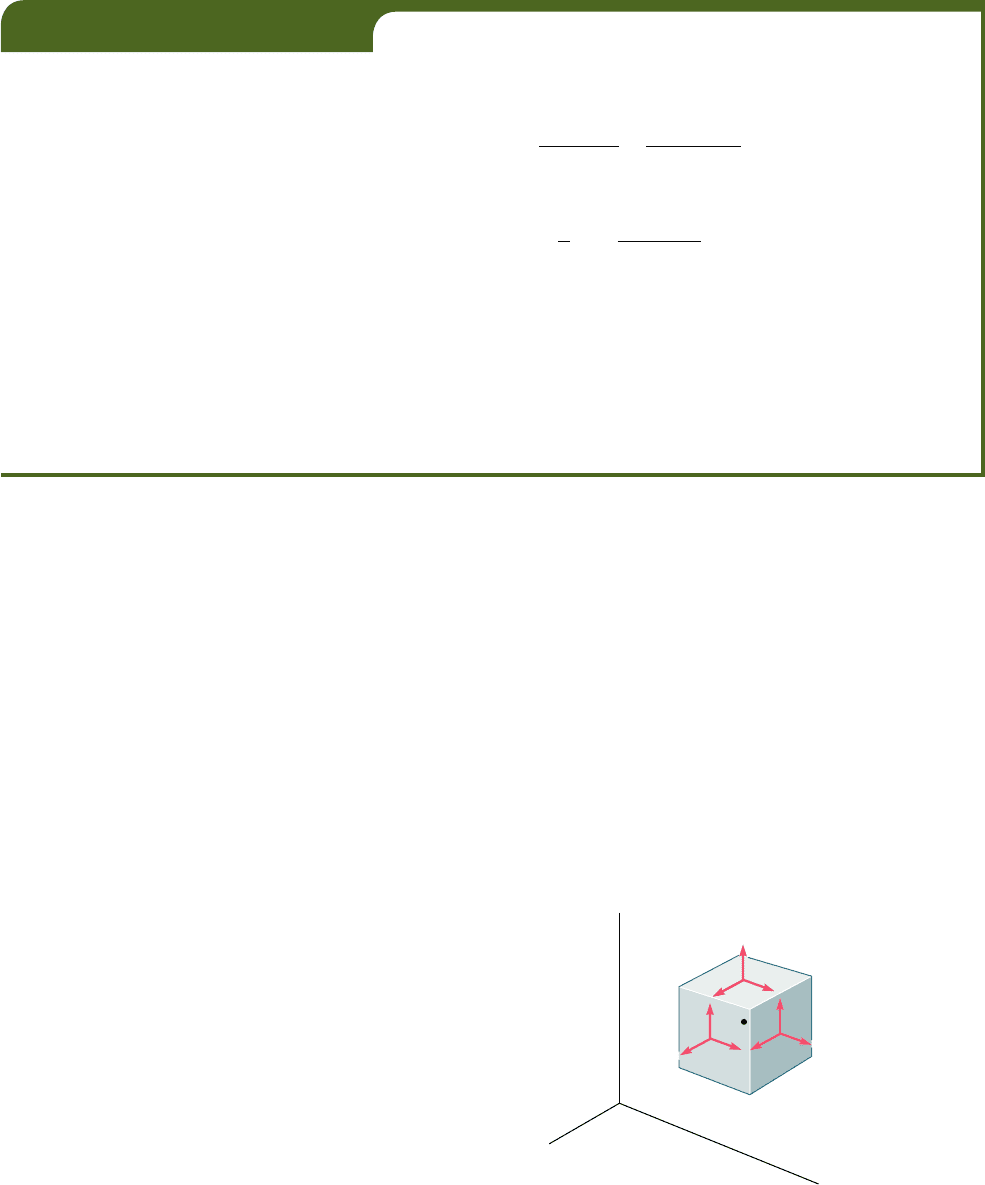
When we derived in Sec. 2.12 the relations (2.28) between normal

stresses and normal strains in a homogeneous isotropic material, we

assumed that no shearing stresses were involved. In the more gen-

eral stress situation represented in Fig. 2.41, shearing stresses t

, and t

will be present (as well, of course, as the corresponding

shearing stresses t

, t

, and t

). These stresses have no direct

effect on the normal strains and, as long as all the deformations

involved remain small, they will not affect the derivation nor

the validity of the relations (2.28). The shearing stresses, however,

will tend to deform a cubic element of material into an oblique

parallelepiped.

Determine the change in volume DV of the steel block shown in Fig. 2.40,

when it is subjected to the hydrostatic pressure p 5 180 MPa. Use E 5

200 GPa and n 5 0.29.

From Eq. (2.33), we determine the bulk modulus of steel,

1 2 2n

200

1 2 0.58

5 158.7 GPa

and, from Eq. (2.34), the dilatation,

e 52

180

MPa

158.7 GPa

521.134 3 10

Since the volume V of the block in its unstressed state is

V 5 (80 mm)(40 mm)(60 mm) 5 192 3 10

and since e represents the change in volume per unit volume, e 5 DVyV,

we have

DV 5 eV 5 (21.134 3 10

)(192 3 10

)

DV 5 2218 mm

EXAMPLE 2.09

␶

␴

␶

Fig. 2.41 General state of stress.

bee80288_ch02_052-139.indd Page 98 11/8/10 8:07:24 PM user-f499bee80288_ch02_052-139.indd Page 98 11/8/10 8:07:24 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

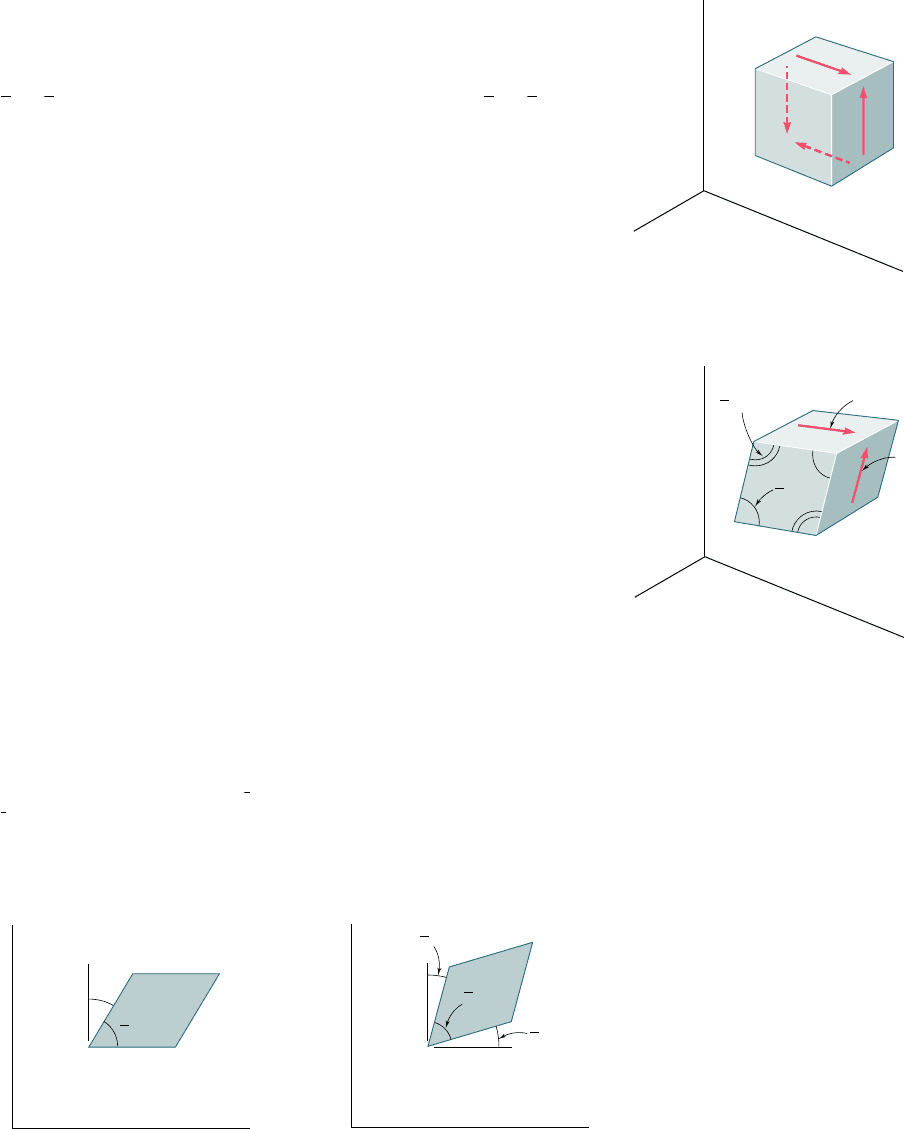
Consider first a cubic element of side one (Fig. 2.42) subjected

to no other stresses than the shearing stresses t

and t

applied to

faces of the element respectively perpendicular to the x and y axes.

(We recall from Sec. 1.12 that t

5 t

.) The element is observed

to deform into a rhomboid of sides equal to one (Fig. 2.43). Two of

the angles formed by the four faces under stress are reduced from

2 g

, while the other two are increased from

1 g

The small angle g

(expressed in radians) defines the shearing strain

corresponding to the x and y directions. When the deformation

involves a reduction of the angle formed by the two faces oriented

respectively toward the positive x and y axes (as shown in Fig. 2.43),

the shearing strain g

is said to be positive; otherwise, it is said to

be negative.

We should note that, as a result of the deformations of the

other elements of the material, the element under consideration can

also undergo an overall rotation. However, as was the case in our

study of normal strains, we are concerned here only with the actual

deformation of the element, and not with any possible superimposed

rigid-body displacement.†

Plotting successive values of t

against the corresponding val-

ues of g

, we obtain the shearing stress-strain diagram for the mate-

rial under consideration. This can be accomplished by carrying out

a torsion test, as you will see in Chap. 3. The diagram obtained is

similar to the normal stress-strain diagram obtained for the same

material from the tensile test described earlier in this chapter. How-

ever, the values obtained for the yield strength, ultimate strength,

etc., of a given material are only about half as large in shear as they

are in tension. As was the case for normal stresses and strains, the

initial portion of the shearing stress-strain diagram is a straight line.

For values of the shearing stress that do not exceed the proportional



Fig. 2.42 Cubic element subjected to

shearing stresses.

†In defining the strain g

, some authors arbitrarily assume that the actual deformation of

the element is accompanied by a rigid-body rotation such that the horizontal faces of the

element do not rotate. The strain g

is then represented by the angle through which the

other two faces have rotated (Fig. 2.44). Others assume a rigid-body rotation such that

the horizontal faces rotate through

counterclockwise and the vertical faces through

clockwise (Fig. 2.45). Since both assumptions are unnecessary and may lead to confu-

sion, we prefer in this text to associate the shearing strain g

with the change in the angle

formed by the two faces, rather than with the rotation of a given face under restrictive

conditions.















Fig. 2.43 Deformation of cubic

element due to shearing stresses.









Fig. 2.44









Fig. 2.45

2.14 Shearing Strain

bee80288_ch02_052-139.indd Page 99 9/4/10 5:18:22 PM user-f499bee80288_ch02_052-139.indd Page 99 9/4/10 5:18:22 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

100

Stress and Strain—Axial Loading

limit in shear, we can therefore write for any homogeneous isotropic

material,

5 Gg

(2.36)

This relation is known as Hooke’s law for shearing stress and strain,

and the constant G is called the modulus of rigidity or shear modulus

of the material. Since the strain g

was defined as an angle in radi-

ans, it is dimensionless, and the modulus G is expressed in the same

units as t

, that is, in pascals or in psi. The modulus of rigidity G

of any given material is less than one-half, but more than one-third

of the modulus of elasticity E of that material.†

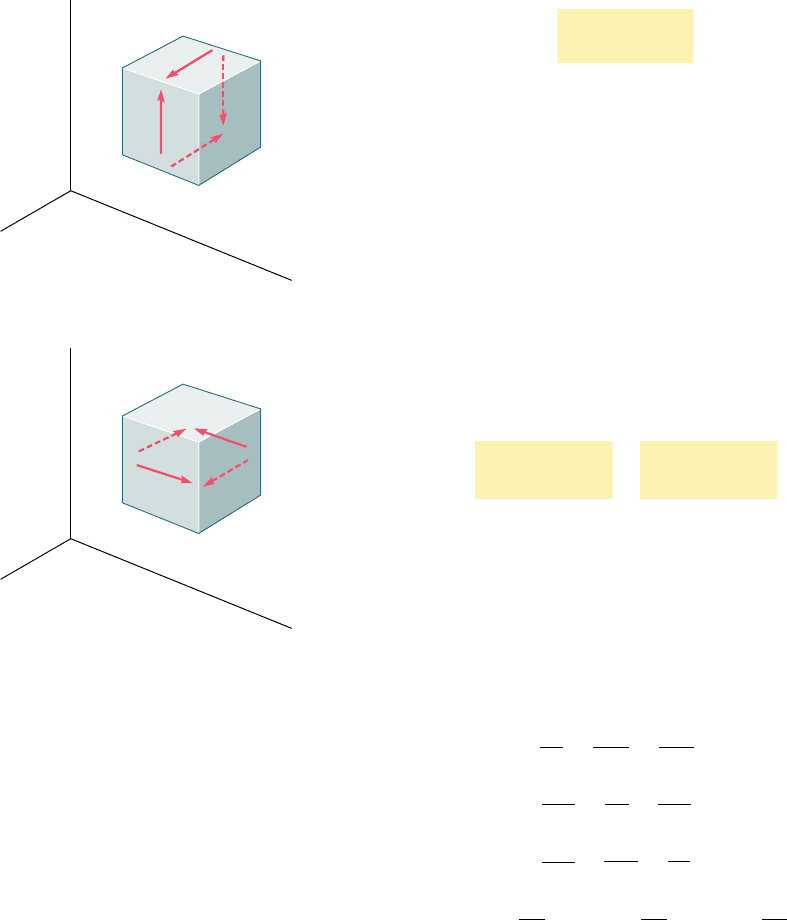
Considering now a small element of material subjected to

shearing stresses t

and t

(Fig. 2.46a), we define the shearing

strain g

as the change in the angle formed by the faces under stress.

The shearing strain g

is defined in a similar way by considering an

element subjected to shearing stresses t

and t

(Fig. 2.46b). For

values of the stress that do not exceed the proportional limit, we can

write the two additional relations

5 Gg

t

5 Gg

(2.37)

where the constant G is the same as in Eq. (2.36).

For the general stress condition represented in Fig. 2.41, and

as long as none of the stresses involved exceeds the corresponding

proportional limit, we can apply the principle of superposition and

combine the results obtained in this section and in Sec. 2.12. We

obtain the following group of equations representing the generalized

Hooke’s law for a homogeneous isotropic material under the most

general stress condition.

(2.38)

g

An examination of Eqs. (2.38) might lead us to believe that

three distinct constants, E, n, and G, must first be determined exper-

imentally, if we are to predict the deformations caused in a given

material by an arbitrary combination of stresses. Actually, only two

of these constants need be determined experimentally for any given

material. As you will see in the next section, the third constant can

then be obtained through a very simple computation.

␶

(a)

␶

(b)

Fig. 2.46

†See Prob. 2.91.

bee80288_ch02_052-139.indd Page 100 11/8/10 8:07:33 PM user-f499bee80288_ch02_052-139.indd Page 100 11/8/10 8:07:33 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

101

2.15 FURTHER DISCUSSION OF DEFORMATIONS

UNDER AXIAL LOADING; RELATION AMONG

E, N, AND G

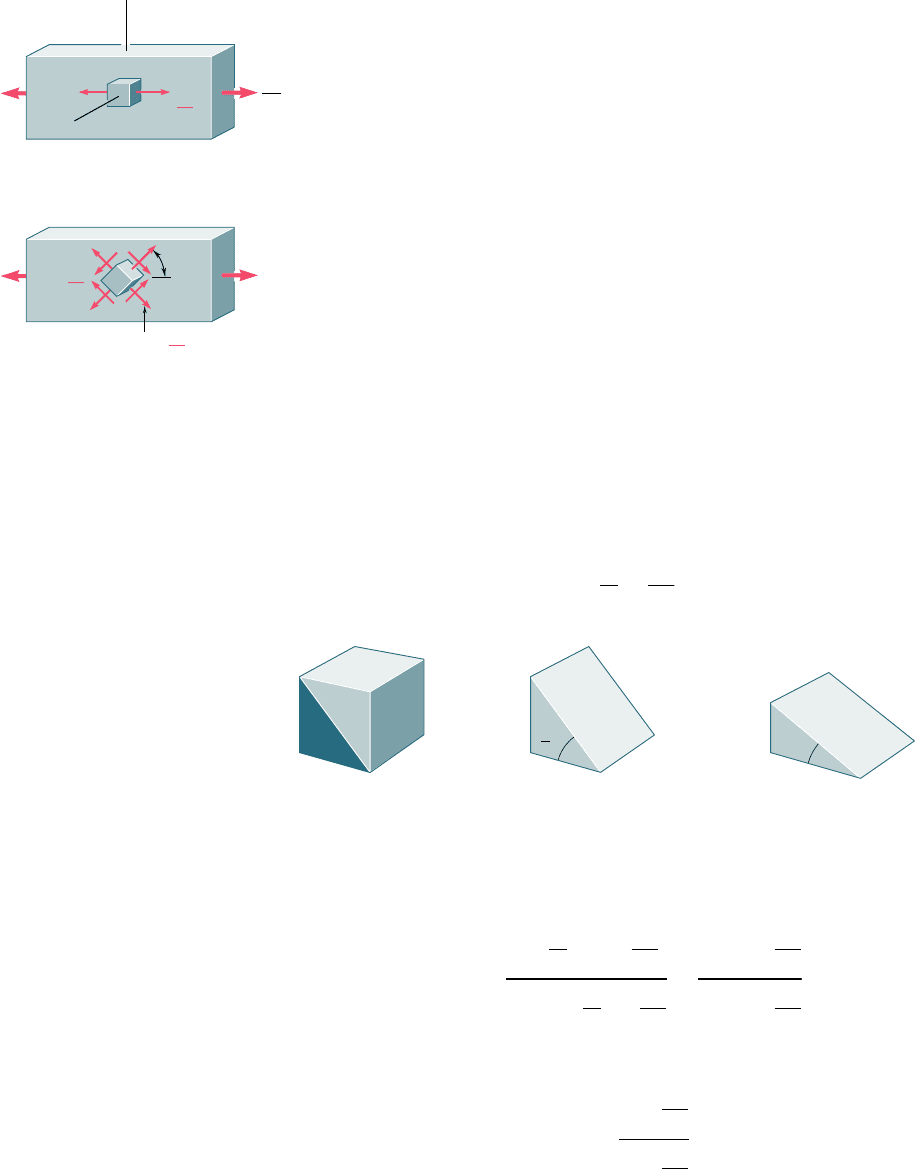
We saw in Sec. 2.11 that a slender bar subjected to an axial tensile

load P directed along the x axis will elongate in the x direction

and contract in both of the transverse y and z directions. If

denotes the axial strain, the lateral strain is expressed as P

5 2nP

, where n is Poisson’s ratio. Thus, an element in the

shape of a cube of side equal to one and oriented as shown in Fig.

2.49a will deform into a rectangular parallelepiped of sides 1 1

, 1 2 nP

, and 1 2 nP

. (Note that only one face of the element

is shown in the figure.) On the other hand, if the element is ori-

ented at 458 to the axis of the load (Fig. 2.49b), the face shown in

the figure is observed to deform into a rhombus. We conclude that

the axial load P causes in this element a shearing strain g9 equal

to the amount by which each of the angles shown in Fig. 2.49b

increases or decreases.†

2.5 in.

2 in.

8 in.

Fig. 2.47

2 in.

0.04 in.

␥

Fig. 2.48

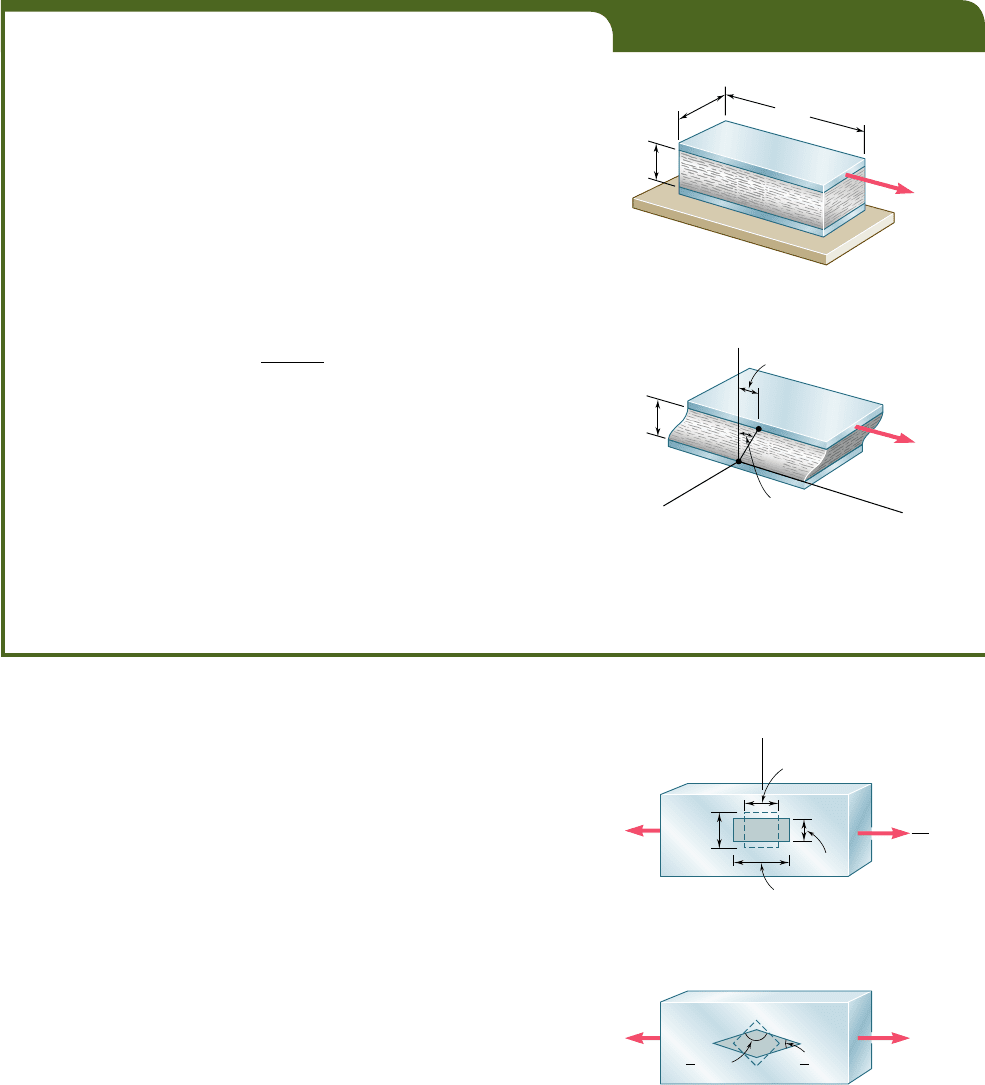
EXAMPLE 2.10A rectangular block of a material with a modulus of rigidity G 5 90 ksi

is bonded to two rigid horizontal plates. The lower plate is fixed, while

the upper plate is subjected to a horizontal force P (Fig. 2.47). Knowing

that the upper plate moves through 0.04 in. under the action of the force,

determine (a) the average shearing strain in the material, (b) the force P

exerted on the upper plate.

(a) Shearing Strain. We select coordinate axes centered at the

midpoint C of edge AB and directed as shown (Fig. 2.48). According to

its definition, the shearing strain g

is equal to the angle formed by the

vertical and the line CF joining the midpoints of edges AB and DE. Not-

ing that this is a very small angle and recalling that it should be expressed

in radians, we write

< tan g

0.04 in.

2 i

g

5 0.020 rad

(b) Force Exerted on Upper Plate. We first determine the shear-

ing stress t

in the material. Using Hooke’s law for shearing stress and

strain, we have

5 Gg

5 190 3 10

psi210.020 rad25 1800 psi

The force exerted on the upper plate is thus

P 5 t

A 5 11800 psi218 in.212.5 in.25 36.0 3 10

P 5 36.0 kips

†Note that the load P also produces normal strains in the element shown in Fig. 2.49b

(see Prob. 2.73).

1 ⫹

⑀

1 ⫺

␯⑀

(a)

(b)

␲

␥

⫺

␲

⫹

PP'

Fig. 2.49 Representations of strain in an

axially-loaded bar.

bee80288_ch02_052-139.indd Page 101 11/2/10 1:11:22 AM user-f499bee80288_ch02_052-139.indd Page 101 11/2/10 1:11:22 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

102

Stress and Strain—Axial Loading

The fact that shearing strains, as well as normal strains, result

from an axial loading should not come to us as a surprise, since we

already observed at the end of Sec. 1.12 that an axial load P causes

normal and shearing stresses of equal magnitude on four of the faces

of an element oriented at 458 to the axis of the member. This was

illustrated in Fig. 1.38, which, for convenience, has been repeated

here. It was also shown in Sec. 1.11 that the shearing stress is maxi-

mum on a plane forming an angle of 458 with the axis of the load.

It follows from Hooke’s law for shearing stress and strain that the

shearing strain g9 associated with the element of Fig. 2.49b is also

maximum: g9 5 g

While a more detailed study of the transformations of strain

will be postponed until Chap. 7, we will derive in this section a

relation between the maximum shearing strain g9 5 g

associated

with the element of Fig. 2.49b and the normal strain P

in the direc-

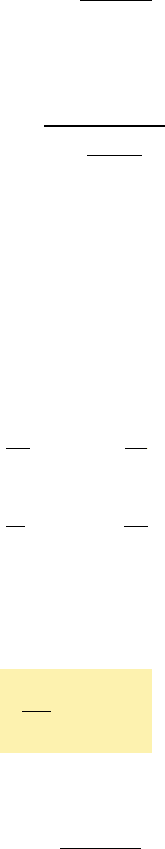
tion of the load. Let us consider for this purpose the prismatic ele-

ment obtained by intersecting the cubic element of Fig. 2.49a by a

diagonal plane (Fig. 2.50a and b). Referring to Fig. 2.49a, we con-

clude that this new element will deform into the element shown in

Fig. 2.50c, which has horizontal and vertical sides respectively equal

to 1 1 P

and 1 2 nP

. But the angle formed by the oblique and

horizontal faces of the element of Fig. 2.50b is precisely half of one

of the right angles of the cubic element considered in Fig. 2.49b.

The angle b into which this angle deforms must therefore be equal

to half of py2 2 g

. We write

b 5

(b)

(a)



45



⫽

Fig. 1.38 (repeated )





1 







(a)(b)(c)

Fig. 2.50

Applying the formula for the tangent of the difference of two angles,

we obtain

tan b 5

tan

2 tan

1 1 tan

tan

1 2 tan

1 1 tan

or, since g

y2 is a very small angle,

tan b 5

1 2

1 1

(2.39)

bee80288_ch02_052-139.indd Page 102 9/4/10 5:18:40 PM user-f499bee80288_ch02_052-139.indd Page 102 9/4/10 5:18:40 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

103

But, from Fig. 2.50c, we observe that

tan b 5

1 2 nP

1 1 P

(2.40)

Equating the right-hand members of (2.39) and (2.40), and solving

for g

, we write

11 1 n2P

1 1

1 2 n

Since P

V 1, the denominator in the expression obtained can be

assumed equal to one; we have, therefore,

5 (1 1 n)P

(2.41)

which is the desired relation between the maximum shearing strain

and the axial strain P

To obtain a relation among the constants E, n, and G, we recall

that, by Hooke’s law, g

5 t

yG, and that, for an axial loading, P

yE. Equation (2.41) can therefore be written as

5 11 1 n2

(2.42)

We now recall from Fig. 1.38 that s

5 PyA and t

5 Py2A, where

A is the cross-sectional area of the member. It thus follows that

5 2. Substituting this value into (2.42) and dividing both

members by 2, we obtain the relation

5 1 1 n

(2.43)

which can be used to determine one of the constants E, n, or G from

the other two. For example, solving Eq. (2.43) for G, we write

G 5

211 1 n2

(2.439)

*2.16 STRESS-STRAIN RELATIONSHIPS FOR

FIBER-REINFORCED COMPOSITE MATERIALS

Fiber-reinforced composite materials were briefly discussed in Sec.

2.5. It was shown at that time that these materials are obtained by

embedding fibers of a strong, stiff material into a weaker, softer mate-

rial, referred to as a matrix. It was also shown that the relationship

between the normal stress and the corresponding normal strain cre-

ated in a lamina, or layer, of a composite material depends upon the

direction in which the load is applied. Different moduli of elasticity,

, E

, and E

, are therefore required to describe the relationship

between normal stress and normal strain, according to whether the

*2.16 Stress-Strain Relationships for Fiber-

Reinforced Composite Materials

bee80288_ch02_052-139.indd Page 103 11/2/10 1:11:29 AM user-f499bee80288_ch02_052-139.indd Page 103 11/2/10 1:11:29 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

104

Stress and Strain—Axial Loading

load is applied in a direction parallel to the fibers, in a direction per-

pendicular to the layer, or in a transverse direction.

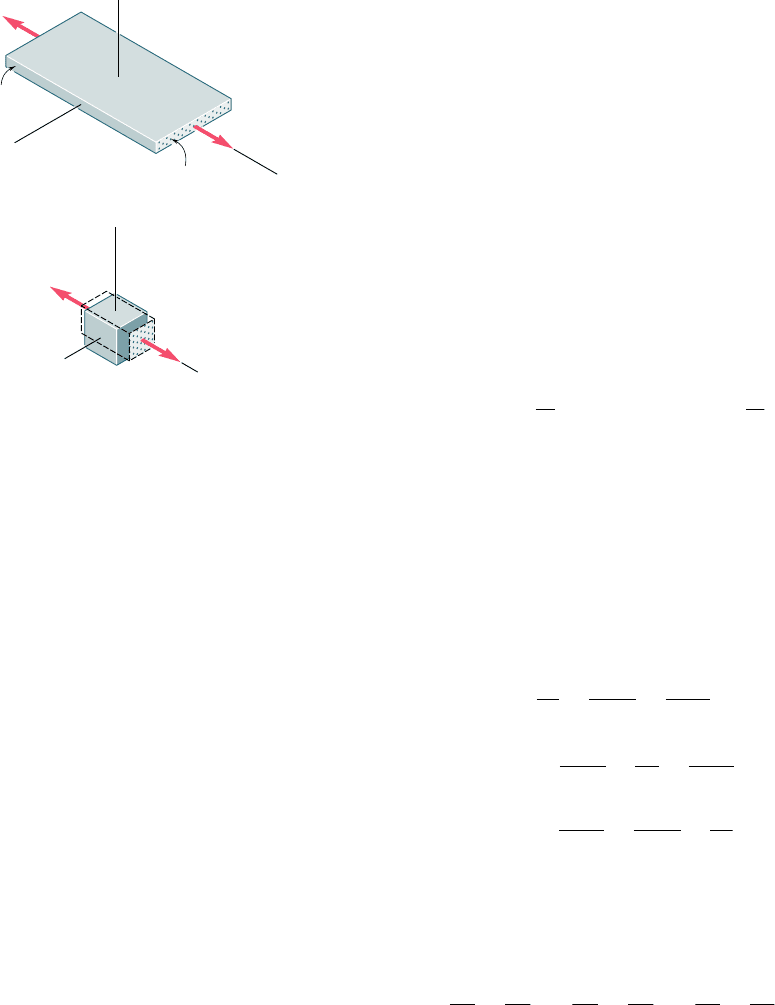
Let us consider again the layer of composite material discussed

in Sec. 2.5 and let us subject it to a uniaxial tensile load parallel to

its fibers, i.e., in the x direction (Fig. 2.51a). To simplify our analysis,

it will be assumed that the properties of the fibers and of the matrix

have been combined, or “smeared,” into a fictitious equivalent homo-

geneous material possessing these combined properties. We now con-

sider a small element of that layer of smeared material (Fig. 2.51b).

We denote by s

the corresponding normal stress and observe that

5 s

5 0. As indicated earlier in Sec. 2.5, the corresponding

normal strain in the x direction is P

5 s

, where E

is the modulus

of elasticity of the composite material in the x direction. As we saw

for isotropic materials, the elongation of the material in the x direction

is accompanied by contractions in the y and z directions. These con-

tractions depend upon the placement of the fibers in the matrix and

will generally be different. It follows that the lateral strains P

and P

will also be different, and so will the corresponding Poisson’s ratios:

and

(2.44)

Note that the first subscript in each of the Poisson’s ratios n

and

in Eqs. (2.44) refers to the direction of the load, and the second

to the direction of the contraction.

It follows from the above that, in the case of the multiaxial load-

ing of a layer of a composite material, equations similar to Eqs. (2.28)

of Sec. 2.12 can be used to describe the stress-strain relationship. In

the present case, however, three different values of the modulus of

elasticity and six different values of Poisson’s ratio will be involved. We

write

(2.45)

Equations (2.45) may be considered as defining the transformation

of stress into strain for the given layer. It follows from a general

property of such transformations that the coefficients of the stress

components are symmetric, i.e., that

(2.46)

These equations show that, while different, the Poisson’s ratios n

and n

are not independent; either of them can be obtained from

the other if the corresponding values of the modulus of elasticity are

known. The same is true of n

and n

, and of n

and n

Consider now the effect of the presence of shearing stresses

on the faces of a small element of smeared layer. As pointed out in

Layer of

material

Fibers

Load

(a)

Fig. 2.51 Fiber-reinforced composite

material under uniaxial tensile load.

(b)

␴

bee80288_ch02_052-139.indd Page 104 11/8/10 8:07:43 PM user-f499bee80288_ch02_052-139.indd Page 104 11/8/10 8:07:43 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02

Apago PDF Enhancer

105

Sec. 2.14 in the case of isotropic materials, these stresses come in pairs

of equal and opposite vectors applied to opposite sides of the given

element and have no effect on the normal strains. Thus, Eqs. (2.45)

remain valid. The shearing stresses, however, will create shearing

strains which are defined by equations similar to the last three of the

equations (2.38) of Sec. 2.14, except that three different values of the

modulus of rigidity, G

, G

, and G

, must now be used. We have

(2.47)

The fact that the three components of strain P

, P

, and P

can

be expressed in terms of the normal stresses only and do not depend

upon any shearing stresses characterizes orthotropic materials and

distinguishes them from other anisotropic materials.

As we saw in Sec. 2.5, a flat laminate is obtained by superposing

a number of layers or laminas. If the fibers in all layers are given the

same orientation to better withstand an axial tensile load, the lami-

nate itself will be orthotropic. If the lateral stability of the laminate

is increased by positioning some of its layers so that their fibers are

at a right angle to the fibers of the other layers, the resulting laminate

will also be orthotropic. On the other hand, if any of the layers of a

laminate are positioned so that their fibers are neither parallel nor

perpendicular to the fibers of other layers, the lamina, generally, will

not be orthotropic.†

†For more information on fiber-reinforced composite materials, see Hyer, M. W., Stress

Analysis of Fiber-Reinforced Composite Materials, McGraw-Hill, New York, 1998.

2.16 Stress-Strain Relationships for Fiber-

Reinforced Composite Materials

EXAMPLE 2.11

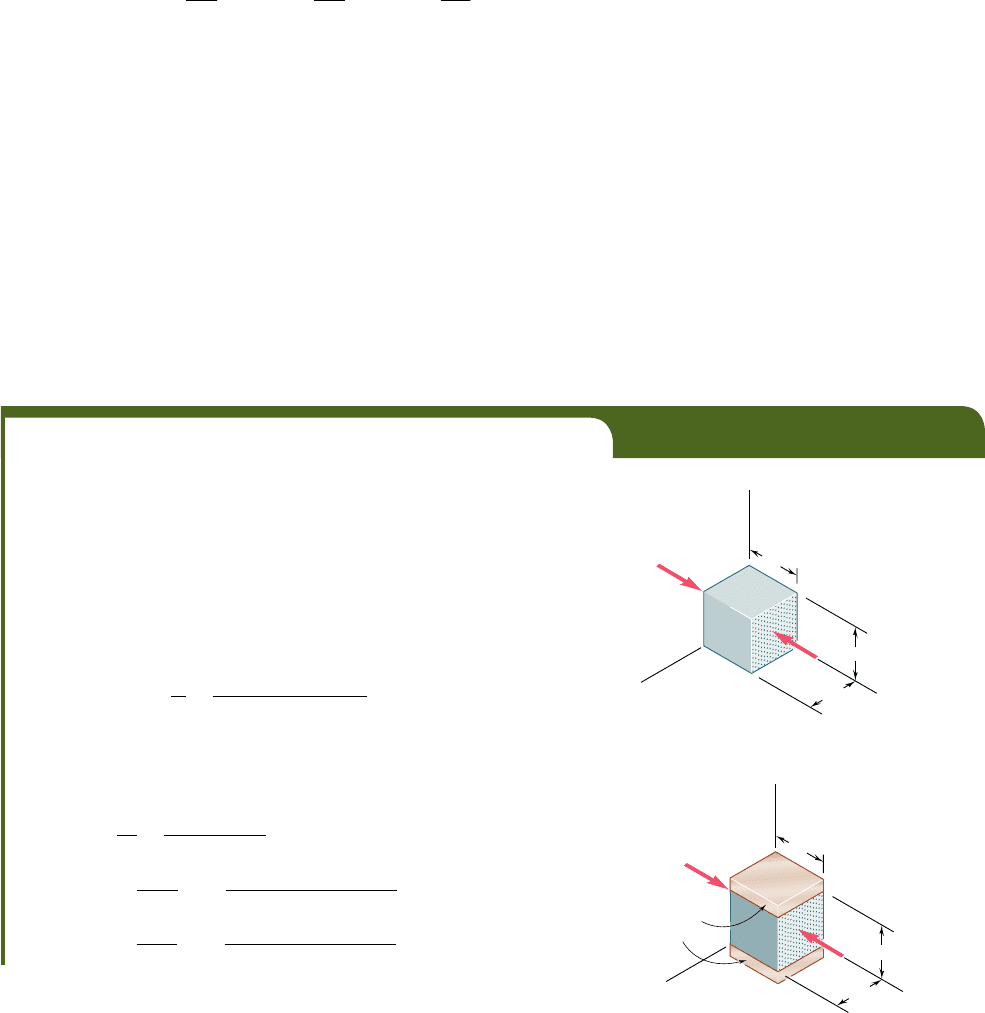
A 60-mm cube is made from layers of graphite epoxy with fibers aligned

in the x direction. The cube is subjected to a compressive load of 140 kN

in the x direction. The properties of the composite material are: E

155.0 GPa, E

5 12.10 GPa, E

5 12.10 GPa, n

5 0.248, n

5 0.248,

and n

5 0.458. Determine the changes in the cube dimensions, knowing

that (a) the cube is free to expand in the y and z directions (Fig. 2.52);

(b) the cube is free to expand in the z direction, but is restrained from

expanding in the y direction by two fixed frictionless plates (Fig. 2.53).

(a) Free in y and z Directions. We first determine the stress s

in the direction of loading. We have

2140 3 10

0.060 m

5238.89 MPa

Since the cube is not loaded or restrained in the y and z directions, we have

5 s

5 0. Thus, the right-hand members of Eqs. (2.45) reduce to their

first terms. Substituting the given data into these equations, we write

MPa

155.0 GPa

52250.9 3 10

0.248

238.89 MPa

155.0 GPa

5162.22 3 10

0.248

238.69 MPa

155.0 GPa

5162.22 3 10

140 kN

60 mm

140 kN

Fig. 2.52

140 kN

60 mm

Fixed

frictionless

plates

60 mm

140 kN

Fig. 2.53

bee80288_ch02_052-139.indd Page 105 11/8/10 9:37:51 PM user-f494bee80288_ch02_052-139.indd Page 105 11/8/10 9:37:51 PM user-f494 volume 201/FREE048/work%0/indd%0/volume 201/FREE048/work%0/indd%0/

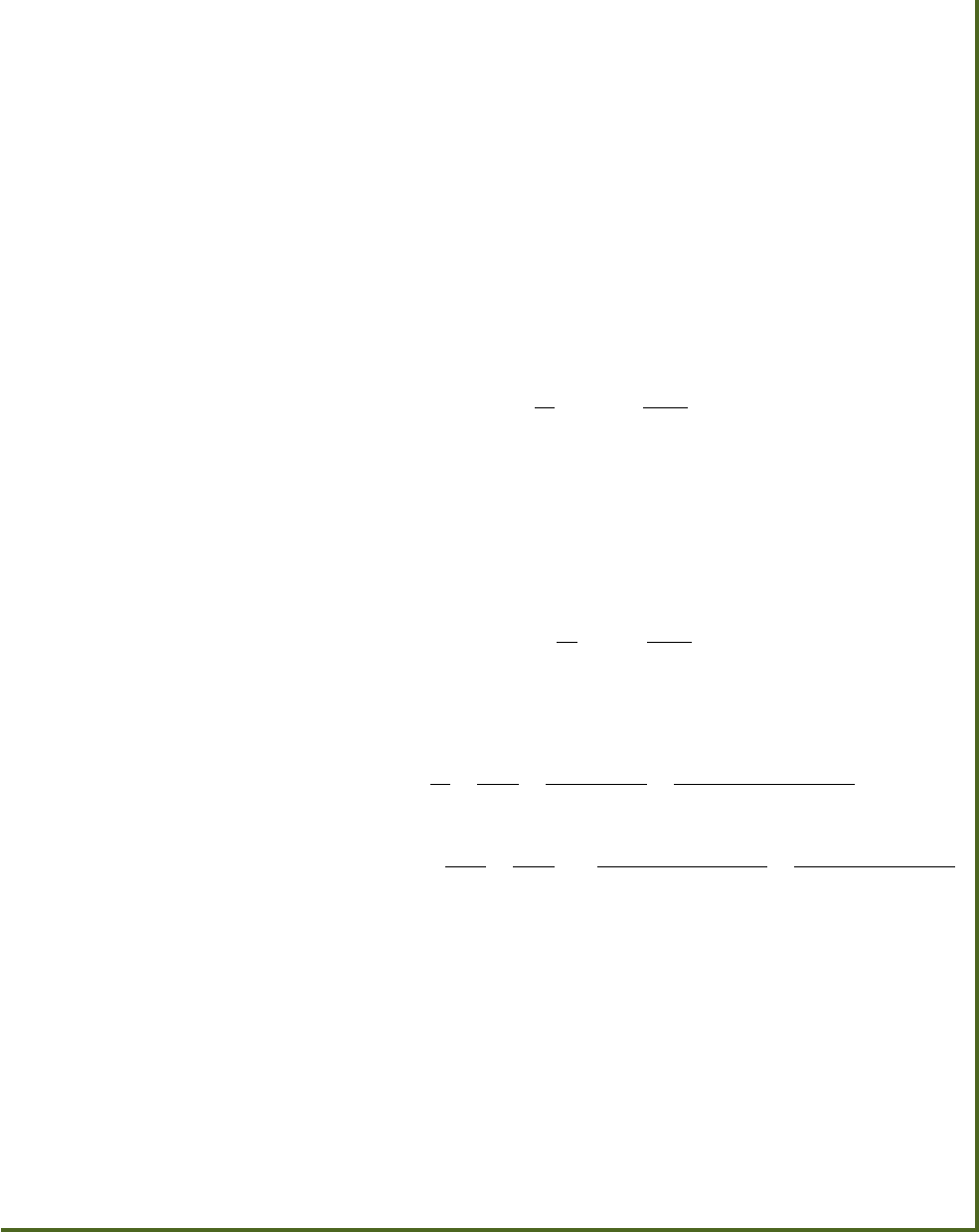
Apago PDF Enhancer

The changes in the cube dimensions are obtained by multiplying the cor-

responding strains by the length L 5 0.060 m of the side of the cube:

5 P

L 5

2250.9 3 10

0.060 m

5215.05 mm

5 P

L 5 1162.2 3 10

210.060 m2513.73 mm

5 P

L 5

162.2 3 10

0.060 m

513.73 mm

(b) Free in z Direction, Restrained in y Direction. The stress

in the x direction is the same as in part a, namely, s

5 238.89 MPa.

Since the cube is free to expand in the z direction as in part a, we again

have s

5 0. But since the cube is now restrained in the y direction,

we should expect a stress s

different from zero. On the other hand,

since the cube cannot expand in the y direction, we must have d

5 0

and, thus, P

5 d

yL 5 0. Making s

5 0 and P

5 0 in the second of

Eqs. (2.45), solving that equation for s

, and substituting the given data,

we have

5 a

b n

5 a

12.10

155.0

b10.24821238.89 MPa2

52752

Now that the three components of stress have been determined, we can

use the first and last of Eqs. (2.45) to compute the strain components

and P

. But the first of these equations contains Poisson’s ratio n

and, as we saw earlier, this ratio is not equal to the ratio n

which was

among the given data. To find n

we use the first of Eqs. (2.46) and

write

5 a

b n

5 a

12.10

155.0

b10.24825 0.01936

Making s

5 0 in the first and third of Eqs. (2.45) and substituting in

these equations the given values of E

, E

, n

, and n

, as well as the values

obtained for s

, s

, and n

, we have

238.89 MPa

155.0 GPa

0.01936

2752.9 kPa

12.10 GPa

52249

7 3 10

0.248

238.89 MPa

155.0 GPa

0.458

2752.9 kPa

12.10 GPa

5190

72 3 10

The changes in the cube dimensions are obtained by multiplying the cor-

responding strains by the length L 5 0.060 m of the side of the cube:

5 P

L 5

2249.7 3 10

0.060 m

5214.98 mm

5 P

L 5

0.060 m

5 0

5 P

L 5

190.72 3 10

0.060 m

515.44 mm

Comparing the results of parts a and b, we note that the difference

between the values obtained for the deformation d

in the direction of

the fibers is negligible. However, the difference between the values

obtained for the lateral deformation d

is not negligible. This deformation

is clearly larger when the cube is restrained from deforming in the y

direction.

106

bee80288_ch02_052-139.indd Page 106 11/2/10 1:11:30 AM user-f499bee80288_ch02_052-139.indd Page 106 11/2/10 1:11:30 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch02