Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

Derivatives

and

Graphs

In this section we see how information about the graph of a function can be obtained from its

derivative

f', and still more information from the derivative of the derivative, f".

(xo) > 0, then since this positive slope is the limit of the slopes of segments from

(xo,

f(xo))

to nearby points (x,

f(x)),

all such segments must have positive slope. Hence,

f(x)

f(xo)

for all x

just

to the right of xo, and

f(x)

f(xo)

for all x just to the left of

Xo.

We say that f has a local maximum at xo, provided

f(xo)

f(x)

for all x sufficiently

close to

xo, and a local minimum at xo, provided

f(xo)

f(x)

for all x sufficiently close to

Xo.

From the argument above it is clear that f can have neither a local maximum nor a local

minimum at

if f'(XO) > 0 or if

f'(xo)

Therefore, if

f(xo)

is either a local maximum

or a local minimum, then

f'(XO) =

The condition

f'(xo)

=0 is necessary for

f(xo)

to be

a local maximum or minimum, but not a sufficient condition;

can be zero at points where

f does not have a maximum or minimum. (See Figure 9.1.) If we are concerned only about

the values of

I(x)

for x in some closed interval [a, b], then I can have a local maximum or

minimum at

a or b without

being zero. Indeed, if, for example,

f'(a)

> 0, then

f(a)

necessarily a local minimum on

[a, b].

If I' (x) > 0 for all x in some interval, then I (x) is strictly increasing throughout that

interval. Similarly,

(x)

is strictly decreasing on any interval on which I'

(x)

These

facts are consequences of the following important result:

Mean Value Theorem:

(x) exists

for

b, then there is some c E (a, b)

such that

f(b)

f(a)

f'(c)(b

- a).

The Mean Value Theorem is simply a precise statement of the obvious fact (Figure 9.2)

that a graph cannot get smoothly (Le., no comers) from

(a,

f(a))

to (b,

f(b))

without pointing

in the right direction for at least one point c between

a and b. As another interpretation of

the Mean Value Theorem, suppose

f(t)

is the distance your car goes from time t = a to time

t = b. The average speed over the interval a

b is

(f(b)

f(a))/(b

- a). The Mean

Value Theorem (and common sense) says that there must be a least one point t

= c at which

the instantaneous speed

Understanding Calculus

Figure 9.1

f'(c)

f(b)

f(a)

b-a

(a,f(a»

(b,f(b»

_____

~_--

-J

f(b)-f(a)

b-a

c b

Figure 9.2

One obvious but very important consequence of the Mean Value Theorem is the fact that

(x)

0 on some interval, then f (x) is constant on that interval. That is, if Xl and

are

any two points, then

f(XI)

= f(X2) because

f(X2) -

f(XI)

= f'(C)(X2 -

Xl)

=o·(Xl -

X2)

A corollary of this fact that we will use in integration theory is the following:

two functions

have the same derivative, then they differ by a constant.

That is, if

f'(x)

g'(x), and

h(x)

f(x)

- g(x), then

h'(x)

0 so

h(x)

f(x)

g(x)

is a constant.

(x) > 0 for all X in some interval

then for any interval

[Xl,

X2] within I we can

apply the Mean Value Theorem to get

f(X2) -

I(XI)

= f'(C)(X2 -

Xl)·

Since

f'(c)

> 0, f(X2) >

f(XI)

and I is increasing in

I'(x)

> 0 on an interval

(Xl,

Xo),

f(x)

is increasing up to

I(xo),

and

I'(x)

< 0

on an interval

(xo,

X2),

I(x)

decreases away from xo, then

I(xo)

is a local maximum.

Similarly, if

I'(x)

< 0 on

(Xl,

xo) and

f'(x)

> 0 on (xo,

X2),

then

f(xo)

is a local minimum.

(xo) = 0, the value of the second derivative at

furnishes an easy way to check

the sign of

f'(x)

on either side of

Xo.

f'(xo)

=0 and

f"(xo)

> 0, then

f'(x)

> 0 on some

interval to the right of

xo, and

f'(x)

< 0 on some interval to the left of

Xo.

It follows that

f(xo)

is a local minimum if I'(xo) =0,

(xo) >

Similarly, if

(xo) =0 and

(xo) < 0, then

f (xo) is a local maximum.

The common functions we deal with in

calculus-powers,

roots, exponentials, loga-

rithms, trigonometric functions, and inverse trigonometric

functions-all

have derivatives of

all orders on any open interval on which the function is defined. The notation for these suc-

cessive derivatives is as follows: if

y =

f(x),

then

_",

_ (4)

dx - f (x),

- f (X),

f (X), dx" - f (x),

....

We write

to indicate the derivative of whatever follows. For example,

f(x)

['(x),

(3x

+sinx) =6x +cosx.

Chapter9 • Derivatives and Graphs 53

The notation

then indicatesthe differentiation operator

applied n times, as suggested

by the notation

(dd )ny =

The third and higher order derivatives become important later

x x

whenwediscusspowerseries,butfor now we will be concernedonly with the firstand second

derivatives.

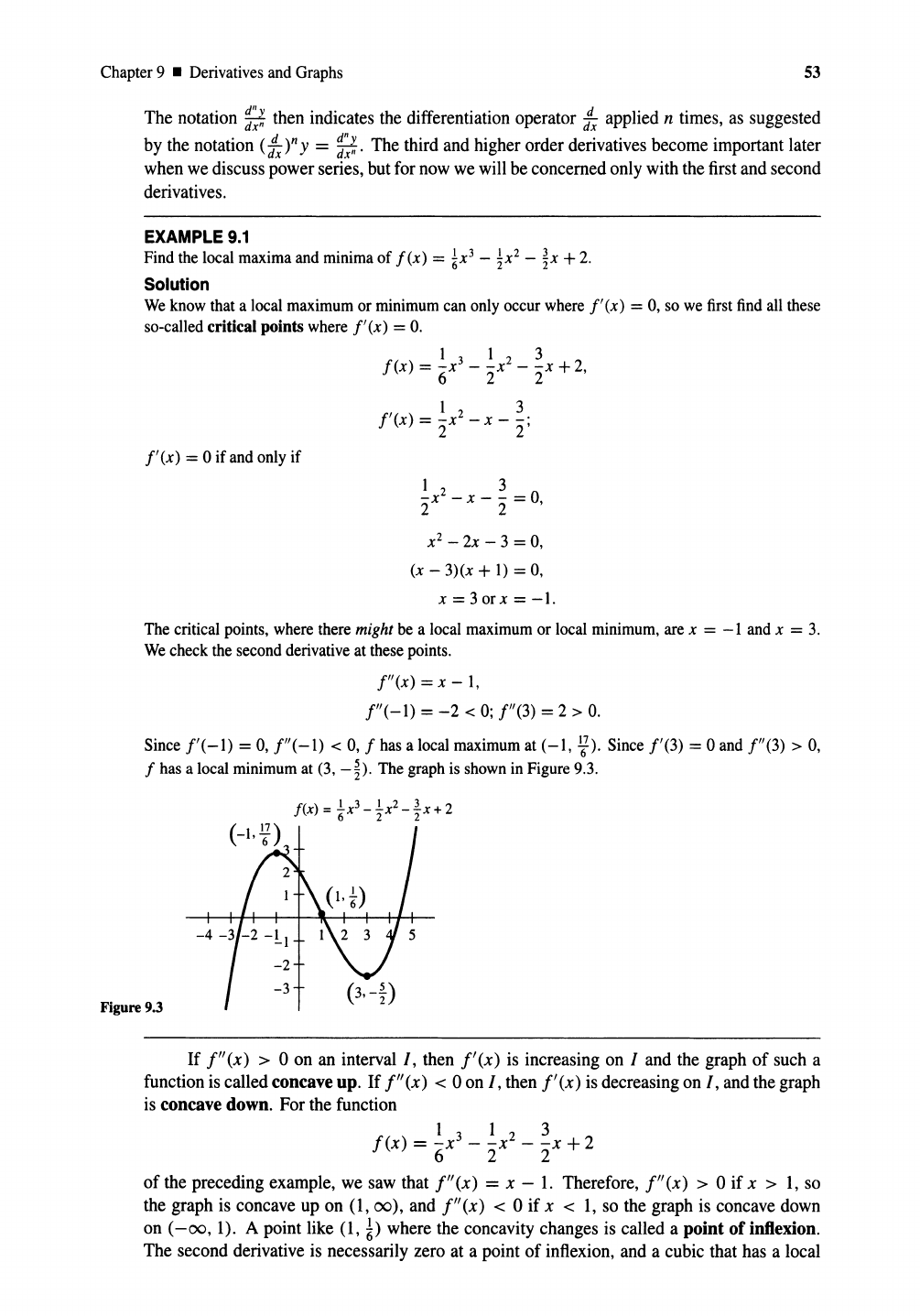
EXAMPLE

9.1

Find the local maximaand minimaof

I(x)

~x3

4X2

+ 2.

Solution

Weknow that a local maximumor

minimum

can only occur where I'

(x)

= 0, so we first find all these

so-called

critical points where

(x) =0.

1 3 1 2 3

I(x)

= 6

- 2

+ 2,

, 1

2 3

l(x)=2

-x-

I'(x)

=°if and only if

1 2 3

-x

- x - - = °

22'

2x - 3 = 0,

(x-3)(x+I)=0,

x = 3 or x =

-1.

The critical points, where there might be a local maximumor local minimum, are x =

-1

and x = 3.

Wecheck the secondderivative at thesepoints.

f"(x)

x-I,

1"(-1)

-2

< 0; f"(3) = 2 >

Since I'(

-1)

= 0,

(

-1)

< 0, f has a local maximumat (

-1,

¥).

Since

(3) = °and I"(3) > 0,

f has a local minimum at (3,

-~).

The graph is shown in Figure 9.3.

Figure 9.3

If I"

(x)

> 0 on an interval

then I'(x) is increasing on I and the graph of such a

functionis calledconcave up. If

I"(x) < 0 on

then I' (x) is decreasingon

andthe graph

is concave down. For the function

3 1

ix)

-x

+ 2

622

of the preceding example, we saw that I"(x) =

x-I.

Therefore, I"(x) > 0 if x > 1, so

the graph is concave up on (1,

(0),

and

I"(X)

< 0 if x < 1, so the graph is concave down

(-00,

1). A point like (1,

where the concavitychanges is called a point of inflexion.

The second derivative is necessarily zero at a point of inflexion, and a cubic that has a local

(9.1)

Understanding Calculus

maximum atXl andlocalminimumat X2 willalwayshaveapointofinflexion midwaybetween

them at

(Xl +x2)/2 (see Problem9.16).

The graph of any equationin

X and y is some sort of curve in the plane, but this curve

need not be the graph of a function. For example, the equationx

+ y2 = 1does not define y

as a function of x, sincetherearetwovaluesof y for eachX in (

-1,

1).

However,

if werestrict

our attentionto part of the

graph-say

the top half of x

I-we

do get the graph of a

function (in this case y =

.JT=X2).

It is easy to find

for such functions y whose graphs

form part of the graph of an equation. We simply differentiate both sides of the equationto

get an equationfor

If you differentiate both sidesof x

= 1, you get

2x+2y-=O,

dy X

dx =-y

Noticethat the valueof

dependson both y and X as it must, since the slope on the top half

of the circle

(y =

.JT=X2)

is the negative of the slopeon the bottomhalf (y =

-.JT=X2).

Ingeneral,itmaynotbepossibleto solvefor y intermsof x. Nevertheless, theprocedure

above,

calledimplicit differentiation, will give a valuefor

at any point of the curve.

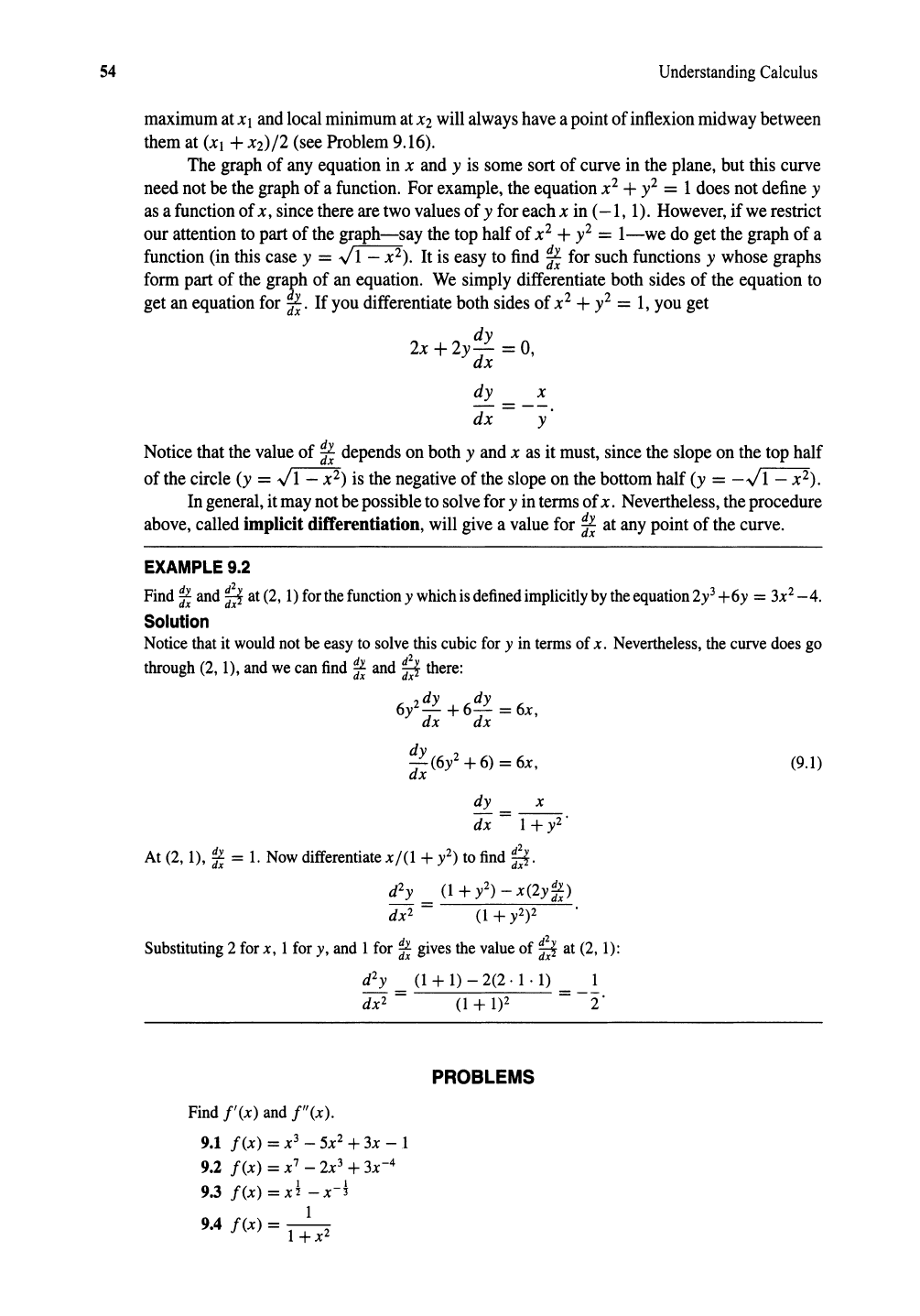
EXAMPLE

9.2

Find

and

at (2, 1)forthefunction y whichis

defined

implicitly bytheequation2

+6y

= 3x

-4.

Solution

Noticethatit wouldnot be easyto solvethis cubicfor

y in termsof x. Nevertheless, the curve does go

through

(2,1), andwe can find

and

there:

dy +6

6x,

dx dx

+6) =

6x,

_ _

- 1 +

y2'

At (2, 1),

= 1. Nowdifferentiate

x/(1

+ y2) to find

(1 + y2)

-x(2y~)

(1 + y2)2

Substituting 2 for x, 1for y, and 1 for *givesthe valueof

at (2, 1):

y _ (1 + 1) - 2(2 . 1 . 1) 1

- (1 + 1)2 2

PROBLEMS

Find

!'(x)

and

!"(x).

9.1

!(x)

= x

+ 3x

-1

9.2

!(x)

3x-

9.3

!(x)

=x~

-x-!

9.4

!(x)

--2

l+x

Chapter9 •

Derivatives

and Graphs

Find

and g.

dx dx

9.5 y = e

x 2

9.6 y = sin2x - cos3x

9.7 y =sin-

_IX

9.8 y = tan

Findthe localmaximaand minimaand graphthe

curve.

9.9 y = x

9.10 y = x

+3x

9.11 y = 4x

9.12 y = x

(1 - x). Hint: If

= 0 at a criticalpoint,consider

on eitherside.

9.13 y = x

+4x +3

9.14 y = x

+9x - 2

9.15 Showthat the local

maximum

of y = x +

is less than the local

minimum.

9.16 If

I(x)

is a cubic polynomial that has a local

maximum

at x = a and a local

minimum

at x = b, then I has an

inflexion

point at x =

Q!b,

which is

halfway

from a to b.

Hint: If I (x) is cubic, then I'(x) is a quadratic.

Moreover,

I'(a) = I'(b) = 0, so

I'(X)

k(x

a)(x

- b).

9.17 Findthe dimensions of therectangle of largestarea that has a perimeter of 40 in.

9.18 A panis to be madefroma 12"squarepieceof tin by cuttingsquaresoutof the cornersand

folding

up the sides. Whatis the

maximum

volume

of such a pan?

9.19 What is the ratio of height

(h) to radius

(r)

if a can of

volume

V is to have

minimum

total

area?

(A = 2Irr

+Znrh; V =

Irr

2h.

V is

given,

so h = V

/Irr

9.20 (i) What is the largestproductof two positive integers whose sum is 10; that is, what is

the largestof the products 1

·9,2·8,3

·7,4·6,5

·5?

(ii) What is the largestproductof twopositive

numbers

whosesumis a givennumberB?

(N.B.numbers, not integers.)

9.21 Find the radius

x and height h of the largestcylinderwhichcan be inscribed in a cone of

.radius

R andheight H.

9.22 Findthe point (x, y) on the line y = 3 -

x whichis closestto (0, 1). Hint: Let s be the

squareof the distanceand find

(x, y) on the line so s is

minimum.

9.23 Findthe point

(x,

y) on the curvey2 =

2(x

- 1) whichis closestto the

origin.

9.24 Find the shortestladder that will fit over a fence h feet high to a wall b feet behind the

fence.

Hint: Let

()

be the angle the laddermakeswith the

ground.

Showthat the lengthis

h b d

0 h Ll h j

--:--e

-9

= w en tan(7 =

1·

9.25 The cost per hour of

running

a cargoboat is proportional to the cube of the speed

through

the

water.

Find the speed

through

the

water,

v, which

minimizes

the cost of a given trip

upriveragainsta a 5 mphcurrent.

Hint: The speedoverthe groundis v - 5, so the timeof

the trip is proportional to

(V~5).

9.26 A pieceof string100in longis cut intotwo

pieces.

Onepiece,of lengthx, is

formed

intoa

circle,andthe restinto a

square.

Let A(x) be the sumof the two

areas.

Findx sothat A(x)

maximum

andfindx so that

A(x)

minimum.

Hint: Thereis one

between0 and 100

such that

A' (xo) = 0, and you can easily tell whetherA(xo) is a

maximum

minimum.

To find the other

extreme

value,

considerhow

A(x)

behaves

on [0,xo] and on [xo, 100].

Remember

that x =0 (allcircle)and x = 100(all

square)

are possibilities.

Following

the

Tangent

Line

The tangent line to y = f (x) at

has the equation

y = f(xo) +

f'(xo)(x

- xo).

(10.1)

Consequently, if you know

f(xo) and f'(xo), you can calculate exactly the value of

yon

the

tangent line for any given

x. If x is close to xo, then the graph of f will be close to the tangent

line, and we can use the value of

y from (10.1) to approximate the value of f (x). In this

section, we examine several ways this approximation can be used.

Suppose we want a rough approximation for

J4.04

or J3.99 and the calculator is not

at hand. If

f(x)

JX,

then

f'(x)

1/2JX

and

f'(4)

We know that J4 = 2 and the

slope of the tangent at

x = 4 is

Therefore,

changes from J4 by about

the"

change in

x from 4. Thus,

. 1

J4.04

= 2 + 4(.04) = 2.01 and

J3.99

2 +

4(-.01)

= 2 - .003 = 1.997.

The notation

(x)

is frequently used for the change on the tangent line corresponding

toachangedx

from x,

anddyisthendefinedbydy

f'(x)dxtocorrespondtothe

f'(x)

notation. In the preceding example we would have

f(x)

JX,

x = 4, dx = .04, giving

dy = /A(.04) = .01.

The expression

(x)

is called the differential

f at x.

As another example of the use of the differential, suppose that your business is stamping

out circular pieces of sheet metal. The buyer cares not only about the accuracy in the radius,

but also about the accuracy in the weight of each piece. You therefore need to know how the

relative error in weight,

,depends on the relative error,

in the radius. If p is the density

(ounces per square inch, say), then

W =

pnr

and dW = 2pnrdr; hence,

2prrrdr

2dr

= =

pit

r? r

Understanding

Calculus

The relativeerror in weight is twice the relativeerror in the radius.

The number of years it takes to double your money at a compound interest rate r is

n =log

log(l+r).

I(x)

log(l+x),

then

I'(x)

1/(I+x).

Hence,1(0) =log 1 =0

and

1'(0) = 1. The change in log(1 +

from x = 0 is approximately 1 · x. Thus,

log(1 +

r. For example, at 5% (r = .05) and using .70 for a crude approximation

to log

2, we get

.70

70.

n = - = - = 14 years.

.05 5

This is thebusinessman'srule of thumb: The numberof yearsto doubleyourmoneyat interest

p% is 70/p. The formula for tripling your money is n = log3/log(1 +

1.10/ r. For

example,at 10%(r = .10), n = 1.10/.10 = 11 years.

Tangential approximation can also be used to evaluatecertain nonobvious limits of the

form

% using the following result:

l'Hospital's Rule: {{lim

I(x)

I(a)

= 0 and lim

g(x)

=g(a) =0, and

x~a x~a

I(x)

I'(x)

I'(a)

g'(a)

0, then hm

= hm

--a

x~a

g(x)

x~a

g'(x)

g'(a)

To verify this, noticethat if we define81

(x)

I(x)

I(a)

_ 1'( ) _

()

a - 81 X ,

x-a

then 81 (x)

0 as x

a since

(a) is the limit of the difference quotient

(x) -

j'(a))/(x

- a). Therefore,since

I(a)

=0,

I(x)

(a)(x - a) +81

(x)(x

- a)

where81

(x)

0 as x

a. Similarly,

g(x)

g'(a)(x

- a) +82(X)(X - a)

where82(X)

0 as x

a. Therefore,for x

::f=

!(x)

!'(a)(x

- a) +81

(x)(x

- a)

--=---------

g(x)

g'(a)(x

- a) +82(X)(X - a)

!'(a)+81(x)

!'(a)

~--

g'(a)

+ 82(X)

g'(a)

To apply I'Hospital's Rule, firstcheck that

!(a)

g(a)

= 0, and then write

lim

I(x)

= lim

f'(x).

x~a

g(x)

x~a

g'(x)

For example,

- l

lim

= lim - = 1;

x~o

. 1 - cosx li sinx 0

Im--=

x~o

limx~a

(x)

/ g'

(x)

also has the form

then apply I'Hospital's Rule again:

. 1 - cosx li sinx

1m =

Im--

x~o

. cosx 1

=hm--=-.

x~o

2 2

Chapter 10 • Following the Tangent Line

We can also use tangential approximation to find numerical estimates for the roots of

equations. In geometric terms, to solve the equation

(x)

= 0 means to find the numbers r

where the curve y = ! (x) crosses the x-axis. If xI is a first approximation to a root r, then

we get a better approximation,

X2, by following the tangent line back to the x-axis as shown

in Figure 10.1.

Newton'smethod

Figure 10.1

The line from (X2, 0) to

(XI,

!(Xl))

has slope

!'(XI),

(10.2)

The number

X2 determined by (10.2) will generally be a better approximation to the root r than

XI.

We can then use the same formula to get a better approximation, X3, from X2:

This technique is called Newton's method.

EXAMPLE 10.1

Use Newton's method to approximate ,J3.

Solution

We let

f(x)

= x

- 3 and search for a root of the equation

f(x)

Since

f(l)

< 0 and

f(2)

> 0,

there will be a root between 1 and 2, and we start with

Xl = 1.5. Since

f'(x)

= 2x, we have

5 (1.5)2 - 3

X2 = 1. - 2(1.5)

(2.25 - 3)

1.5-

---

3.0

.75

= 1.5 + 3 = 1.75.

Understanding Calculus

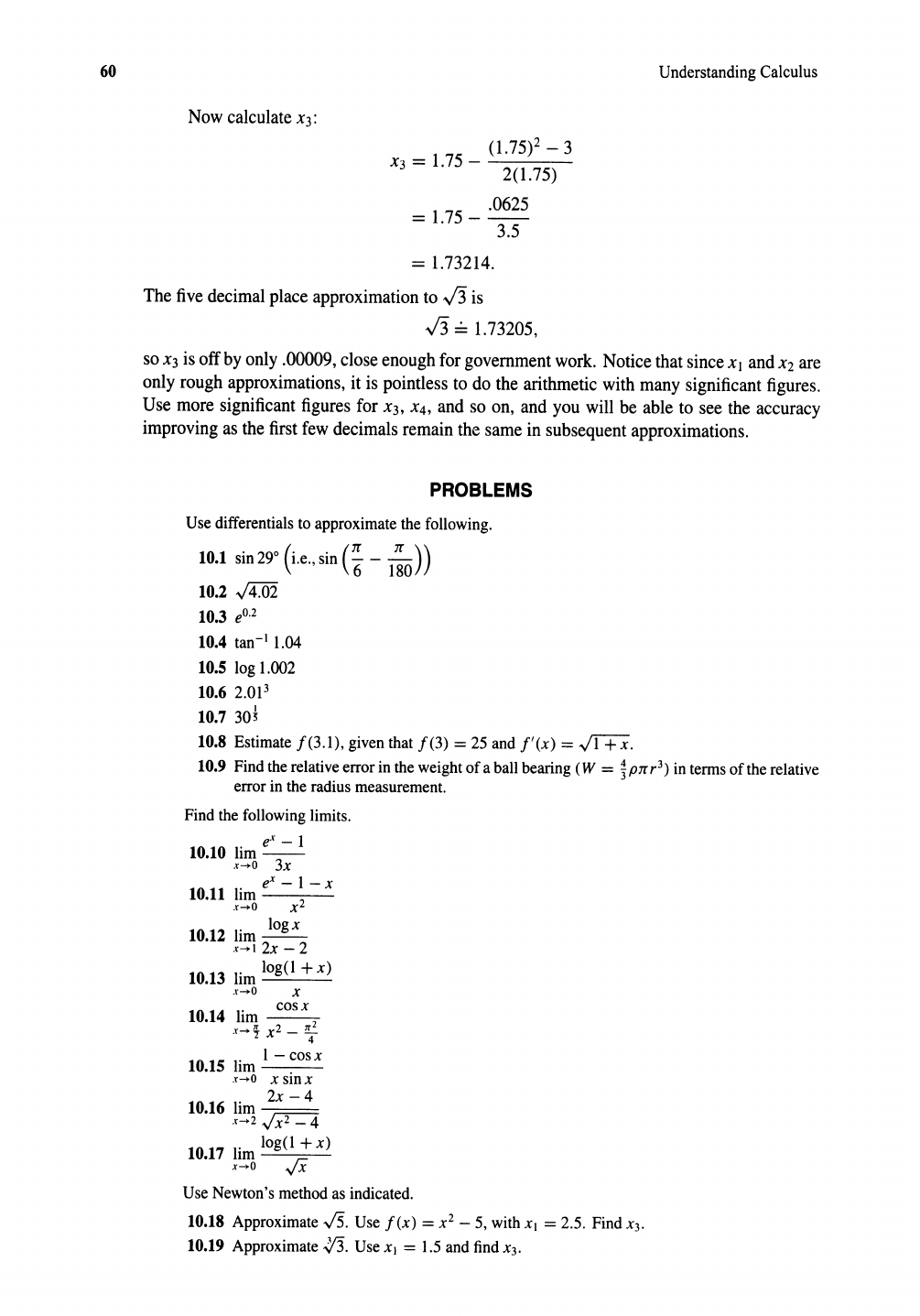
Nowcalculate X3:

(1.75)2

- 3

X3 = 1.75-

----

2(1.75)

.0625

= 1.75

---

3.5

= 1.73214.

The

five

decimal

place approximation to J3is

1.73205,

X3 is offbyonly.00009, closeenoughfor government

work.

Noticethat sinceXl andX2 are

only rough approximations, it is pointless to do the arithmetic with many significant

figures.

Use more significant

figures

for X3, X4, and so on, and you will be able to see the accuracy

improving as the

first

fewdecimals remainthe samein subsequent approximations.

PROBLEMS

Use differentials to approximate the following.

10.1 sin

29°

(i.e.,sin

l~O))

10.2 J4.02

10.3

eO.

10.4 tan" 1.04

10.5 log 1.002

10.6 2.01

10.7 30!

10.8

Estimate

f(3.1),

given that

f(3)

= 25 and

f'(x)

JT+X.

10.9 Find the relative error in the weight of a ball bearing

= 1pJrr

)

in terms of the relative

error in the radius measurement.

Find the following limits.

- 1

10.10

lim--

x~o

e' -

I-x

10.11

lim 2

x~o

10.12 lim

_lo_g_x_

x~l

2x - 2

10.13 lim log( 1+

x~o

. cosx

10.14

x~"!

X -

4""

1 - cosx

10.15 lim

--.--

x~o

X smr

-4

10.16 lim

x~2

",x

10.17 lim 10g(1 +

x~o

y'X

Use Newton's method as indicated.

10.18 Approximate

J5.

Use

f(x)

= x

5, with

= 2.5. Find X3.

10.19 Approximate

c/j.

Use

= 1.5 and find X3.

Chapter 10 • Following the

Tangent

Line

10.20 Approximate the root of x

x-I

= 0 which lies between 0 and 1. Use Xl = .5 and

continueuntil you are sure of threedecimalplaces.

10.21 (i) Showthat

xe'

- 1 =0 has exactlyone root.

Hint:

f(x)

xe'

- 1,then

f(x)

< 0

X < 0, and

(x) > 0 if x >

(ii) Approximate the root of

xe'

- 1 = 0 to three decimalplaces.