Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

Chapter 14 • Parametric Equations

PROBLEMS

Graph the following curves. Notice that both x and y have a specific finite range of values for all

these curves. Indicate these ranges clearly on your graph.

14.1 x = 1, Y =1

0:::: t

::::

14.2 x =

(2,

Y =

- 1, 0:::: 1

::::

14.3 x = 2 cos t, Y = sin 1, 0:::: 1

::::

14.4 x = f' y = t,

1::::

::::

Write the Cartesian equations of the following curves, and graph them.

14.5

x=sin

2t+l,

y=COS(,

-00<1<00

14.6x=t

y=21

- 1,

-00<1<00

14.7 x = 1 - e', y =e";

-00

< t <

2 2 tt

• X = sin 1, Y = cos 1, 0:::: 1

::::

Find the equation of the tangent line at the indicated point. Does the curve lie under or over the

tangent line near this point?

14.9 x = 21

1, y = 1

1 = 2

14.10x=e

y=cost,

t=O

14.11 x = t

- 1, y = t

+ I, t = 113

14.12 x = e'; y = e",

14.13 A ball is thrown at an angle of 45° with the ground and an initial velocity of 64 ft/sec. Find

how high it goes by finding when

14.14 If a body is projected upward at an angle

we have seen that its path is given by x =

(v cos e) t, Y = (v sin e) 1 - 16t

where v is the initial velocity. At what time does the

body reach maximum height, and when does it hit the ground? At what angle does the

curve hit the ground? What is the slope of the curve when the body hits the ground?

Find the lengths of the following curves.

14.15 x = a cos 1, y =a sin t, 0

21f

8 3

14.16 y =

0:::: x s 5

14.17 x =

v'T+"fI,

y = log(t + v'T+"fI), 0

( < 5

14.18

x = tan:" t, y = ! log(l + t

) ,

14.19 x = e' cos t, y = e' sin t, 0

14.20 y = cosh x, 0

(See Problem 7.28, Chapter 7.)

14.21 Find the parametric equations of the hyperbola

= 1 using the parameter edefined

x = asec

Hint: tan?e+ 1 = sec?

14.22 Find the parametric equations of the parabola ay =x

using as parameter the slope of the

line from

(0,0)

(x,

y).

14.23 If a circle rolls along the x-axis, the point P on the circle that starts at

(0,0)

traces out a

cycloid. Show that the parametric equations are

x =

- a sin

y=a- a cos

where

a is the radius of the circle and eis the angle through which the radius to P has turned.

14.24 Find the parametric equations of the ellipse

= 1 using as parameter the angle e

defined by x = a cos

14.25 A rod AB moves with its end A on the y-axis and its end B on the x-axis. Find the

parametric equations of the curve traced out by the point

P on the rod which is a units

from

A and b units from B. Hint: Use as parameter the angle ethe rod makes with the

x-axis.

Change

Variable

Wehavealreadyseen that most integration problems

involve

the chainrule in reverse, and the

u-substitution is a useful way to keep track of the constantsin these problems. For example,

to integrate

2(x

+ 1)4 dx

we firstrecognizethat x

is the essentialpart of the derivative of x

+ 1,and we let

u =x

+ 1, du =3x

dx,

dx =

duo

This gives

x2(x3 + 1)4dx =f

~U4

du =

..!..u

= ..!..(x

+ 1)5. (15.1)

3 15 15

For definite integralswe can carry this techniqueone step further and incorporate the

limits of integration in the change of variable. For example, using the

final

answerin (15.1)

we can evaluate the following definite integral:

1 x2(x3 + 1)4 dx = ..!..(x3 + 1)5]1 = 2

-1

Here we ignored the intermediate steps involving u and used just the

final

antiderivative

(~)(x3

+ 1)5. Instead of this approach, notice that if u = (x

+ 1), then u = 0 when

x =

-1

and u = 2 when x = 1. Therefore, we can make the u-substitution with the new

limits:

1 X\X3 + 1)4 dx =

~U4

du = "!"u

5]2

..!..

(15.2)

-1

3 15 0 15

The generalrule for changingthe variable in a definite integralis the following

formula:

lU(b)

f(u(x))u'

(x) dx =

f(u)

duo

(15.3)

u~)

(15.4)

(15.5)

UnderstandingCalculus

In Equation

(15.2),

u =x

+ 1,

!(u(x»

3(x

+ 1)4,

u'(x)dx

= 3x

2dx,

!(u)du

= 3u4du,

u(-I)

=0,

u(l)

=2.

To verify the changeof variable equation (15.3), we let

F(x)

be any antiderivative of

!(x),

F'(x)

!(x).

follows

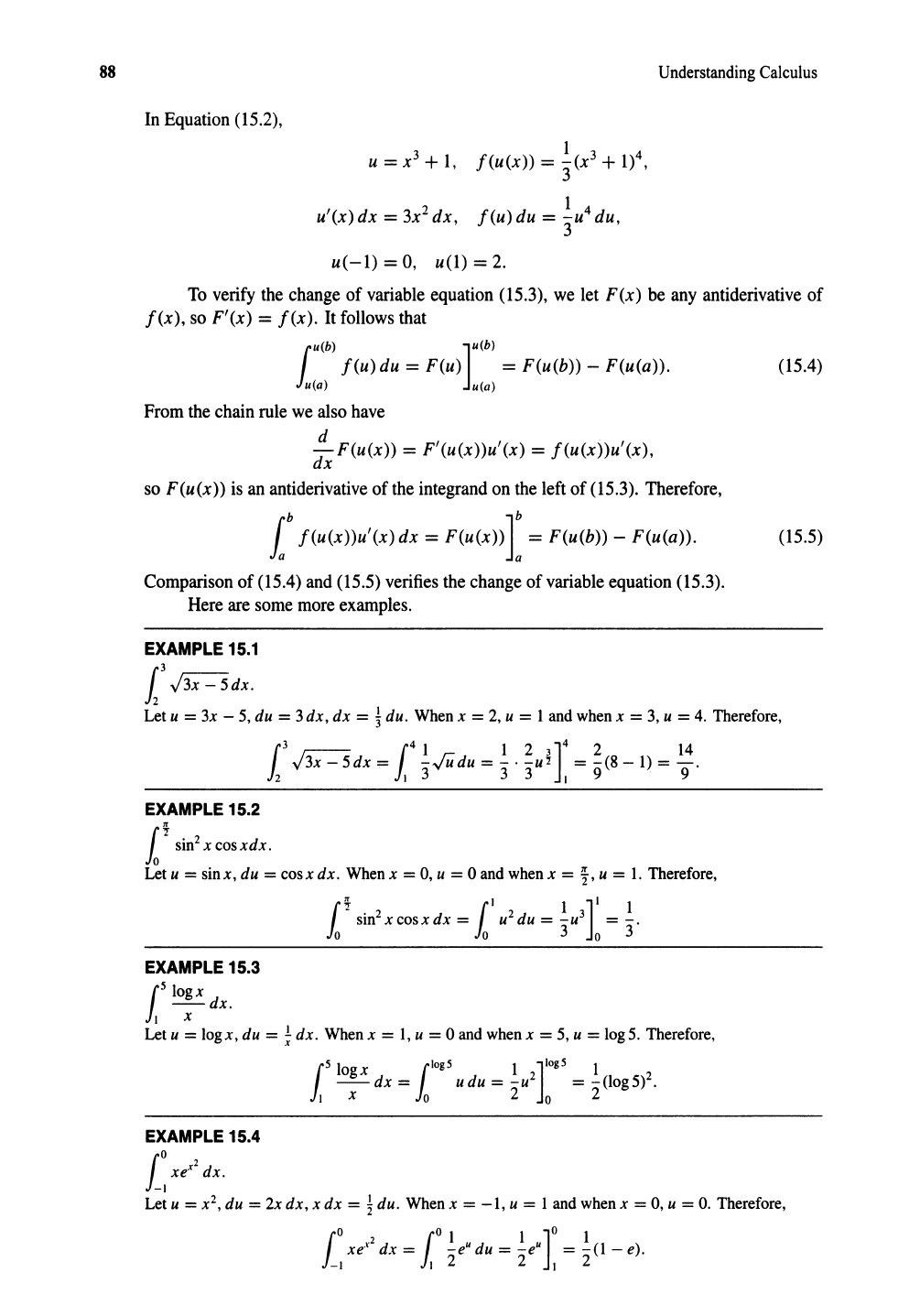
that

JU(b)

f(u)

du =

F(u)

. =

F(u(b))

F(u(a)).

u(a) u(a)

Fromthe chainrule we alsohave

d " . ,

-F(u(x))

= F

(u(x))u

(x) =

j(u(x))u

(x),

F(u(x))

is an anti

derivative

of the integrand on the left of (15.3). Therefore,

!(u(x»u/(x)

dx = F(u(x» I= F(u(b» -

F(u(a».

Comparison of (15.4)and (15.5)

verifies

the changeof variable equation(15.3).

Hereare somemoreexamples.

EXAMPLE

15.1

·J3x - 5dx.

Let u = 3x - 5, du =

3dx,

dx = 1

duo

When x = 2, u = 1 and when x = 3, u = 4. Therefore,

1 1 2

3]4

2 14

J3x-5dx=

-y'Udu=-.-u'1

=-(8-1)=-.

2 1

EXAMPLE

15.2

sirr'x cosxdx,

Let u = sinx, du =

cosx

dx. When x = 0, u = 0 and when x = I' u = 1. Therefore,

1]] 1

o sirr' x cosx dx = 0 u

2du

3"u

0 =

3"'

EXAMPLE

15.3

5 logx dx.

1 X

Let u = logx, du =

dx. When x = 1, U = 0 and when x = 5, u = log5. Therefore,

5 logx 1

108 5

]10

-dx

udu

= _u

= _(log5)2.

1 X ° 2 ° 2

EXAMPLE

15.4

0 xex2dx.

-1

Let u = x

, du = 2x dx, x dx =

duo

When x =

-1,

u = 1 and when x = 0, u =

Therefore,

1°

1 1

dx = _e

du = _e

-(1

- e).

-1

) 2 2 1 2

Chapter 15 • Change of Variable

The answer is negative, which we expect since the first integrand xe

x 2

is negative on

[-1,

0]. The

integrand after the substitution,

~eu,

is positive,but the integration is from right to left (from I to 0), so

the result is negative.

We have already used the important differentiation-integration formulas

_II!

-1

tan x = 1 +x

;

1 +x

=tan x;

sin-

x . !

sin-

=~'

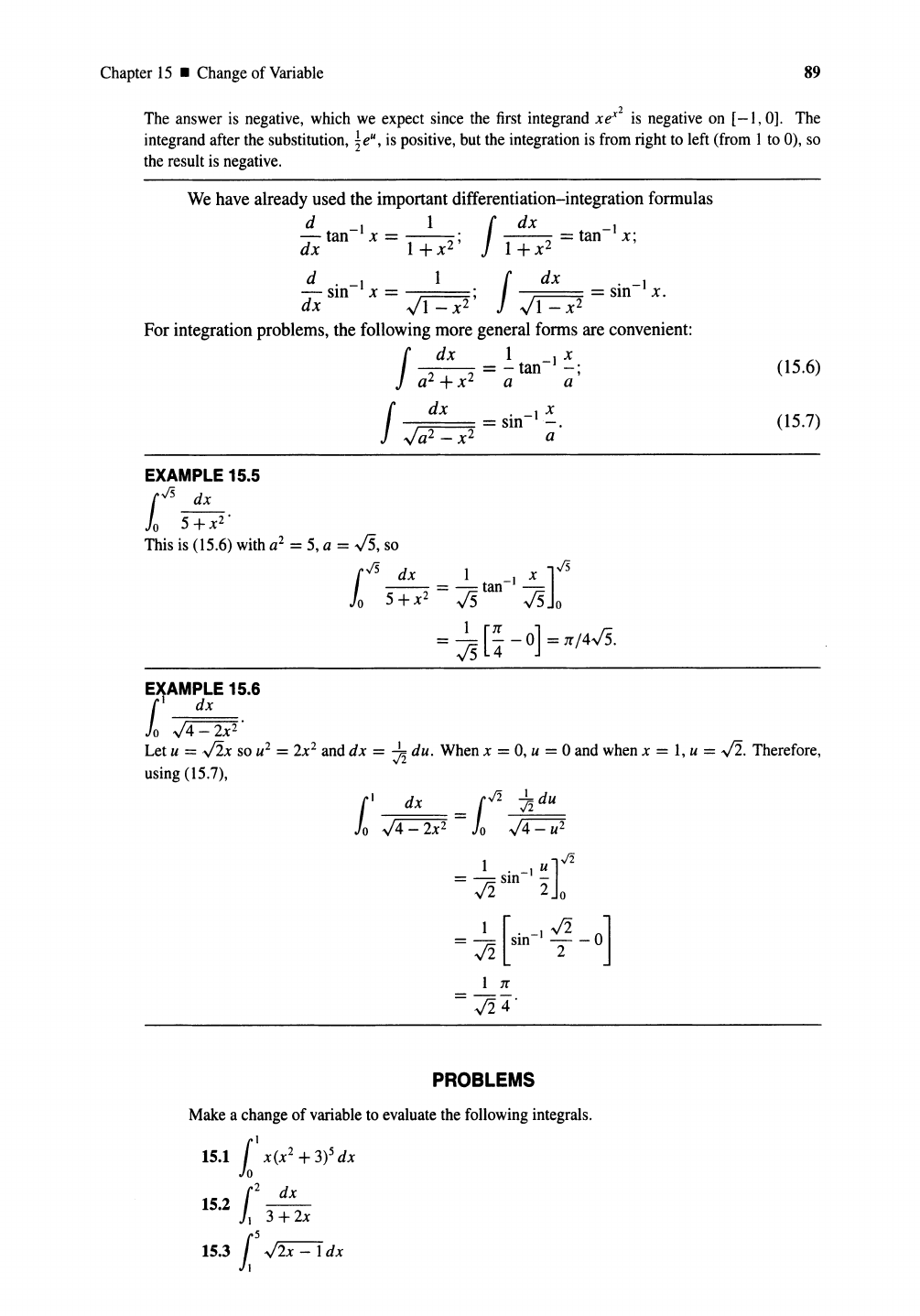
For integration problems, the following more general forms are convenient:

-I

~tan

-1

~::;::::::==:;:

Sln-.

2-x

EXAMPLE

15.5

[v'S

5 +x

•

This is (15.6) with a

=5, a =

J"S,

[./5

dx 1 x

]./5

10 5 +x

.J5

tan-I

.J5

~[~-O]=1T/4J5.

(15.6)

(15.7)

EXAMPLE

15.6

- 2x

Let u = ·../2xso u

= 2x

and dx =

duo

When x = 0, u = 0 and when x = 1,u =

,,/2.

Therefore,

using (15.7),

[../2

- 2x

- u

1 .

-1

U]v'2

= ..j2 sin 2 0

[Sin-

-0]

1 1r

,,/24".

PROBLEMS

Make a change of variableto evaluate the following integrals.

15.1 1

x(x

+ W

15.21

1 3

+2x

15.3 1

.J2x

- 1dx

15.4

i:X~dX

15.5 1

cosx sinx dx

15.6

v'sinx cosx dx

15.71

log2x dx

1 x

15.81

(logx)3 dx

e x

15.9 rx

+ 1 dx (Divide

first.)

x + 1

15.10 1

3xe

x 2

e../X

15.11 I

.JX

15.12

4 dx 2

15.13

1 + 4x

15.14

xdx

1+ x

15.15

1 + e

15.16

J16

- x

15.17 r

5 - 3x

15.18

sin2x dx

15.

9 dx 2

+4x

15.20. r

J16

- 9x

15.21

1 4 + 25x

15.22 (7 2x + 1

+ 5

15.23

x(x

VOdx

Let u =

x-I.

15.24 1

xv'x

+2dx Let u = x + 2.

x+2

15.25

~dx

+ 1

15.26 1

2(x

- 1)

15.27

~dx

Understanding Calculus

Integrating

Rational

Functions

rational

function is an expression

~~~~,

where

P(x)

and

Q(x)

are polynomials. There is

a standard algorithm for finding the antiderivative of any rational function in which you can

completely factor the denominator. In most cases, the method requires a ridiculous amount of

algebra and is not practical. However, if Q(x) is linear or quadratic, or a product of distinct

linear factors, the method is quite straightforward.

The first thing to notice when integrating any rational function

~~:~

is that if

P(x)

has

degree greater than or equal to the degree of Q(x), you can divide and get

P(x)

R(x)

Q(x)

S(x)

Q(x)'

where

S(x)

is a polynomial, and

R(x)

has degree strictly smaller than that of

Q(x).

The

polynomial S

(x)

is easy to integrate, so we only have to consider rational functions

~~~~

which the numerator has smaller degree than the denominator. IMPORTANT: Firstdivide ifyou

can.

Now we consider only cases where the numerator has smaller degree. If

Q(x)

is linear,

the integration is straightforward.

- dx = k log [x - a

x-a

Now suppose

Q(x)

is quadratic. We can always factor out the coefficient of x

so we

assume

Q(x)

has the form

Q(x)

= x

+ C. There are three cases to consider:

(i)

Q(x)

= (x - a)2

(ii)

Q(x)

= (x -

a)(x

- b) with a -I b

(iii)

Q(x)

= x

+ C with B

4C < 0, so

Q(x)

does not factor.

We illustrate each case with a specific example which shows the technique.

Understanding Calculus

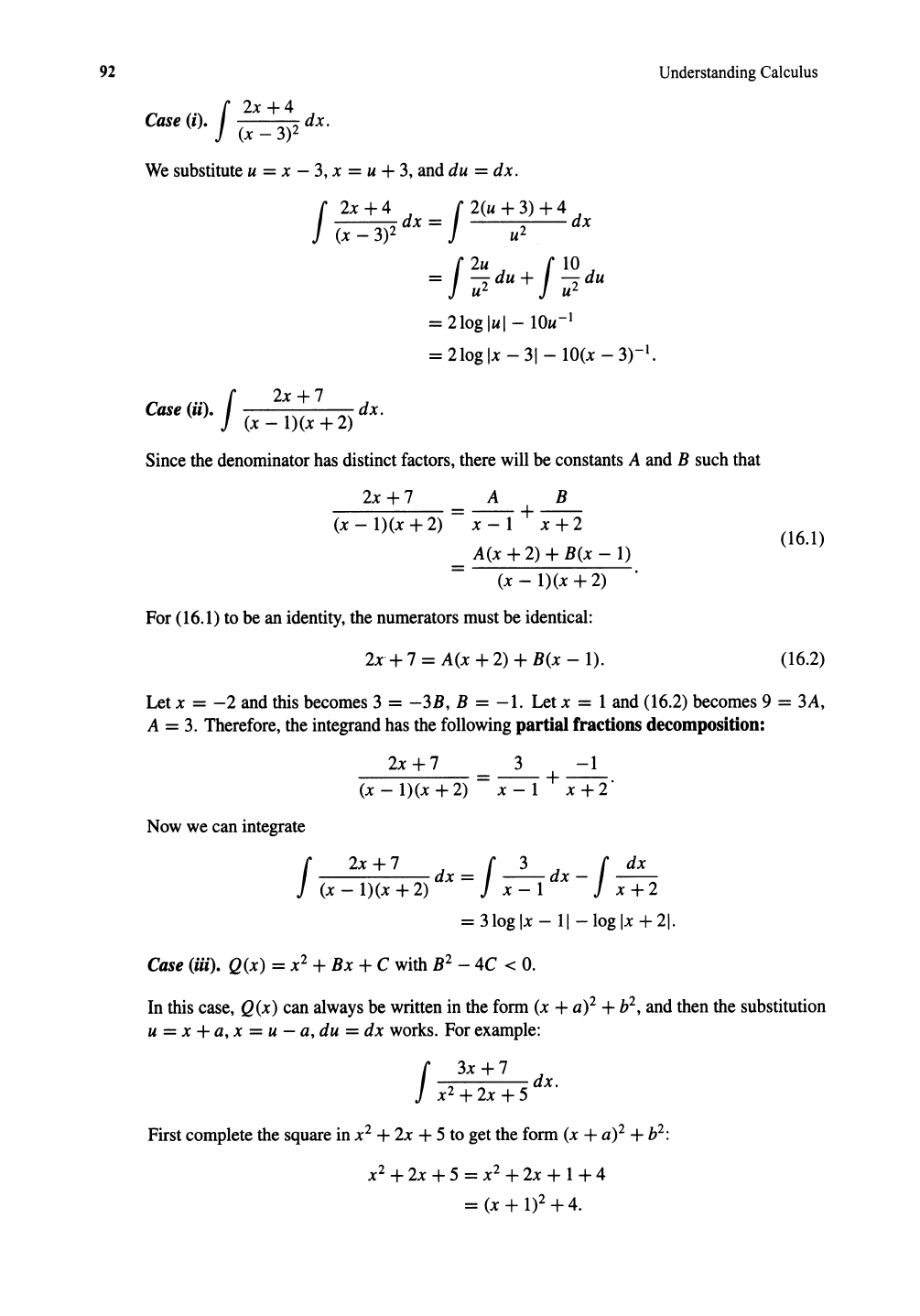
e / 2x

Case

(I). 2

dx.

(x - 3)

Wesubstituteu =x - 3, x =u + 3, and du =

dx.

+ 4

= / 2(u + 3) + 4

(x - 3)2 u

2u /

-du+

-du

=2 log

lul-

IOu-

= 2 log Ix -

31-

10(x - 3)-1.

(

ee) / 2x

ase

• x.

(x -

I)(x

+2)

Since the denominatorhas distinctfactors,there will be constants A and B such that

2x+7

A B

-----

+--

(x -

I)(x

+2) x - I x + 2

A(x

+ 2) +

B(x

- I)

(x -

I)(x

+ 2)

For (16.1) to be an identity, the numeratorsmust be identical:

2x' + 7 =

A(x

+ 2) +

B(x

- I).

(16.1)

(16.2)

Let

x =

-2

and this becomes 3 =

-3B,

B =

-I.

Let x = I and (16.2) becomes 9 = 3A,

A =3. Therefore,the integrandhas the following partial fractions decomposition:

2x+7

-I

-----=--+--.

(x - 1)(x +2)

x-I

x +2

Now we can integrate

2x+7

dx=/_3

dX-/~

(x -

I)(x

+2)

x-I

x +2

= 3log [x -

- log [x +

21.

Case

(iii).

Q(x)

+ C with B

4C <

In this case,

Q(x)

can always be written in the form (x + a)2 + b

and then the substitution

u =x +a, x =u - a, du =

works. For example:

+ 2x + 5 x.

First completethe squarein x

+ 2x + 5 to get the form (x +

a)2

+ b

+ 2x + 5 = x

+2x + 1 + 4

(x + 1)2 + 4.

Chapter 16 • Integrating Rational Functions

Now let u =x + 1, x =u - 1, du =

dx:

3x + 7 dx - f 3x + 7

2+2x+5

(x+l)2+4

=f 3(u - 1) + 7 du

=f3u

3 f

2udu

f du

--+4

- 2 u

+4 u

=2:1oglx2+2x+51+2tan-1

-2-

The partial fractions technique we used for quadratic denominators of the form

(x - a) (x - b) will actually work for any rational function

~~~~,

where R(x) has degree

less than that of

Q(x),

and

Q(x)

can be completely factored into linear and unfactorable

quadratic factors. For example, if

Q(x)

= (x -

a)(x

b)(x

- c), with a, b, c distinct, then

there are numbers

A, B, C such that

R(x)

ABC

-------

--.

(x -

a)(x

b)(x

- c) x - a x - b x - c

If Q(x) has repeated factors, then these factors must occur in the partial fractions. For

example,

R(x)

ABC

D E

-----=--+

+--+

(x - a)3(x - b)2 x - a (x - a)2 (x - a)3 x - b (x - b)2

Q(x)

has both linear and nonfactorable quadratic factors, then the decomposition

looks like the following.

R(x)

+ C

(x -

l)(x

+ 4) =

x-I

+ (x

+ 4) ,

(16.3)

R(x)

ABC

Dx+E

Fx+G

--------

+ + +

---

(x -

1)(x

- 2)2(x

+4)2

x-I

x - 2 (x - 2)2 x

+4 (x

4)2'

(16.4)

We know how to integrate all the terms on the right above except

(X2~4)2'

and we will see how

to do this by trigonometric substitution in Chapter 19. The following example illustrates how

to find the constants

A, B, C, and so on.

EXAMPLE 16.1

5x2 - 3x + 13

-----dx.

(x -

1)(x

+4)

Understanding Calculus

The partial fractions decomposition is (16.4):

2-3x+13

Bx+C

-----

---

(x - 1)(x

+ 4)

x-I

(A + B)x

+ (C - B)x + (4A - C)

(x -

1)(x

+4)

For this to be an identity we must have

A+B=5

C - B

=-3

4A - C = 13.

Adding the corresponding sides of the first two equations gives

A+C=2

4A - C = 13.

Adding again, we find

5A = 15, A = 3; then from die first equation, B = 2, and from the second

equation C

-1.

Thus,

5x2 - 3x + 13 d J 3 J2x - 1

--dx+

--dx

(x - 1)(x

+4)

x-I

,.,

1 I X

= 310glx -11 + log

Ix'"

+41-

-tan-

2 2

PROBLEMS

16.1 Jx

2 dx

16.2 J

(x - 3)

16.3 J

_3_

4x + 1

16.4 Jx + 1 dx

x-I

16.5 f x

+: + I dx

16.6

--dx

x+4

16~

(x - 5)(x - 2)

1~8

(x + 1)(x - 2)

16.9 J

xdx

(x -

l)(x

- 2)

16.10

--/:-

-1

16.11

--2

4-x

Chapter 16 • Integrating Rational Functions 95

16.12 f dx

+ 2x + 2

16.13 f dx

+4x + 5

16.14 f

x + I

2x + 1

16.15 x

+9 dx

16.16

f x

I dx

16.17 f x2

+::

+10 dx

16.18

f dx

+ 3x + 2

3x-6

16.19"

8 dx

+4x

16.20

f 5x

x + 3 dx

(x + 1)(x

+ 1)

16.21 f 6x

+ 11x + 8 dx

x(x

+ 2x + 2)

16.22 f 2x

lOx+ 2 dx Hint:

(x -

l)(x

+ 2)(x - 3)

- lOx+ 2

ABC

-------

(x-I)(x+2)(x-3)

x-I

x+2

x-3

is equivalent to

- lOx+ 2 = A(x + 2)(x - 3) + B(x - 1)(x - 3) + C(x - 1)(x + 2).

Let x = 1 to find A, x = - 2 to find B, and x = 3 to find C.

16.23 f 2x

+7x + 4 dx

x(x

l)(x

+ 2)

16.24 (i) Find A, B, C so that

x(x

+4 ) =

~'2~;'

(ii) Integrate J

x(x

+4 ) dx.