Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

Integration

Parts

Integration by parts is the name given to the product rule for differentiation when it is used

as an integration technique. From the differentiation formula

d "

(f(x)g(x))

f(x)g

(x) +

g(x)f

(x),

we get the integration formula

f(x)g(x)

= f

f(x)g'(x)dx

g(x)f'(x)dx,

or, equivalently,

f(x)g'(x)

f(x)g(x)

- !

g(x)f'(x)

dx.

(17.1)

(17.2)

(17.3)

Formula (17.1) allows you to trade one integral, J

f(x)g'(x)

dx,

for another, J

g(x)f'(x)

dx.

The trick is to recognize the cases in which the second integral is simpler than the first.

The utilitarian form of (17.1) is

udv

= uv - !v du,

where we have let u =

f(x),

dv = g'(x)

dx,

so v = g(x), and du =

f'(x)

dx.

A noteworthy particular case of equation (17.1) is the caseg (x) = 1, or, in the notation

of (2),

dx.

In this case,

f(x)dx

xf(x)

- f

xf'(x)dx.

Sometimes

xf'(x)

is easier to integrate than

f(x),

and we will use (17.3) to integrate

logx

and the inverse trigonometric functions.

EXAMPLE 17.1

flogxdx.

Welet u =logx, dv =

dx,

so v =x, and du =

dx. Then

flog x dx = x logx - fx .

=xlogx

- f dx

xlogx

-x.

Understanding Calculus

(17.4)

Since log x is one of our standard elementary functions, (17.4) should be considered one of

our standard integration formulas. It wouldn't hurt to memorize it.

EXAMPLE 17.2

fsin-I x dx,

Let u = sin" x, dv =

dx,

so v =x and du =

dx.

Thus,

I-

x 2

sin"! x dx = x sin-

x - f n dx

1- x

= x sin-I x

+.JI=X2.

The technique of Example 17.2 also works easily for

cos-

x, tan" x, but these integrals

occur infrequently, and it is easier to go through the integration technique or look in the

integration table than it is to memorize the formula.

The more usual situation, where

is not simply

dx,

is illustrated in the next example.

EXAMPLE 17.3

fxe" dx.

Let u =x, dv =

dx,

so v =

and du = dx. Thus,

xe'

dx =

xe'

- f

For definite integrals the integration by parts formula is

1u dv

uv a

-1

vdu.

EXAMPLE 17.4

x~/2dx.

Here we let u =x, dv = e

x/ 2,

so v =

~ex/2

and du = dx. Then we

have.

1\e

= x

-1

~eX/2

= 2 .

. e _

/2]

2 0

=e - (e - 1) = 1.

Chapter 17 • Integration by Parts

EXAMPLE

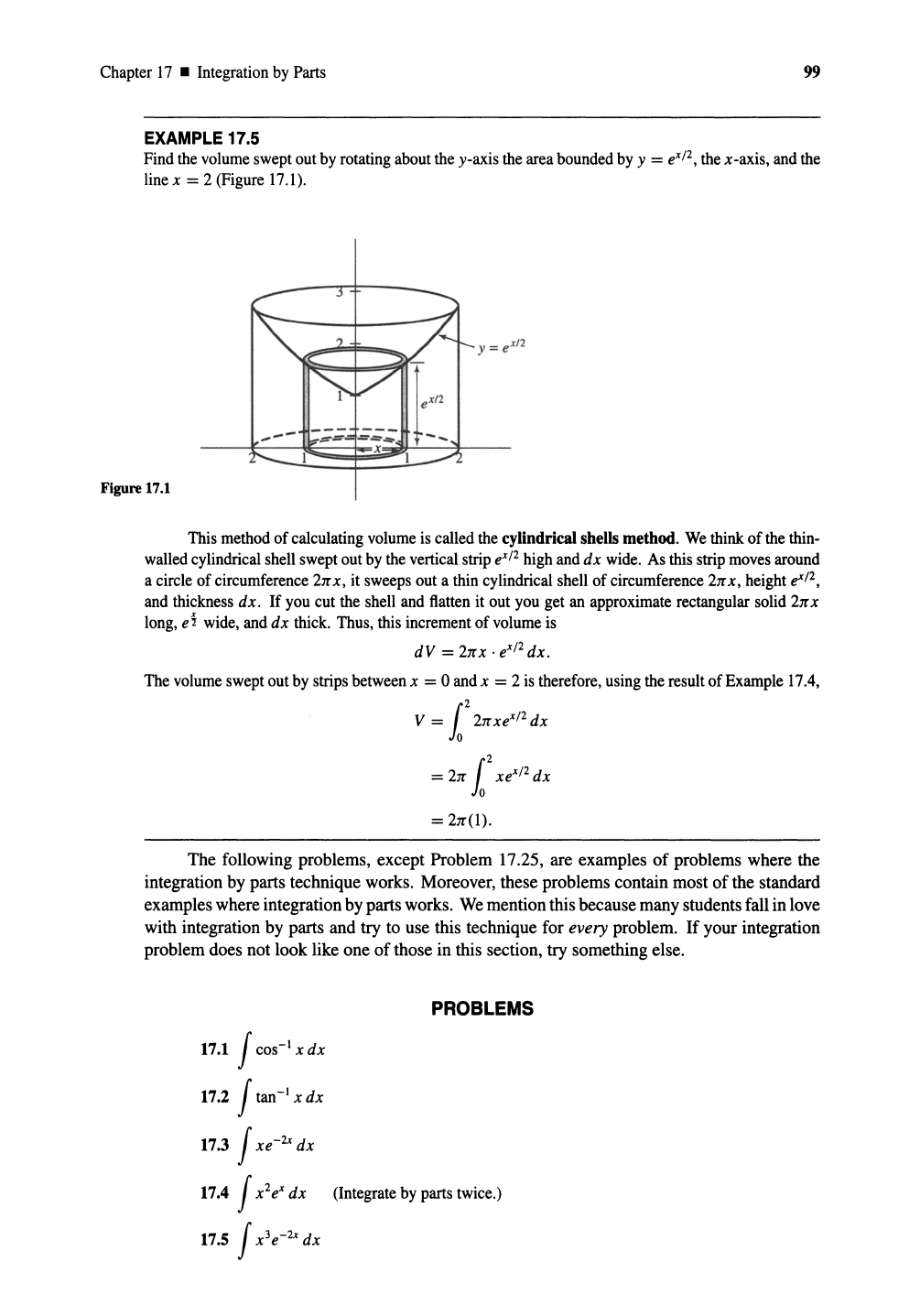
17.5

Findthe volumesweptout by rotatingaboutthe y-axis theareaboundedby y =e

, thex-axis,and the

line

x =2 (Figure 17.1).

Figure 17.1

This methodof calculating volumeis called the cylindrical shells method. Wethinkof the thin-

walledcylindrical shell sweptout by the verticalstrip e

highand dx wide. As this strip

moves

around

a circleof circumference

21f

x, it sweepsout a thin cylindrical shell of circumference

21f

x, heighte

and thickness dx. If you cut the shell and

flatten

it out you get an approximate rectangular solid

21f

long, e2 wide,and dx thick. Thus,this increment of volumeis

=21fx . e

dx .

The volumesweptout by stripsbetweenx =0 andx =2 is therefore, usingthe resultof Example17.4,

V =1

21f

=21f(l).

The following problems, except Problem 17.25, are examples

problems where the

integration by parts technique works. Moreover, these problems contain most

the standard

examples where integration by parts works. We mention this because many students fall in love

with integration by parts and try to use this technique for

every problem.

your integration

problem does not look like one

those in this section, try something else.

PROBLEMS

17.1 fcos"! x dx

17.2

ftan" x dx

17.3

xe-

17.4

dx (Integrate by parts twice.)

17.5 fx

3e-

100

Understanding Calculus

17.6 f

xsinxdx

17.7 fx

cos3xdx

17.8 fx

2sin5xdx

17.9 fx logx dx

17.10 f

.JXlogxdx

17.11

7logx

17.12 f(log

r)?

17.13 f(log r r' dx

17.14 f

xv'T+Xdx

17.15 flogtl

2)dx

17.16 f

sinx dx. (Integrateby parts twice, with u =

each time, and solve the resulting

equationfor the integral.)

17.17 f log(x + 1) dx

17.18 fx

3e-

x 2

17.19 1

cosx

1 f x

2dx

17.20 0 x log(l +x) dx Hint:

is an easy Chapter 16 problem.

x+l

17.21

l\..;x

2dx

17.22

sin-

12xdx

17.23 Usethe cylindrical shellsmethodto findthe volumeobtainedby rotatingaboutthe y-axis

the area in the firstquadrantunder

y = logx, for 1

17.24 Find the volumeobtainedby rotatingabout the y-axis the area in the firstquadrantunder

y =sinx, for 0 s x s I'

17.25 Usethecylindrical shellsmethodto findthe

volume

of thehemisphere obtainedbyrotating

aboutthe y-axis the area under

y = Ja

for 0

a. (This is not an integration

by parts problem.)

17.26 (i) Integrate Jsec' x dx by parts usingu =secx, dv = sec

x dx.

(ii) Use the sameidea to integrateJsec"x dx to get the reductionformulaJsec"x dx =

-L

seen

-2

x tanx +

(n-2)

Jsee

x dx.

n-I

11-1

Trigonometric

Integrals

Integrals involving trigonometric functions arise very frequently, in part because of substitu-

tions that

tum

radical expressions into trigonometric functions. It is therefore necessary to gain

some proficiency in such integrals, and that is our goal in this chapter. We start by reviewing

some familiar integrals:

fsinx

=- cosx;

fcos x

=sinx;

tan x

= f sinx

-log

Icos r] = log Isec x]:

cosx

fsec

tanx.

The following standard trigonometric identities will be used constantly, and should be

memorized:

cos(x + y) = cosx cos y -

sinx

sin y;

cos(x - y) =cos x cos y + sin x sin y;

sin

x + cos

X = 1;

tarr'

x + 1 = sec

sin(x+y)=sinxcosy+cosxsiny;

sin(x - y) = sin x cos y - cos x sin y;

sin

x = (1 -

cos2x)j2;

cos

x = (1 + cos

2x)j2.

(18.1)

(18.2)

(18.3)

(18.4)

(18.5)

(18.6)

101

102

Understanding

Calculus

Wedeal primarily with sinx, cosx, tanx, and secx. Wehave integrationformulasfor

sin

x, cosx, and tan x, and the integralof secx is the following:

secxdx

=log I secx +tanx I· (18.7)

Formula(18.7) can easily be checkedby differentiating the right side:

d secx tanx +sec

- log I secx + tanx

1=------

dx secx +tanx

secx (tanx + sec

=-------

(secx + tan

=secx.

If you put integralsigns in front of the aboveformulasand read from down to up, you get the

usual "derivation"of formula (18.7).

The simpleu-substitution,

u =sinx, du =cosr dx, worksfor any integralof the form

sink

x cosx dx = Iukdu =

_1_

sin

(18.8)

k+l

Here k can be

any

numberexcept

-1;

thatis, k can be positiveor negative, fractionor integer.

The u-substitution of (18.8) is so simple that the intermediate formula

f ukdu need not be

writtendown. The following integralsare examplesof (18.8):

Isin

x cosx dx =

sirr' x;

.Jsinx

cos x dx =

sin~

cosx

1 . 4

dx =

SIn-

sirr' x 4

The same trick works, of course, if the sines and cosines are interchanged; in this case

u = cosx, du = - sinx dx, and we have

cos

xsinxdx

fuk(-l)dU

I_COSk+l

x. (18.9)

k+l

The following are examplesof (18.9):

cos~

x sin x dx =

cos~

COS-2

x sinx dx =

_1_

= secx;

cosx

Icos" x sin x dx =

cos"x.

The following example illustrates a simple variationof the J

sink

x cosx dy integrals.

The trick dependson cos

x occurringto an odd

power.

Isin

x cos

x dx =Isin

x cos

x cos x dx

=Isin

x(l

- sin

x) cos r dx

=fsin

x cos x dx - fsin" x cos x dx

1 . 3

= -

SIn

x - -

SIn

3 5

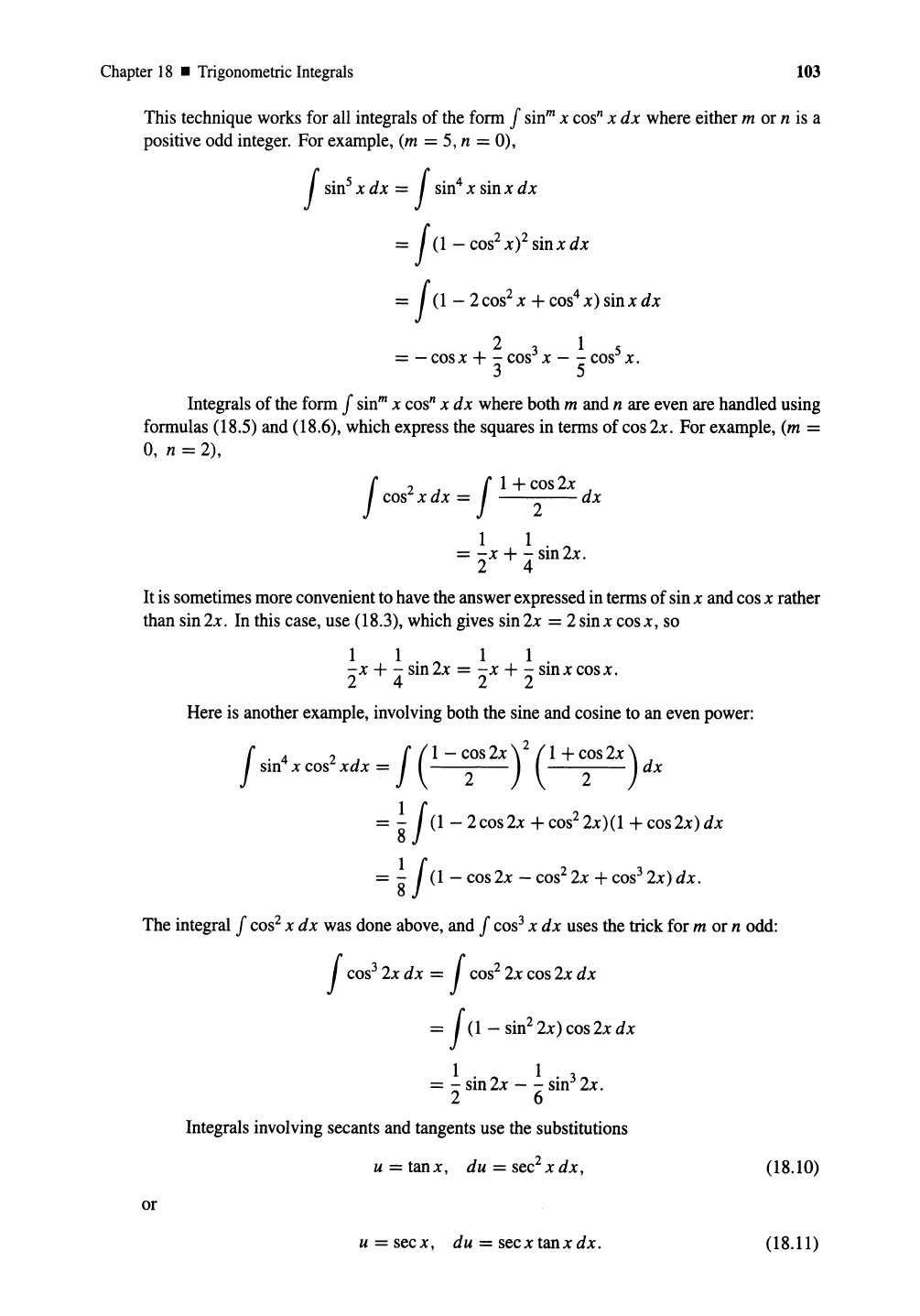
Chapter

18 •

Trigonometric

Integrals

103

This

technique

works

forall

integrals

of the

form

Jsin" x cos"x dx

where

either

m orn is a

positive

odd

integer.

For

example,

(m = 5, n = 0),

fsin

= fsin"x sinx dx

- cos

x)2 sinx dx

- 2cos

X +cos"x) sinx dx

2 3 1 5

= - cosx + - cos x - - cos x.

3 5

Integrals

of the

form

Jsin" x cos"x dx

where

both

and

are

even

are

handled

using

formulas

(18.5)

and

(18.6),

which

express

the

squares

terms

of cos2x.

For

example,

(m =

0, n = 2),

2 d f 1 + cos2x d

cos x x = 2 x

-x

+ - sinZr.

2 4

Itis

sometimes

convenient

have

the

answer

expressed

terms

of sinx

and

cosx

rather

than

sin2x. In

this

case,use (18.3),

which

gives sin2x = 2 sinx cosx, so

1 1 1 1

- x + - sin2x =

-x

+ - sinx cosx.

2 4 2 2

Here

another

example,

involving

both

thesine

and

cosineto aneven

power:

fsin"x cos

xdx

= fC-

~OS2X

YC+

~OS2X)

- 2cos 2x +cos

2x)

+cos

2x)

cos2x - cos

2x + cos''

2x)

The

integral

Jcos

x dx was

done

above,

and

Jcos?x dx usesthe

trick

form orn

odd:

fcos"2x dx = fcos

2x cos

2.x

= f

- sin

2x)

cos2x dx

1 . 2

= - SIn x - - SIn x.

2 6

Integrals

involving

secants

and

tangents

usethe

substitutions

u = tanx, du = sec

x dx,

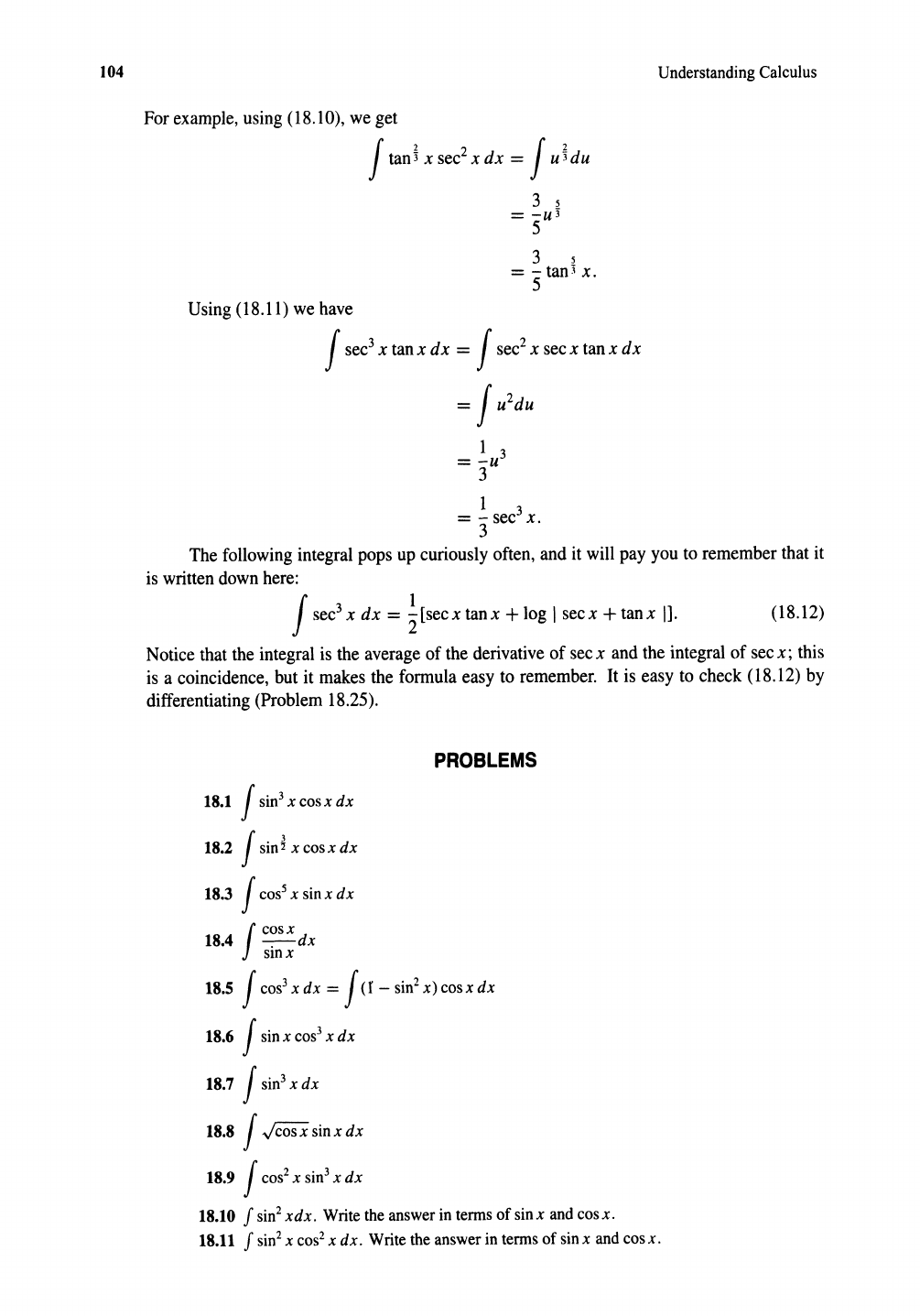
u = secx, du = secx tan x dx.

(18.10)

(18.11)

104

Understanding Calculus

For

example,

using (18.10), we get

ftan

x sec

u~du

3 5

-U

3 5

stan)

Using (18.11)we have

fsec' x tan x

= fsec

x secx tan x

=fu

2du

1 3

-u

1 3

= - sec x.

The following integral pops up curiously often, and it will pay you to remember that it

is writtendownhere:

fsec' x

l[secx

tanx +log I secx +tan x

I].

(18.12)

Notice that the integral is the averageof the derivative of sec

x and the integral of secx; this

is a coincidence, but it makes the formula easy to remember. It is easy to check (18.12) by

differentiating (Problem 18.25).

PROBLEMS

18.1 fsirr' x cos x dx

18.2

sin~

x cosx dx

18.3

fcos"x sinx dx

18.4 f

c~s

x dx

smx

18.5 fcos' x dx =f

- sin

x) cos x dx

18.6

fsinx cos' x dx

18.7

fsirr' x dx

18.8

.Jcosx

sin x dx

18.9 fcos

x sirr' x dx

18.10 f sirr'

xdx.

Write the answer in terms of

sinx

and

cosx.

18.11

Jsirr' x cos?x dx. Write the answer in terms of sinx and cosx.

Chapter 18 • Trigonometric Integrals 105

18.12 fcos"x dx

18.13 fcos"x sirr'x

18.14 fsec

2x dx

18.15 ftarr' x sec

18.16 ftarr' x dx

18.17 fsec" 3x tan 3x dx

18.18

f tan x

secx

18.19 f

tan x sec

18.20 ftan:'

xdx

= f

(sec'

x -

l)tanxdx

18.21

f 1 +

sinx

cosx

18.22 fsec' 4x dx

18.23

f sec 2x

cos

22x

18.24

f tan x

cosx

18.25 Verify formula (17.12) by showing that the derivative of k[sec x tan x +log I sec x +tan x I]

sec'

18.26 (i) Integrate by parts to derive the reduction formula Jsin" x

= - *sin

x cos x +

(n~l)

Jsin

dx.

(ii) Use the formula twice to integrate Jsin" x

dx.

18.27 Find the area under y = sin

x cos? x for 0

18.28 Find the volume obtained by rotating around the x-axis the area under y = sirr' x, 0

::s

18.29 Let x I be the largest negative number where the curves y = cos x, y = sin x intersect, and

let

X2 be the smallest positive number where the curves intersect. Find the area between

y =

cosx

and y =

sinx

for

X2.

18.30 Show that for integers m and n,

sin

cos

= O. Hint: sin

cos

k[sin(m +

n)x

+ sin(m - n)x].

18.31 Show that for all integers n,

2 7f

sirr'

2 7f

cos?

= n.

18.32 Find the indefinite integrals (cf. Problem 18.30).

(i)

Jsin 3x cos 2x

(ii) Jsin x cos 5x

Trigonometric Substitution

Integrands that contain one of the radical expressions Ja

+ x

can

frequently be simplified by making a trigonometric substitution.

For

let x = a sin e, dx = a cos ede.

For Ja

let x = a tan e, dx = a sec

ede.

ForJx

-a

let x =a sect), dx =

asecetanede.

With these substitutions the radicals simplify as follows:

= Ja

tan

()

= a sec 8;

= Ja

sec? e- a

= a tan 8.

(19.1)

(19.2)

(19.3)

(19.4)

(19.5)

(19.6)

In these substitutions we assume that a > 0 and 8 is such that the right sides of (19.4), (19.5),

(19.6) are positive.

EXAMPLE 19.1

Find the area of the top half of the circle x

= 4.

The equation of the top half of the circle is

y = J4 - x

and the area is

- x

2dx.

-2

(19.7)

We make the substitution x

2sin9,

2cos9d9.

When x =

-2,

sin() =

-1

and () =

-~.

107