Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

Chapter 28 • First-Order Linear Equations

171

EXAMPLE

28.6

- + 2y =

5e-

Here y =

ce-

is the general solution of the reduced equation, and to get a particular solution we try

y =

Axe-

Ae-

2Axe-

Substituting these in the equation, we get

(Ae-

2Axe-

)

+2Axe-

=5e-

Ae-

5e-

A =5.

The general solution is

EXAMPLE

28.7

Recall from the last chapter the differential equation for the velocity v in ft/sec of a falling body with air

resistance:

- =

32-kv,

+kv

= 32.

The constant right side, 32, is a zero degree polynomial, so there will be a constant particular solution

y = A. Substituting, we get

+kA

= 32.

Since

= 0, A =

¥,

and the general solution is

v=-+Ce-

With the initial condition v = 0 at t = 0, this gives C = - ¥.

EXAMPLE

28.8

If T is the temperature of a beaker of water at time T and T = 90°C at t = 0, T = 40°C at t = 10

minutes in a room of temperature

25°C, what is T at t = 20 minutes? Assume Newton's law of cooling:

k(T

- 25).

Solution

We write the equation

-kT

-25k.

The coefficient

p(x)

is constant,

-k,

and the right side is constant, so there will be a constant particular

solution

T = A. Substituting gives

-kA

-25k,

or A = 25. Thus, the solution is

T =25 +

ce:".

Since T = 85 at t =0, C =60 and

T = 25 + 60e

-kt

•

Since T = 40 at t = 10,

=25 + 60e-

lOk

_ =

(e-k)1O

172

Understanding CalcuIus

For any

and when t = 15,

T =25 + 60

(~)!

+ 25 + 60

(~)

=32.5.

PROBLEMS

Solvetheseequations:

dy 1

28.1 - +

-y

= 0

dx x

28.2

-+-y=x

dx x

28.3 dx + 2xy = 0

28.4 - +2xy = x

dy x

-+y=e

28.6

+ y =

2e-

(Try

y = Axe-x.)

28.7 - - 3y = 6x + 1

28.8 dy + 2y = 4x

+4x

28.9 -

-6y

= -15s1n3x

28.10 - + y = cos2x

28.11 - + y =

+ cos2x

Hint:

Look at Problems 28.5 and 28.10, and the linearity

condition(28.2).

28.12 dy - 6y =6x - 15sin 3x Hint: Use Problem28.9.

28.13 dy + 3y =5e

28.14 Showthat

+ay = K e

has a solutiony = Ae

unlessb =

-a.

If b =

-a,

there is a

solutionof the form

y = Axe-ax.

28.15 Supposea light body falls under

gravity,

with air resistanceas in Example 28.7, and its

terminalvelocityis 16ft/sec. Howfast is it fallingafter one second?

28.16 If

T =25 +

Ce-

as in Example28.8, and T = 75 whent = 0 and T =65 when t =5

min, what is the temperature at 15min?

Homogeneous

Second-Order

Linear

Equations

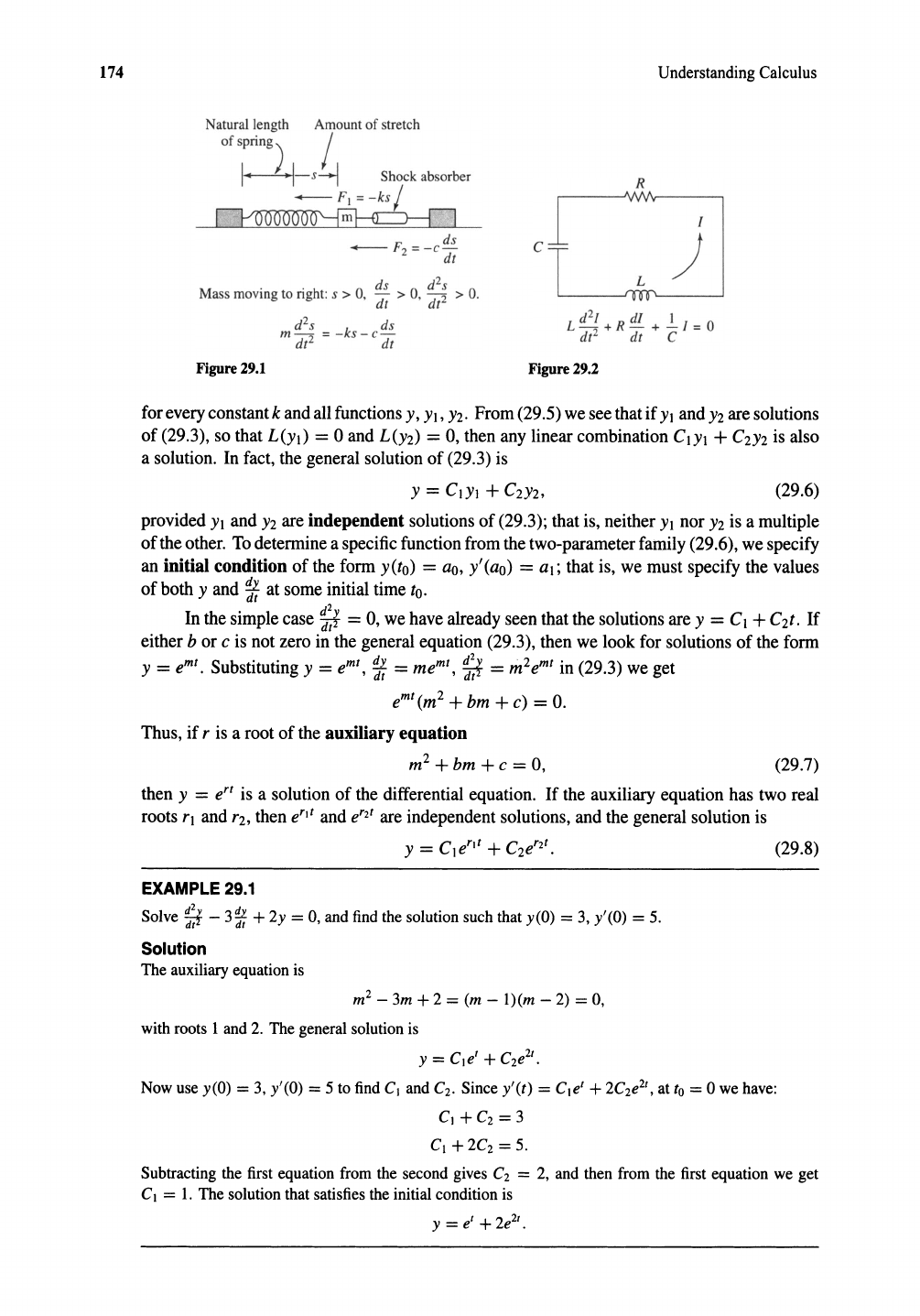
The two common physical situations indicated schematically in Figures 29.1 and 29.2 are

typical applications of the kind of equation we study in this chapter. In Figure 29.1 we show

a mass

m attached to a spring and a damping device that could simply represent friction. The

spring exerts a force

-ks

on the mass, where s is the amount the spring stretched (s > 0) or

compressed

(s < 0), and the damper exerts on the mass a retarding force

-c~

proportional

to the speed. Newton's law says that force is mass times acceleration, so we have the equation

of motion

-ks

-C-,

C ds k

-+--+-s=O.

(29.1)

dt? m

Figure 29.2 represents a simple LCR circuit with an inductance L, a capacitance C, and

a resistance

R. The current I in the circuit is determined by

I R

dt2

+ L dt +

LeI

(29.2)

Both equations (29.1) and (29.2) have the same form,

+b dt + cy = 0, (29.3)

where

band

c are constants. Equation (29.3) is the general form of a homogeneous

linear

second-order

equation

with

constant

coefficients.

If we denote the left side of (29.3) by

L(y);

that is, let

L(y)

= dt

+cy,

(29.4)

then the linearity properties of L are

L(ky)

kL(y)

and L(YI + Y2) = L(YI) + L(Y2)

(29.5)

173

174 Understanding Calculus

L d

[

+ R d[ + l [ = 0

dt C

Natural length Amount of stretch

of Spring)

I--LJ-

Shock absorber

-k

7':1I

OO'""'OO><"><"

o'---fill----Q--

. .

ds 0 d

s 0

Mass movmg to ng t: s > , dt > ' dt

> .

ks-c-

[

)

Figure 29.1

Figure 29.2

for every constant k and all functions y, YI,

. From (29.5) we see that if YI and

are solutions

of (29.3), so that L(YI) =°and L(Y2) =0, then any linear combination CIYI +

C2Y2

is also

a solution. In fact, the general solution

(29.3) is

Y = CIY] +

C2Y2,

(29.6)

provided YI and

are

independent

solutions of (29.3); that is, neither YI nor

is a multiple

of the other. To determine a specific function from the two-parameter family (29.6), we specify

an initial condition of the form y(to) =ao, y' (ao) =

; that is, we must specify the values

of both Y and

at some initial time to.

In the simple case

= 0, we have already seen that the solutions are Y = CI + C2t.

either b or c is not zero in the general equation (29.3), then we look for solutions of the form

Y =e'" , Substituting Y =e'";

=me'",

in (29.3) we get

t(m

+bm +c) =0.

Thus,

if,

is a root of the auxiliary

equation

+bm + c = 0, (29.7)

then Y = e'' is a solution of the differential equation. If the auxiliary equation has two real

roots r, and '2, then e'" and e'" are independent solutions, and the general solution is

Y = CI e

C2erzt.

(29.8)

EXAMPLE 29.1

Solve

+ 2y =0, and find the solution such that yeO) =3, y'(O) =5.

Solution

The auxiliary equation is

3m + 2 = (m -

l)(m

- 2) = 0,

with roots 1 and 2. The general solution is

y =C.e' + C

Now use

yeO)

=3, y' (0) =5 to find C. and C

•

Since y' (r) =C. e' +2C

at to =0 we have:

+2C

=5.

Subtracting the first equation from the second gives C

= 2, and then from the first equation we get

C. = I. The solution that satisfies the initial condition is

y=e'+2e

•

Chapter29 •

Homogeneous

Second-Order Linear

Equations

175

(29.9)

If theauxiliary equation hasonlyonerealroot,', thenit is easyto check

(Problem

29.6)

that

eft

and t

eft

are twoindependent solutions of the differential equation.

EXAMPLE

29.2

Solve

-2

+4-

+4y

dt dt

Solution

Herethe auxiliary

equation

+4m +4 = (m + 2)2 = 0,

with the singleroot r =

-2.

The

functions

and

te-

are

independent

solutions, and the

general

solution is

Therootsof the auxiliary equation for thegeneral equation

(29.3)

are

-b

~b2

- 4c =

± /

(~r

-c.

If band c are both positive, as theyare in the physical situations pictured in Figures

29.1

and

29.2, then both the roots

and

(29.9)

will be

negative,

e'"

and e

f 2t

both

approach

zeroas t

00.

Thisis an

obvious

physical

necessity,

sinceneither

system

has anyexternal

sourceof

energy

and all solutions are necessarily transients.

If the auxiliary equation has

complex

roots,

+ is and r - is, then Yl =

e(f+is)t

and

e(f-is)t

are

complex

valued

solutions of the differential

equation.

The

famous

Euler

formula

statesthat

e(f±is)t

eft

cosst ± i

sinst.

Boththerealandimaginary partsofthis

complex

function

willberealsolutions tothe

differen-

tial

equation.

Thatis, if r ±is are

complex

rootsof the auxiliary equation, thenYl =

eft

cosst

and

eft

sin

stare

independent solutions to the differential equation, and the general

solution is

EXAMPLE 29.3

Solve

-2

+ 13y =

dt dt

Solution

The auxiliary equation is

+4m

+ 13 = 0,

andthequadratic

formula

givesthe roots

-4±JI6-4.13

r ± is = 2

-2

- 13=

-2

± 3i.

Thesolutions are

176

Understanding

Calculus

EXAMPLE 29.4

Solve

-2

+9y =

Solution

The two roots of m

+9 = 0 are 3i and - 3i. Since r =0 and

eOt

= 1, the two solutions are cos 3t and

sin

3t, and the general solution is

y = C1 cos 3t + C

sin 3t.

Any equation of the form

lliy

= 0,

as in Example 29.4, describes what is called simple

harmonic

motion. The general solution

y = C

coswt + C2sin

cor.

By rewriting (29.10) as follows,

JCf

[ C

coswt +

sinwt] ,

JCf+Ci JCf+Ci

letting K = J

ci,

and defininga by

. C

Sltl

= , cos

= ,

we can write the general solution (29.10) in the form

y = K sin(wt +

ex).

(29.10)

(29.11)

This formula exhibits the simple harmonic motion as a sine wave with

amplitude

K and

frequency

2~.

The number

is called the

phase

shift.

EXAMPLE 29.5

The general solution of the simple harmonic motion

+9y =0 can be written either as y = C1 cos 3t +

sin 3t as in Example 29.4 or as y = K sin(3t + a). Find K and

oso

that y(O) = 1, y'(O) = 3. What

is the frequency?

Solution

We have

y(t) =

Ksin(3t

+a),

y(O) =

Ksina,

y'(t) = 3K cos(3t +

a),

y'(O) = 3K cos o.

Therefore, sin a =

y~)

and cos a =

y~~),

sina

= 3y(O) =

= 1,

coso y'(O) 3

4".

Hence,

7C 1

y(O) = 1 = K sin - =

K-,

K=~.

Chapter 29 • Homogeneous Second-Order Linear Equations

Hence, the amplitude, K, is

,J2,and the phase shift,

ex,

The frequency is f;. The solution is

y = J2sin

(3t

PROBLEMS

29.1 Verify that

L(y),

defined in (29.4), has the linearity properties (29.5).

Find the general solutions, as well as the specific solution that satisfies the initial condition.

177

29.2 - + 5- + 6y = 0; y(O) = 1, y' (0) = 5

dt? dt

29.3 dt

- dt - 2y = 0; y(O) = 2, y'(O) = 7

29.4 dt

+ 3dt + 2y = 0; y(O) =

-1,

y'(O) = 1

29.5 2 3y - O' )'(1) = 2e

y'(l)

= 6e

- dt -

29.6 Suppose the auxiliary equation has

just

one root, r, so the equation is (m - r)2 =

Write

the differential equation and verify that

te" is a solution.

29.7 Find the general solution of

+ y =

29.8 Find the general solution of

10*

+ 25y =

29.9 Show that the auxiliary equation for

~~I

- 2A

+ (A

+ B

)y = 0 has complex roots

A ± iB. Check that Yl =

cos Bt and

sin Bt are independent solutions.

Find the general solution and the specific solution that satisfies the given initial conditions.

29.10 dt

- 2 dt + 2y = 0; y(O) = 3, y' (0) = 10

29.11 dt

- 2 dt + 5y = 0; y(O) = 3, y'(O) = 11

29.12 dt

6 dt + lOy = 0; y(O) = 0, y'(O) = 6

Y dy ( n )

1f,

(1f

) n

29.13 dt

4 dt + 20y = 0; y

-e"!,

= -2e"!

29.14 Solve

+4y = 0 and find the frequency of this simple harmonic motion.

29.15 Write the solution of

+4y = 0 in the form y = K sin(wt +

ex).

What is

here? Find

K and

so that y(O) = ,)3, y' (0) = 2.

29.16 If a pendulum has length l feet and () is the angle the pendulum has swung from the vertical

at time

t, then

~()

= 0, where g = 32

ft/sec?

is the acceleration of gravity.

(a) How long should

l be so that the pendulum swings from one side to the other in one

second?

Hint: One cycle would consist of a swing from the extreme right to the extreme

left and back to the extreme right. The frequency in this situation is therefore

! cycle

per second.

(b) With

l the length of part (a), suppose () = 0 when t = 0 (so the phase shift

is zero),

and

= .495 (radians per second, or about 28 degrees/sec) when t =

Find () in

terms of

t. What is the maximum angle () the pendulum makes with the vertical?

Nonhomogeneous

Second-Order Equations

In this chapter, we treat the nonhomogeneous linear second-order equation

+ a

+ by =

q(t),

(30.1)

where

a and b are still constants but

q(t)

is an arbitrary function. In physical terms,

q(t)

could

be an imposed electromotive force in the LCR circuit of the last chapter or an outside force

acting on the spring-mass system.

The reduced form of (30.1) is the homogeneous equation

+a dt +by =

(30.2)

If we let

L(y)

denote the left side of (30.1) or (30.2), then

L(y)

is a linear operator, which

means that

(30.3)

for all constants k and functions

y, YI,

Y2.

From the linearity properties (30.3) it is clear

that if

is any solution of (30.1), so L

(Yo)

= q(t), and YI,

are solutions of (30.2), so

L(YI)

= L(Y2) = 0, then

Y =

CIYI

C2Y2

(30.4)

is a solution of (30.1), since

L(yo + CIYl +

C2Y2)

= L(yo) +

ClL(Yl)

+ C2

L(Y2)

q(t)

+ C

·0+

In fact, (30.4) is the general solution of (30.1) provided YI and

are independent solutions

of (30.2); that is, neither is a multiple of the other.

Recall that for the first-order equation

dx + p(x)y

=q(x)

(30.5)

179

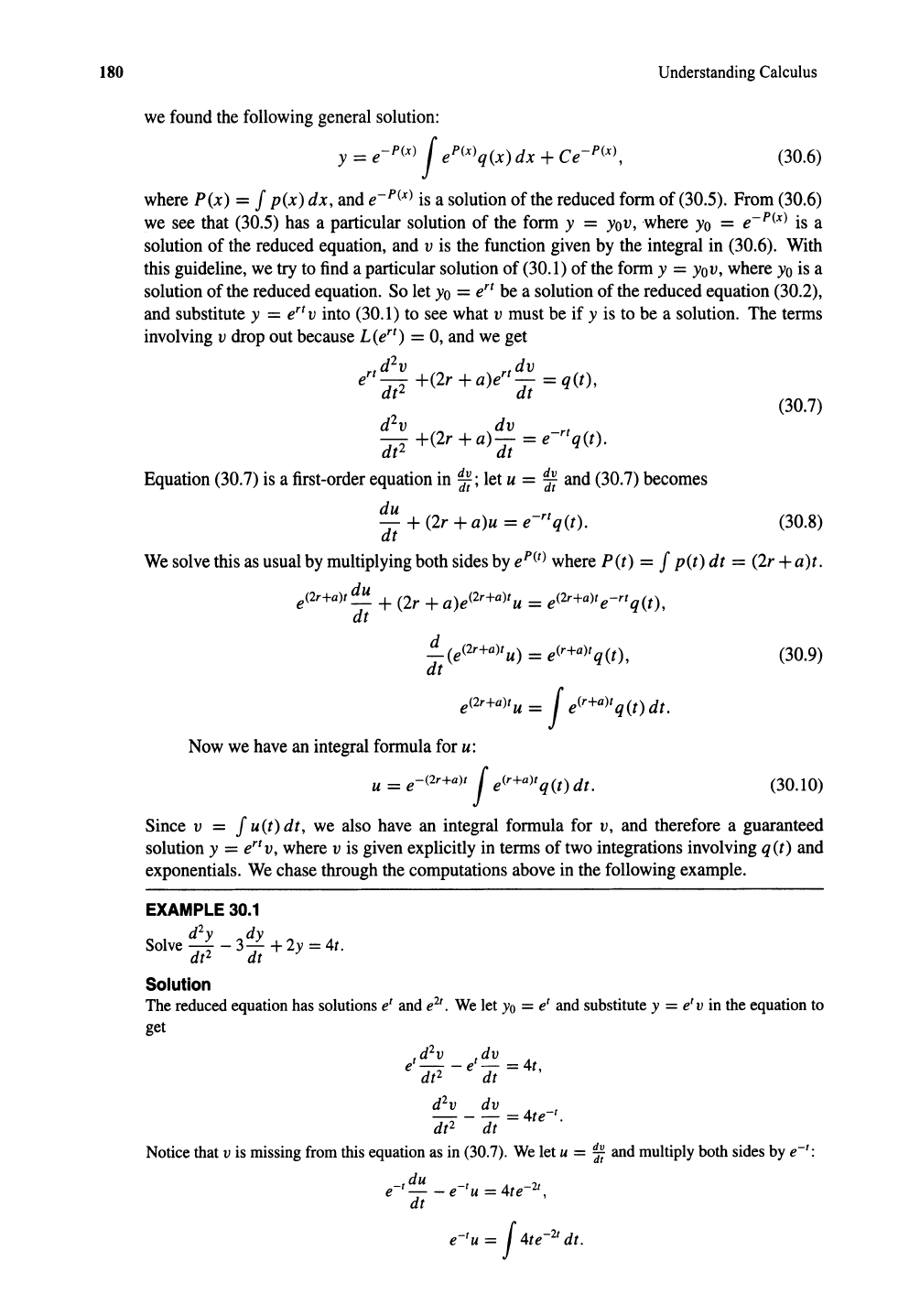
(30.8)

(30.7)

(30.9)

180

Understanding Calculus

we found the following general solution:

y = e-P(x) f

eP(x)q(x)dx

c«:"»,

(30.6)

where

P(x)

p(x)

dx,

and e-P(x) is a solution

the reduced form

(30.5).

From

(30.6)

we see that (30.5) has a particular solution

the form y =

YoV,

·where

= e-P(x) is a

solution

the reduced equation, and v is the function given by the integral in (30.6). With

this guideline, we try to find a particular solution

(30.1)

the form y =

v, where

is a

solution

the reduced equation. So let

= e" be a solution

the reduced equation (30.2),

and substitute

y = e" v into (30.1) to see what v must be if y is to be a solution.

The

terms

involving

v drop out because

Lie")

= 0, and we get

+(2r +

ate"

- =

q(t),

+(2r

+a)

«"

q(t).

Equation (30.7) is a first-order equation in

~~;

let u =

and (30.7) becomes

- + (2r +

a)u

e-rtq(t).

We solve this as usual by multiplying both sides by eP(t) where

P(t)

= J

p(t)

= (2r +

a)t.

e(2r+a)t

_ + (2r + a)e(2r+a)t

e(2r+a)t

e-rt

q(t),

_(e(2r+a)t

=e(r+a)tq(t),

e(2r+a)t

=fe(r+a)tq(t)dt.

Now we have an integral formula for u:

u = e-(2r+a)t f

e(r+a)t

q (t)

dt.

(30.10)

Since v =

Ju(t)dt,

we also have an integral formula for v, and therefore a guaranteed

solution

y =

v, where v is given explicitly in terms

two integrations involving

q(t)

and

exponentials. We chase through the computations above in the following example.

EXAMPLE

30.1

Solve dt

3 dt +2y = 4t.

Solution

The reduced equation has solutions e' and e", We let

= e' and substitute y = e'v in the equation to

get

d 2V

.d»

e--e-=4t,

-t

- - -

=4te

Notice that v is missing from this equation as in (30.7). We let u =

and multiply both sides by e:':

- -

u =

4te-

Chapter30 • Nonhomogeneous Second-Order Equations

Integrateby parts (or consult the table of integrals)to get

e:'

u =

e:"

(-2t

- 1),

u = e-

(- 2t - 1).

Nowintegrateu to get v:

= fe-

t(-2t

l)dt

= e-

t(2t

+3).

This gives the particularsolution

y = e

v = e

•

(2t + 3) = 2t + 3.

181

Noticethatalthough q(t) =t isa verysimplefunction, thecomputations arenevertheless

formidable. Thisprompts ustoinvestigate whatgeneralforma solution wouldhavefor certain

functions

q(t).

For example, if we knew that a polynomial

q(t)

would

always

lead to a

polynomial solution (and it does), then it would be much easierjust to substitute a general

polynomial

+B in the equationto determine the coefficients A and B.

Suppose

q(t)

= e

Qn(t), where Qn(t) is an nth degree polynomial. Here are some

examples of this form of

q(t):

q(t)

= 5e

3t;

Qo(t) = 5, k = 3;

q(t)

= 3t

+ t; Q3(t) = 3t

+ t, k = 0 so e" = 1;

q(t)=2te-

Ql(t)=2t,k=-I.

q(t)

= e

Qn(t), thenthe integral in (30.10) has the form

e{r+a)titQn(t)dt

=fe

Qn(t) dt,

(30.11)

wherec =r +a +k is a constant. Fromthe integral tables(or see Problem 30.1),we findthat

if c

0, then

Qn(t)

Rn(t),

where Rn(t) is anothernth degreepolynomial. Hence(30.9)lookslike this:

e{2r+a)t

= f

e{r+a+k)t

Qn(t) dt

e(r+a+k)t

Rn(t),

fromwhich

(30.12)

(30.13)

u = e(k-r)t Rn(t). (30.14)

The function v is the integral of u, so if k - r

=j:.

v =fe{k-r)tRn(t)

=e{k-r)tSn(t),

(30.15)

for some new nth degree polynomial Sn(t). If k = r, so e

is a solution of the reduced

equation, then

v =f

Rn(t)dt

1(t),

(30.16)

where T

(t)

is a (n + l)st degreepolynomial with no constantterm. Wecan write T

(t)

in the form t S;(r).

Finally,

the solutiony = e" v has the form

y = ertv = erte(k-r)t s,(t) = e

s,(t) (30.17)