Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

150

Understanding Calculus

The equations (25.1)determine

ao,

at,

a2,

...

.a«, so we know what Pn(x) must be:

111

Pn(x) = f(O) + f'(O)x +

2l!"(0)x

3!f

lll

(0)X

+ ... + nlf(n)(O)x

(25.2)

The polynomial (25.2), called the nth

Taylor

polynomial for

f(x)

at 0, is the unique nth

degreepolynomial whosefirstn derivatives at zero matchthose of

f(x).

EXAMPLE

25.1

Find Pn(x) for

f(x)

=e".

f(x)

=e", then f(n)(x) =

for all n, so f(n)(0) = 1 for all n. Therefore,

2 1 3 1 n

P, (x) = 1 + Ix +

-x

...

-x

n 2! 3! n!

(25.3)

EXAMPLE

25.2

Find the general Taylor polynomial for sin x and for cos x.

We calculate the successive derivatives of sin x at x =

This fortunately also gives us the derivatives

of cos

x at 0, since cos x is the first derivative of sin x.

f(x)

sinx,

f(O) =0,

ao =0,

f'(x)

cosx,

f'(O) = 1,

at = 1,

(x) = - sin x, f"(O) =0,

a2 =

2!'

f"'(x)

-cosx,

f"'(O) =

-1,

-1

(25.4)

a3 =

3T'

f(4)(X) =

sinx,

f(4)(0) =0,

a4 =

4!'

j(5)(x)

cosx,

j(5)(0)

= 1,

as = 5!'

The derivatives repeat after n = 4, and the pattern for the derivatives of sin x at °is 0, 1, 0,

-1,

0, 1, 0,

-1

....

Therefore, the Taylor polynomials for sin x are given by

(25.5)

The successive derivatives of cos

x can also be read from the list (25.4); the values at zero are

1,0,

-1,0,1,0,

-1,0,

....

Therefore, the Taylor polynomials for

cosx

are

(25.6)

Nowwe will see whatwe can say about how well Pn(x) agreeswith

f(x)

for

values

x near zero. The graph of PI(x) is just the tangentline to Y =

f(x)

at x =

Howclose the

linearfunction

PI(x) is to f (x) dependson howrapidly

(x) changesas x movesawayfrom

0, and that dependson how large

f"(x)

is. Supposewe havethe estimate

f"(x)

. .

on someinterval[0, a]. If RI (x) =

f(x)

- PI(X), then RI (0) = 0 and

(0) = 0 since PI(X)

has the same valueand slopeas

f(x)

at X =

Moreover,

R~(x)

f"(x),

since P{'(x) =

Chapter25 •

Taylor

Polynomials

Therefore, if °

m s

R~(x)

s M,

mdx

R~(x)dx

M dx,

mx s

Ri(x)

Mx,

151

(25.7)

mxdx

R;(x)dx

Mxdx,

~mx2

RI(x)

~Mx2.

2 - - 2

Since

f(x)

= PI(x) + R

(x), we can conclude from

(25.7)

that

f(x)

lies between two

parabolas overthe interval [0,

a]:

1 1

Pt(x)

+ 2

mx2

::::

f(x)

::::

Pt(x)

+ 2

M x2

If we havean estimateon the sizeof

f"'(x)-say

flll(X)

M on [0,

a]-then

the

argument

above,

withone moreintegration,

shows

that

~mx3

< R

2(x)

~Mx3,

3! - - 3!

and

f(x)

lies betweentwo cubicsover [0, a]:

m M

P2(X)+ 3! x

f(x)

P2(X)

+ 3"!x

•

(25.8)

In general, if m

f(n+1)(x)

M for 0

a, then on this interval

m x

< R (x) < M x

•

(n + I)! - n - (n + I)!

EXAMPLE

25.3

Usethe

fifth-Taylor

polynomial

Ps(x)for e" to

approximate

e!.

Estimate

the

error.

From(25.3)we have

1 1 1 1

P5(X)

= 1+x +

X 2

3"!x

+ Sixs,

fromwhichwe get the

approximation

(

1) 1 1

(1)2

(1)3

(1)4

(1)5

= 1+ - + - - + - - + - - + - - =

1.64870

2 2 2! 2 3! 2 4! 2 5! 2 .

Sincee < 3, e! < 2, and for 0 s x s !' 1 s

~ex

s 2. Therefore,

.ocooz

(~)6

< s,

(~)

(~)6

= 00004

6! 2 2 6! 2 . ,

and

1.64870

accurate

to four

places.

In fact,sincetheerroris

between

.ססoo2

and

.00004,

wecan say

e! =

1.64873

.ooooi.

EXAMPLE

25.4

Use

(x ) for e' to

approximate

x 2

dx,

Since1

3 for 0

1,we

know

thatfor these

values

of x,

1 5 X 3 5

(x ) +

Six

< e < P

(x ) +

Six

(25.9)

152 Understanding Calculus

Since 0

1 if 0

1, we can substitute x

for x in (25.9), and get

p (x

)

+~xlO

< e

x 2

< P (x

) +

~xlO

4 5! 4

5!'

1 P4(X2)dx

+_1_

< 1

2dx

< 1

) dx

_3_.

II·5!

0 0

II·5!

Substituting x

for x in

(x) and integrating, we get an approximation to the integral with error between

11~5!

= .00076 and

11~5!

= .0023. Rounding off, we would have to use .001 and .002 for lower and upper

bounds on the error. Now calculate the integral of

) :

1 P4(x2)dx = 1

~X4

~X6

~x8)

o 0 2! 3! 4!

1 1 1 1

= 1 +

+ 5

·2!

+ 7 . 3! + 9

·4!

= 1.462.

Using the error estimates of .001 and .002, we can finally say that

1.463 <

x 2

dx < 1.464.

If we wanted more accuracy, we could go to

)

and get an error between 13

1.6!

= .0001 and

li6!

.0003 (see Problem 25.10).

As a comparison, Simpson's Rule with

n = 10, and carrying eight places for the arithmetic, gives

1.4626814 for

x 2

dx.

course, we have no way to estimate the accuracy of the Simpson's Rule

number.

The preceding examples involving

are particularly simple since all the derivatives of

are the same, and we considered intervals to the right of

In case we are interested in

negative

x, or the derivatives of f

(x)

have different signs, it is more convenient to use the

following estimate for

R; (x):

lflf(n+l)(x)1

M on

[-a,

a], then IRn(x)1 s (n + I)! Ixl

•

EXAMPLE 25.5

Calculate cos 1 = cos 57.2958° to within .0005.

Let

j (x) = cos x, and notice that for all n, j(n+1) (x) equals ± cos x or ± sin x. Therefore, I

j(n+1)

(x) I

for all

n and all x. Consequently, we have the following estimates:

R (x)1 <

_1_lxl

n - (n + I)!

IRn(I)1

s (n +

I)!'

We find the smallest n such that (nl1)! < .0005:

1 1 1 1

= .16,

= .04,

= .008,

= .0014,

= .0002.

Therefore,

6(1)

will be within .0002 of cos 1. Notice, however, that P

(x )

(x ) since the coefficient

is O. Therefore, R

(1) will also give the difference between P

(1) and cos 1. IR

(1) I

.ססOO2,

and

111

6(1)

= P

7(1)

= 1 - - + - - - = .54028.

2! 4! 6!

Therefore, cos 1

= .54028 ± .00002.

Chapter 25 • TaylorPolynomials

153

We can translate the whole approximation process to any

and use polynomials in

(x - xo) rather than polynomials in x. For example,it makesno sense to try and approximate

log

x with polynomials in x, since log0 is not

defined.

However,

logx and its derivatives are

easy to calculateat

= 1, so it does make sense to use polynomials in

x-I.

In general,the

nth

Taylor polynomial for f (x) at

(x)

= f (xo) +

(xo)(x - xo) + ;,

(xo)(x -

xO)2

1 '" 3 1 (n) n

+ 3!f (xo)(x - xo) +

...

+ n! f (xo)(x - xo) ,

with the usual error estimate:

Ir:1) (x) I

M on [xo - a,

+a], then

for

x in this interval,

R (x)1 < [x

-x

•

n -

(n + I)! 0

EXAMPLE

25.6

Calculate the nth Taylorpolynomialat 1 for logx and find log 1.5to twodecimal places.

Let

f (x) = logx, and calculatethe derivatives at

= 1:

f(x)

=logx,

f(l)

=0,

f'(x)

X-I,

f'(I)

= 1,

f"(x)

-x-

f"(I)

-1,

f"'(x)

2x-

f"'(I)

=2,

f(4)(X) =

-3!x-4,

f(4)(I) =

-3!,

and in general we see that f(n) (1) =

(-1

)n+

(n - I)!. Hence,

1 2 2 3 3! 4

P;(x) = (x - 1) -

(x - 1) +

(x - 1) -

(x - 1)

(_I)n+l(n

- I)! n

+... + (x - 1)

1 2 1 3 1 4

(x·-

1) -

2(x

- 1) +

3(x

- 1) -

4(x

- 1)

(_l)n+l

...

--(x

_1)n.

For x

::::

1, If(n+l)(x)1 =

In!x-(n+1)1

nL Therefore, for x

::::

1, IRn(x)1

(n:

1)! (x - l)n+l. For

x = 1.5,

n(1.5)1

n + 1(.5)n+l.

Wecalculate valuesof

\~~;)I

until we get a value less than .005:

(.W

= .04, (.5)4 = .02,

(.W

= .006,

345

(.5)6 = .003.

Therefore, with an error less than .003,

(

1) 1

(1)2

(1)3

(1)4

(1)5

log 1.5

(1.5) = 2 .2 2 + 3 2 -

2 + 5 2 = .407.

The calculator gives log 1.5

= .405.

154

Understanding

Calculus

PROBLEMS

25.1 Find PI

(x),

(X ) , P

3(x),

and P

4(x)

for

f(x)

=5x

+ 3x - 1.

25.2 Find P

4(x)

for

f(x)

(l~X)'

25.3 Find P

3(x)

for

f(x)

=tan-

25.4 (a) Find P

4(x)

for

f(x)

= sinr

(b) Substitute x

for x in P

(x)

for sinx.

25.5 Find Ps(x) at

= 1for

f(x)

x!.

25.6 Let P

(x ) be the fourth Taylorpolynomial for e", Use

P4(~)

to approximate ,ifi, and

estimatethe error.

25.7 Let P

3(x)

be the thirdTaylor.polynomial for sinx. Evaluate

)

to approximate

sinx

2dx.

Estimatethe error. Noticethat P

3(x)

= P

4(x).

25.8 Let P

(x)

bethefourthTaylorpolynomial forcosx. Evaluate

(-JX)dx

toapproximate

cos-JX

dx.

Estimatethe

error.

25.9 Use Simpson'sRule withn = 10 to approximate

x 2

dx.

(SeeExample25.4).

25.10

Let Ps(x) bethefifthTaylorpolynomial for

on [0, 1]. Calculate

s(x

2)dx

toestimate

x 2

dx and estimatethe error.

Taylor

Series

We have seen that if 1(x) has a power series representation,

I(x)

= ao+atX +

a2x2

a3x3

+ ... +a.x" + ... ,

then 1

(x)

has derivatives of all orders, and the derivatives have power series

f'(x)

+2a2x +

3a3x2

4a4x3

+ .·. ,

f"(x)

2a2

+3 . 2a3x +

4·

3a4x2

+ ... ,

and all these power series have the same radius of convergence. By substituting 0 for x in the

series for

I(x),

I'(x),

I"(x),

and so on, we see that

, 1 "

ao = 1(0),

= 1 (0),

= 2!1 (0),

...

and in general

an =

/(n)

(0). (26.1)

Formula (26.1) shows that the an are determined by the function, so a power series represen-

tation is unique.

1(x) is any function with derivatives of all orders at zero, we can form the power

series with the coefficients (26.1), and the result is the Taylor series

for

1(x) in

powers

I(n)

(O)x

(26.2)

n=O n.

This series does not always converge to f

tx),

For example, if

I(x)

e-;!

for x

=1=

0, and

1(0) = 0, then

I(n)

(0) = 0 for all n. The Taylor series for this function is identically zero, but

the function is obviously positive if

=1=

This function is severely

atypical-all

the common

elementary functions

calculus do have a Taylor series that converges to the function on some

interval.

155

156

Understanding Calculus

We will show that the Taylor series (26.2) converges to f (x) for several elementary

functions. Let

P; (x) be the nth partial sum of the series (26.2):

Pn(x)

/(0)

!'(O)x

!,,(0)x

+ ... +

/(n)(O)x

(26.3)

2! n!

Notice that P;

(x)

is the same as the nth Taylor polynomial for j

(x).

We show that P;

(x)

----*

j(x)

by showing that

Rn(x)

j(x)

Pn(x)

----*

Recall that if

Ij(n+l)(x)1

M on an

interval

[-a,

a], then for these values

R (x)1 < M

Ixn+11

< M a

•

(26.4)

n - (n +I)! - (n + I)!

If all

the derivatives

j(n)

(x)

satisfy the same estimate,

Ij(n)(x)1

M on

[-a,

a]-that

is,

if the bound

M does not depend on

n-then

R; (x) ----* 0 for all x E

[-a,

a], since

----* 0

for any

Considerthe functions sin x and cos x. If j

(x)

= sin x or j

(x)

= cos

then I

j(n)

(x)

1 for all x and all n, so for both sin x and cos x,

Ixl

IRn(x)1

(n +

1)!'

(26.5)

and so

Pn(x)

----*

j(x)

for all x for both

j(x)

= sin x and

j(x)

cosx.

Using the Taylor

polynomials from the last chapter, we see that sin

x has the Taylor series

sin x = x - - + - - - +... (26.6)

3! 5! 7!

and the series converges for all x. Similarly, the Taylor polynomials for cos x converge to

cos

x for all

and cos x has the Taylor series

cosx

= 1 - - + - - -

+...

. (26.7)

2! 4! 6!

EXAMPLE 26.1

Find

cos(~)

to within .00005.

We could use the remainder estimate, but we get the same estimate very simply now that we know the

series (26.7) converges to cos

x. The series (26.7) is a proper alternating series for IxI < 1, so the error

is less than the first term omitted. We calculate several terms at

x =

.13;

.003;

.00002.

Hence,

1 1

(1)2

(1)4

cos 2

1 -

2 +

.87760.

The error will be negative, since the first term omitted is negative, so

.87760 > cos 2 > .87758.

The Taylor polynomials for

are

(x)

= 1 +x + - + - + ... + -

n 2! 3!

n!'

and

(26.8)

Chapter 26 • Taylor Series

157

On any fixedinterval

[-a,

a],

lexi

e",

Ixl

so on

[-a,

a],

eaa

IRn(x)1

::: (n + 1)!

Thus, Pn(x)

on everyinterval

[-a,

a],

and the Taylorseries for

convergesto

for

all

= 1

+ - + - +

...

+ - + ....

2! 3! n!

(26.9)

EXAMPLE

26.2

Find the Taylor series for «'.

Since the series (26.9) converges for all x, we can substitute anything for x in (26.9). In particular,

substituting

for x, we get

-:2 2 x

e' = 1 +x + - + - + - + ... . (26.10)

2! 3! 4!

Since (26.10) is a power series and power series representations are unique, (26.10) is

the power series

for

r',

EXAMPLE

26.3

Find the Taylor series for

.JT+X

and find the radius of convergence.

We calculate the derivatives at 0 and the coefficients

an:

/(x)

= (1 +

1 1

/'(x)

= 2(1 +

X)-1;

/"(x)

= 2

-2

(1 +

X)-1;

(3)

(x)

= 2 - 2 - 2 (1 +

1 . 3 . 5 7

1(4)(x)

-~(1

+X)-1;

In general, we see that

/(0)

= 1;

/'(0)

= -;

/,,(0)

(

-D

;

·3

1"'(0)

23;

·3 ·5

/(4)(0) =

-~;

az =

(-D

(-1

)n+1 1 . 3 .

5·

...

(2n - 3)

n_!_.2_n

and

l(n+l)(X)

= ± 1

·3

·5·

....

(2n - 1)

(l+x)~'

(26.11)

(26.12)

If 0

::::

x < 1, then (1 +

-(2;+ 1)

::::

1, and

1 . 3 . 5·

...

. (2n - 1) Ix In+

IRn(x)1 s 2

(n +

I)!'

(26.13)

For

n = 2, 3, 4,

...

, the coefficients of Ix

In+l

in (26.13) are

1 1

1·3

1~3·5

22 .

2 = .13,

23'

6= .06;

24 = .04;

and so on. The coefficient of

IxI

is obtained by multiplying the coefficient of Ix

In+

2n+l

---=--<1,

2 . (n + 2) 2n +4

IRn(x)1

::s

lx l

where all

::s

= .13 for all n

2. Clearly, Rn(x)

0 if 0

::::

x < 1, and the

Taylor series

for.JT+X

converges to the function on [0, 1). The series therefore converges on

(-1,

1),

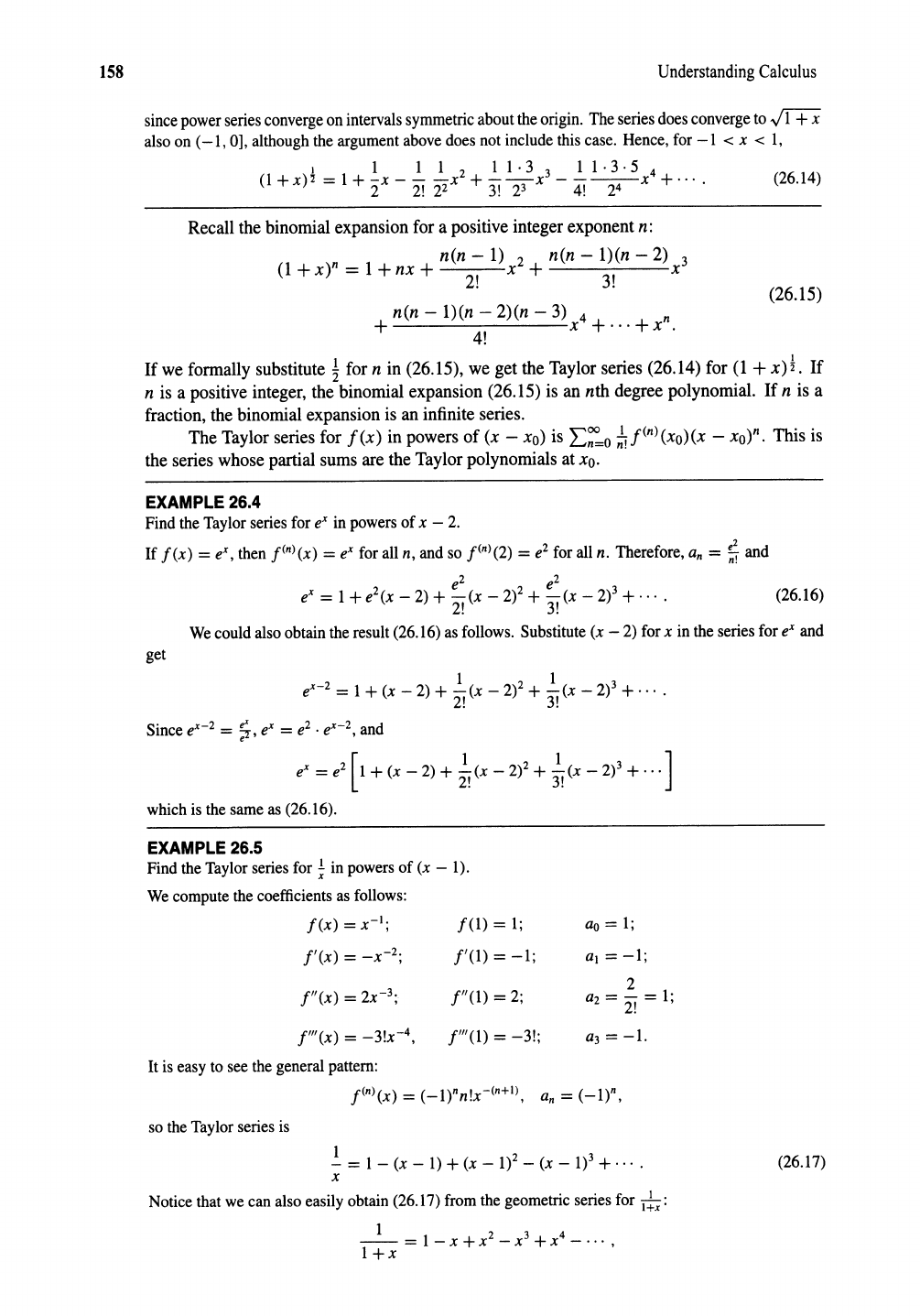
(26.15)

158

Understanding Calculus

sincepowerseriesconvergeon intervalssymmetricabout the origin. The series does convergeto

v'f+X

also on

(-1,

0], although the argument above does not include this case. Hence, for

-1

< x < 1,

1 1 1 1 2

11·3

11·3·5

(l+x)!=1+

2x-2i2

+3Ip

-4"!24x

+....

(26.14)

Recallthe binomialexpansionfor a positiveintegerexponentn:

n(n

- 1) 2

n(n

1)(n

- 2) 3

(1 +x)" = 1+

+ 2! x + 3! x

n(n

1)(n

2)(n

- 3) 4 n

+ X + ... +x .

If we formally substitute 4for n in (26.15), we get the Taylorseries (26.14) for (1 +

x)!.

n is a positive integer, the binomial expansion (26.15) is an nth degree polynomial. If n is a

fraction, the binomialexpansionis an infiniteseries.

The Taylorseries for f

(x)

in powers of (x - xo) is E:o

f(n)

(xo) (x - xo)n. This is

the series whose partial sums are the Taylorpolynomialsat xo.

EXAMPLE

26.4

Find the Taylorseries for

in powers of x - 2.

f(x)

= e", then f(n) (x) =

for all n, and so f(n)(2) = e

for all n. Therefore, an =

and

X 2 e

=1+e(x-2)+2"!(x-2)

+3!(x-2)

+....

(26.16)

Wecould also obtain the result (26.16)as follows. Substitute

(x - 2) for x in the series for

and

get

X 2 1 2 1 3

e - = 1 + (x - 2) + - (x - 2) + - (x - 2)

+....

2! 3!

X 2[ 1 2 1 3 ]

e = e 1 + (x - 2) + - (x - 2) + - (x - 2) + ...

2! 3!

which is the same as (26.16).

EXAMPLE

26.5

Find the Taylor series for

in powers of (x - 1).

Wecompute the coefficientsas follows:

f(x)

X-I;

f'(x)

-x-

1(1)

= 1;

f'(I)

-1;

f"(I)

=2;

ao = 1;

a2 = - = 1;

f"'(x)

-3!x-

f"'(I)

-3!;

a3 =

-1.

It is easy to see the general pattern:

fen)

(x)

= (-I)nn!x-(n+l), an =

(_I)n,

so the Taylor series is

1 2 3

- = 1 - (x - 1) + (x - 1) - (x - 1) + ....

Notice that we can also easily obtain (26.17) from the geometric series for

11x:

234

--=I-x+x

-x

...

l+x

(26.17)

Chapter 26 • Taylor Series

so if

< 1,

1 2 3

---

= 1 - (x - 1) + (x - 1) - (x - 1) +

....

x 1+ (x - 1)

EXAMPLE 26.6

Find the Taylor series at 1 for log x.

We simply integrate the series (26.17):

x 1 1 2 1 3

logx=

-dt=(x-l)--(x-I)

+-(x-l)

....

I t 2 3

EXAMPLE 26.7

Use the series for

sinx

to show that sinx

1 and

--6

as x

x x

Since

sin x = x - - + - - - + ...

3! 5! 7!

we have, for all x

=1=

sinx

-=1--+---+···

x 3! 5! 7! '

so sinx

asx

Also

sinx-x

=~[_X3

+ ... ]

3! 5! 7!

=-3!+5T-

7! +

...

and hence, as x

sinx

- x 1

-3!.

PROBLEMS

159

(26.18)

26.1 Find cos

to within .0005. Do you need more terms for this accuracy or fewer than for

cos

as in Example 26.1?

26.2 Find sin

to within 10-

•

26.3 Evaluate

to within .0002.

26.4 Find five terms (through

x")

of the Taylor series for (1 +

*,and check with the binomial

expansion.

26.5 Use the Taylor series (26.14) for

.JT+X

to obtain the Taylor series for

v"f=-X

and for

v'f+X2.

26.6 Find the Taylor series for

in powers of x - 3 and in powers of x + 1.

26.7 Find the Taylor series for

in powers of (x - 2) two ways:

(i) Calculate

j(n)

(2) for all n;

.. I I n. I

(11)

use - =

-2~.

]+(T)

26.8 Find the Taylor series for log x in powers of x - 2. Hint: log x = log 2 +

fir

so you

can integrate the series in Problem 26.7.

26.9 Use the series for cos x to show that

(COSx~-I)

as x

26.10 For x

=1=

sinx

-=1--+---+···.

x 3! 5! 7!