Basdevant J.-L., Rich J., Spiro M. Fundamentals in Nuclear Physics: From Nuclear Structure to Cosmology
Подождите немного. Документ загружается.

50 1. Basic concepts in nuclear physics
Since in nuclear processes one measures momenta of particles, it is through
the angular distributions of particles that one measures the conservation of
angular momentum.
We note that the infinitesimal generators J
x
,J
y
and J
z
do not commute,
i.e. the group of rotations is non-Abelian. A consequence of this is that the
end result of two successive rotations (about different axes) depends on the
order that the two rotations are performed. This can be confirmed by rotating
macroscopic objects.
The invariances associated with the additive quantum numbers can be
understood by considering transformations generated by one component of
theangularmomentumwhichwetaketobethez component:
D(ϕ)=exp(iϕJ
z
/¯h) . (1.122)
These transformations form an Abelian group.
TheinvarianceofH under rotations around the z axis yields the conserva-
tion of the component J
z
of the angular momentum along this axis. Consider
a set of n subsystems whose state vector is factorized :
|ψ = |α
1
,m
1
⊗|α
2
,m
2
⊗...⊗|α
n
,m
n
. (1.123)
The z component of the angular momentum is
M =
m
i
⇒ J
z
=
i
J
iz
(1.124)
and the rotation operator around the z axis is
D(ϕ)|ψ = e
iMϕ
|ψ . (1.125)
Therefore, M appears as an additive quantum number. The conservation of
M =
m
i
can also be seen as the invariance of the matrix element χ|H
T
|ψ
under the phase transformations :
|ψ→e
iMϕ
|ψ, |χ→e
iM
ϕ
|χ . (1.126)
The group of phase transformations defined in this way is called the unitary
group U(1). It is the rotation group in the plane : x + iy → e
iϕ
(x + iy).
This is how one can represent the conservation of any additive quantum
number. Consider for instance the electric charge Q and a union of subsystems
as in (1.123)
|ψ = |α
1
,q
1
⊗|α
2
,q
2
⊗...⊗|a
n
a
q
(1.127)
whose total charge is Q =
q
i
. Under the phase transformation |ψ→
e
iλQ
|ψ, |χ→e
iλQ
|χ where λ is an arbitrary real number, the invariance
of χ|H|ψ→e
iλ(Q−Q
)
χ|H|ψ implies the conservation of charge : Q = Q
.
This type of invariance is called gauge invariance of the first kind. In 1929,
Hermann Weyl remarked that the choice λ = constant is non-natural from
the point of view of relativity. In fact, space-like separated points are not
related, therefore it doesn’t have much physical meaning to change the phase

1.7 Charge independence and isospin 51
of states in these points in the same way. If λ depends on the point under
consideration : λ(x,t), one deals with a gauge transformation of the second
kind. In this latter formulation, gauge invariance has dynamical consequences
which underly all present theories of fundamental interactions. At present,
the conservation of electric charge appears to be an absolute conservation
law. On the other hand, baryon number conservation is expected to be only
approximately conserved.
We end this discussion by noting that in a more formal approach to quan-
tum mechanics, one can start with invariance principles, next define observ-
ables by making use of the infinitesimal generators of various transforma-
tions, and finally deduce the commutation relations of the observables from
the structure of the invariance groups under consideration (Galileo group,
Poincar´e group, etc.). The proof of the fundamental commutation relation
[x, p
x
]=i¯h starting form (1.118) is an example.
In many cases, the Hamiltonian is invariant under other transformation
groups than translations or rotations. In all cases, the same property will
appear, i.e. a symmetry induces a conservation law. Such properties are very
important in the theories of fundamental interactions and, because of this,
group theory plays a crucial role in elementary particle physics.
1.7 Charge independence and isospin
We argued in Sect. 1.4 that nuclear forces are rather insensitive to the electric
charge. For instance, we have compared the binding energies of
3
Hand
3
He,
whose difference can be understood as originating solely from the Coulomb
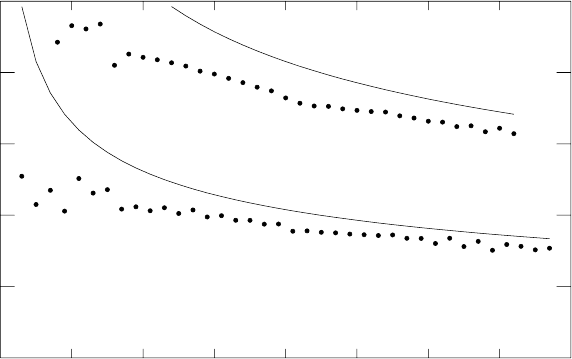
repulsion of the protons. Figure 1.15 shows that this is a systematic effect for
mirror nuclei, i.e. pairs of nuclei for which N and Z are interchanged.
Many other observations confirm charge independence. Spectroscopy pro-
vides many spectacular examples. For example, the spectra of the mirror
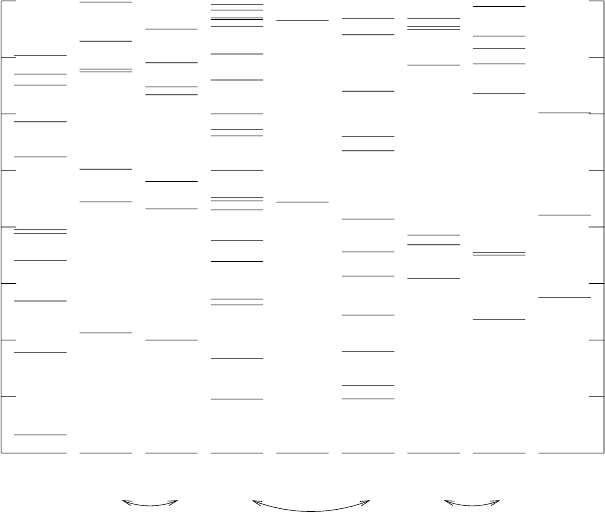
nuclei for A = 11, 12 and 13, shown on Fig. 1.16, are remarkably similar.
1.7.1 Isospin space
Charge independence is more subtle than just saying that protons and neu-
trons can be replaced by one another in nuclear forces. It is formalized by
using the concept of “isotopic spin” or, better, isospin T, that was introduced
by Heisenberg in 1932.
6
A spin 1/2 particle is a two-state system, since a measurement of the
projection of its spin along an axis can lead to one of the two results ±¯h/2.
Similarly, the proton and the neutron can be considered as two different states
T
3
= ±1/2ofasingle physical object of isospin T =1/2, the nucleon. We
6
The translation of Heisenberg’s original paper can be found in D.M. Brink, Nu-
clear Forces.
52 1. Basic concepts in nuclear physics
B/A (MeV)∆
0.00 80.00
0.00
0.50
A
Fig. 1.15. The difference in binding energy per nucleon, B/A, for pairs of “mirror
nuclei”, i.e. pairs with Z and N interchanged. The lower points are pairs with
|Z − N| = 1 and the upper points are pairs with |Z − N| = 2. The lines show the
prediction of the semi-empirical mass formula (2.13) which supposes that neutrons
and protons have identical strong interactions and that the difference in binding
energy comes only from the Coulomb repulsion of the protons.
therefore introduce an abstract three-dimensional Euclidean-like space called
“isospin space.” We will choose operators that allow us to perform rotations
in this space. The spin formalism in isospin space is the same as that for
normal spin in the Euclidean space.
Let T be the vector isospin operator, i.e. a set of three operators
{T
1
,T
2
,T
3
}. These three operators have the commutation relations of a usual
angular momentum, up to the ¯h factor :
T ∧ T = iT . (1.128)
The eigenvalues T (T +1) and T
3
of the commuting observables T
2
and T
3
are the same as those of usual angular momentum (up to ¯h factors).
Heisenberg’s idea is to assume that nuclear strong interactions are rotation
invariant in isospin space. The families of “hadrons”, i.e. particles which
have strong nuclear interactions, can be classified in isospin multiplets ( T =
0, 1/2, 1, 3/2 ...). Different members of each multiplet can be distinguished
by their value of T
3
which is linearly related to their charge.
1.7.2 One-particle states
The nucleon has isospin 1/2. In other words, each of the operators T
1
,T
2
and T
3
which are associated with this particle have eigenvalues ±1/2. The
1.7 Charge independence and isospin 53
11
Be
11
B
11
C
12 12
12
N
13
13
13
O
BC
C
N
mirr
o
r mirr
o
r mirr
o
r
0
8
8
0
4 4
E (MeV)
1/2+
1/2−
3/2−
1/2−
5/2−
3/2−
3/2+
3/2−
1/2−
5/2−
3/2−
3/2+
1+
2+
2−
1−
0+
3−
2+
4−
1+
1−
1−
2−
0+
2+
0+
1+
2+
2−
1−
0+
3−
1+
2−
3−
1−
1−
1/2−
1/2+
5/2+
5/2+
5/2−
1/2−
1/2+
5/2+
3/2+
7/2+
5/2−
3/2+
3/2−
7/2−
1/2+
7/2−
3/2−
3/2−
5/2+
1/2+
5/2+
5/2+
1+
3+
3−
7/2+
3/2+
1−
2−
Fig. 1.16. Spectra of the low-lying levels for nuclei with A = 11, 12, and 13. The
pairs of nuclei with N and Z interchanged (mirror nuclei) have remarkably similar
spectra
operator T
2
= T
2
1
+ T
2
2
+ T
2
3
is proportional to the identity with eigenvalue
3/4.
The states |p and |n,are,bydefinition,theeigenstatesoftheparticular
operator T
3
T
3
|p =(1/2)|p ,T
3
|n =(−1/2)|n . (1.129)
In actual physics, the operator T
3
plays a special role since electric charge
is related to T
3
by
Q = T
3
+1/2 . (1.130)
The action of T
1
and T
2
on these states, with T
±
= T
1
±T
2
, can be written
as
T
+
|p =0 T
−
|n = 0 (1.131)
T
1
|p =(1/2)|n T
1
|n =(1/2)|p (1.132)
T
2
|p =(i/2)|n T
2
|n =(−i/2)|p . (1.133)
An arbitrary nucleon state |N is written

54 1. Basic concepts in nuclear physics
|N = α |p + β |n|α|
2
+ |β|
2
=1 . (1.134)
We remark that all of this is an abstraction applicable only to a world
without electromagnetism. A state such as
1
√
2
(|T
3
=1/2 + |T
3
= −1/2) , (1.135)
which is oriented along the direction T
2
cannot be observed physically. Since
it is a superposition of a proton and a neutron, it is both of charge 0 and 1;
at the same time it creates and doesn’t create an electrostatic field. As such,
it is a superposition of two macroscopically different states, an example of a
“Schr¨odinger cat.”
It is often convenient to use matrix representations for the states and
operators. For the single-nucleon space we have
|p =
1
0
, |n =
0
1
, |N =
α
β
(1.136)
We use the Pauli matrices τ ≡{τ
1
,τ
2
,τ
3
},
τ
1
=
01
10
τ
2
=
0 −i
i 0
τ
3
=
10
0 −1
(1.137)
which satisfy the commutation relations
τ ∧ τ =2i τ . (1.138)
The nucleon isospin operators are:
T =(1/2)τ . (1.139)
Rotation invariance in isospin space amounts to saying that the nucleon–
nucleon interaction is invariant under the transformations
|p
=e
−iφ/2
cos(θ/2)|p+e
iφ/2
sin(θ/2)|n ,
|n
= −e
−iφ/2
sin(θ/2)|p +e
iφ/2
cos(θ/2)|n .
(1.140)
Besides nucleons, there are hundreds of other strongly interacting parti-
cles. These particles, most of which are highly unstable, are called hadrons.
Hadrons are characterized by their isospin, their electric charge, and other
additive more exotic quantum numbers such as “strangeness,” “charm,” etc,
that we will study in Chap. 4. For instance, the π mesons, which mediate some
of the nuclear strong forces, have zero baryon number and form a triplet of
charge states (π
+
,π
0
,π
−
) whose masses are close. It is natural to consider
them as the three states of an isospin triplet, the π meson, of isospin T =1.
Following (1.129) we write
T
3
|π
±
= ±|π
±
,T
3
|π
0
=0 . (1.141)
The relation (1.130) between electric charge and T
3
can be generalized to
Q = T
3
+ Y/2 , (1.142)
where this relation defines the “hypercharge” Y for a multiplet. Clearly we
have Y = 1 for nucleons and Y =0forπ mesons.

1.7 Charge independence and isospin 55
1.7.3 The generalized Pauli principle
The Pauli principle states that two identical fermions must be in an antisym-
metric state. If the proton and the neutron were truly identical particles up
to the projection of their isospin along the axis T
3
, a state of several nucleons
should be completely antisymmetric under the exchange of all variables, in-
cluding isospin variables. If we forget about electromagnetic interactions, and
assume exact invariance under rotations in isospin space, the Pauli principle
is generalized by stating that an A-nucleon system is completely antisymmet-
ric under the exchange of space, spin and isospin variables. This assumption
does not rest on as firm a foundation as the normal Pauli principle and is only
an approximation. However, we can expect that it is a good approximation,
up to electromagnetic effects.
The generalized Pauli principle restricts the number of allowed quantum
states for a system of nucleons. We shall see below how this determines the
allowed states of the deuteron.
1.7.4 Two-nucleon system
The isospin states of a two-nucleon system are constructed in the same man-
ner as the states of two spin 1/2 particles.
The total isospin T of the system corresponds to:
T =1 or T =0
and the four corresponding eigenstates are :
|T =1,T
3
:
⎧
⎨
⎩
|T =1,T
3
=1 = |pp
|T =1,T
3
=0 =(|pn + |np)/
√
2
|T =1,T
3
= −1 = |nn
(1.143)
|T =0,T
3
=0 : |0, 0 =(|pn−|np)/
√
2 . (1.144)
We recall that, just as for spin, the three states |T =1,M are collectively
called the isospin triplet.Theyaresymmetric under the exchange of the
components of the two particles along T
3
. The state |T =0, 0 is called the
isospin singlet state. It is antisymmetric in that exchange. The triplet state
transforms as a vector under rotations in isospin space. The singlet state is
invariant under those rotations.
A series of simple but important consequences follow from these consid-
erations.
• The Hamiltonian of two (or more) nucleons is invariant under rotations
in isospin space so we can expect that the energies of all states in a given
multiplet are equal (neglecting electromagnetic effects). In the two-nucleon
system, rather than three independent Hamiltonians (i.e. one for p−p, one
for p − n and one for n − n), there are only two, one Hamiltonian for the
three T = 1 states and an independent Hamiltonian for the T = 0 state.

56 1. Basic concepts in nuclear physics
• The antisymmetric isospin T =0stateisthestateofthedeuteron,the
only nucleon–nucleon bound state. The deuteron has a symmetric spatial
wavefunction. It is mainly an s–wave.
7
Owing to the generalized Pauli
principle, it must have a symmetric spin state, i.e. S = 1, and a total
angular momentum J =1.
• There are no bound symmetric isospin states (T = 1). The interaction is
only slightly weaker than in the T = 0 state, there exists what is called
technically a “virtual state,” nearly bound.
• Weseewiththisexamplethatchargeindependenceismoresubtlethan
a simple invariance with respect to interchange of neutrons and protons.
Otherwise, we would be sure to observe a neutron–neutron bound state
(possibly unstable under β decay) in addition to the deuteron.
Isospin states of a system of A nucleons are constructed in the same way
as total spin states of A spin-1/2 particles. If a nucleus has isospin T ,we
expect to observe 2T + 1 isobars which have similar physical properties. This
isthecasefortheisobars
11
B
5
and
11
C
6
whose spectra are shown on Fig.
1.16, and which form an isospin 1/2 doublet.
A nucleus (Z, N) has an isospin T at least equal to |N −Z|/2. We expect
to observe at least 2T +1 = |N −Z|+ 1 isobars of different charges, but with
similar nuclear properties.
The electric charge of a system of A nucleons, of total isospin T is, ac-
cording to (1.130),
Q = T
3
+ A/2 .
A is (obviously) the baryon number of the system.
1.7.5 Origin of isospin symmetry; n-p mass difference
The near-equality of the proton and neutron masses is a necessary ingredient
for isospin symmetry to appear. This symmetry can be understood quite
naturally in the context of the quark model where nucleons are states of
three quarks. The proton is a (uud) bound state of two u quarks of charge
2/3 and one d quark of charge -1/3. The neutron is a (udd) bound state, with
two d quarks and one u quark.
Quarks interact according to the laws of “quantum chromodynamics”
or QCD. In this theory, forces are universal in the sense that they make
strictly no distinction between types, or flavors, of quarks involved. The only
difference between the u and d quarks are their masses or charges and we
can expect that the proton and neutron masses differ because of the differing
quark masses and/or from electromagnetic effects.
It is tempting to suppose that isospin is an exact symmetry of strong
nuclear interactions and that electromagnetism is a calculable, and compar-
atively small, correction. In that framework, it would be natural to assume
7
A small d-wave component is necessary in order to explain that the deuteron is
not spherical, as mentioned previously.

1.7 Charge independence and isospin 57
that, in the absence of electromagnetic forces, the proton and neutron masses
should be equal and that their difference originates from calculable electro-
magnetic effects.
We know experimentally that the proton and neutron are extended ob-
jects; as we shall see in Chap. 3, the proton has a radius of the order r ∼ 1fm.
To first approximation, the neutron does not have an electrostatic energy. The
electrostatic energy of the proton is of the order of
E
es
q
2
e
4πε
0
r
1.3MeV , (1.145)
which is indeed very close to the observed value neutron–proton mass differ-
ence, except for the wrong sign!
This argument shows that, unfortunately, within our assumptions, the
proton should be heavier than the neutron. This is a very old problem, and
nobody has ever been able to give an answer, except that there must be an
additional contribution which reverses the sign of this number.
The only way out, at present, is to shift the problem down to quark
masses. Since the proton is a (uud) state and the neutron a (udd) state, we
simply assume that the d-quark mass is larger than the u-quark mass. This is
completely arbitrary. The origin of mass, i.e. quark masses and more generally
all particle masses, is one of the great issues of contemporary physics.
Of course, this ad hoc explanation is not at all satisfactory. We need
an explanation because the sign of the neutron–proton mass difference has
tremendous consequences. If the proton were heavier than the neutron, it
would be unstable either by β-decay
p → ne
+
ν , (1.146)
or by electron capture:
e
−
p → ν n . (1.147)
From the point of view of chemistry and biology, this could have serious
consequences since all existing life relies on the existence of molecules that
contain
1
H which could not be stable if m
p
>m
n
.
The forms of life we know of would only exist if one could replace
1
Hby
deuterium
2
H which is chemically quite similar. As we shall see in Chap. 9,
most of the
1
Hand
2
H in the Universe is a remnant of the nuclear reactions
that occurred in the first three minutes of the Universe. Very little deuterium
was produced (in the actual situation with m
p
<m
n
) and it is difficult to
see how much more would have been produced if the mass hierarchy were
reversed. To summarize the argument, most of the nuclei produced were
1
H
and
4
He. Interchanging the proton and neutron masses would amount to
replacing the
1
H −
4
He mixture by a similar mixture of free stable neutrons
and
4
He. The
2
H necessary for life would have to be produced later (for
instance during the formation of the solar system) via neutron fusion nn →

58 1. Basic concepts in nuclear physics
2
He
−
¯
ν. Compared to the enormous supply of
1
H kindly supplied by the big
bang in the case of m
n
>m
p
, this source of hydrogen seems problematic.
If sufficient hydrogen could not be produced, it is not clear whether an-
other form of chemistry could exist and be rich enough to generate life. At
the very least, it is probable that the absence of hydrogen would considerably
increase the time scale necessary in order to build living systems.
1.8 Deformed nuclei
In our discussion of nuclear radii, we implicitly assumed that nuclei have a
spherical shape. This is a good approximation for nuclei that have “magic
numbers” of neutrons or protons: 8, 20, 28, 50, 82, or 126. We will see in Sect.
2.4, that these numbers come from the shell structure of the nucleus that is
analogous to the shell structure of atomic electrons. Nuclei with magic num-
bers of neutrons or protons have a “closed shell” that encourages a spherical
shape.
prolate Q>0 oblate Q<0
Fig. 1.17. Two charge distributions, one with a positive quadrupole moment Q>0
(prolate) and one with a negative moment Q<0 (oblate). The vertical lines shown
the axis of cylindrical symmetry. Nuclear states with angular momentum quantum
numbers (j, m) will have the symmetry axis oriented randomly according to the
appropriate spherical harmonic, Y
lm
(θ, φ)withl = j. For example, a j = 0 deformed
nucleus has a randomly oriented symmetry axis.
Nuclei with Z or N far from a magic number are generally deformed. The
simplest deformations are so-called quadrupole deformations where the nu-
cleus can take either an prolate shape (rugby ball) or oblate shape (cushion),
as illustrated in Fig. 1.17. A quadrupole deformation retains one symmetry
axis (z axis), and the electric quadrupole is defined by

1.8 Deformed nuclei 59
Q =
1
V
d
3
r(3z
2
− r
2
)ρ(r) , (1.148)
where ρ(r) is the charge density and V is the volume of the nucleus. Spher-
ically symmetric distribution have Q = 0 while rugby balls have Q>0and
cushions have Q<0. For an ellipsoid of uniform density, the relative differ-
ence between the lengths of the major and minor axes, β, is simply related
to the the quadrupole moment:
Q ∼
6
√
5π
ZeR
2
ββ 1 . (1.149)
As shown in Fig. 1.8, deformations of order 10% are common in the ground
states of nuclei far from magic numbers. Excited “super-deformed bands”
with higher deformations are also observed. They are currently an important
topic of research.
It is important to distinguish between the intrinsic deformation of a clas-
sical charge distribution from that of a nucleus in a state of definite angular
momentum quantum numbers, (j, m). In quantum mechanics, the symmetry
axis of a quadrupole cannot be taken to point in a fixed direction. Rather it
has an amplitude to point in any direction that is determined by the appro-
priate spherical harmonic Y
lm
(θ, φ) with j = l. For example, an intrinsically
deformed nucleus with j = 0 has its symmetry axis pointed in a random di-
rection, since Y
00
=1/
√
4π. Thus, this quantum state has a spherically sym-
metric charge distribution in spite of the fact that the nucleus is deformed.
This is analogous to the case of the ground state atomic hydrogen where the
vector pointing from the proton to the electron is randomly oriented.
Nuclear deformation has several physical manifestations that are mostly
related to the non-spherical distribution of electric charge.
• Rapid transitions between nuclear rotation levels of energy (1.40). As we
will discuss more thoroughly in Section 4.2, this can be understood clas-
sically since a spinning spherically symmetric charge distribution does not
create a classical radiation field whereas a spinning asymmetric distribu-
tion does. Thus, bands of rotation states in deformed nuclei like
242
Pu in
Fig. 1.6 exhibit rapid cascades where an excited state decays via a series
of (J +2) → J decays down to the ground (j = 0) state. This results
in a “picket fence” spectrum of evenly spaced lines, as shown in Fig. 1.9
for
152
Dy. More complicated deformations, octopole, hexapole, and so on,
result in more complicated spectra [25].
• The elimination of diffraction minima in electron–nucleus scattering, as
discussed in Sect. 3.4.4. Diffraction minima that are pronounced in spher-
ical nuclei are washed out for deformed nuclei because the orientation of
the nucleus must be averaged over, leading to a “fuzzier” nucleus with no
well-defined surface.
• The hyperfine splitting of the energy levels of atoms and molecules (Ex-
ercise 1.12). As illustrated in Fig. 1.17, this comes about if the nucleus
