ASM Metals HandBook Vol. 8 - Mechanical Testing and Evaluation

Подождите немного. Документ загружается.

objective lens quality and design, optical resolution limits, crosshair technique, and information on the use of

field and aperture diaphragms to control contrast and brightness. The latter are especially important for

translucent or transparent ceramics and glasses. Much of the difference in interpretation of indentation tip

location between observers can be traced to illumination and contrast control.

Although many machines have digital readouts to 0.1 μm (4 μm in.), users should recognize this is less than the

wavelength of light and does not represent the true machine accuracy or machine precision, which are probably

several times larger. In practice, the resolution of the indentation tips and the subjectivity of the viewer usually

leads to a between-observer variability of 0.5 to 1.0 μm in diagonal size. In light of the importance of optical

technique, it is amazing and regrettable that some contemporary commercial hardness machines have no

aperture diaphragm. Some have neither an aperture nor a field diaphragm. Optimum indentation illumination on

ceramics and glasses cannot be achieved with such equipment. The emphasis with some contemporary

machines seems to be on capturing the indentation image with a video camera and projecting the image onto a

monitor and interpreting results with a computer. Computer analysis and controls cannot overcome basic

optical limitations of an instrument. In many instances, a single monitor pixel can represent as much as 0.5 μm

and is a significant fraction of the size of a small ceramic indentation. Of course, whatever system is used to

measure diagonal lengths, it should be verified by use of a calibrated stage micrometer or other magnification

verification device. Ceramic hardness reference blocks with certified indentation sizes may be used to verify

length measurements as well.

With some care and practice, an operator should be able to measure a well-formed ~50 μm Vickers indentation

diagonal size to within 0.5 μm and to within 1.0 μm for a 50 to 100 μm Knoop indentation with 400 to 500×

optical magnification. As previously noted, different observers using the same equipment and viewing the same

indentations typically agree within 0.5 and 1.0 μm (20 and 40 μin.) for Vickers and Knoop indentations,

respectively. Practice with reference blocks can improve this precision considerably.

Knoop Hardness of Ceramics and Glasses. Frederick Knoop developed his elongated pyramidal indenter as an

alternative to the square base pyramidal Vickers indenter, in large part to overcome the cracking observed in

brittle materials (Ref 13, 14). Knoop indentations are far less apt to manifest severe cracking than Vickers

indentations. Several key standards for Knoop hardness of ceramics or glasses are listed in Table 1. The

European Community standard CEN ENV 843-4 has Knoop as well as Vickers and Rockwell A and N hardness

methods. The new ISO 14705 standard from Technical Committee TC 206, Fine Ceramics, includes both

Knoop and Vickers hardness.

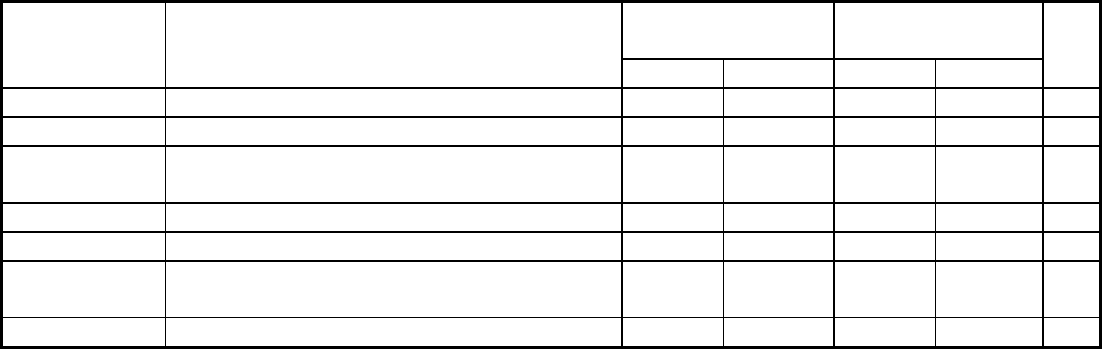
Table 1 Knoop hardness standard test methods for ceramics and glasses

Preferred test

load

Alternate test

load

Standard Materials covered

N kgf N kgf

Ref

ASTM C 730 Glass 0.98 0.1 … … 3

ASTM C 849 Ceramic whitewares 9.8 1 … … 4

ASTM C

1326

Advanced ceramics 19.6 2 9.8 1 5

CEN 843-4 Advanced technical ceramics 9.8 1 … … 16

DIN 52333 Glass and glass ceramics 0.98 0.1

(a)

… … 17

ISO 14705 Fine (advanced, advanced technical)

ceramics

19.6 2 9.8 1 7

ISO 9385 Glass and glass-ceramics 0.98 0.1

(a)

… … 18

(a) At least two other test loads that are not likely to cause excessive fracture shall also be used.

The preferred loads for testing are all within the range of most microindentation hardness testing machines. The

standards usually include provisions for alternative (usually smaller) loads if cracking is a problem. The three

ASTM standards all refer back to the master standard E 384 (Ref 15), “Microindentation Hardness of

Materials,” but each have specific conditions and requirements for ceramics or glasses. For example, the

indenter displacement rate is much slower in C 730 than for the other standards, which reflects some concern

about loading rate effects. In addition, C 730 and C 849 include an important correction factor for optical

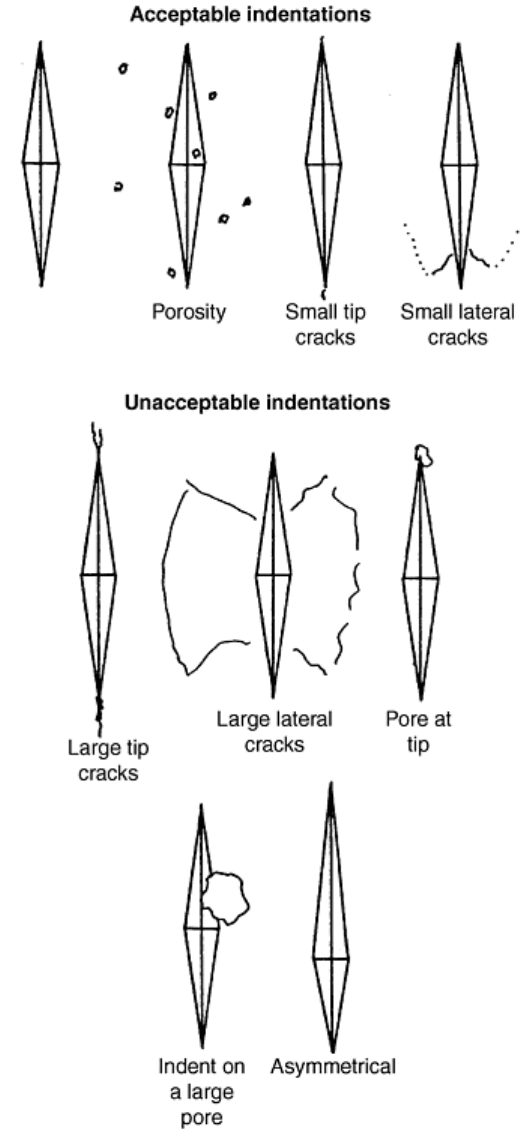
resolution limitations. Figure 5 shows acceptable and unacceptable indentations according to ASTM C 1326

and ISO 14705. The glass standards all recommend 0.98 N (100 gf) as the standard test force. This small load is

used because glasses have low hardness, and so a small test load will produce a moderate-sized indentation.

The small load also avoids cracking. The ceramic standards all prescribe 19.6 or 9.8 N (2 or 1 kgf). The larger

19.6 N (2 kgf) force is advantageous since the larger indentation produces more precise readings, as is

discussed below. The 9.8 or 19.6 N forces may not be sufficiently large to ensure that hardness has reached the

plateau, however. These forces were chosen because they are within the load capacity of most commercial

microindentation hardness machines.

Fig. 5 Acceptable and unacceptable Knoop indentations in ceramics

As noted earlier, it is essential that the test force be reported along with any ceramic hardness number. ISO

14705 requires the use of one of two methods of reporting Knoop hardness. The preferred scheme is SI

compatible and has the symbol HK preceded by the hardness value and supplemented by a number representing

the test force:

15.0 GPa HK 9.807 N

which denotes a Knoop hardness of 15.0 GPa determined with a test force of 9.807 N (1 kgf). Alternately:

1500 HK 1

denotes a Knoop hardness of 1500 (dimensionless) determined with a test force of 9.807 N (1 kgf).

Fortunately, all world standards and the entire ceramics community use the traditional definition of Knoop

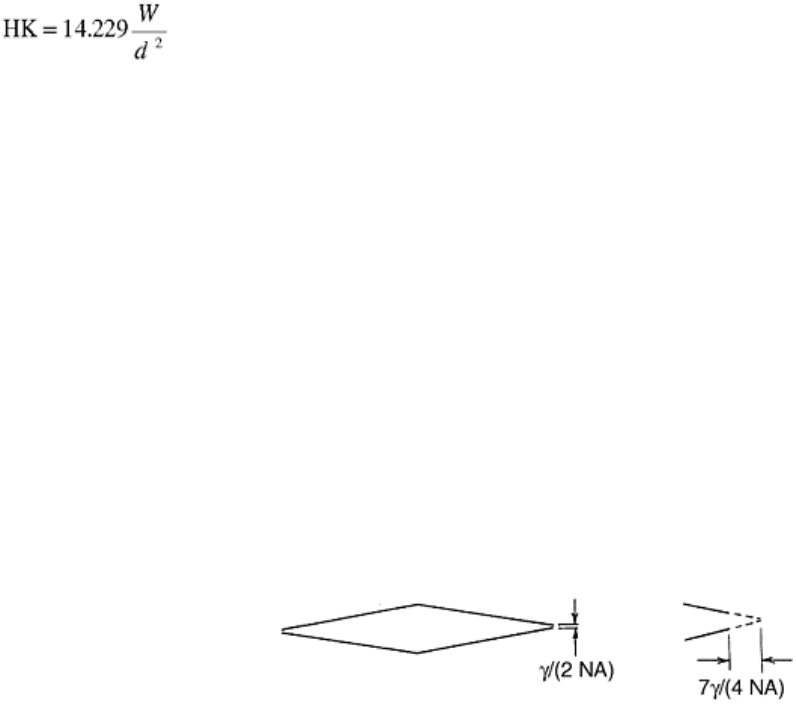
hardness based upon test force divided by projected surface area:

(Eq 1)

where W is the indenter force and d is the long diagonal length.

Knoop indentations are 2.8× longer than Vickers indentations made at the same load. The longer indentations in

principle should make an easier-to-read indentation, but in practice the length advantage is offset by the greater

difficulty in determining where the tapered tip ends. A major advantage of the Knoop indentation over Vickers

for ceramics is that larger indentation loads may be used without cracking. Even if the sides of the indentation

are displaced or cracked, a credible diagonal length reading and hardness estimate may be made. The tip

uncertainty is often of the order of 0.5 to 1.0 μm, irrespective of the indentation size, and consequently the

percentage error is minimized with long indentations. In addition, it is easier to measure hardness in the

constant-hardness region of the indentation size effect curve. Knoop hardness is not fully utilized or appreciated

by the ceramics community.

Optical microscope resolution limitations are a problem for Knoop indentations due to the slender tapered tip

(Fig. 6). The error in underestimating the true tip location has been estimated as 7λ/2(NA) where λ is the

wavelength of light and NA is the objective lens numerical aperture (Ref 12). For a typical microscope having a

40×, 0.65 NA objective lens, the calculated correction for green light (λ = 0.55 μm) is thus 3.0 μm, a significant

number. A correction for this is incorporated in the two older ASTM Knoop standards; C 730 for glass and C

849 for ceramic whitewares, but is not used either in the master microindentation hardness of materials standard

E 384 or in the advanced ceramic standard, C 1326. The confusion about whether to add this correction factor

reached the point where a major glass manufacturer at one time included both uncorrected and corrected

numbers for Knoop hardness numbers in their product data handbook.

Fig. 6 Resolution limits of light microscopy when measuring the long, slender tips of Knoop indents. λ,

wavelength of light; NA, numerical aperture of objective lens

To determine whether the 7λ/2(NA) correction is appropriate, the National Institute of Standards and

Technology (NIST) compared calibrated scanning electron microscope (SEM) diagonal length measurements to

optical microscope measurements on a Knoop impression in a silicon nitride reference block (Ref 19, 20). The

indentation was measured optically by four skilled operators. Three used the optical system on a conventional

microindentation hardness machine, and the fourth used a metallograph used to certify metallic

microindentation hardness standard reference materials (SRMs). The length was measured using several lenses

with different numerical apertures, all calibrated using the same NIST-certified stage micrometer. Five or ten

repetitions were made by each observer. The SEM measurements benefited from higher magnification

photographs (1500×, 5000×), which aided the interpretation of the exact tip location. The mean SEM length

measurement was 146.8 μm (standard deviation ±0.2 μm). The mean optical diagonal lengths were 0.4 to 2.1

μm shorter than the SEM readings. These differences are less than the full 7λ/2(NA) = 3.0 μm correction

probably because the optical observers did discern the tip as a faint black line, albeit not as two distinguishable

tip lines as shown in Fig. 6. Later, as part of an eleven-laboratory international round-robin, three certification

laboratories using their normal optical microscopes obtained average diagonal length readings (10 indentations)

that were within 0.4 to 1.2 μm of the NIST SEM readings. This is better than 1% agreement on 142 μm long

indentations and underscores how the percentage error may be kept small by utilizing large Knoop indentation

sizes. In conclusion, with careful optical microscopy the diagonal length readings for this opaque reference

material were close to the calibrated SEM values, and the 7λ/2(NA) correction factor is excessive.

Knoop indenters sometimes are used to create a controlled surface microflaw in ceramic fracture specimens for

fracture toughness determination by the surface crack in flexure method. The indentation and the residual stress

damage zone underneath it are removed by polishing or hand grinding. The specimen is fractured and fracture

toughness evaluated from the fracture load and the precrack dimensions. This approach has been adopted in

ASTM and ISO standards. Indentation loads are usually 19.6 to 49.0 N (2–5 kgf), which cause no damage to

the diamond indenter (see the article “Fracture Toughness of Ceramics and Ceramic Matrix Composites” in this

Volume).

Vickers Hardness of Ceramics. The square pyramidal Vickers indenter creates smaller, deeper impressions than

Knoop indentations at the same load. Vickers indentations are more apt to crack. There are no Vickers standard

test methods for glasses, undoubtedly due to cracking problems. Table 2 shows some of the current key world

standards and preferred test loads. All standards have settled on a reference test force of 9.8 N (1 kgf), which is

within the capacity of nearly all microindentation hardness testing machines, but probably is not on the

hardness plateau for a hardness versus load curve for many ceramics. Japanese Industrial Standard (JIS) R 1610

and ISO 14705 also allow 98.0 N (10 kgf), but these large loads cause extreme fracturing in ceramics such as

silicon carbide and boron carbide.

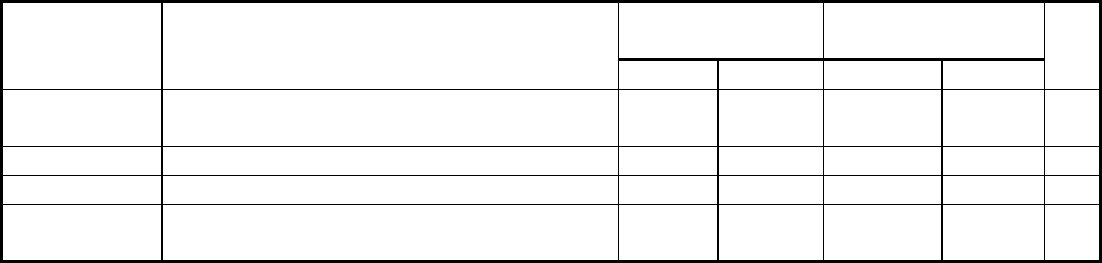
Table 2 Vickers hardness standard test methods for ceramics

Preferred test

load

Alternative test

load

Standard Materials covered

N kgf N kgf

Ref

ASTM C

1327

Advanced ceramics 9.8 1 … …

CEN 843-4 Advanced technical ceramics 9.8 1 … …

JIS R 1610 Fine ceramics 9.8 1 98.0 10

ISO 14705 Fine (advanced, advanced technical)

ceramics

9.8 1 98.0 10 7

The late 1970s and early 1980s saw the advent of indentation fracture studies wherein Vickers indentations

were used to study fracture behavior and, in particular, to estimate fracture toughness. In this approach, a

Vickers indentation is made into a polished surface, and the lengths of the four long cracks that emanate from

the indentation corners are measured. Many workers began to try to measure hardness and fracture toughness at

the same time on the same indentations. Indentation loads were varied in order to adjust the crack sizes. This

frequently led to the use of very large indentation loads, much greater than the 9.8 N (1 kgf) or even the 98 N

(10 kgf) forces recommended in the hardness standards. Often the hardness data were of poor quality. Mangled

indentations and mangled diamond indenters often resulted. Diamond indenters may be damaged by edge

cracking or loss of the meticulously prepared tips, rendering them useless for quality hardness measurements in

the microhardness range.

The optical resolution limits are estimated to be only 1.0λ/2(NA) or ~0.4 μm for Vickers indentations (Ref 12)

with a 40×, 0.65 NA objective lens. Correction factors are rarely applied to the length measurements and are

not incorporated into any Vickers standard. Figure 2 shows a typical well-formed indentation in a silicon nitride

specimen and illustrates the three-dimensional nature of the indentation as well as a modest amount of tip

cracking. There is considerable uplift to the indentation sides. Although the indentation tips are “blunter” and

easier to judge than the slender, tapered Knoop indentations, the Vickers indentation lengths are much shorter.

Consequently, any error in measuring the diagonal size of a Vickers indentation is a larger fraction of the

diagonal size. The hardness uncertainty is similar to or greater than that for Knoop hardness measurements at

the same load. The cracks at the Vickers indentation corners typically are wider and more pronounced and may

interfere with the interpretation of the tip location. In the limit, cracking can be so extensive that portions of the

indentation spall off as shown in Fig. 7, making measurements imprudent or impossible. Figure 8 illustrates

acceptable and unacceptable Vickers indentations according to ASTM C 1327 and ISO 14705.

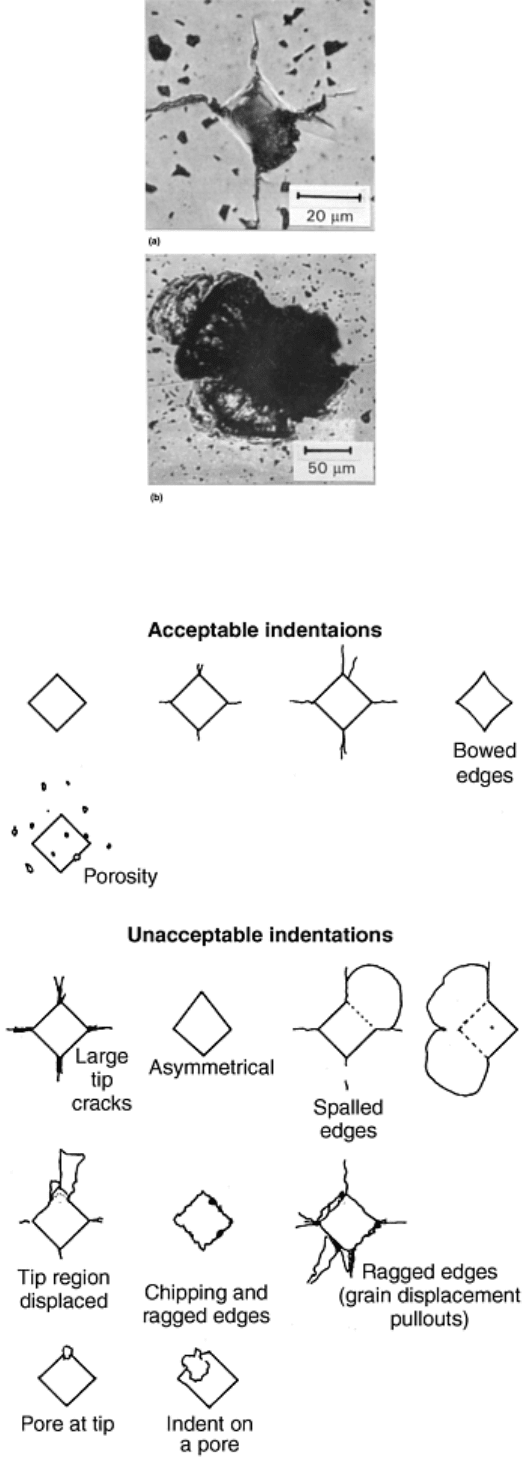
Fig. 7 Badly spalled and fractured Vickers indentation in boron carbide at indentation loads of (a) 9.8 N

(1 kgf) and (b) 98.0 N (10 kgf)

Fig. 8 Acceptable and unacceptable Vickers indentations in ceramics

It is essential that the test force be reported along with any ceramic hardness number. ISO 14705 requires the

use of one of two methods of reporting Vickers hardness. The preferred scheme is to use the symbol HV

preceded by the hardness value and supplemented by a number representing the test force:

15.0 GPa HV 9.807 N

which denotes a Vickers hardness of 15.0 GPa determined with a test force of 9.807 N (1 kgf). Alternately:

1500 HV 1

denotes a Vickers hardness of 1500 (dimensionless) determined with a test force of 9.807 N (1 kgf).

All world standards and most of the ceramics community use the traditional definition of conventional Vickers

hardness based upon test force divided by contact area:

(Eq 2)

where W is the indenter force and d is the long diagonal length. This is the preferred, consensus world standard

formulation for conventional Vickers hardness. Unfortunately, some practitioners in the ceramics community

(those who use Vickers indentations to study fracture, or those who use instrumented or load and depth-sensing

methods) use a formula for hardness (H) based upon test force divided by projected surface area:

(Eq 3)

which is sometimes expressed alternately as

(Eq 4)

where a is the indentation half diagonal size. Many papers or reports do not state which equation was used to

compute a conventional Vickers hardness value or even the indentation load. Hardness values in such cases

should be discounted because they are almost worthless. If the symbol HV is used in reporting hardness, the

odds are very good that the data were generated by a sound test method and hardness calculated by Eq 2.

References cited in this section

3. “Standard Test Method for Knoop Indentation Hardness of Glass,” C 730-98, Annual Book of ASTM

Standards, Vol 15.02, ASTM, 1999

4. “Standard Test Method for Knoop Indentation Hardness of Ceramic Whitewares,” C 849-88, Annual

Book of ASTM Standards, Vol 15.02, ASTM, 1999

5. “Standard Test Method for Knoop Indentation Hardness of Advanced Ceramics,” C 1326-99, Annual

Book of ASTM Standards, Vol 15.01, ASTM, 1999

7. “Fine Ceramics (Advanced Ceramics, Advanced Technical Ceramics), Test Method for Hardness at

Room Temperature,” ISO 14705, International Organization for Standards, Geneva, Switzerland

8. D.M. Butterfield, D.J. Clinton, and R. Morrell, “The VAMAS Hardness Tests Round-Robin on Ceramic

Materials,” Report No. 3, Versailles Advanced Materials and Standards/National Physical Laboratory,

April 1989

9. R. Morrell, D.M. Butterworth, and D.J. Clinton, Results of the VAMAS Ceramics Hardness Round

Robin, Euroceramics, Vol 3, Engineering Ceramics, G. de With, R.A. Terpstra, and R. Metselaar, Ed.,

Elsevier, London, 1989, p 339–345

10. N. Thibault and H. Nyquist, The Measured Knoop Hardness of Hard Substances and Factors Affecting

Its Determination, Trans. ASM, Vol 38, 1947, p 271–330

11. L. Tarasov and N. Thibault, Determination of Knoop Hardness Numbers Independent of Load, Trans.

ASM, Vol 38, 1947, p 331–353

12. B.W. Mott, Micro-Indentation Hardness Testing, Butterworth, London, 1955

13. F. Knoop, C. Peters, and W. Emerson, A Sensitive Pyramidal-Diamond Tool for Indentation

Measurements, J. Res. Nat. Bur. Std., Vol 23, July 1939, p 39–61

14. C.G. Peters and F. Knoop, Resistance of Glass to Indentation, Glass Ind., Vol 20, May 1939, p 174–176

15. “Standard Test Method for Microhardness of Materials,” E 384-89 (1997)e2, Annual Book of ASTM

Standards, Vol 3.01, ASTM, 1999

16. “Advanced Technical Ceramics, Monolithic Ceramics, Mechanical Properties at Room Temperature,

Part 4. Vickers, Knoop and Rockwell Superficial Hardness Tests,” CEN EN 843-4, European

Committee for Standardization, Brussels, 1994

17. “Knoop Hardness Testing, Glass and Glass Ceramic,” DIN 52333, Entwurf German Institute for

Standards, Berlin, 1987

18. “Glass and Glass-Ceramics, Knoop Hardness,” ISO 9385, International Organization for Standards,

Geneva, 1990

19. R.J. Gettings, G.D. Quinn, A.W. Ruff, and L.K. Ives, Development of Ceramic Hardness Reference

Materials, New Horizons for Materials, P. Vincenzini, Ed., Proc. Eighth World Ceramic Congress,

CIMTEC (Florence, Italy), July 1994, Techna, Florence, 1995, p 617–624

20. R.J. Gettings, G.D. Quinn, A.W. Ruff, and L.K. Ives Hardness Standard Reference Materials (SRMs)

for Advanced Ceramics (No. 1194), Proc. Ninth International Symposium on Hardness Testing in

Theory and Practice, (Dusseldorf), Nov 1995, VDI Berichte 1995, p 255–264

21. “Standard Test Method for Vickers Indentation Hardness of Advanced Ceramics,” C 1327–99, Annual

Book of ASTM Standards, Vol 15.01, ASTM, 1999

22. “Testing Method for Vickers Hardness of High Performance Ceramics,” JIS R 1610, Japanese

Standards Association, Tokyo, 1991

Indentation Hardness Testing of Ceramics

G.D. Quinn, Ceramics Division, National Institute of Standards and Technology

Hardness and Microstructure

Ceramic hardness is strongly dependent on the microstructure of the material. Grain size, grain morphology,

porosity, and secondary phases (even in trace amounts), all affect measured hardnesses (Ref 6, 23). The

porosity dependence of hardness may be expressed as:

H = H

exp

-bP

(Eq 5)

where H

is the hardness with zero porosity, P is the volume fraction of porosity, and b is a constant. Values for

b, which range from 3 to 11 depending upon the ceramic, attest to the significant influence of porosity on

hardness. Hardness usually increases with decreasing grain size in accordance with a Hall-Petch relationship,

wherein hardness varies with the inverse square root of grain size (Ref 23, 24, 25, 26). At very large grain sizes,

the trend may change and hardness may increase with increasing grain size and approach single-crystal

hardness values (Ref 23). Examples of the effect of grain size on Vickers hardness are shown by Rice et al. (Ref

23), Clinton and Morrell (Ref 24), and Krell and Blank (Ref 25), and for Knoop hardness by Skrovanek and

Bradt (Ref 26). Just as one cannot generalize and attribute a value of hardness to “steel,” one should not

generalize and assign a value to “alumina.” The more specific one can be about the ceramic (i.e., its code

designation, grain size, and porosity) the better.

References cited in this section

6. I.J. McHolm, Ceramic Hardness, Plenum, 1990

23. R.W. Rice, C.C. Wu, and F. Borchelt, Hardness-Grain Size Relations in Ceramics, J. Am. Ceram. Soc.,

Vol 77 (No. 10), 1994, p 2539–2553

24. D.J. Clinton and R. Morrell, Hardness Testing of Ceramic Materials, Mater. Chem. Phys., Vol 17, 1989,

p 461–473

25. A. Krell and P. Blank, Grain Size Dependence of Hardness in Dense Submicrometer Alumina, J. Am.

Ceram. Soc., Vol 78 (No. 4), 1995, p 1118–1120

26. S.D. Skrovanek and R.C. Bradt, Microhardness of a Fine-Grain-Size Al

, J. Am. Ceram. Soc., Vol 62

(No. 3–4), 1979, p 215–216

Indentation Hardness Testing of Ceramics

G.D. Quinn, Ceramics Division, National Institute of Standards and Technology

Ceramic Hardness Reference Materials

The poor results from a 1988–1989 VAMAS round-robin study (Ref 8, 9) with Knoop, Vickers, and Rockwell

tests on alumina ceramics underscored the need for standard reference materials (SRMs). In response to this

need, NIST subsequently prepared for Knoop hardness NIST SRM 2830, which is a hot isopressed silicon

nitride disk that was prepared from a ceramic bearing ball (Ref 19, 20). It has a high-quality polish with five

well-defined indentations and has a nominal hardness of 14.0 GPa (1400 kgf/mm

). A typical 19.6 N (2 kgf)

impression is shown in Fig. 1. The average diagonal length (~142.0 μm, or 5590 μin.) for each block is listed

and certified to within 0.6 μm (24 μin.) (0.4%) at a 95% confidence interval. The 19.6 N (2 kgf) load is used to

exploit the advantage that long Knoop indentations can reduce the percentage error to such remarkably small

levels. Hardness is certified to within 0.9% or within 0.12 GPa (12 kgf/mm

). A calibrated scanning electron

microscope was used to make all length measurements. An eleven-laboratory international round-robin verified

that operators with conventional optical microscopes obtained readings in excellent agreement with the SEM

readings. This was especially true for the three participating certification labs: NIST's Metallurgy Division,

Wilson Division of Instron, and the Materials Testing Institute, Nordrhein-Westfalen, Germany. The

Fraunhofer Institute for Ceramic Technologies and Sintered Material (IKTS), Dresden, also has prepared

ceramic Knoop hardness reference blocks to support EN 843-4.

NIST SRM 2831 for Vickers hardness was still in preparation as of 2000. It is a tungsten carbide with cobalt

binder with five indentations made at a load of 9.8 N (1 kgf) (Ref 19, 20). A tungsten carbide was chosen since

it is an opaque ceramic (hardmetal) that does not crack at the tips.

References cited in this section

8. D.M. Butterfield, D.J. Clinton, and R. Morrell, “The VAMAS Hardness Tests Round-Robin on Ceramic

Materials,” Report No. 3, Versailles Advanced Materials and Standards/National Physical Laboratory,

April 1989

9. R. Morrell, D.M. Butterworth, and D.J. Clinton, Results of the VAMAS Ceramics Hardness Round

Robin, Euroceramics, Vol 3, Engineering Ceramics, G. de With, R.A. Terpstra, and R. Metselaar, Ed.,

Elsevier, London, 1989, p 339–345

19. R.J. Gettings, G.D. Quinn, A.W. Ruff, and L.K. Ives, Development of Ceramic Hardness Reference

Materials, New Horizons for Materials, P. Vincenzini, Ed., Proc. Eighth World Ceramic Congress,

CIMTEC (Florence, Italy), July 1994, Techna, Florence, 1995, p 617–624

20. R.J. Gettings, G.D. Quinn, A.W. Ruff, and L.K. Ives Hardness Standard Reference Materials (SRMs)

for Advanced Ceramics (No. 1194), Proc. Ninth International Symposium on Hardness Testing in

Theory and Practice, (Dusseldorf), Nov 1995, VDI Berichte 1995, p 255–264

Indentation Hardness Testing of Ceramics

G.D. Quinn, Ceramics Division, National Institute of Standards and Technology

Cracking from Vickers Indentations

Hardness testing usually seeks to avoid the cracking that interferes with the hardness measurement. On the

other hand, the ceramics community has contrived a simple method to estimate fracture toughness (K

) from

Vickers indentation cracking. The indentation crack length or “indentation fracture” method is based on

measurement of the crack lengths emanating from the corners of Vickers hardness indentations on polished

surfaces (Ref 27, 28). The lengths of the cracks and the indentation half diagonal size are related to the

hardness, elastic modulus, and fracture toughness of the material by a semiempirical analytical expression. The

expression inevitably has a calibration constant with considerable uncertainty. The early work on this

methodology claimed that K

calculations were accurate to within 30 to 40%. Despite this uncertainty, the

method is popular because of its seeming simplicity, the need for only one small piece, and the potential to

make repeat measurements.

The mediocre success of the early equations prompted the ceramics community to spawn a plethora of

alternative expressions, to the point that massive confusion now reigns in the ceramics community (Ref 6, 29)

(see, for example, the chapter “Cracked Indents—Friend or Foe” in Ref 6). The failure of a single equation to

apply and the large uncertainty in the calibration constants originate in the complicated, material-specific

deformation-crack patterns and residual stress fields underneath a hardness indentation. The method also suffers

from the drawback that toughness depends on the measured crack length raised to the 1.5 power. The

substantial uncertainties in measuring the crack size (far worse than measuring indentation size) are thus

magnified. Data consistency among laboratories is usually poor due to variations in the interpretation of the

crack length arising from microscopy limitations as well as operator experience or subjectivity. A VAMAS

round-robin demonstrated variability of almost a factor of 2 in reported toughness (Ref 30, 31). The

requirement to obtain cracks lengths that are sufficiently long (>2.0× the half diagonal size) has led some to use

enormous loads (sometimes up to 500 N, or 50 kgf) that cause severe shattering, prompting one skeptical group

to remark that indentations in some materials might resemble “nuclear bomb craters” (Ref 32). This method

may have some utility within a laboratory for research purposes, but experience belies its suitability for

producing accurate fracture toughness results that can be compared between laboratories.

References cited in this section

6. I.J. McHolm, Ceramic Hardness, Plenum, 1990

27. A. Evans and E. Charles, Fracture Toughness Determination by Indentation, J. Am. Ceram. Soc., Vol 59

(No. 7–8), 1976, p 371–372

28. G. Anstis, P. Chantikul, B. Lawn, and D. Marshall, A Critical Evaluation of Indentation Techniques for

Measuring Fracture Toughness: I, Direct Crack Measurements, J. Am. Ceram. Soc., Vol 64 (No. 9),

1981, p 533–538

29. C.B. Ponton and R.D. Rawlings, Dependence of the Vickers Indentation Fracture Toughness on the

Surface Crack Length, Br. Ceram. Trans. J., Vol 88, 1989, p 83–90

30. H. Awaji, T. Yamada, and H. Okuda, Results of the Fracture Toughness Test Round Robin on

Ceramics, VAMAS Project, J. Ceram. Soc. Jpn., Int. Ed., Vol 99 (No. 5), 1991, p 403–408

31. G. Quinn, J. Salem, I. Bar-on, K. Chu, M. Foley, and H. Fang, Fracture Toughness of Advanced

Ceramics at Room Temperature, J. Res. NIST, Vol 97 (No. 5), Sept–Oct 1992, p 579–607

32. Z. Li, A. Ghosh, A. Kobayashi, and R. Bradt, Reply to Comment on Indentation Fracture Toughness of

Sintered Silicon Carbide in the Palmqvist Crack Regime, J. Am. Ceram. Soc., Vol 74 (No. 4), 1991, p

889–890

Indentation Hardness Testing of Ceramics

G.D. Quinn, Ceramics Division, National Institute of Standards and Technology

Instrumented Hardness Testing

Instrumented hardness testing, wherein displacement is monitored during load application, is an important

emerging technology. Load displacements typically appear as shown in Fig. 9, but test cycles with partial

unloading and reloading are often used. Vickers or Berkovich (triangular pyramid) indenters may be used. The

Berkovich indenter avoids the flat, or “chisel tip” that inevitably exists in Vickers pyramids in which four

surfaces or edges meet almost (but not quite) at a point. Tip shape is crucial in instrumented hardness tests at

low loads. Various indices of hardness may be deduced from the load and depth of penetration of the indenter,

and, if it is assumed that the diamond indenter does not change shape, it is even possible to compute a

conventional HV hardness. An enormous advantage of this methodology is that it obviates the need for

microscopy for measuring the indentation size, thereby eliminating operator skill or subjectivity and

microscopy limitations. On the other hand, complications arise because of the need to make assumptions about

the analytical form of the load-displacement curves. Unloading curves may be analyzed to determine the elastic

modulus, but again assumptions about the indenter shape and penetration geometries must be made. There is no

consensus on the interpretation of these curves. For example, one commercial apparatus that is widely used in

Europe to measure a so-called “universal hardness” actually measures a hardness defined as the load divided by

assumed contact area while the load is still applied. Consequently, this universal hardness includes both plastic

and elastic deformation components. Another problem common to all instrumented hardness testers is the

uncertainty associated with determining the exact initial contact point.